Trans. Nonferrous Met. Soc. China 25(2015) 3399-3409
CFD-supported optimization of flow distribution in quench tank for heat treatment of A357 alloy large complicated components
Xia-wei YANG1, Jing-chuan ZHU2, Wen-ya LI1
1. State Key Laboratory of Solidification Processing, Shaanxi Key Laboratory of Friction Welding Technologies, Northwestern Polytechnical University, Xi’an 710072, China;
2. School of Materials Science and Engineering, Harbin Institute of Technology, Harbin 150001, China
Received 9 December 2014; accepted 4 May 2015
Abstract: The flow distribution in quench tank for heat treatment of A357 alloy large complicated components was simulated using FLUENT computational fluid dynamics (CFD) software. The flow velocity and the uniformity of flow field in two types of quench tanks (with or without agitation system) were calculated. The results show that the flow field in the quench tank without agitation system has not evident regularity. While as for the quench tank with agitation system, the flow fields in different parameters have certain regularity. The agitation tanks have a distinct advantage over the system without agitation. Proper process parameters were also obtained. Finally, the tank model established in this work was testified by an example from publication. This model with high accuracy is able to optimize the tank structures and can be helpful for industrial production and theoretical investigation in the fields of heat treatment of large complicated components.
Key words: A357 alloy; flow distribution; quench tank; computational fluid dynamics (CFD) simulation
1 Introduction
A357 (Al-7Si-0.6Mg) alloy has been widely applied in aerospace industries, automotive applications and other aspects due to its good corrosion resistance, excellent castability and high specific strength in the heat-treated condition [1-3]. Many large thin walled complicated components (e.g., frame, wall panel and thin walled beam) used in aeronautic and astronautic industries have been cast using this alloy [4]. In order to obtain an adequate mechanical property, the Al-Si-Mg cast alloys are generally heat-treated to the T6 state (solution heat treatment, quenching and artificial aging) [5,6]. As for A357 alloy large complicated components, inhomogeneous distortion of the large complicated components occurs due to the stresses and strains caused by quenching treatment. Enough quench speed must be provided so as to inhibit the formation of Mg-Si precipitates of A357 alloy [7]. Therefore, for the large complicated components, suitable flow velocity should afford in the quench tank. But an increase in quench speed will lead to large residual stresses for quenched components. Good control of quench process for A357 alloy large complicated components will obtain better result of solid solution treatment and will be helpful to control the part distortion [8]. Therefore, it is very important to optimize the flow uniformity in quench zone and to predict the flow rate of quenching medium.
Computational fluid dynamics (CFD) is widely used for calculating the flow velocity and uniformity of quenching medium in quench tank [9]. CFD can be used to solve the complex problems of fluid flow and predict the performance of fluid-thermal process that is often too difficult to be solved with experimental or analytical techniques [10]. The non-uniformity of medium flow in quench tank was investigated by using the CFD modeling [11,12]. Using the CFD analysis, HALVA and VOLNY [13] investigated the relationship between the homogeneity of fluid flow and agitator placement. GARWOOD et al [14] researched the fluid flow in an agitated quench tank by using the CFD code and the experiments. The effect of the placement of submerged spray educators on quench non-uniformity was researched by CFD method [15].
As shown in the above, many researchers used the high fidelity CFD to investigate the medium flow distribution in the quench tank. But there are a few reports about the investigation of medium flow in the quench tank with different structures. In the present study, two types quench tanks (with or without agitator system) were selected as the objects. FLUENT CFD software was selected as a simulation tool. The influence of various parameters on flow field in the tank was studied. The correctness of the quench tank model established was testified by a numerical example from a publication.
2 Typical large complicated component
Figure 1 shows three-dimensional model of A357 alloy large complicated thin-wall component. As can be seen in Fig. 1, the structural characteristics of this large complicated component are as follows: four visual windows, distribution of strengthening bosses in the inside wall of the component, strengthening plates along radial and axial directions, and four grooves on the bottom end side. The schematic of thin-wall component with dimensions is shown in Fig. 1(c). The height, the wall thickness, the diameter of bottom face and the cone angle of this component are 700 mm, 8 mm, 600 mm and 3°, respectively.
3 Modeling of quench tank
3.1 Modeling of quench tank without agitation system
Figure 2 shows the sketch maps of heating furnace (Fig. 2(a)) and quench tank without agitation system (Fig. 2(b)) for heat treatment of aluminum alloy large component. The heating furnace is a cuboid with dimensions of 3.2 m × 2.2 m × 2.4 m. The quench tank is a cuboid with dimensions of 2.5 m × 2.5 m × 3 m. The inlet diameter is 0.2 m, and the outlet is overflow. As shown in Fig. 2(a), the heating furnace includes furnace chamber, two hooks, heating system, large basket and baffle plate. As shown in Fig. 2(b), the quench tank includes inlet, outlet, distributary nozzles, overflow channel, top cover and base plate. During the heating process, the aluminum component is located in large basket. After the heat preservation, the component quickly immerses into the quench tank. The inlet velocities are set as 0.2, 0.4, 0.6, 0.8 and 1 m/s, respectively. The number of distributary nozzles is 5.
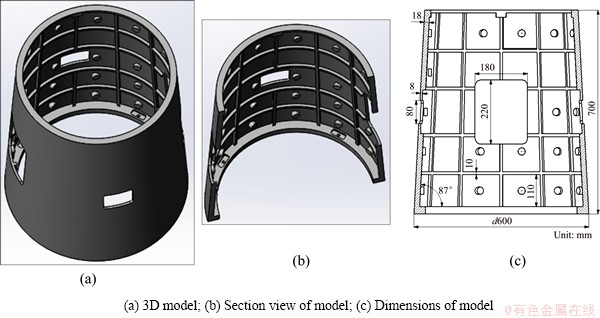
Fig. 1 Typical A357 alloy large complicated component
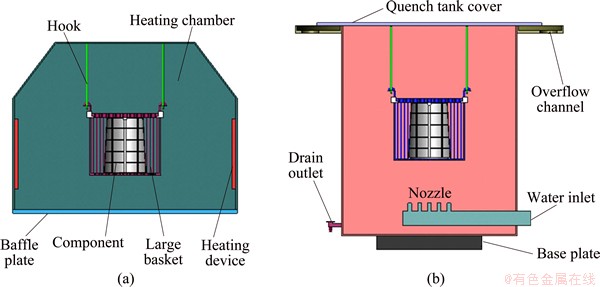
Fig. 2 Heating furnace (a) and quench tank (b) of aluminum alloy large complicated component
FLUENT software can use triangle, quadrilateral, tetrahedral, hexahedral and hybrid meshes. The mesh can be adaptively adjusted during the calculation process. Dimensions, mesh and boundary conditions of quench tank model are shown in Fig. 3. The inlet tube is located at the bottom of the quench tank, so as not to stir the iron filings and other contaminants deposited at the bottom of the quench tank, and enough distance between inlet tube bottom and the quench tank must be set. Here, the distance is set to be 0.15 m, as shown in Fig. 3(a). The meshing types selected are Tet/Hybrid type (Tgrid type), Hex/Wedge type (Cooper type) and Hex type (Map type), as shown in Fig. 3(b).
3.2 Modeling of quench tank with agitation system
The quench tank with agitation system consists of two agitators, two draft tubes, two impellers, two overflow baffles and two directional flow baffles, as shown in Fig. 4(a). It is 2.5 m in length, 2.5 m in width and 3 m in height. The quench zone is a cuboid with 1 m × 1 m × 1 m. The quench tank has a vertical symmetry plane and two vertical marine propellers that rotate in the same direction. The simplified model is shown in Figs. 4(b) and (c). The structural characteristics of impeller are diameter of 450 mm, blade number of 3 and pitch setting of 65 mm. The dimensions of draft tube and flow baffle are also shown in Fig. 4(d). Tet/Hybrid elements, Tgrid type and interval size of 0.05 were created. The total nodes and total elements are 67926 and 365666, respectively. The interval sizes of 0.04 (total nodes 3573 and total elements 16136) were selected for draft tube because this part is more complex than other tank parts. Figure 5 shows the mesh of simulation model.
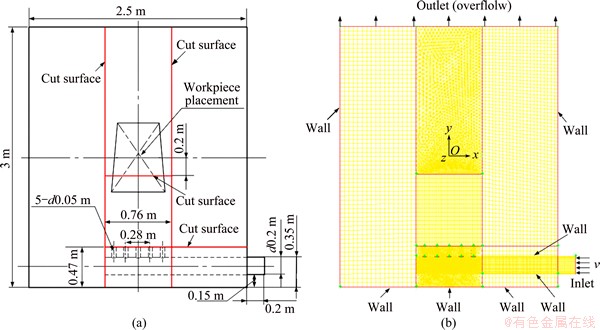
Fig. 3 Dimensions (a), mesh and boundary condition (b) of quench tank
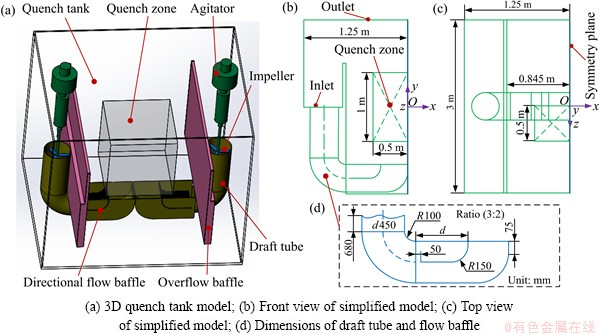
Fig. 4 Structures of quench tank with agitation system
Fig. 5 Mesh diagram of quench tank
Water is selected as the quenching medium. The density and viscosity of water at 25 °C are 997.04 kg/m3 and 8.904×10-4 Pa·s, respectively. They are set as constant during simulation. Continuum hypothesis and nonslip condition at walls are applicable. The forward propulsion force caused by impeller agitation is only considered, so the rotational force can be ignored. x-velocity (vx) and z-velocity (vz) of the inlet are all equal to zero. y-velocity (vy) of the inlet can be calculated using impeller parameters, which include impeller diameter (d450 mm), pitch setting (p=65 mm), rotational speed (n) and number of blades (N=3). When n values are 300, 450, 600, 750, 900, 1050 and 1200 r/min, vy values are -0.975, -1.463, -1.95, -2.438, -2.925, -3.413 and -3.9 m/s, respectively. The pressure is set as 1.013×105 Pa at the outlet. A semi-empirical model, standard k-Epsilon turbulence model, based on the turbulent kinetic energy equation and the dissipation rate equation is selected for FLUENT simulation.
4 Results and discussion
4.1 Flow simulation of quench tank without agitation system
Figure 6 shows the influence of inlet velocity on y-component flow velocity of water. As shown in Fig. 6, different inlet velocities can afford different flow velocities of water in the quench zone. The water flow velocity in the quenching zone increases with increasing inlet velocity, but the flow filed uniformity does not show an evident regularity. The position of each distributary nozzle is shown in Fig. 6(a). As shown in Fig. 6(a), when the inlet velocity is 0.2 m/s, the first distributary nozzle affords higher flow velocity than other nozzles. As shown in Fig. 6(b), when the inlet velocity is 0.4 m/s, the second distributary nozzle affords higher flow velocity than others. As shown in Fig. 6(c), when the inlet velocity is 0.6 m/s, the third and the forth distributary nozzles afford higher flow velocity than others. So, the rule of the flow velocity changing with the inlet velocity does not show an evident regularity. In order to thoroughly investigate the effect of inlet velocity on the flow velocity of quenching medium, the influences of two paths on y-component flow rate were studied (Fig. (7)). It can be seen from Figs. 7(b) and (c) that the flow field distribution presents fluctuation. The flow velocity is higher in middle zone of quench tank than in other zones, but there is no evident rule for the appearance of the curve peak position.
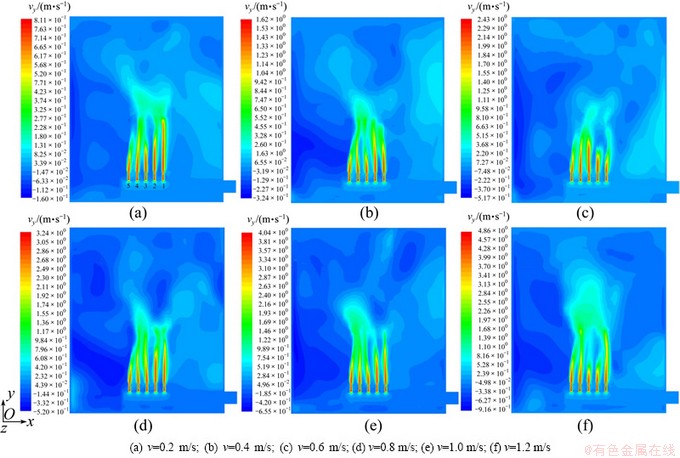
Fig. 6 Influence of inlet velocity (v) on y-component flow velocity (vy) of water
Therefore, as for the quench tank without agitation system, although the water flow rate of quench tank without agitation system can be adjusted by changing the inlet velocity, the appearance of the position of the maximum of flow rate has no evident regularity.
4.2 Flow simulation of quench tank with agitation system
4.2.1 Effect of shape of draft tubes and directional flow baffles on flow field
Figure 8 shows the effect of the shape of the draft tubes and the directional flow baffles on flow field. As shown in Fig. 8(a), at Position 1 of the draft tube, round and square corners are modeled, respectively. In addition the conditions of Position 3 with or without the directional flow baffles were modeled, respectively. The quenching medium in quench tank is water at room temperature. The inlet is set as 2 m/s. As shown in Fig. 8, when the draft tube has round corners in Positions 1 and 2 (Figs. 8(d) and (e)), the water flow streamline in the tank is better than that of the draft tube with square corners (Figs. 8(a) and (b)). The direction of the flow streamline is changed and the flow field uniformity is improved due to the setting of the directional flow baffles (Fig. 8(e)). In addition, the distance between the speed spread center and the outlet of draft tube was also investigated. The distance in Fig. 8(b) is represented by l1, in Fig. 8(c), that is represented by l2, and in Fig. 8(c), that is represented by l3. There is little difference between l1 and l2 values. But l3 value is significantly higher than l1 or l2 value. The above results show that the draft tube with square corners has adverse effects on water flow. There are some velocity losses after the water flow into the square corners’ position of draft tube. If only setting the round corners of draft tube in Position 1, while Position 2 is set as square corners. These draft tube structures have not evident effect for increasing the flow velocity.
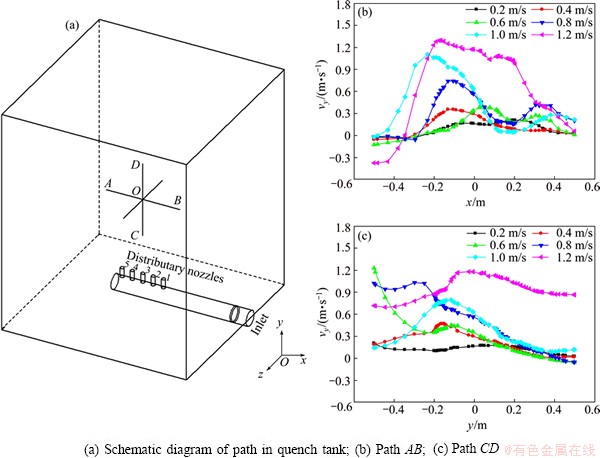
Fig. 7 Influence of path on y-component flow velocity (vy) of water
Based on the analysis of the results about the effect of the shape of the draft tubes and the directional flow baffles on flow field, the authors concluded that the draft tube with round corners and the tank with directional flow baffles will play a beneficial effect on the flow velocity and the flow field uniformity. Therefore, the tank was all modeled by this structure in the following part.
4.2.2 Effect of rotational speed of propeller on flow field
Figure 9 shows influence of rotational speed of the propeller on quenching medium flow field when propeller diameter is 400 mm and flow baffle is 500 mm (x-y plane, z=0). As shown in Fig. 9, the water flow velocity increases with the increase of the rotational speed of the propeller. Figure 10 shows the water flow streamline under different rotational speeds of the propeller when propeller diameter is 400 mm and flow baffle is 500 mm. It can be seen from Fig. 10 that the vortex generates in the tank due to the mechanical stirring. As shown in Fig. 10(a), five characteristic positions are marked as 1, 2, 3, 4 and 5, respectively. Two vortexes emerge under the overflow baffles (Positions 2 and 3, Fig. 10(a)), while the other two vortexes appear on the upper side of the overflow baffles (Positions 1 and 4, Fig. 10(a)). Position 5 is set as the quench zone. It can be seen from Fig.10 that the size of the vortex region increases with the increase of the rotational speed of the propeller. The minimum size of the vortex region appears under the condition of the rotational speed of 300 r/min (Fig. 10(a)), while for the maximum size, the rotational speed is 1200 r/min (Fig. 10(d)). Although the flow velocity increases with increasing the rotational speed, the size of the vortex region also increases. This will inevitably cause a decrease in the water flow uniformity. Above all, increasing rotational speed of the propeller benefits the increase of the flow velocity, but at the same time the disorder degree of flow field also increases.
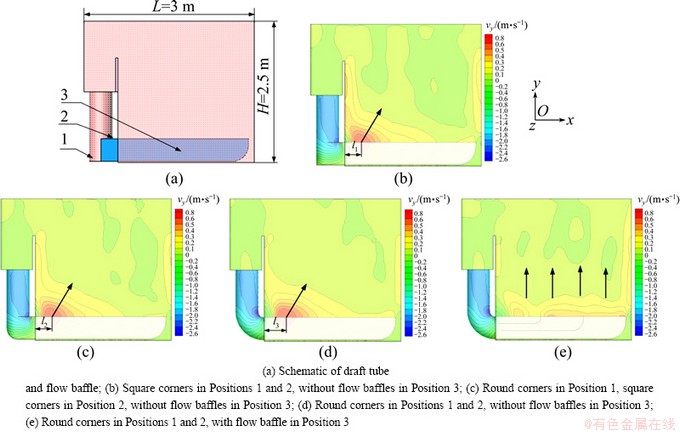
Fig. 8 Influence of draft tube shape and flow baffle on flow field of quenching medium (x-y plane, z=0)
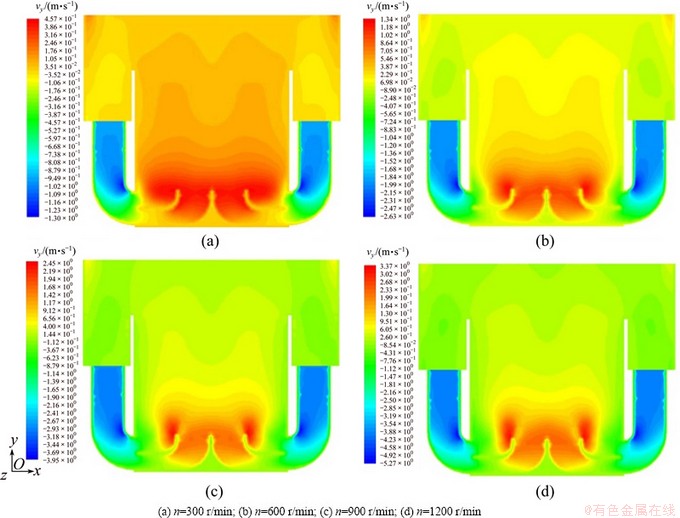
Fig. 9 Influence of rotational speed of propeller on water flow field at propeller diameter of 400 mm and flow baffle of 500 mm (x-y plane, z=0)
4.2.3 Effect of propeller diameter on flow field
Figure 11 shows influence of propeller diameter on water flow field when rotational speed of the propeller is 450 r/min (x-y plane, z=0). It can be seen that there is a large difference in the flow field distribution under different propeller diameters. Figure 12 shows the influence of rotational speed of propeller on y-velocity (z=0, y=0, x=-0.5-0 m). The uniformity of y-velocity at propeller diameter of 400 mm is better than that at propeller diameter of 350 mm. When the propeller diameter is 350 mm, y-velocity increases with increasing the x-coordinate value. But it decreases with the increase of x-coordinate value along positive direction when the propeller diameter is 400 mm.
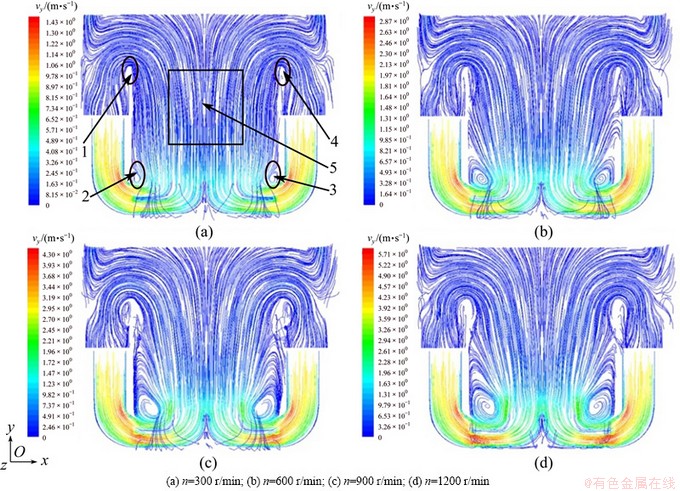
Fig. 10 Water flow streamline under different rotational speeds of propeller at propeller diameter of 400 mm and flow baffle of 500 mm (x-y plane, z=0)
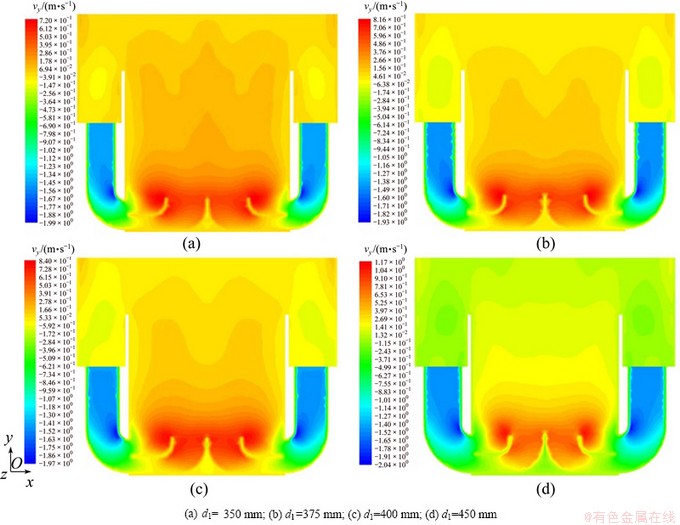
Fig. 11 Influence of propeller diameter (d1) on flow field at rotational speed of propeller of 450 r/min (x-y plane, z=0)
4.2.4 Effect of directional flow baffle position on flow field
Figure 13 shows the influence of flow baffle position on water flow field along y direction when rotational speed is 450 r/min and propeller diameter is 450 mm. Figure 13(a) shows the schematic of directional flow baffle. D is a constant (D=850 mm), d is a variable, which describes the directional flow baffle position. A and B respectively denote two divided inlets. The values of d are set as 300, 400, 425, 450 and 550 mm, respectively. The signs of 1 and 2 in Figs. 13(b) and (f) are the two flow zones caused by the A and B divided inlets. It can be seen from Fig. 13 that the water volume in Zone 1 increases gradually with the movement of the directional flow baffle along x-axis positive, while the water volume in Zone 2 decreases gradually. The value and direction of flow velocity also change with the movement of the baffle, which makes the change of the flow velocity and the flow field uniformity in the central position in tank.
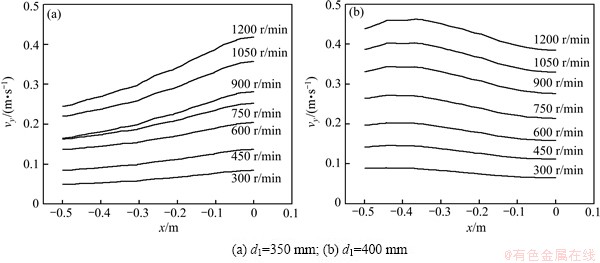
Fig. 12 Influence of rotational speed of propeller on y-velocity (z=0, y=0, x=-0.5-0)
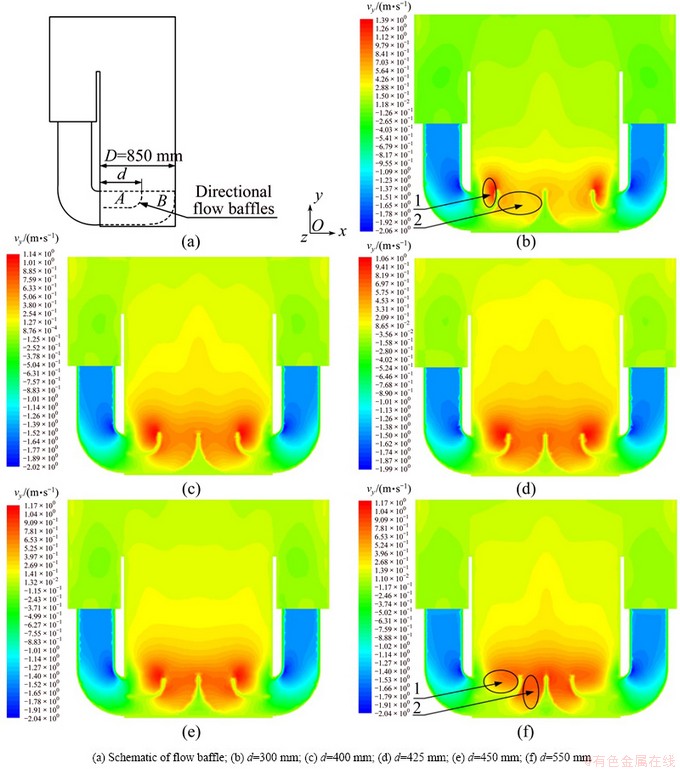
Fig. 13 Influence of directional flow baffle position on flow field along direction y at rotational speed of 450 r/min and propeller diameter of 450 mm
Figure 14 shows the influence of directional flow baffle position on y-velocity when rotational speed is 450 r/min and propeller diameter is 450 mm (z=0, y=0, x=-0.5-0 m). It can be seen from Fig. 14 that, the velocity-position curves show a great fluctuation when d values are 300, 400, 425 and 550 mm, respectively. The y-velocity in central position of the tank is larger than that in other zones. The y-velocity decreases with the increase of the distance from the assumed zone to the central position. At the tank central position, the flow velocity is appropriately 0.21 m/s. The average value of flow velocity is apparently 0.15 m/s at the position of the farthest away from the central position of the quench zone. In addition, the curve in the condition of d=300 mm is steeper than those in other conditions. When d is 450 mm, the curve is relatively flat, and the flow velocity is about 0.15 m/s.
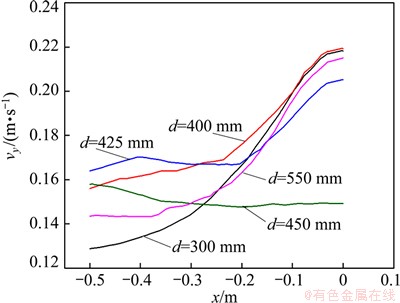
Fig. 14 Influence of directional flow baffle position on y-velocity of medium field at rotational speed of 450 r/min and propeller diameter of 450 mm
4.3 Numerical example of CFD
In this study, a numerical example [16] was used to testify the validity and correctness of the quench tank model. CHEN et al [16] used ANSYS software to simulate the flow distribution in quench tank, while in the present study, FLUENT software was used to conduct the numerical simulation. The present simulation results of a three-dimensional (3D) quench tank model are compared with data in Ref. [16], as shown in Figs. 15 and 16. Compared with the simulation data in Ref. [16], the present simulation results show a higher accuracy in predicting the water flow velocity in the quench zone. In addition, the trends of flow velocity curves from the present simulation also coincide with the experimental curves from Ref. [16]. After qualitative and quantitative analysis of the flow velocity curves, it can be concluded that the performance of the model of water quench tank should be satisfactory and the simulation results are credible.
5 Conclusions
1) Two quench tank models (with or without agitation systems) were established using FLUENT software to research the water flow distribution in the tanks.
2) Although the water flow rate of quench tank without agitation system can be adjusted by changing the inlet velocity, the appearance of the position of the maximum of flow rate has no evident regularity.
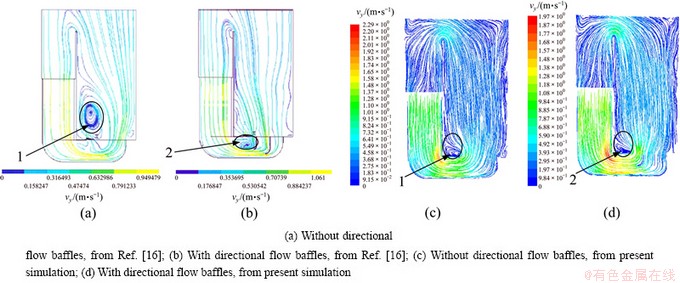
Fig. 15 Pathlines of flow velocity magnitude (x-z plane, y=250 mm) from present simulation and Ref. [16]
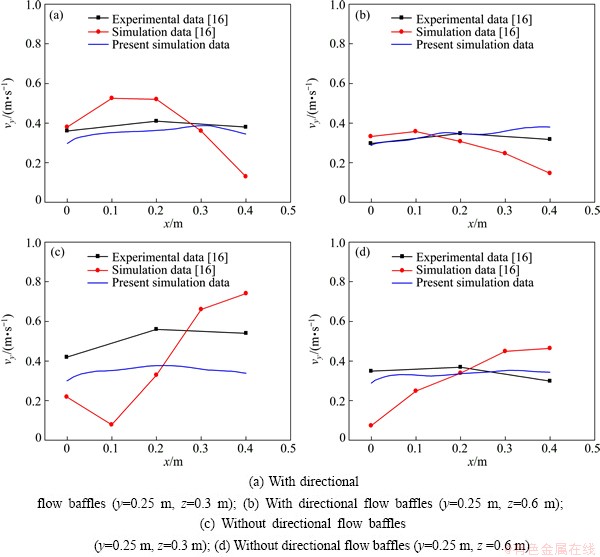
Fig. 16 Comparison of present simulation results and publication data with or without directional flow baffles
3) As for the quench tank with agitation system, the agitation speed and the position of directional flow baffle have important influences on the flow distribution and flow velocity of water. The increase in agitation speed brings a large flow velocity in quench zone, but it also increases the fluctuation of water flow velocity. The position of directional flow baffles plays a crucial role in the control of the trend of velocity-position curves. When the rotational speed is 450 r/min, the propeller diameter is 450 mm and the variable d is 450 mm, the y-velocity in quench zone is relatively stable, which is about 0.15 m/s.
4) The accuracy of the present simulation results is better than that of the simulation results in a numerical example due to the fact that the present simulation results are more close to the experimental data. The high accuracy of CFD model proposed in this paper can provide an optimum design model for quench system and can guide solid solution treatment of A357 alloy large complicated components.
References
[1] YANG Xia-wen, ZHU Jing-chuan, LAI Zhong-hong, LIU Yong, HE Dong, NONG Zhi-sheng. Finite element analysis of quench temperature field, residual stress and distortion in A357 aluminum alloy large complicated thin-wall workpieces [J]. Transactions of Nonferrous Metals Society of China, 2013, 23(6): 1751-1760.
[2] ES-SAID O S, LEE D, PROST W D, GAMBERINI A, MESSIERI S. Alternative heat treatments for A357-T6 aluminum alloy [J]. Engineering Failure Analysis, 2002, 9(1): 99-107.
[3] YANG Xia-wei, ZHU Jing-chuan, NONG Zhi-sheng, HE Dong, LAI Zhong-hong, LIU Yong, LIU Fa-wei. Prediction of mechanical properties of A357 alloy using artificial neural network [J]. Transactions of Nonferrous Metals Society of China, 2013, 23(3): 788-795.
[4] LI Xin-lei, HAO Qi-tang, JIE Wan-qi, ZHOU Yu-chuan. Development of pressure control system in counter gravity casting for large thin-walled A357 aluminum alloy components [J]. Transactions of Nonferrous Metals Society of China, 2008, 18(4): 847-851.
[5] PENG Jin-hua, TANG Xiao-long, HE Jian-tang, XU De-ying. Effect of heat treatment on microstructure and tensile properties of A356 alloys [J]. Transactions of Nonferrous Metals Society of China, 2011, 21(9): 1950-1956.
[6] YANG Xia-wei, ZHU Jing-chuan, HE Dong, LAI Zhong-hong, NONG Zhi-sheng, LIU Yong. Optimum design of flow distribution in quenching tank for heat treatment of A357 aluminum alloy large complicated thin-wall workpieces by CFD simulation and ANN approach [J]. Transactions of Nonferrous Metals Society of China, 2013, 23(5): 1442-1451.
[7] CALLISTER W D. Materials science and engineering: An introduction [M]. New Jersey: John-Wiley, 1994: 783-784.
[8] YANG X W, ZHU J C, NONG Z S, LAI Z H, HE D. FEM simulation of quenching process in aluminum alloy cylindrical bars and reduction of quench residual stress through cold stretching process [J]. Computational Materials Science, 2013, 69: 396-413.
[9] ELKATATNY I, MORSI Y, BLICBLAU A S, DAS S, DOYLE E D. Numerical analysis and experimental validation of high pressure gas quenching [J]. International Journal of Thermal Sciences, 2003, 42(4): 417-423.
[10] BAKER A J. Potential for CFD in heat treating (computational fluid dynamics) [J]. Advanced Materials & Processes, 1997, 10: 44-47.
[11] CANALE L C F, TOTTEN G E. Quenching technology: A selected overview of the current state-of-the-art [J]. Materials Research, 2005, 8(4): 461-467.
[12] TOTTEN G E, BATES C E, CLINTON N A. Handbook of quenchants and quenching technology [M]. Ohio: ASM International, 1993: 339-411.
[13] HALVA J, VOLNY J. Modeling the flow in a quench bath [J]. Hutnicke Listy, 1993, 48(10): 30-34.
[14] GARWOOD D R, LUCAS J D, WALLIS R A, WARD J. Modeling of the flow distribution in an oil quench tank [J]. Journal of Materials Engineering and Performance, 1992, 1(6): 781-787.
[15] BOGH N. Quench tank agitation design using flow modeling [C]//Heat Treating: Equipment and Processes. Ohio: ASM International, 1994: 82-91.
[16] CHEN N L, LIAO B, PAN J S, LI Q, GAO C Y. Improvement of the flow rate distribution in quench tank by measurement and computer simulation [J]. Materials Letters, 2006, 60(13-14): 1659-1664.
基于计算流体力学的A357合金大型复杂构件所用淬火槽内介质流场分布模拟
杨夏炜1,朱景川2,李文亚1
1. 西北工业大学 凝固技术国家重点实验室 陕西省摩擦焊接工程技术重点实验室,西安 710072;
2. 哈尔滨工业大学 材料科学与工程学院,哈尔滨 150001
摘 要:基于FLUENT计算流体力学软件,模拟A357铝合金大型复杂构件所用淬火槽内介质流场分布情况。分别采用两类淬火槽结构(未置搅拌系统和配置搅拌系统),对槽内介质流速及流场均匀性进行模拟计算。结果表明,对于未置搅拌系统的淬火槽,其内部介质流场未发现明显的规律性;而对于配置搅拌系统的淬火槽,在不同工艺参数条件下,其内部介质流场均具有一定的规律性。可知,配置搅拌系统的淬火槽优势明显。另外,获得了流场均匀性较合适工艺参数。最后,通过与文献中的结果进行对比,验证所建计算模型,该模型具有很高的计算精度,能对淬火槽的结构进行优化,从而为大型构件的热处理提供技术支持和理论指导。
关键词:A357合金;流场分布;淬火槽;计算流体动力学模拟
(Edited by Wei-ping CHEN)
Foundation item: Project (51405389) supported by the National Natural Science Foundation of China; Project (2014003) supported by the Shanghai Key Laboratory of Digital Manufacture for Thin-walled Structures, China; Project (3102015ZY024) supported by the Fundamental Research Funds for the Central Universities, China; Project (108-QP-2014) supported by the Research Fund of the State Key Laboratory of Solidification Processing, Northwestern Polytechnical University, China
Corresponding author: Xia-wei YANG; Tel: +86-29-88495226; E-mail: yangxiawei@nwpu.edu.cn
DOI: 10.1016/S1003-6326(15)63975-9