Evolution of dislocation cells during plastic deformation
ZHOU Zhi-min(周志敏)1, SUN Yan-rui(孙艳蕊)1, ZHOU Hai-tao(周海涛)2
(1. College of Science, Northeastern University, Shenyang 110004, China;
2. State Key Laboratory of Metal Matrix Composite, Shanghai Jiaotong University, Shanghai 200030, China)
Abstract: In recent years, materials with ultrafine grain size(UFG) have attracted much attention. By using severe plastic deformation(SPD) techniques, materials with fine grain size as small as 200-250nm have been obtained. However, the nature of the grain boundaries has not been theoretically understood. It is still an unsolved question whether or not finer grain sizes down to 100nm could be reached. A semi-quantitative model for the evolution of dislocation cells in plastic deformation was proposed. The linear stability analysis of this model leads to some interesting results, which facilitate the understanding of the formation of cell structures and of the factors determining the lower limit of the cell size of SPD materials.
Key words: evolution of dislocation cells; ultrafine grain; severe plastic deformation CLC number: TG1
Document code: A
1 INTRODUCTION
It is expected that materials with fine grain size have good performances during service. With the development of new materials processing techniques, such as equal channel angular extrusion(ECAE), bulk materials with ultrafine grain size(UFG) can be produced. However, further clarification on the nature of grain or cell boundaries is needed. Thus, it is still under study whether the severely plastically deformed(SPD) material is truly polycrystalline down to grain sizes of 100nm[1]. The deformation mechanisms and their simulation need further research.
In recent years, the evolution of dislocation patterns has attracted much attention to achieve deep understanding of the deformation mechanisms. It is found that dislocation patterns appearing in deformed metals belong to the combination of the four basic patterns found in copper under cyclic deformation at room temperature[2]. Rolling and torsion lead to a cell structure belonging to the basic patterns[3-6]. Dislocation cell or subgrain is readily formed in the process of creep and drawing[7-9]. With the development of computer technology, many researchers have studied dislocation patterning by computer simulation. For example, Abraham et al[10] simulated the plasticity of FCC crystals by using molecular dynamics in approa-ching micron scale using up to one billion atoms. The evolution of dislocation patterns could be visual on the computer. Their work has important significance in the establishment of continuum theories for plastic deformation in small structures. In theoretical studies of the formation of dislocation patterns, Holt[11] investigated the rearrangement of screw dislocations under the drive of applied stress without considering the generation and annihilation of dislocations. In 1985, Walgraef and Aifantis[12] indicated that plasticity should be viewed as a nonlinear irreversible process driven to a far from dynamic equilibrium point. The evolution of dislocation patterns is a typical nonlinear phenomenon that should be described by the principle of dissipative structure and synergetics. This method has been widely used in plasticity, especially in strain hardening[13, 14].
In the process of severe plastic deformation, dislocations are generated, which move and interact mutually. By the self-organization of dislocations, cell structures are formed and then shrink down to a steady dimension[15-19]. With this consideration, we can assume that the lower limit grain size of SPD materials should be equal to the possibly formed minimum cell size. In this paper, an attempt is made to establish a semi-quantitative model of the evolution of dislocation distributions. By the theoretical analysis of this model, we obtain two generally noteworthy formulae and a criterion for the limiting cell size. These results will promote the understanding of the mechanism of the cell forming process.
2 MODEL
For the sake of simplicity, we consider here only a hierarchy of mobile and immobile dislocations. It is assumed that the dislocations are straight lines, with the Burgers vector parallel to the x direction, unlike dislocations moving with the same rate in opposite directions, and all of the slip systems are active simultaneously where the changes of dislocation density are the same.
In the light of the theory of dissipative structures and the principle of synergetics, any kind of dislocation should obey the following transport-reaction equation[12, 20-22]:

where the subscript k denotes the dislocation type, which is denoted by k=m and k=i for mobile and immobile dislocations, respectively; J denotes the flux of dislocation, and g the generation and reaction term. These terms can be readily expressed in terms of dislocation theory[15, 16]. Hence, we obtain the semi-quantitative dislocation model as follows:
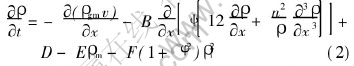
where n indicates the number of dislocation spacing, v the gliding rate of dislocations, ρ the total dislocation density, ρg the difference between the densities of the positive and negative dislocations, which is expressed as[17, 18]:

The factors ψ and φ are as follows:

B, D, E and F are coefficients.
In the process of plastic deformation, the compatibility condition should be satisfied, as follows[16]:

whereτe is the derivative of effective shear stress τe with respect to time, G the shear modulus, v the gliding rate of dislocations. That is to say, at any given local place, the total strain rate is the sum of elastic and plastic strain rate. Based on Eqns.(2-6), a qualitative study of the evolution of cell structure can be made, and some interesting results can be obtained.
3 MECHANISM OF EVOLUTION OF DISLOCATION ARRANGEMENT AND LOWER LIMIT SIZE OF CELL STRUCTURE
The established model is a nonlinear partial differential equation, which contains some unknown coefficients. Therefore, it is difficult to obtain a theoretical solution by using this model. However, we can make a linear stability analysis to achieve some interesting results and to obtain a good understanding of the evolution process. It is known that dislocations tend to form ordered structures of alternative appearing of high and low dislocation density zones. Therefore, we can express the function of spatio-temporal distribution of dislocation by using Fourier expansion. Assuming the investigated system length to be 2l, the solution of the model can be considered as the superposition of an initial dislocation density ρ0 and a number of dislocation density waves[15-17]:
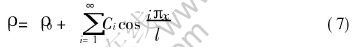
where Ci is the amplitude of the waves. Substituting Eqn.(7) into Eqn.(2), and taking into account the orthogonality relations of the trigonometric function, we can obtain a set of equations as follows:

where ξj is a trivial term that does not affect the evolution of the system.
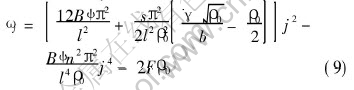
The characters of the system are mainly determined by the value and variation of ωj[17]. When ωj is negative, the system remains stable. Otherwise, the system will lose its stability. Since different j corresponds to different ωj, it is significant to know the change of ωj with j as well as which j is the dominant wave number. Let

Then
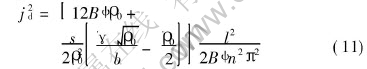
where jd denotes the dominant wave number, s the ratio of the effective and the threshold stresses.
In general ρ0≥107m-2, the magnitude of b is in the order of 10-10m, s in the order of 1, ψ≈1. If the strain rate is in the range of 1-10-4 s-1, the second term in the brackets of Eqn.(11) can be neglected. Thus

The critical dislocation density corresponding to the dominant wave number jd is as follows:

Eqns.(12) and(13) are helpful to understanding the evolution of the cell structure, which will be discussed later. It is reasonable to consider the cell size to be the dominant wavelength. Then the subsequent cells will have the size

Since the elastic interactions between dislocations will be screened beyond a critical distance(assumed here n times the average dislocation distance)[17, 19], n might be as small as one with regard to a high dislocation density. Therefore, the minimum size of cells or subgrains is

where ρcmax denotes the maximum dislocation density in cell interiors. For cold-deformed metals, the dislocation density in cell walls can reach the order of 1016m-2, and in cell interiors the order of 1014m-2. Then the minimum size reached by SPD is 115nm(corresponding to ρcmax=5×1014m-2).
4 DISCUSSION
When we ignore the interaction existing in the system, i.e. the term in the right-hand side of Eqn.(1) or the last three terms of Eqn.(2), we can also obtain the above results, i.e. Eqns.(12-15). This facilitates the whole derivation process. This result is consistent with that of Holt[11].
It is shown from Eqn.(12) that the dominant wave number jd is only dependent on the system length and the dislocation density. The larger the system length is, the larger the dominant wave number will be, i.e. the more the cells will be. For a given deformation, as the dislocation density increases, the smaller dominant wave number jd will appear at first. Hence, the cell number increases with strain up to a steady value. If we consider 2l as the diameter of the initial grain size, the cell forming condition can readily be satisfied in large grains. When the grain sizes are smaller than a critical value, cells will not be formed further. Therefore, we can say that in any plastic deformation there exists a lower limit size for cells. It is obvious that the grain sizes obtained by SPD techniques cannot break through this limit too.
With regard to SPD-copper with the grain size of 200-250nm undergoing fatigue deformation, the stress applied must be smaller than that in the SPD process. Taking into account the features of deformation, the dislocation density during fatigue cannot exceed that in SPD. In terms of Eqn.(14), the cells cannot be formed in the existing grains. This is in consistence with Ref.[1]. Another similar example is superplastic deformation[16]. Because of its high straining temperature and its low strain rate, the produced dislocations disappear readily. Thus, the dislocation density is very low in the interiors of the grains, and cells cannot be formed.
In terms of Eqns.(12-15) as well as the discussion above, it is obvious that the generation of cells(or subgrains) does not depend on the physical parameters of the materials, only on a structural parameter, i.e. the dislocation density. This reflects a general character of materials. Therefore, we can study the microstructural properties based on the combination of dislocation theory with synergetics. This is the fundamental approach to predict the microstructural behavior.
5 CONCLUSIONS
1) The formulae for dominant wave number jd, critical dislocation density ρc and lower limit size dmin are generally applicable to materials.
2) It is hard to achieve a grain size smaller than 100nm for materials deformed by severe plastic deformation.
3) When a SPD material undergoes fatigue deformation, cells are hardly formed in the interior of grains or preexisting cells or subgrains.
ACKNOWLEDGMENT
The authors are grateful to Professor H. Mughrabi for a critical reading of the paper and for a number of helpful comments.
REFERENCES
[1]Mughrabi H. On the grain-size dependence of metal-fatigue [A]. Lowe T C, Valiev R Z. NATO Advanced Research Workshop: Investigations and Applications of Severe Plastic Deformation [C]. Kluwer Academic Publisher, 2000.
[2]Laird C, Charsley P, Mughrabi H. Low energy dislocation structures produced by cyclic deformation [J]. Mater Sci Eng, 1986, 81: 433-450.
[3]Hansen N, Hung X. Microstructure and flow stress of polycrystals and single crystals [J]. Acta Mater, 1998, 46: 1827-1836.
[4]Duly D, Baxter G J, Shercliff H R, et al. Microstructure and local crystallographic evolution in an Al-1wt% Mg alloy deformed at intermediate temperature and high strain rate [J]. Acta Mater, 1996, 44: 2847-2962.
[5]Liu C D, Bassim M N. Dislocation structure evolution in torsion of pure copper [J]. Metall Trans A, 1992, 24A: 361-367.
[6]Bassim M N, Liu C D. Dislocation cell structures in copper in torsion and tension [J]. Mater Sci Eng, 1993, A164: 170-174.
[7]Straub S, Blum W, Maier H J, et al. Long-range internal stresses in cell and subgrain structures of copper during deformation at constant stress [J]. Acta Mater, 1996, 44: 4337-4350.
[8]Kubin L P, Estrin Y. Evolution of dislocation densities and the critical conditions for the Portevin-Le Chatelier effect [J]. Acta Metall Mater, 1990, 38: 697-708.
[9]Cizek P, Wynne B P, Parker B A. The behavior of incidental dislocation boundaries and geometrically necessary boundaries during warm tensile deformation of aluminium [J]. Scripta Mater, 1996, 35: 1129-1134.
[10]Abraham F F, Walkup R, Gao H, et al. Simulating materials failure by using up to one billion atoms and the worlds fastest computer: work-hardening [J]. Proc National Academy of Sciences of USA, 2002, 99: 5783-5787.
[11]Holt D. Dislocation cell formation in metals [J]. J Appl Phys, 1970, 41(8): 3197-3201.
[12]Walgraef D, Aifantis E C. Dislocation patterning in fatigued metals as a result of dynamical instabilities [J]. J Appl Phys, 1985, 58: 688-691.
[13]Barlat F, Glazov M V, Brem J C, et al. A simple model for dislocation behavior, strain and strain rate hardening evolution in deforming aluminum alloys [J]. International Journal of Plasticity, 2002, 18: 919-939.
[14]LUO Y S, GUO X G, MEI Y F, et al. Dislocation evolution with rheological forming of metal [J]. Trans Nonferrous Met Soc China, 1999, 9(3): 623-628.
[15]ZHOU Z M. Evolution of Dislocation Patterns [M]. Shenyang: Northeastern University Press, 2003.(in Chinese)
[16]ZHOU Z M. Dynamic Model for the Evolution of Dislocation Patterns and Its Computer Simulation [D]. Shenyang: Northeastern University, 1999.(in Chinese)
[17]GAO W L. Dissipative Structure and Synergetics Model for Plastic Deformation and the Hot Deformation Behavior of the Nb-bearing Low Carbon Steel [D]. Shenyang: Northeastern University, 1993.(in Chinese)
[18]GAO W L, BAI G R, ZHOU Z M, et al. An evolution model of dislocation patterns in plastic deformation and its application [J]. Science in China(Series A), 1995, 38(7): 875-883.
[19]Kubin L P. Dislocation patterning, in Materials Science and Technology [M]. Mughrabi H. Weinheim: VCH Verlagsgesellschaft, 1993. 137-190.
[20]Nicolis G, Prigogine I. Self-organization in Nonequilibrium Systems [M]. Beijing: Science Press, 1986.(in Chinese)
[21]Glansdoff P, Prigogine I. Thermodynamic Theory of Structure, Stability and Fluctuations [M]. New York: Wiley, 1971.
[22]Haken H. Advanced Synergetics [M]. 2nd ed. Berlin: Springer Verlag, 1987.
(Edited by YUAN Sai-qian)
Foundation item: Project supported by the Foundation of Education Ministry for Returned Scholars; Project supported by the Foundation of Northeastern University for Returned Doctors; Project(2003033372) supported by the National Postdoctoral Foundation of China
Received date: 2003-12-01; Accepted date: 2004-06-28
Correspondence: ZHOU Zhi-min, PhD; Tel: +86-24-83678479; E-mail: zhou92@163.com