双模量悬臂梁在线性分布荷载作用下的Kantorovich解
吴晓,杨立军,黄翀,孙晋
(湖南文理学院 土木建筑工程学院,湖南 常德,415000)
摘要:基于双模量悬臂梁在分布载荷作用下发生弯曲变形时,会形成各向同性的拉伸区和压缩区,为此,将双模量悬臂梁看成2种各向同性材料组成的层合梁,采用弹性理论建立双模量悬臂梁在均布载荷作用下的静力平衡方程,利用静力平衡方程确定双模量悬臂梁的中性面位置。在此基础上,利用Kantorovich法研究分布载荷作用下双模量悬臂梁的平面应力问题,推导出悬臂梁的应力公式,并将该应力公式计算结果与有限元法计算结果进行比较,以验证双模量悬臂梁的应力公式的可靠性。研究结果表明:在分布载荷作用下,双模量悬臂梁的平面应力问题不宜采用相同弹性模量弹性理论计算,而应该采用双模量弹性理论计算。
关键词:双模量;悬臂梁;分布载荷;Kantorovich法;弯曲
中图分类号:O343.5 文献标志码:A 文章编号:1672-7207(2014)01-0306-06
Kantorovich solution for bimodulous cantilever under linear distributed loads
WU Xiao, YANG Lijun, HUANG Chong, SUN Jin
(College of Architecture & Civil Engineering, Hunan University of Arts and Science, Changde 415000, China)
Abstract: Considering that the bimodulous cantilever can form isotropic compression and tensile area under distributed load, bimodulous cantilever was regarded as laminated beam composed of two kinds of otropic material. Static equilibrium equation of bimodulous cantilever under uniform load was established by using elastic mechanics theory. The location of neutral plane in bimodulous cantilever was determined by using static equilibrium equation. Plane stress problem of bimodulous cantilever under distributed loads was studied by Kantorovich method, and the stress formula was derived. The calculation results obtained by finite element were compared to verify the reliability of this method. The results show that the plane stress problem of bimodulous cantilever under distributed loads can not be computed using the same elastic modulus theory, and the bimodulous elastic theory should be used.
Key words: bimodulous; cantilever beam; distributed loads; Kantorovich method; bending
在工程实际中,石墨、增强复合材料、金属合金、陶瓷、玻璃、铸铁等许多材料都具有拉压弹性模量不同的双模量特性,所以,用双模量本构关系对这些材料制成的结构进行计算分析备受关注[1-5]。对于拉压弹性模量不同的双模量材料,弹性系数不仅依赖于结构材料,而且随各点位移或应力状态的不同而不同,即与结构材料、形状、边界条件及外载荷有关[6-8]。在梁、弹性平面等问题的结构中,已开始考虑材料的双模量特性[9-12]。一些研究者采用Kantorovich法研究了柱形杆的扭转问题,但未见到采用Kantorovich法研究双模量悬臂梁的平面应力问题的报道。经典弹性理论研究分布载荷作用下悬臂梁的平面应力问题多采用半逆法或三角级数法。半逆法要靠经验假设应力函数多项式需要确定的待定常数较多,三角级数法存在收敛慢且计算过程复杂、繁琐的缺陷,为此,本文作者采用Kantorovich法研究在分布载荷作用下双模量悬臂梁的平面应力问题。
1 悬臂梁的Kantorovich解
对于图1所示分布载荷作用下的悬臂梁,可知其分布载荷的集度表达式为
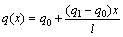 (1)
(1)
式中:q0为梁上线性分布载荷最小值;q1为梁上线性分布载荷最大值;x为梁横截面的位置;l为梁的跨长。
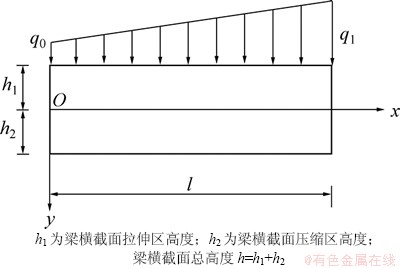
图1 双模量悬臂梁
Fig. 1 Bimodulous cantilever
悬臂梁上下面的边界条件及中性轴的应力条件分别为
 (2)
(2)
式中:上标“+”表示拉伸区,“-”表示压缩区。
假设分布载荷作用下悬臂梁拉伸区和压缩区的应力函数分别为
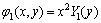 ,
, (3)
(3)
所以,分布载荷作用下双模量简支梁的应力表达式为
 (4)
(4)
对于平面应力问题,分布载荷作用下双模量悬臂梁的余能为

 (5)
(5)
将式(4)代入式(5)可得:
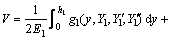
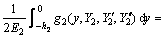

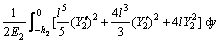 (6)
(6)
式中:E1为拉伸区弹性模量;E2为压缩区弹性模量。对式(6)进行一阶变分可得:

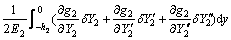 (7)
(7)
利用微分符号与变分符号可交换性及边界条件式(2)可得:
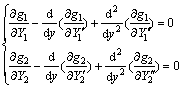 (8)
(8)
把有关函数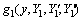 和
和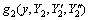 表达式代入式(8)得:
表达式代入式(8)得:
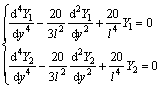 (9)
(9)
由式(9)可以求得 和
和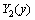 的表达式为
的表达式为
 (10)
(10)
式中: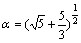 ;
;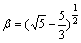 。
。
将式(10)代入式(4)可求得拉伸区及压缩区应力表达式为:
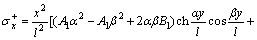

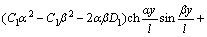
 ; (11)
; (11)
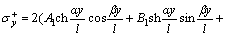
 ; (12)
; (12)
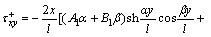
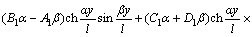
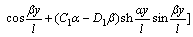 ; (13)
; (13)
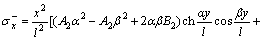

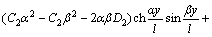
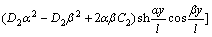 ; (14)
; (14)

 (15)
(15)
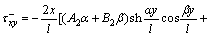
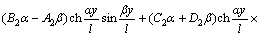
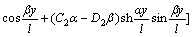 。 (16)
。 (16)
利用边界条件式(2)及式(11)~(16)可得:
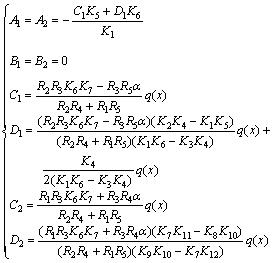 (17)
(17)
式中:
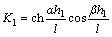 ;
;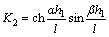 ;
;
 ;
;
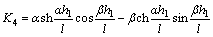 ;
;
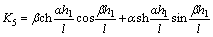 ;
;
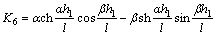 ;
;
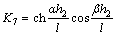 ;
;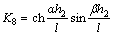 ;
;
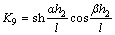 ;
;
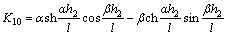 ;
;
 ;
;
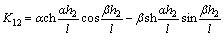 ;
;
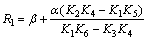 ;
;
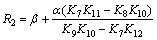 ;
;
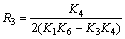 ;
;
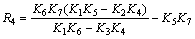 ;
;
 。
。
2 材料力学应力表达式
由于双模量梁在外荷载作用下弯曲时会形成弹性模量不同的拉伸区和压缩区,由弹性理论可知双弹性模量梁弯曲时的应力和应变关系为
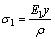 ,
,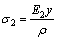 (18)
(18)
由弹性理论可知双模量梁弯曲时横截面内力应满足以下关系:
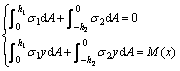 (19)
(19)
把式(18)代入(19)可求得:
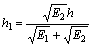 ,
,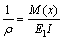 (20)
(20)
式中: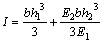 。所以,材料力学推导出的双模量简支梁应力表达式为
。所以,材料力学推导出的双模量简支梁应力表达式为
 ,
,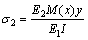 (21)
(21)
图1所示的双模量悬臂梁固定端的弯矩为
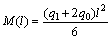 (22)
(22)
图1 所示的双模量悬臂梁固定端得最大拉应力及最大压应力分别为
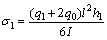 ,
,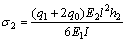 (23)
(23)
3 算例分析与讨论
首先采用有限元法检验本文Kantorovich解的计算精度。假设某宽度b为1 m的双模量悬臂梁弹性模量E1=93.2 GPa,E2=124.36 GPa(设为材料1)。下面采用式(11)~(16)和(23)及有限元法计算双模量悬臂梁固定端处的最大拉应力及最大压应力。其中,采用有限元建立双模量悬臂梁实体模型,模型由2层梁组成,梁高为100 mm,下层梁高为46.4 mm,材料模型为mat1,E2=124.36 GPa;上层梁高为53.6 mm,材料模型为mat 2,E1=93.2 GPa,单元为8节点SOLID185单元。另外,为了比较双模量材料的影响,假设了2种相同弹性模量材料:对于材料2,E1=E2=93.2 GPa;对于材料3,E1=E2=124.36 GPa。采用式(11)~(16)和(23)计算悬臂梁固定端处的最大拉应力及最大压应力,计算结果见表1~4(其中,括号内数字为有限元法计算结果)。本文方法计算结果与材料力学方法计算结果可见表3和表4。分析表1~4可知:
(1) 采用Kantorovich法研究双模量悬臂梁固定端弯曲应力的计算结果与有限元法的计算结果很接近。这说明采用Kantorovich法研究双模量悬臂梁弯曲应力的计算精度很高,所得双模量悬臂梁的应力公式是可靠的。
表1 q1=q0时双模量悬臂梁固定端处弯曲应力
Table 1 Bending stress of bimodulous cantilever at fixed end when q1=q0 ×q0

表2 q1=2q0时双模量悬臂梁固定端处弯曲应力
Table 2 Bending stress of bimodulous cantilever at fixed end when q1=2q0 ×q0

表3 q1=q0时本文方法与材料力学方法所得双模量悬臂梁固定端处弯曲应力的比较
Table 3 Comparison of bending stress of bimodulous cantilever at fixed end using the method in the paper and materials method when q1=q0 ×q0

表4 q1=2q0时本文方法与材料力学方法所得双模量悬臂梁固定端处弯曲应力的比较
Table 4 Comparison of bending stress of bimodulous cantilever at fixed end using the method in the paper and materials method when q1=2q0 ×q0

(2) 采用相同弹性模量弹性理论得到双模量悬臂梁固定端处弯曲应力,与采用双模量弹性理论得到双模量悬臂梁固定端处弯曲应力的相对误差均在5%以上。理论上,采用相同弹性模量弹性理论研究双模量悬臂梁的弯曲应力,拉压区的弯曲应力绝对值是相等的。而事实上,双模量悬臂梁的拉压区的弯曲应力绝对值不相等,会随着双模量悬臂梁拉压区弹性模量的变化而变化。在本文中,双模量悬臂梁的拉压区弯曲应力相对误差均达10%以上,所以,双模量悬臂梁的平面应力问题不宜采用相同弹性模量弹性理论求解,而应采用双模量弹性理论求解。
(3) 在外载荷作用下,随着双模量悬臂梁长高比的增大,双模量悬臂梁的拉压区的弯曲应力也随着增大。因为随着双模量悬臂梁长高比的增大,将导致分布载荷对双模量悬臂梁产生的弯矩也相应增大。
(4) 在分布载荷作用下,双模量悬臂梁固定端的弯曲应力大于均布载荷作用下双模量悬臂梁固定端的弯曲应力。这是因为分布载荷对双模量悬臂梁产生的弯矩大于均布载荷对双模量悬臂梁产生的弯矩。
从表3和表4可以看出:采用材料力学方法研究双模量悬臂梁的弯曲应力,得到的双模量悬臂梁拉伸区的弯曲应力与本文Kantorovich法得到的双模量悬臂梁拉伸区的弯曲应力很接近;但是,采用材料力学方法研究双模量悬臂梁的弯曲应力,得到的双模量悬臂梁压缩区的弯曲应力与本文Kantorovich法得到的双模量悬臂梁压缩区的弯曲应力相差较大,误对误差均在50%以上,并且有限元法所得计算结果也证明了这一点。这说明采用材料力学方法研究双模量悬臂梁的弯曲应力存在局限性。
4 结论
(1) 采用Kantorovich法研究双模量悬臂梁弯曲应力的计算精度较高,表明所得双模量悬臂梁的应力公式是可靠的。
(2) 采用材料力学方法研究双模量悬臂梁的弯曲应力存在局限性。双模量悬臂梁的平面应力问题不宜采用相同弹性模量弹性理论计算,而应该采用双模量弹性理论计算。
(3) 在外载荷作用下,随着双模量悬臂梁长高比的增大,双模量悬臂梁的拉压区的弯曲应力也随着增大。在分布载荷作用下,双模量悬臂梁的弯曲应力大于均布载荷作用下双模量悬臂梁的弯曲应力。
参考文献:
[1] 李战莉, 黄再兴. 双模量泡沫材料等效弹性模量的细观力学估算方法[J]. 南京航空航天大学学报, 2006, 38(4): 464-468.
LI Zhanli, HUANG Zaixing. Meso-mechanical method for estimating equivalent elastic modulus of foam-solid with double-modulus[J]. Journal of Nanjing University of Aeronautics & Astronautics, 2006, 38(4): 464-468.
[2] 曾纪杰. 对中柔度压杆的双模量理论的修正[J]. 机械强度, 2006, 28(3): 462-464.
ZENG Jijie. Revision of the formula with bimodulusim intermediate column[J]. Journal of Mechanical Strength, 2006, 28(3): 462-464.
[3] 蔡来生, 俞焕然. 拉压模量不同弹性物质的本构[J]. 西安科技大学学报, 2009, 29(1): 17-21.
CAI Laisheng, YU Huanran. Constitutive relation of elastic materials with different elastic moduli in tension and compression[J]. Journal of Xi’an University of Science and Technology, 2009, 29(1): 17-21.
[4] 吴晓, 杨立军, 孙晋. 双模量圆板弯曲变形的计算分析[J]. 西安建筑科技大学学报(自然科学版), 2009, 41(1): 88-92.
WU Xiao, YANG Lijun, SUN Jin. Bending deformation calculation of bimodulous circular plate[J]. Journal of Xi’an University of Architecture. & Technology (Natural Science Edition), 2009, 41(1): 88-92.
[5] 罗战友, 夏建中, 龚晓南. 不同拉压模量及软化特性材料的柱形孔扩张问题的统一解[J]. 工程力学, 2008, 25(9): 79-84.
LUO Zhanyou, XIA Jianzhong, GONG Xiaonan. Unified solution for expansion of cylindrical cavity in strain-softening materials with different elastic moduli in tension and compression[J]. Engineering Mechanics, 2008, 25(9): 79-84.
[6] 阿巴尔楚米扬. 不同模量弹性理论[M]. 邬瑞锋, 张允真, 译. 北京: 中国铁道出版社, 1986: 11-22.
Ambartsumyan S A. Elasticity theory of different modulus[M]. WU Ruifeng, ZHANG Yunzhen, trans. Beijing: China Railway Press, 1986: 11-22.
[7] 姚文娟, 叶志明. 不同模量横力弯曲梁的解析解[J]. 应用数学和力学, 2004, 25(10): 1014-1022.
YAO Wenjuan, YE Zhiming. Analytical solution for bending beam subject to later force with different modulus[J]. Applied Mathematics and Mechanics, 2004, 25(10): 1014-1022.
[8] 周怡之. 双模量Winkler地基简支长圆板剪切屈曲[J]. 上海工业大学学报, 1993, 14(6): 478-484.
ZHOU Yizhi. Shear stability of long circular plates on bi-moduli elastic foundations[J]. Journal of Shanghai University of Technology, 1993, 14(6): 478-484.
[9] 王子昆. 拉压不同模量圆柱薄壳在均匀轴压下的对称失稳[J]. 西安交通大学学报, 1989, 23(6): 94-100.
WANG Zikun. Symmetrical buckling of circular cylindrical thin shell with different elastic moduli intension and compression under a well-distributed axial load[J]. Journal of Xi’an Jiaotong University, 1989, 23(6): 94-100.
[10] 高潮, 刘相斌, 吕显强. 用拉压不同模量理论分析弯曲板[J]. 计算力学学报, 1998, 15(4): 448-455.
GAO Chao, LIU Xiangbin, L Xianqiang. Analysis for the plate with the theory of different extension compression elastic modulis[J]. Chinese Journal of Computational Mechanics, 1998, 15(4): 448-455.
Xianqiang. Analysis for the plate with the theory of different extension compression elastic modulis[J]. Chinese Journal of Computational Mechanics, 1998, 15(4): 448-455.
[11] Zheng X J, Zhou Y H. On convergence of interpolated-iterative method of geometrically nonlinear equations of plates[J]. Science in China (Series A), 1989, 32(3): 316-327.
[12] Zheng X J, Zhou Y H. Exact solution to large deflection of circular plate under compound loads[J]. Science in China (Series A), 1987, 30(4): 391-404.
(编辑 陈灿华)
收稿日期:2013-03-10;修回日期:2013-05-12
基金项目:湖南省科技计划项目(2008FJ3067);湖南省“十二五”重点建设学科(机械设计及理论)资助项目(湘教发2011[76])
通信作者:杨立军(1976-),男,湖南邵阳人,副教授,从事结构振动理论研究;电话:15873665127;E-mail: yanglj9601@163.com