Forming limits of nickel coating on right region

ZHOU Li-qun(周里群)^{1, 2}, LI Yu-ping(李玉平)¹, ZHOU Yi-chun(周益春)²

1. School of Mechanical Engineering, Xiangtan University, Xiangtan 411105, China;

2. Key Laboratory of Low Dimensional Materials and Application Technology of Ministry of Education,Xiangtan University, Xiangtan 411105, China

Received 14 December 2006; accepted 24 May 2007

Abstract: The forming limits of nickel coating on the right region were studied, so as to direct the preparation of the material and help the production of workpieces. The electrodeposited nickel coating was prepared on steel substrate to form advanced structures, and its plastic instability was investigated by the Swift Plastic Instability Theory. By using the compound law for laminated sheet metals, explicit equations for the calculation of the instable eigen values were deduced. The forming limit diagrams of the nickel coating on the right region were plotted. It is exhibited that the forming limit of the coating sheet is between the forming limits of the individual nickel coating and steel substrate. The forming limit of the nickel coating is not so good as that of the steel substrate, and the forming limit strain of the coating sheet tends to diminish with the increase of thickness of the coating. The greater the normal anisotropic coefficient of the materials is, the better the forming limit is.

Key words: nickel coating; instability criterion; forming limit diagram; sheet metal forming

1 Introduction

Thin coatings have applied in industries for a wide range of engineering purposes. The evaluation and enhancement of the mechanical reliability of these inhomogeneous structures have emerged as high priority that subjects to both the materials science and solid mechanics communities[1-3]. Usage of some coatings to form workpiece is a new technology. The coating plastic forming performance is worth studying[4-6].

The forming performance of coatings are vital since a coating should not crack or flake as the metal sheet deforms. In most cases, it is the coating that contacts with dies, so the forming characteristics and friction properties of the coating are important. HAUW et al[7] ever studied the stamping process of galvanized sheets, but they used the average mechanical data of the coating and substrate to calculate, and their results are coarse. PARISOT et al[8] studied the mechanical behavior of a multicrystalline zinc coating on a hot-dip galvanized steel sheet, but the coating thickness (10 μm) obtained seemed to be too small for significant strain and stress gradients to develop from the interface to the free surface. KIM et al[9] studied the forming characteristics of coated sheet metals by experiment, and found that the coating material’s forming limit is restricted by the coating’s defects (cracking, flaking, scratching, etc).

An electrodeposited nickel coating was prepared on a steel sheet substrate and the materials were used in advanced structure applications[10]. The materials were dynamically impacted by projectiles in a 57 mm light air cannon, and it was found that the nickel electrodeposited steel sheet had good interface quality[11]. So as to study the formability of these materials, their forming limit diagrams in left region were studied[4]. In this study, the forming limit diagrams of the right region for the materials are investigated by the concept of necking. It means that the plastic instability of the coating sheet metals under 3-dimensional strains and tensile stresses is investigated by adopting the Swift Plastic Instability Theory. This study is helpful for the preparation of the materials and the manufacture of special workpieces.

2 Experimental determination of stress— strain relations

The stress—strain relation of the electrodeposited nickel coating was determined with a (JEOL)JSM- 5600LV SEM(scanning electron microscopy), in which the applied tensile force could be produced by a tensile equipment. In this method, the stress—strain properties of the steel substrate without coating were first determined for the specimen loaded by a tensile load. Then the stress—strain properties of coating/substrate composite were determined for the specimen loaded by an applied tensile force. The surface of the specimen could be observed by scanning electron microscope (SEM) during the loading process. In this case, the initiation of micro-cracks and the propagation of macro-crack could be observed. Fig.1 shows a schematic diagram of the specimen configuration and dimensions. The strain gauge was on the surface of the substrate. The stress—strain data for substrate and nickel coating can be tested and calculated as Figs.2 and 3[12]. So as to conveniently analyze the materials’ forming limit, the stress—strain data are fitted as curves of power law by least squares method, which are also shown in Figs.2 and 3, that is σ=946.3ε^{0.369 6} for low carbon steel substrate and σ=1 805.6ε^{0.357 0} for the nickel coating.

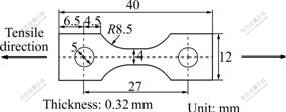
Fig.1 Schematic diagram of specimen configuration and dimensions

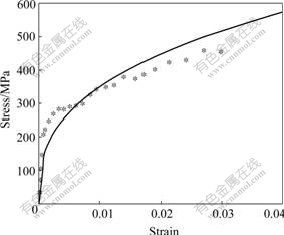
Fig.2 Stress—strain fitted curve and tested points for nickel coating

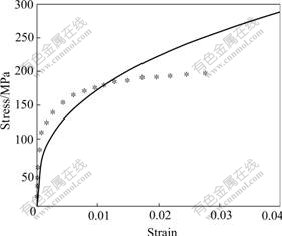
Fig.3 Stress—strain fitted curve and examined points for substrate sheet

3 Plastically instable limits by uniaxial tensile loading

Based on the dynamic impact test, the nickel electrodeposited steel sheet is postulated to have enough interface strength, and not to delaminate in deformation, and furthermore the influence of the structure and property’s variations in the combined interface on the flow stresses is neglected. Thus the flow stresses on the cross section under uniaxial tensile force are shown in Fig.4.

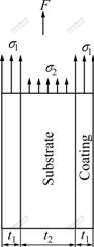
Fig.4 Nickel coating sheet under uniaxial tensile loading

3.1 Plastically instable point property for coating sheet

Assume the thickness of the unilateral nickel coating is t₁, and the thickness of the steel sheet is t₂, as shown in Fig.4. The stress—strain relations of the coating and the substrate are σ₁=σ₁(ε) and σ₂=σ₂(ε), respectively.

For the compound sheet of coating and substrate, it is also a kind of laminated sheet metals. The stresses in component section comply with the compound law of laminated sheet metals[13], that is

σ=2λσ₁+(1-2λ)σ₂                              (1)

where  σ is the equilibratory stress in the total section at any instance, and λ is a constructional parameter.

Because the volume of the materials cannot be compressed during the deformation, the parameter λ becomes

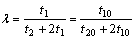                       (2)

where  t₁₀ and t₂₀ are the initial thicknesses of the nickel coating and the steel substrate before deformation, respectively. Thus the parameter λ represents the amount of the nickel coating thickness in the total section thickness, and it has the scope of 0＜λ＜0.5.

According to the Swift Instability Theory[14], the instability criterion for the coating sheet in uniaxial tensile loading is dF=0, where F is the total tensile axial force. Thus based on Eqn.(1), for the specimen under uniform deformation condition, by use of the Swift Instability Criterion, we can obtain

      (3)

where  ε is the strain at the instable point in the coating and substrate. Because the coating and the substrate are combined together firmly and the interface does not delaminate, so the strains in the coating and in the substrate are equal to each other.

Substituting Eqn.(1) into Eqn.(3), and the stress and strain of the coating sheet in plastically instable point is assumed to be σ_pi and ε_pi, then we can get the instability criterion of the coating sheet:

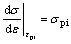                               (4)

From above equation, we can find that the plastic instability criterion for the coating sheet is just like that of uniform materials[15]. If the constitute equations for the coating and the substrate are given, then by Eqn.(4), we can determine the two eigen values σ_pi and ε_pi for the coating sheet under instable condition.

At the plastically instable point, utilizing Eqn.(1), the instable stress of the coating sheet can be obtained:

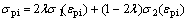              (5)

3.2 Calculation of plastically instable parameters

For metal with a power law of strain hardening, the constitute relations for the nickel coating is

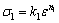                                  (6)

For the steel substrate, it is

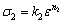                                  (7)

Substituting Eqns.(6) and (7) into Eqn.(1), then into Eqn.(4), the following equation can be obtained:

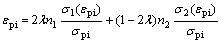         (8)

In Eqn.(8), it contains two unknown eigenvalue parameters σ_pi and ε_pi. If it is combined with Eqn.(5), the exact values of the two unknown parameters can be obtained. But it needs to solve nonlinear equations. The numerical calculation is not convenient.

A lot of tests exhibit that, near the plastically instable point, the slope of stress—strain curve of individual metal material varies very little. For clad sheet, the characteristics at the plastically instable point mainly depend on the stress—strain properties of their individual components in the scope of (ε_pi1, ε_pi2). Here ε_pi1 and ε_pi2 are strain eigen values of the two components. In this study, they are just the strain parameters of the nickel coating and steel substrate at the plastically instable point. So, by use of the tangent equations of the stress—strain curves near the instable point instead of the stress— strain curves for calculation of the eigen values of instability, it is acceptable compared with experiment and will not make apparent errors[16].

The tangent equations of the stress—strain curves at the instable points under uniaxial tension are

σ₁=σ_pi1(1-ε_pi1+ε)                              (9)

σ₂=σ_pi2(1-ε_pi2+ε)                             (10)

where  σ_pi1 and σ_pi2 are stress eigen values in the strains of eigen values ε_pi1 and ε_pi2 for the nickel coating and the steel substrate at the plastically instable point.

By substituting Eqns.(9) and (10) into Eqn.(8), omitting high order small quantity, we can obtain

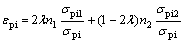               (11)

By substituting Eqns.(9) and (10) into Eqn.(5), omitting high order small quantity, we can also obtain

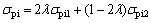                   (12)

Eqns.(11) and (12) explicitly express the eigen values of strain and stress for the coating sheet at plastically instable point. By utilizing the two equations we can easily obtain the eigen values σ_pi and ε_pi.

4 Plastic instability of coating sheet under tensile stress

The equivalent strain of a strain state is

  (13)

It is the comprehensive effects of all strain components and a governing measure of the degree of plastic deformation. Plastic instability will occur when the largest principal strain is equal to the equivalent strain at a critical value.

For the coating sheet in uniaxial tension, when the largest principal strain ε₁ equals the equivalent strain at a critical value, plastic instability of diffused necking will occur[17], namely,

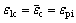                               (14)

For a three-dimensional strain state, with constant volume in plastic deformation, we have

ε₁+ε₂+ε₃=0                                  (15)

where  ε₁, ε₂ and ε₃ are three-dimensional true strains respectively.

Let

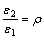                                    (16)

Substituting ε₃ and ε₂ in Eqns.(15) and (16) into Eqn.(13), then

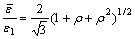                      (17)

In Eqn.(17), when ε₁=ε_pi, the equivalent strain will be at a critical value, thus

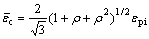                    (18)

For coating sheet metals under tensile stresses, plastic instability occurs when the largest principal strain ε_major is equal to 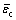[17] or

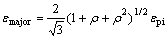                 (19)

Let R be the ratio of anisotropic strain to thickness. The equivalent strain for coating sheet metals forming process will be[18]

(20)

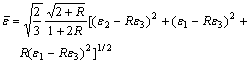
and similarly,

(21)

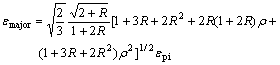
When R=1, Eqn.(21) is just Eqn.(19), that is the isotropic strain state. On the other hand, according to Eqn.(16), the minor strain ε_minor is

ε_minor=ρε_major                                (22)

For the steel sheet with nickel electrodeposited coating, if neglecting the anisotropy of the thickness, or letting R=1, from Eqn.(19) we know that ε_major will be the greatest when ρ=1 and the least when ρ=0.

5 Results and discussion

We prepare the nickel electrodeposited coating on low carbon steel sheet. The steel substrate thickness is 0.3 mm, and the nickel coating unilaterial thickness is 6.2 μm. The stress—strain relations of the coating and substrate are tested as mentioned above. When their constitutive relations are known, the stress—strain relation of the coating sheet may be calculated as Fig.5 in terms of the compound law. From Fig.5, it is shown that the stress—strain curve of the coating sheet is between the nickel coating and the steel substrate, very close to the substrate.

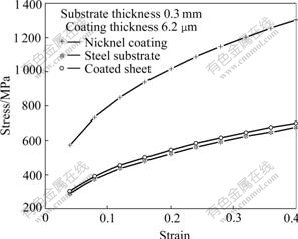
Fig.5 Strain—stress relations of single coating, substrate and coating sheet

According to the power laws of the components of nickel coating and steel substrate, the eigen value of strain of the nickel coating at instable point is ε_pi1=n₁=0.357 0, and the eigen value of strain of the steel substrate is ε_pi2=n₂=0.369 6. For the coating sheet, thus we may calculate the instability stress is σ_pi=704.2 MPa, and the instability strain ε_pi=0.367 7. Therefore we can calculate the forming limit curves for the individual nickel coating, individual steel substrate and the coating sheet under tensile stresses, as shown in Fig.6.

From Fig.6, we can find that the forming limit curve of the nickel coating is lower than that of the steel substrate, and the forming limit curve of the coating sheet is between them and very close to that of the substrate. This is because the substrate is much thicker than nickel coating. Because the forming limit of the nickel coating is not so good as the substrate, the forming of the nickel coating is very challenged. If the coating is deteriorated in the forming process, the workpiece will be discarded. So we cannot disregard the nickel coating’s forming performance in cold forming process.

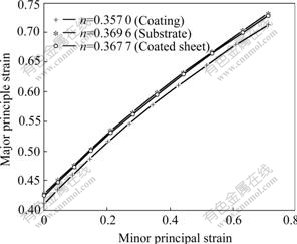
Fig.6 Forming limit curves of single coating/substrate and coating sheet under tensile stresses

We can further determine the limited strain of the coating sheet with the variation of the nickel coating thickness. By utilizing Eqns.(2), (11) and (12), we can calculate the limited strain ε_pi for the coating sheet under different coating thicknesses as shown in Fig.7. From this figure, we can find that the thicker the nickel coating is, the lower the forming limited strain of the coating sheet is. The forming limit of the substrate is greater than that of the coating, which can be seen from Fig.6, so the thicker the nickel coating is, the nearer the forming limited curve of the coating sheet is to that of the nickel coating. From Fig.7 we can also discover that the limited strain ε_pi for the coating sheet is in inverse proportion to the thickness ratio of the coating/substrate in the strain scope of eigen values [ε_pi1, ε_pi2]. At the same time, the instability stress of the coating sheet σ_pi is also inversely proportional to the thickness ratio of the coating/ substrate in the stress scope of eigen values [σ_pi1, σ_pi2], as found in Eqn.(12). So the instable point of the coating sheet in uniaxial tension is between the instable points of the individual coating and substrate, and their eigen values vary inversely linearly with the ratio of the components thickness.

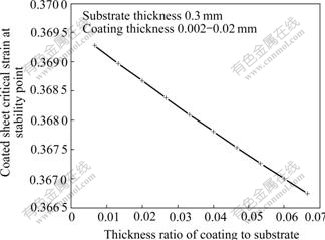
Fig.7 Coating sheet stability strain with variation of coating thickness

In terms of Eqns.(21) and (22), we may discover the effect of the normal anisotropic coefficient on the forming limit of the coating sheet, as shown in Fig.8. Fig.8 illustrates that if the anisotropic coefficient of the materials is greater than 1, the forming will be favorable; if it is smaller than 1, the forming will be unfavorable. This is the reason that the nickel coating sheet should be rolled before output. This conclusion matches the related law for monolithic materials[19].

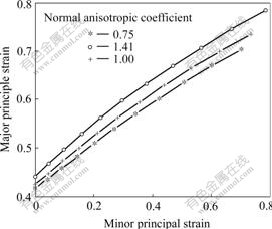
Fig.8 Effect of normal anisotropic coefficient on forming limit of coating sheet

6 Conclusions

1) The forming limit of the nickel coating sheet is between the forming limits of the individual coating and substrate, and its eigen values vary inversely linearly with the ratio of the component thickness.

2) The forming limit of the nickel coating is not so good as that of the steel substrate, and the forming limited strain of the coating sheet tends to diminish with the increase of thickness of the coating.

3) The greater the normal anisotropic coefficient of the materials is, the better the forming limit is.

References

[1] BIALUCKI P, KOZERSKI S. Study of adhesion of different plasma-sprayed coatings to aluminium [J]. Surface and Coatings Technology, 2006, 210(5): 2061-2064.

[2] ARI-GUR P, SARIEL J, VEMUGANTI S. Residual stresses and texture in Ni/SiC nanocomposite coatings [J]. Journal of Alloys and Compounds, 2007, 434/435: 704-706.

[3] ZHOU Q J, HE J Y, HAO X P, CHU W Y, QIAO L J. The effect of hydrogen on plastic deformation of electroless nickel phosphorous amorphous coating [J]. Materials Science and Engineering A, 2006, 437(2): 356-359.

[4] ZHOU L Q, LI Y P, ZHOU Y C. Forming limit of electrodeposited nickel coating in the left region [J]. Journal of Materials Engineering and Performance, 2006, 15(3): 287-294.

[5] ZHOU L Q, ZHOU Y C, PAN Y. Coating thickness variation during multistep drawing processes [J]. Journal of Materials Science, 2004, 39: 757-760.

[6] LI Yu-ping, ZHOU Li-qun, ZHOU Yi-chun. Elastic-plastic solution to stamping a thin strip on an elastic foundation [J]. Trans Nonferrous Met Soc China, 2006, 16(2): 339-345.

[7] HAUW B, DUBAR L, OUDIN J. Improvement of stamping computations by means of the identification of the bulk behaviour of coatings: Application to galvanized sheets [J]. Journal of Materials Processing Technology, 1999, 94: 23-29.

[8] PARISOT R, FOREST S, GOURGUES A F, PINEAU A, MAREUSE D. Modeling the mechanical behavior of a multicrystalline zinc coating on a hot-dip galvanized steel sheet [J]. Computational Materials Science, 2000, 19: 189-204.

[9] KIM H Y, HWANG B C, BAE W B. An experimental study on forming characteristics of pre-coated sheet metals [J]. Journal of Materials Processing Technology, 2002, 120: 290-295.

[10] ZHOU L Q, LI Y P, ZHOU Y C. Numerical analysis of electrodeposited nickel coating in multistage drawing processes [J]. Journal of Engineering Materials and Technology (ASME), 2005, 127(4): 233-243.

[11] ZHOU L Q, ZHOU Y C, PAN Y. Impact performance of electrodeposited nickel coating on steel substrate [J]. Journal of Materials Science, 2004, 39: 753-755.

[12] ZHOU L Q. Impact performance and stamping forming processes analysis for electrodeposited nickel coating [D]. Xiangtan: Xiangtan University, 2004.

[13] ZHAO Jun, LI Shuo-ben. The compound law on hardening curve of laminated sheet metal [C]//SHI Chang-xu, ed. Proceedings of the 17th International Deep Drawing Research Group Biennial Congress. Shenyang, China, 1992: 337-343.

[14] SWIFT H W. Plastic instability under plane stress [J]. Journal of the Mechanics and Physics of Solids, 1952, 1: 11-18.

[15] HU S G. Sheet cold forming theory [M]. Beijing: National Defence Industry Press, 1979: 131-133. (in Chinese)

[16] ZHAO Jun, LI Shuo-ben. Plastic instability of laminated sheet metal when stretched [J]. Chinese Journal of Mechanical Engineering, 1995, 31: 102-108. (in Chinese)

[17] WANG Z. Forming limits for sheet metals under tensile stresses [J]. Journal of Materials Processing Technology, 1997, 71: 418-421.

[18] SLATER R A C. Engineering plasticity [M]. New York: The Macmillan Press LTD, 1977: 162-165.

[19] WAN M, YANG Y Y, LI S B, Determination of fracture criteria during the deep drawing of conical cups [J]. Journal of Materials Processing Technology, 2001, 114: 109-113.

Foundation item: Project(KF0613) supported by the Open Project Program of Key Laboratory of Low Dimensional Materials & Application Technology, Ministry of Education, China; Project(06IND04) supported by the Key Foundation of Xiangtan University, China

Corresponding author: ZHOU Li-qun; Tel: +86-732-2371301; Fax: +86-732-8292210; E-mail: johnzlq@163.com

(Edited by YANG Bing)