
J. Cent. South Univ. (2019) 26: 865-872
DOI: https://doi.org/10.1007/s11771-019-4055-1
Numerical treatment for Darcy-Forchheimer flow of carbon nanotubes due to an exponentially stretching curved surface
Tasawar HAYAT1, 2, Farwa HAIDER1, Taseer MUHAMMAD3, Ahmed ALSAEDI2
1. Department of Mathematics, Quaid-I-Azam University, Islamabad 44000, Pakistan;
2. Nonlinear Analysis and Applied Mathematics (NAAM) Research Group, Department of Mathematics, Faculty of Science, King Abdulaziz University, Jeddah 21589, Saudi Arabia;
3. Department of Mathematics, Government College Women University, Sialkot 51310, Pakistan
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2019
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2019
Abstract: This article gives a numerical report to two dimensional (2D) Darcy-Forchheimer flow of carbon-water nanofluid. Flow is instigated by exponential extending curved surface. Viscous liquid in permeable space is described by Darcy-Forchheimer. The subsequent arrangement of partial differential equations is changed into ordinary differential framework through proper transformations. Numerical arrangements of governing frameworks are set up by NDSolve procedure. Outcomes of different sundry parameters on temperature and velocity are examined. Skin friction and heat transfer rate are also shown and inspected.
Key words: carbon nanotube; Darcy-Forchheimer flow; exponentially stretching curved surface; numerical solution
Cite this article as: Tasawar HAYAT, Farwa HAIDER, Taseer MUHAMMAD, Ahmed ALSAEDI. Numerical treatment for Darcy-Forchheimer flow of carbon nanotubes due to an exponentially stretching curved surface [J]. Journal of Central South University, 2019, 26(4): 865–872. DOI: https://doi.org/10.1007/s11771-019-4055-1.
1 Introduction
Carbon nanotubes (CNTs) are the minimum complex engineered creation and atomic holding outline of graphene sheet structures climbed in a condition of barrel. CNTs have extraordinary physical, electrical, warm, engineered and mechanical properties, on account of the blend of their little size, tube formed structure and tremendous surface zone. Dependent upon the amount of graphene layers, carbon nanotubes have been subdivided into single-walled (SWCNTs) and multi-walled (MWCNTs) carbon nanotubes. A typical thermal conductivity change in oil based nanofluids containing carbon nanotube is portrayed by CHOI et al [1]. XUE [2] proposed a model in light of Maxwell speculation for transport properties of CNTs-based composites. Heat transfer of liquid suspensions of multi-walled carbon nanotubes is analyzed by DING et al [3]. Further pertinent commitments toward this path can be investigated through the attempts [4–20] and different examinations therein.
Convective fluid flow and heat transfer subject to permeable space is encountered in chemical reactors, geology, drying and liquid composite molding, biological and combustion applications. Darcy built up a pioneering semi-experimental equation substantial for flow under low porosity and small velocity conditions. For high Reynolds number, nonlinearity shows up in semi- observational equation which is because of expanding part of inertial force. FORCHHEIMER [21] anticipated a changed expression in particular Darcy-Forchheimer expression by presenting nonlinear term of velocity in energy equation. MUSKAT [22] named it as Forchheimer term. Darcy-Forchheimer mixed convection in a fluid saturated porous space with thermophoresis and viscous dissipation is analyzed by SEDDEEK [23]. Having such in see, promoting important attempts on Darcy-Forchheimer model can be cited through examinations [24–30] and numerous investigations therein.
Researchers at present are engaged in examining flow instigated by an extending surface. It is because of its applications in manufacturing and industry mechanisms, for example, condensation process, annealing and tinning of copper wire, cooling of an endless metallic plate in a cooling shower, continuous stretching of plastic films, extrusion of plastic sheets and several others. Initially, CRANE [31] considered the fluid flow caused by a stretching surface. Much efforts in past have been devoted to planer stretching surface. Few studies have been only undertaken for curved stretching sheet. For example, flow of viscous fluid induced by a curved extending sheet is investigated by SAJID et al [32]. ROSCA et al [33] studied flow because of porous bended extending/contracting surface. Effects of magnetic field are present. Radiative flow of micropolar liquid by a bended extending sheet is examined by NAVEED et al [34]. ABBAS et al [35] analyzed hydromagnetic slip flow of nanofluid initiated by a bended extending sheet with heat generation and radiation. IMTIAZ et al [36] investigated impacts of homogeneous- heterogeneous responses in MHD flow caused by a bended extending sheet. Flow of micropolar liquid actuated by a bended extending surface with homogeneous-heterogeneous responses is analyzed by HAYAT et al [37]. SANNI et al [38] provided a numerical solution for flow of viscous liquid actuated by a nonlinearly extending bended surface. Flow of viscous liquid initiated by an exponentially extending bended sheet is introduced by OKECHI et al [39]. HAYAT et al [40] numerically contemplated Darcy-Forchheimer flow actuated by a bended extending sheet with Cattaneo-Christov theory and homogeneous-heterogeneous responses. Further recent examinations on bended extending surface can be cited through the investigations [41–46].
Our inspiration in current paper is covered by three novel features. Firstly to model and analyze two dimensional (2D) Darcy-Forchheimer flow of water-based carbon nanotubes initiated by an exponentially extending curved surface. Secondly to use XUE model [2] for nanofluid transport process. Thirdly to construct numerical solutions for velocity and temperature fields through NDSolve technique. The commitments of different physical parameters are examined and discussed. Furthermore, skin friction and heat transfer rate are computed and interpreted.
2 Statement
We assume steady two dimensional (2D) flow of carbon-water nanofluid. Flow is induced by an exponential extending bended sheet coiled in circle of radius R (see Figure 1). Permeable space by Darcy-Forchheimer model is considered. Here 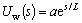 depicts the exponential velocity with a>0. Resulting boundary-layer relations for mass, momentum and energy are [44, 45]:
depicts the exponential velocity with a>0. Resulting boundary-layer relations for mass, momentum and energy are [44, 45]:
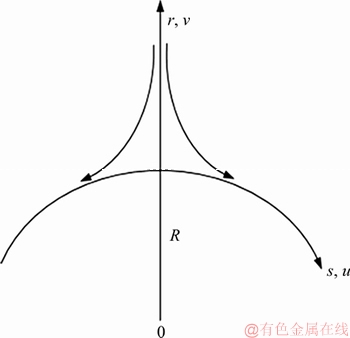
Figure 1 Geometry of problem
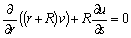 (1)
(1)
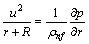 (2)
(2)
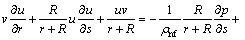
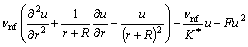 (3)
(3)
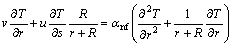 (4)
(4)
where one has the following prescribed conditions [39, 45]
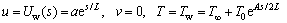
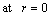 (5)
(5)
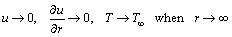 (6)
(6)
where u and v denote velocity components in s– and r– directions, K* is the permeability of porous space, Cb is the drag coefficient, 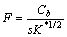 is the non-uniform inertia coefficient, Tw and T∞ are the wall and ambient temperatures respectively. Theoretical model suggested by XUE [2] is defined by
is the non-uniform inertia coefficient, Tw and T∞ are the wall and ambient temperatures respectively. Theoretical model suggested by XUE [2] is defined by
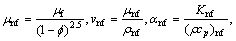
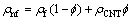 ,
,
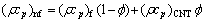 ,
,
 (7)
(7)
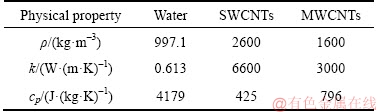
where f depicts the volume fraction of nanoparticle, μnf is the nanofluid effective nanofluids dynamic viscosity, ρnf is the nanofluids density, (ρcp)nf is the nanofluids heat capacity, knf is the nanofluids thermal conductivity, ρCNT is the carbon nanotubes density, ρf is the base fluid density, kCNT is the CNTs thermal conductivity and kf is the base fluid thermal conductivity. Table 1 represents thermo-physical features of carbon nanotubes and water.
Table 1 Thermo-physical features of carbon nanotubes and water [2]
Considering [39, 45]
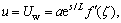
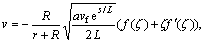
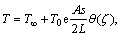
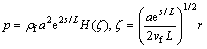 (8)
(8)
Now, Eq. (1) is automatically verified and Eqs. (2)–(7) yield
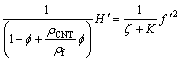 (9)
(9)
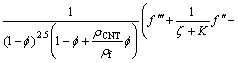
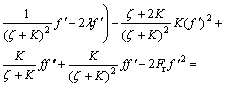
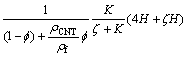 (10)
(10)
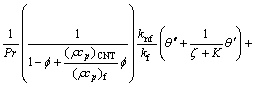
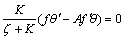 (11)
(11)
Eliminating pressure H from Eqs. (9) and (10), we get
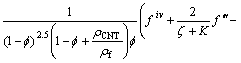
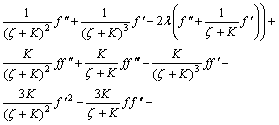
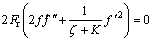 (12)
(12)
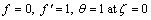 (13)
(13)
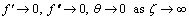 (14)
(14)
In the above relations, Pr depicts the Prandtl number, Fr is the Forchheimer number, λ is the local porosity parameter and K is the curvature parameter. These variables are:
 (15)
(15)
Skin friction coefficient and local Nusselt number are
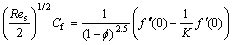 (16)
(16)
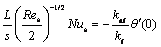 (17)
(17)
where elucidates local Reynolds number.
elucidates local Reynolds number.
3 Discussion
Local-similar arrangements of Eqs.(11) and (12) via (13) and (14) are figured numerically by utilizing NDSolve method. This portion outlines impacts of solid volume fraction of nanoparticles f local porosity parameter λ, curvature parameter K, Forchheimer number Fr and temperature exponent A on velocity f ′(ζ) and temperature θ (ζ) fields. The outcomes are accomplished for SWCNTs and MWCNTs. Features of f on velocity f ′(ζ) are plotted in Figure 2. Larger f causes an enhancement in velocity field f ′(ζ) for SWCNTs and MWCNTs. Figure 3 is interpreted to analyze impact of curvature parameter K on velocity field f ′(ζ). It is observed that an increment in K shows higher velocity field f ′(ζ) for both SWCNTs and MWCNTs. In Figure 4, it is clearly examined that lower velocity field f ′(ζ) is generated by using larger local porosity parameter λ for both SWCNTs and MWCNTs. Behavior of Forchheimer number Fr on velocity field f ′(ζ) is shown in Figure 5. Here velocity field f ′(ζ) reduces for higher Fr for both SWCNTs and MWCNTs. Figure 6 displays impact of f on temperature θ(ζ). It is revealed that increment in f enhances temperature θ(ζ) for SWCNTs and MWCNTs. The variation of curvature K on temperature field θ(ζ) is depicted in Figure 7. By increasing K, a reduction in temperature field θ(ζ) for SWCNTs and MWCNTs is observed. Figure 8 depicts that how local porosity λ affects temperature field θ(ζ). Here higher λ leads to enhancement in temperature θ(ζ) for SWCNTs and MWCNTs. Figure 9 displays that larger Forchheimer number Fr yields stronger temperature field θ(ζ) for SWCNTs and MWCNTs. Figure 10 is sketched to characterize the consequences of temperature exponent A on temperature field θ(ζ). It is noted that an increment in A causes weaker temperature field θ(ζ) for SWCNTs and MWCNTs.
Skin friction coefficient 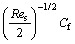 for various pertinent flow variables such as f, K and λ is plotted in Figures 11 and 12. Here skin friction is higher for increasing estimations of f for both SWCNTs and MWCNTs. Figures 13 and 14 elucidate local Nusselt number
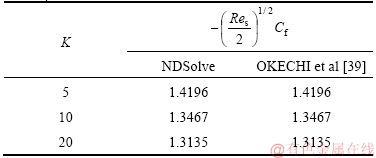
for various pertinent flow variables such as f, K and λ is plotted in Figures 11 and 12. Here skin friction is higher for increasing estimations of f for both SWCNTs and MWCNTs. Figures 13 and 14 elucidate local Nusselt number 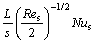 for SWCNTs and MWCNTs. We concluded that local Nusselt number is increased for higher f, K and λ. Table 2 is developed to validate present outcomes with previously published outcomes by OKECHI et al [39]. From this Table, we examined that present NDSolve solution is in good agreement with the previous solution by OKECHI et al [39].
for SWCNTs and MWCNTs. We concluded that local Nusselt number is increased for higher f, K and λ. Table 2 is developed to validate present outcomes with previously published outcomes by OKECHI et al [39]. From this Table, we examined that present NDSolve solution is in good agreement with the previous solution by OKECHI et al [39].
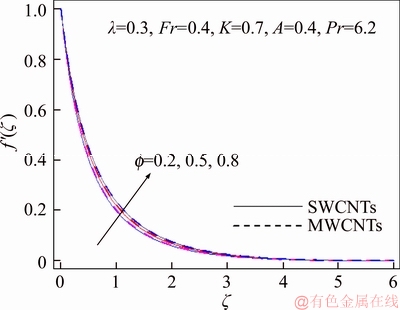
Figure 2 Plots of f ′(ζ) for f
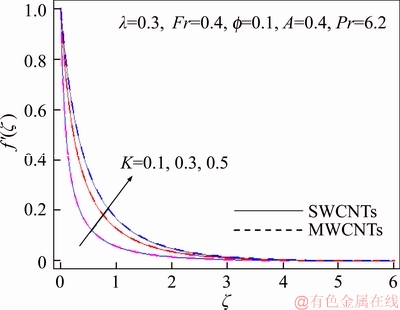
Figure 3 Plots of f ′(ζ) for K
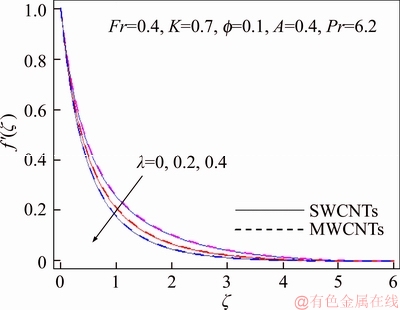
Figure 4 Plots of f ′(ζ) for λ
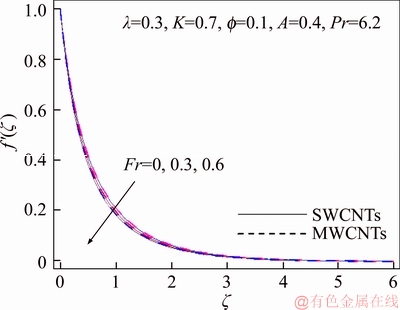
Figure 5 Plots of f ′(ζ) for Fr
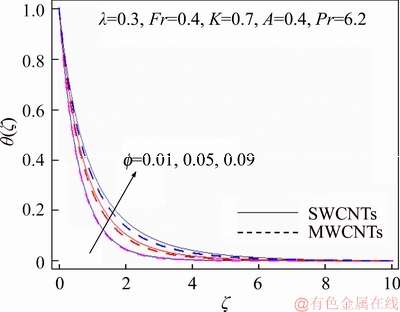
Figure 6 Plots of θ (ζ) for f
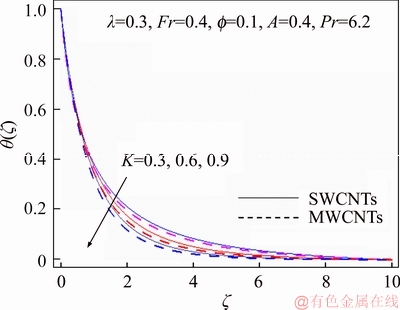
Figure 7 Plots of θ (ζ) for K
4 Conclusions
Darcy-Forchheimer flow of carbon nanotubes (CNTs) by an exponentially extending bended surface is examined. Fundamental perceptions of present examination are:
1) Opposite behavior is noted in velocity and temperature fields when local porosity parameter λ and Forchheimer number Fr are enhanced.
2) Both velocity and temperature fields show similar trend for larger solid volume fraction of nanoparticles f.
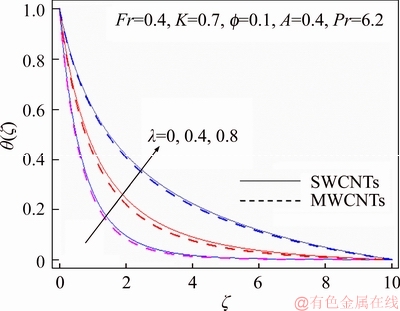
Figure 8 Plots of θ (ζ) for λ
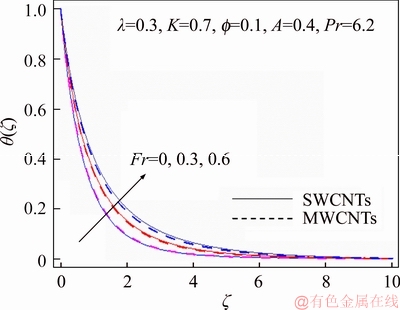
Figure 9 Plots of θ (ζ) for Fr
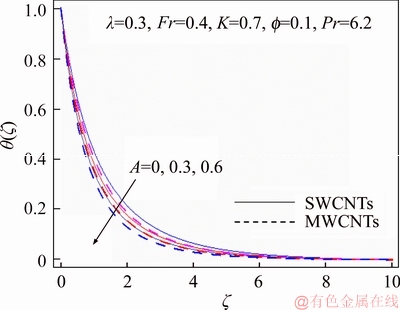
Figure 10 Plots of θ (ζ) for A
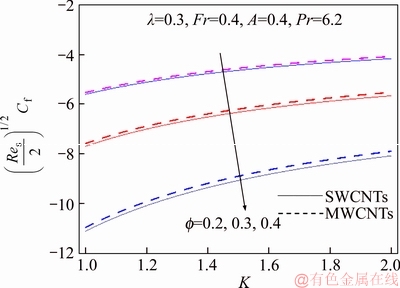
Figure 11 Plots of  for f and K
for f and K

Figure 12 Plots of  for f and λ
for f and λ
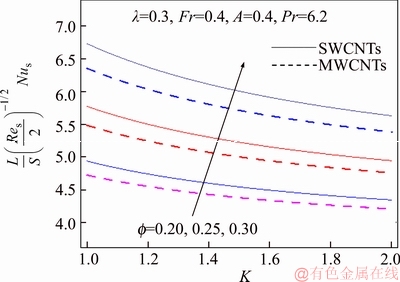
Figure 13 Plots of 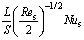 for f and K
for f and K
3) An increment in curvature parameter K yields higher velocity field.
4) Larger temperature exponent A exhibits decreasing trend for temperature.
5) Both skin friction and heat transfer rate are increased for larger f.
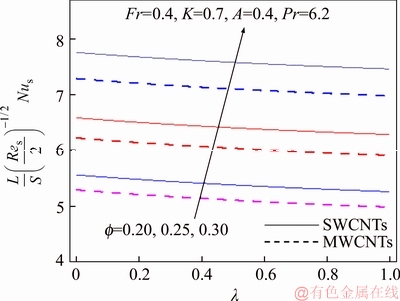
Figure 14 Plots of 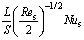 for f and λ
for f and λ
Table 2 Comparative data of 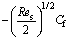 for varying K when f=λ=Fr=0
for varying K when f=λ=Fr=0
Nomenclature
u, v
Velocity components
r, s
Space coordinate
μf
Fluid dynaminc viscosity
vf
Kinematic fluid viscosity
kf
Basefluid thermal conductivity
αf
Thermal diffusivity of base fluid
Tw
Wall temperature
p
Pressure
a
Positive constant
F
Non-uniform inertia coefficient
Cb
Drag coefficient
Cf
Skin friction coefficient
λ
Local porosity parameter
Fr
Forchheimer number
K
Curvature parameter
CNTs
Carbon nanotubes
Pr
Prandtl number
R
Radius
Uw
Surface stretching velocity
ρf
Fluid density
vnf
Kinematic nanofluid viscosity
knf
Nanofluids thermal conductivity
αnf
Thermal diffusivity of nanofluid
T∞
Ambient temperature
kCNT
CNTS thermal conductivity
K*
Permeability of porous medium
f
Nanomaterial volume fraction
Res
Local Reynolds number
Nus
Local Nusselt number
ζ
Dimensionless variable
f′
Dimensionless velocities
θ
Dimensionless temperature
H
Dimensionless pressure
A
Temperature exponent
References
[1] CHOI S U S, ZHANG Z G, YU W, LOCKWOOD F E, GRUKLE E A. Anomalous thermal conductivity enhancement in nanotube suspensions [J]. Applied Physics Letters, 2001, 79: 2252.
[2] XUE Q Z. Model for thermal conductivity of carbon nanotube-based composites [J]. Physica B: Condensed Matter, 2005, 368: 302–307.
[3] DING Y, ALIAS H, WEN D, WILLIAMS R A. Heat transfer of aqueous suspensions of carbon nanotubes (CNT nanofluids) [J]. International Journal of Heat and Mass Transfer, 2006, 49: 240–250.
[4] WANG J, ZHU J , ZHANG X, CHEN Y. Heat transfer and pressure drop of nanofluids containing carbon nanotubes in laminar flows [J]. Experimental Thermal and Fluid Sciences, 2013, 44: 716–721.
[5] SAFAEI M R, TOGUN H, VAFAI K, KAZI S N, BADARUDIN A. Investigation of heat transfer enhancement in a forward-facing contracting channel using FMWCNT nanofluids [J]. Numerical Heat Transfer, Part A, 2014, 66: 1321–1340.
[6] ELLAHI R, HASSAN M, ZEESHAN A. Study of natural convection MHD nanofluid by means of single and multi walled carbon nanotubes suspended in a salt water solutions [J]. IEEE Transactions on Nanotechnology, 2015, 14: 726–734.
[7] HAYAT T, HUSSAIN Z, MUHAMMAD T, ALSAEDI A. Effects of homogeneous and heterogeneous reactions in flow of nanofluids over a nonlinear stretching surface with variable surface thickness [J]. Journal of Molecular Liquids, 2016, 221: 1121–1127.
[8] IQBAL Z, AZHAR E, MARAJ E N. Transport phenomena of carbon nanotubes and bioconvection nanoparticles on stagnation point flow in presence of induced magnetic field [J]. Physica E: Low-dimensional Systems and Nanostructures, 2017, 91: 128–135.
[9] KHAN U, AHMED N, MOHYUD-DIN S T. Numerical investigation for three dimensional squeezing flow of nanofluid in a rotating channel with lower stretching wall suspended by carbon nanotubes [J]. Applied Thermal Engineering, 2017, 113: 1107–1117.
[10] HAYAT T, HAIDER F, MUHAMMAD T, ALSAEDI A. On Darcy-Forchheimer flow of carbon nanotubes due to a rotating disk [J]. International Journal of Heat and Mass Transfer, 2017, 112: 248–254.
[11] HAYAT T, MUHAMMAD T, ALSAEDI A, ALHUTHALI M S. Magnetohydrodynamic three-dimensional flow of viscoelastic nanofluid in the presence of nonlinear thermal radiation [J]. Journal of Magnetism and Magnetic Materials, 2015, 385: 222–229.
[12] SULOCHANA C, SANDEEP N, SUGUNAMMA V, KUMAR B R. Aligned magnetic field and cross-diffusion effects of a nanofluid over an exponentially stretching surface in porous medium [J]. Applied Nanoscience, 2016, 6: 737–746.
[13] SANDEEP N, CHAMKHA A J, ANIMASAUN I L. Numerical exploration of magnetohydrodynamic nanofluid flow suspended with magnetite nanoparticles [J]. Journal of Brazilian Society of Mechanical Sciences and Engineering, 2017, 39: 3635–3644.
[14] BABU M J, SANDEEP N, SALEEM S. Free convective MHD Cattaneo-Christov flow over three different geometries with thermophoresis and Brownian motion [J]. Alexandria Engineering Journal, 2017, 56: 659–669.
[15] KUMAR M S, SANDEEP N, KUMAR B R, DINESH P A. A comparative analysis of magnetohydrodynamic non- Newtonian fluids flow over an exponential stretched sheet [J]. Alexandria Engineering Journal, 2018, 57: 2093–2100. https://doi.org/10. 1016/j.aej.2017.06.002.
[16] SUCHARITHA G, LAKSMINNARAYANA P, SANDEEP N. Joule heating and wall flexibility effects on the peristaltic flow of magnetohydrodynamic nanofluid [J]. International Journal of Mechanical Sciences, 2017, 131: 52–62.
[17] REDDY J V R, SUGUNAMMA V, SANDEEP N. Dual solutions for nanofluid flow past a curved surface with nonlinear radiation, Soret and Dufour effect [J]. Journal of Physics: Conference Series, 2018, 1000: 012152.
[18] SHEIKHOLESLAMI M, HAYAT T, MUHAMMAD T, ALSAEDI A. MHD forced convection flow of nanofluid in a porous cavity with hot elliptic obstacle by means of Lattice Boltzmann method [J]. International Journal of Mechanical Sciences, 2018, 135: 532–540.
[19] HAYAT T, MUHAMMAD K, MUHAMMAD T, ALSAEDI A, Melting heat in radiative flow of carbon nanotubes with homogeneous-heterogeneous reactions [J]. Communications in Theoretical Physics, 2018, 69: 441.
[20] HAYAT T, HAIDER F, MUHAMMAD T, ALSAEDI A. Darcy-Forchheimer squeezed flow of carbon nanotubes with thermal radiation [J]. Journal of Physics and Chemistry of Solids, 2018, 120: 79–86.
[21] FORCHHEIMER P. Water movement through ground [J]. Journal of the Association of German Engineers, 1901, 45: 1782–1788.
[22] MUSKAT M. The flow of homogeneous fluids through porous media [M]. Edwards, MI. 1946.
[23] SEDDEEK M A. Influence of viscous dissipation and thermophoresis on Darcy-Forchheimer mixed convection in a fluid saturated porous media [J]. Journal of Colloid and Interface Science, 2006, 293: 137–142.
[24] SADIQ M A, HAYAT T. Darcy-Forchheimer flow of magneto Maxwell liquid bounded by convectively heated sheet [J]. Results in Physics, 2016, 6: 884–890.
[25] BAKAR S A, ARIFIN N M, NAZAR R, ALI F M, POP I. Forced convection boundary layer stagnation-point flow in Darcy-Forchheimer porous medium past a shrinking sheet [J]. Frontiers in Heat and Mass Transfer, 2016, 7: 38.
[26] HAYAT T, MUHAMMAD T, AL-MEZAL S, LIAO S J. Darcy-Forchheimer flow with variable thermal conductivity and Cattaneo-Christov heat flux [J]. International Journal of Numerical Methods for Heat and Fluid Flow, 2016, 26: 2355–2369.
[27] UMAVATHI J C, OJJELA O, VAJRAVELU K. Numerical analysis of natural convective flow and heat transfer of nanofluids in a vertical rectangular duct using Darcy- Forchheimer-Brinkman model [J]. International Journal of Thermal Sciences, 2017, 111: 511–524.
[28] HAYAT T, HAIDER F, MUHAMMAD T, ALSAEDI A. Darcy-Forchheimer flow due to a curved stretching surface with Cattaneo-Christov double diffusion: A numerical study [J]. Results in Physics, 2017, 7: 2663–2670.
[29] HAYAT T, AZIZ A, MUHAMMAD T, ALSAEDI A. Darcy-Forchheimer three-dimensional flow of Williamson nanofluid over a convectively heated nonlinear stretching surface [J]. Communication in Theoretical Physics, 2017, 68: 387.
[30] MUHAMMAD T, ALSAEDI A, SHEHZAD S A, HAYAT T. A revised model for Darcy-Forchheimer flow Maxwell nanofluid subject to convective boundary condition [J]. Chinese Journal of Physics, 2017, 55: 963–976.
[31] CRANE L J. Flow past a stretching plate [J]. Journal of Applied Mathematics and Physics (ZAMP), 1970, 21: 645–647.
[32] SAJID M, ALI N, JAVED T, ABBAS Z. Stretching a curved surface in a viscous fluid [J]. Chinese Physics Letters, 2010, 27: 024703.
[33] ROSCA N C, POP I. Unsteady boundary layer flow over a permeable curved stretching/shrinking surface [J]. European Journal of Mechanics-B/Fluids, 2015, 51: 61–67.
[34] NAVEED M, ABBAS Z, SAJID M. MHD flow of a microploar fluid due to curved stretching surface with thermal radiation [J]. Journal of Applied Fluid Mechanics, 2016, 9: 131–138.
[35] ABBAS Z, NAVEED M, SAJID M, Hydromagnetic slip flow of nanofluid over a curved stretching surface with heat generation and thermal radiation [J]. Journal of Molecular Liquids, 2016, 215: 756–762.
[36] IMTIAZ M, HAYAT T, ALSAEDI A, HOBINY A. Homogeneous-heterogeneous reactions in MHD flow due to an unsteady curved stretching surface [J]. Journal of Molecular Liquids, 2016, 221: 245–253.
[37] HAYAT T, SAJJAD R, ELLAHI R, ALSAEDI A, MUHAMMAD T. Homogeneous-heterogeneous reactions in MHD flow of micropolar fluid by a curved stretching surface [J]. Journal of Molecular Liquids, 2017, 240: 209–220.
[38] SANNI K M, ASGHAR S, JALIL M, OKECHI N F. Flow of viscous fluid along nonlinearly stretching curved surface [J]. Results in Physics, 2017, 7: 1–4.
[39] OKECHI N F, JALIL M, ASGHAR S. Flow of viscous fluid along an exponentially stretching curved surface [J]. Results in Physics, 2017, 7: 2851–2854.
[40] HAYAT T, SAIF R S, ELLAHI R, MUHAMMAD T, AHMAD B. Numerical study for Darcy-Forchheimer flow due to a curved stretching surface with Cattaneo-Christov heat flux and homogeneous-heterogeneous reactions [J]. Results in Physics, 2017, 7: 2886–2892.
[41] HAYAT T, SAIF R S, ELLAHI R, MUHAMMAD T, AHMAD B. Numerical study of boundary-layer flow due to a nonlinear curved stretching sheet with convective heat and mass conditions [J]. Results in Physics, 2017, 7: 2601–2606.
[42] HAYAT T, AZIZ A, MUHAMMAD T, ALSAEDI A. Numerical study for nanofluid flow due to a nonlinear curved stretching surface with convective heat and mass conditions [J]. Results in Physics, 2017, 7: 3100–3106.
[43] IMTIAZ M, HAYAT T, ALSAEDI A. Convective flow of ferrofluid due to a curved stretching surface with homogeneous-heterogeneous reactions [J]. Powder Technology, 2017, 310: 154–162.
[44] HAYAT T, RAFIQUE K, MUHAMMAD T, ALSAEDI A, AYUB M. Carbon nanotubes significance in Darcy- Forchheimer flow [J]. Results in Physics, 2018, 8: 26–33.
[45] HAYAT T, HAIDER F, MUHAMMAD T, ALSAEDI A. Numerical study for Darcy-Forchheimer flow of nanofluid due to an exponentially stretching curved surface [J]. Results in Physics, 2018, 8: 764–771.
[46] JAVED M, FAROOQ M, AHMAD S, ANJUM A. Melting heat transfer with radiative effects and homogeneous- heterogeneous reaction in thermally stratified stagnation flow embedded in porous medium [J]. Journal of Central South University, 2018, 25: 2701–2711.
(Edited by HE Yun-bin)
中文导读
碳纳米管指数拉伸曲面上Darcy-Forchheimer流动的数值处理
摘要:本文对碳水纳米流体的二维流动进行了数值模拟。流体是由指数扩展曲面引起的。本文用Darcy-Forchheimer方法描述了渗透空间中的黏性液体。通过适当的变换,将偏微分方程的后续排列转化为常微分框架。采用NDSolve程序建立了控制框架的数值排布。考察了不同参数对温度和速度的影响, 并对表面摩擦和传热速率进行监测。
关键词:碳纳米管;Darcy-Forchheimer流;指数拉伸曲面;数值解
Received date: 2018-05-06; Accepted date: 2018-10-23
Corresponding author: Taseer MUHAMMAD, PhD, Assistant Professor; Tel: +92-321-7132571; E-mail: taseer_qau@yahoo.com