Application of Anand’s constitutive model on twin roll casting process of  AZ31 magnesium alloy

HU Xiao-dong(胡小东)¹, JU Dong-ying(巨东英)^{1, 2}

1. Department of Material Science and Engineering,Saitama Institute of Technology, Fusaiji 1690, Fukaya, Japan

2. Research Center of High Technology,Anshan University of Science and Technology, Anshan 114044, China

Received 10 April 2006; accepted 25 April 2006

Abstract: Twin-roll thin strip casting process combines casting and hot rolling into a single process, in which thermal stress and thermal mechanical stress were involved. Considering the high temperature gradient, the existing of liquid and solid regions and rolling deformation, suitable constitutive model is the key to describe the process. Anand’s model is a temperature-dependent, rate-dependent and unified of creep and plasticity model and the Jaumann derivative was employed in Anand’s model which makes the constitutive model frame-indifferent or objective, therefore the highly nonlinearities behavior in the twin-roll casting process can be simulated. The parameters of the Anand’s model were regressed based on the compression tests of AZ31 magnesium alloy. The simulation results reveal that the Anand’s model can well describe the deformation characteristics of twin-roll casting process. Based on the simulation results, the form of evolution equations in Anand’s model was discussed.

Key words: AZ31 Mg alloy; twin-roll casting; thin strip; visco-plasticity; Anand’s constitutive model; magnesium alloy

1 Introduction

Twin-roll casting process is a rapidly solidification process combining with hot rolling. In the process the molten metal is solidified starting at the point of first metal-roll contact and ending before the kissing point. This near-net-shape process can directly produce thin strips in one step. It has more advantages due to its higher productivity, low cost and energy saving. Therefore, more and more researchers have concentrated their studies on the process. But the most of researches were focus on the thermal flow process and a few on thermal mechanical stresses generating in the twin-roll casting process [1-3]. Calculation stresses is difficult and complex because the high temperature gradient and the existing of melt and solid state in the casting region. Therefore rolling action was simplified or not to be considered and only thermal stresses were calculated [4,5].

There are some important aspects in stresses modeling that must to be considered [6]. These include the effects of anisotropic properties, phase transformations, interaction with the mold, fluid flow, temperature dependence of elastic modulus, liquid properties, combined creep and plasticity, microstructure effects, mesh refinement, and two dimensional stress state. In this work, we focuses the research work on the constitutive equations, other aspects will be simplified. So a 2D FEM model was employed to simulate the thermal-mechanical behavior during twin-roll casting process of Mg alloy AZ31. Firstly, the thermal fluid flow of twin-roll casting process was simulated by a CFD model based on ANSYS FLOTRAN; then the inelastic solid model based on Anand’s model was employed to calculate the thermal stress in casting process. The stresses results combining with temperature filed and visco-plastic deformation were obtained. Finally, the form of evolution equations in Anand’s model was discussed.

2 Thermal flow simulation

The schematic drawing of twin-roll casting process is shown in Fig.1. Considering symmetry, half of the twin-roll casting model was employed. The governing

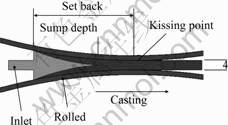
Fig.1 Schematic diagram of twin-roll casting process

Navier-Stokes equation combined with the continuity equation, the thermal transport equation and the constitutive property relationship is given by Eqn.(1)

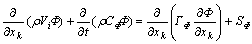     (1)

where  ρ is the density, Ф represents the dependent variable and C_Ф, Γ_Ф and S_Ф indicate transient items, diffusion items and source items, respectively.

The 2D geometry, loads, and constraints were defined as follow: the roll gap was set at 4 mm; roll diameter 425 mm; the casting speed was 1 m/min; casting temperature 973 K; set back distance 42 mm; strip/roll heat transfer coefficient 10 kW/(m²?K). The material parameters of AZ31 are as follows: density1 810 kg/m³; solidus 848 K; liquidus 903 K; heat conductivity 51 W/(m?K); latent heat 372 kJ/(kg?K); The viscosity was temperature dependent and equivalent specific heat method was adopted to deal with latent heat. Following assumptions were made for thermal flow simulation: heat transfer coefficient on the strip/roll interface was 10 kW/(m²?K); rollers surface temperature  was 373 K; side dams and nozzle were adiabatic; non-slip conditions were assumed on roll/metal interface. Based on the assumptions steady-state simulations were performed. The temperature field result of twin-roll casting process is shown in Fig.2.

Fig.2 Temperature field results of twin-roll casting process

2 Strain rates

In twin-roll thin strip casting process, stresses primarily arise due to high thermal gradient and rolling deformation. The total strain ε_ij can be decomposed in Eqn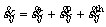.(2):

                          (2)

where 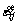 ,  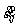and 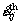 are elastic, plastic and thermal strain rate, respectively.

The stresses rate related to the elastic strain rate:

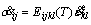                            (3)

where  E_ijkl(T) is the temperature dependent elastic modulus.

Thermal strain rate is given by:

                              (4)

where 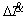  is the change rate of current temperature and the reference temperature at the point, α is thermal coefficient of expansion.

For high temperature and low strain rate deformation process, the inelastic strain usually consists of plastic and creep strain, which is significant and hard to be distinguished from plastic strain. So constitutive model unified creep and plasticity should be adopted. Anand’s model is one of the kinds of unified models.

3 Anand’s model

A set of internal type constitutive equations for large elastic-viscoplastic deformation at high temperature was proposed by BROWN et al [7-9]. There are two basic features in this model. First, this model needs no explicit yield condition and no loading/unloading criterion. The plastic strain is assumed to take place at all nonzero stress values, although at low stresses the rate of plastic flow may be immeasurably small. Second, this model employs a single scalar as an internal variable to represent the isotropic resistance to plastic flow offered by the internal state of the material. The internal variable s represents an averaged isotropic resistance to macroscopic plastic flow offered by the underlying isotropic strengthening mechanisms. The deformation resistance s is consequently proportional to the equivalent stress σ. That is

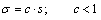
where σis the equivalent stress and c is a material parameter which is constant in the constant strain rate and can be defined as

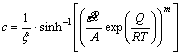               (5)

where 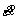   is effective inelastic strain rate, A is the pre-exponential factor, Q is the activation energy, m is the strain rate sensitivity, ξ is the multiplier of stress, R is the gas constant and T is the absolute temperature, respectively.

The flow equation is

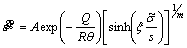              (6)

and the evolution equation for the internal variable s:

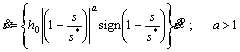       (7)

with

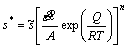                       (8)

where  h₀ is the hardening constant, A is the strain rate sensitivity of hardening, s* is the saturation value of s, 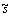 is a coefficient, and n is the strain rate sensitivity for the saturation value of deformation resistance, respectively. The nine parameters of Anand constitutive model A, Q, ξ, m, h₀, 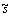, n, a and s₀ can be obtained from compression tests, by which large strains can be achieved due to the absence of necking and fully developed plastic flow. Isothermal constant true strain rate tests of AZ31 alloy with different strain rates and temperatures were carried out the true strain versus stress curves are shown in Fig.3.

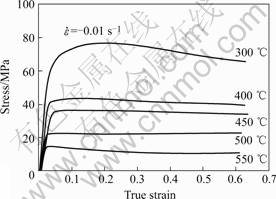
Fig.3 AZ31 compression true strain vs stress curves at different temperatures and strain rates -0.01 s^-1

The Anand’s model can be divided into two parts. The first part is to obtain the predictions for the relationship of saturation stress with different temperature and strain rates based on flow equation (Eqn.6), and the second part is to accurate simulate the stress-strain curve i.e. transient region as shown in Fig.4 based on the internal variable evolution equation (Eqn.7).

Using Eqn.8, the flow Eqn.(6) can be rearranged for the saturation stress as

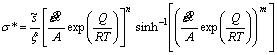 (9)

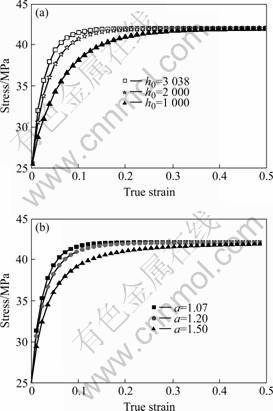
Fig.4 Effects of hardening parameters h₀ (a) and a (b) on transient region of stress—strain curve

From Eqn.9, five material constants, Q/R, A, s, 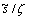 , m and n can determined by a nonlinear least square fitting of  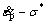from the experimental results. The parameterξis chosen such that the constant c is less than unity (Eqn.5). Then  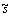can be determined from the combination term .

The other three constants can be determined from the evolution equation. From Eqn.7 and the given relationσ=c s, the internal variable of the evolution equation can be expressed in terms of stress as

                       (10)

or equivalently

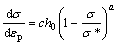                        (11)

The integrated form of Eqn.10 can be written as

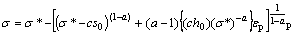        (12)

Three material constants h₀, a and s₀ of Eqn.12 can be determined by applying a nonlinear least square fitting of ε_p-σ curves near the transient region. Fig.4 shows the effects of parameters h₀ and a on the transient region of stress-strain curves.

The set of constants were obtained for each constant strain rate test and they were averaged. They are A: 3.5×10⁷ s^-1 , Q: 160 kJ/mol, ξ: 8.5, m: 0.28, h₀: 3 038 MPa, n: 0.018, a: 1.07, s₀: 35 MPa, 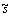: 50 MPa. The prediction of stress—strain relations by Anand model is shown on Fig.5.

Fig.5 AZ31 Anand model prediction at different temperatures and strain rates -0.01 s^-1

4 Thermal stresses

Since both liquid and solid regions are part of calculation domain, a special procedure is employed to handle the liquid region [4]. A value of Poisson’s ratio very close to 0.5 is assigned at the nodes where temperature is above the coherence temperature. This makes the liquid phase close to being incompressible for mechanical loading. The elastic modulus is set to a very small number at the nodes above the coherence temperature.

ANSYS structural model, in which Anand model was built-in, was employed to calculate stresses. The thermal flow result of temperature field was imposed as body load and the reference temperature was set as the average temperature of strip surface [5]. The strip surface set as free surface because of solidifying shrinkage. The thermal stresses results are shown in Fig.6. The stress status of strip surface along casting direction is tensile stress; this is one of main reasons causing strip crack defects.

5 Thermal mechanical stresses

To simulate rolling action in twin-roll casting

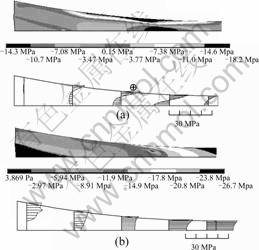
Fig.6 Contours and distributions of σ_x (a) and Von Mises  stresses (b) caused by thermal gradient

process, displacement load along roller tangent direction was imposed. The results of stresses and deformation are shown in Figs.7 and 8, respectively.

6 Discussion

The deformation of twin-roll casting process is very similar to rolling process. Nature point, forward slip and backward slip are existed. High temperature gradient makes the strip center deformation great than surface deformation. This non-uniform deformation will increase strip surface additional tensile stress and may cause surface crack defect. The rolling stress is much higher than thermal stress. Control the solidification end near the kissing point can decrease rolling deformation and decrease the crack tendency.

The parameter s₀ is the averaged value of deformation resistance s at each test temperature, it is temperature dependent. But in ANSYS the parameter is set as constant, this will result some deviation. XU et al suggest the following forms for s₀ and h₀ [10], this can effectively improve the prediction ability of Anand model.

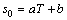                                (13)

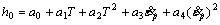         (14)

The assumption of no slip condition between roll/metal interfaces is not accurate in twin-roll casting process, this will result in some increased compress stress level.

In multidimensional case, the evolution equation of Anand model can be expressed as Eqn.15, the stress employs the Jaumann derivative which renders this model properly frame-indifferent.

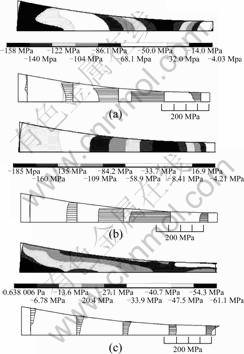
Fig.7 Thermal mechanical stresses contours and distributions: (a) σ_x; (b) σ_y; (c) Von mises

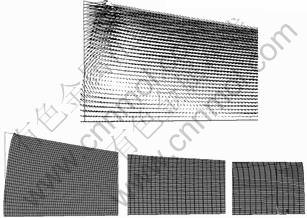
Fig.8 Translation vector display in molten region and deformation results

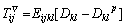                      (15)

with

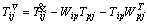                   (16)

where 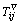 is Jaumann derivative of Cauchy stress T_ij, E_ijkl is elasticity modulus, D_kl is stretching tensor, W_ip is the spin tensor. This means that Anand model is suitable for simulating large deformation at high temperature, which can be seen from Fig.8.

7 Conclusions

1) The deformation of twin-roll casting process is non-uniformed, which will result in additional tension stress in strip surface and increase the tendency of surface creak.

2) The rolling stress is much higher than thermal stress. Controlling the solidification end near the kissing point can decrease the crack tendency.

3) Anand’s model is suitable for stresses and large deformation analysis for twin-roll casting process.

The support provided by High Technology Research Center in Saitama Institute of Technology for this work is gratefully acknowledged.

[1] JU D Y, ZHAO H Y, HU X d. Thermal flow simulation on twin roll casting process for thin strip production of magnesium alloy [J]. Materials Science Forum, 2005, 488-489: 439-444.

[2] Daaland O, Espedal A B, Nedreberg M L, Alvestad I. Thin gauge twin-roll casting pess capabilities and product quality [A]. Light Metals TMS[c]. Seattle: TMS, 1997. 745-752.

[3] hwang j d, lin h j, huang w s, hu c t. Numerical simulation of metal flow and heat transfer during twin roll stripp casting [J]. ISIJ Int 1995, 35(2): 170-177

[4] Gupta M, Sahai Y. mathematical modeling of thermally induced stresses in two-roll melt drag thin strip casting of steel [J]. ISIJ Int 2000, 40(2): 137-143.

[5] Sahai Y, Saxena A. modeling of twin-roll thin strip casting of aluminum alloys [A]. Light Metals TMS[c]. Seattle: TMS, 2002. 643-650.

[6] THOMAS B G. Issues in thermal-mechanical modeling of casting processes [J]. ISIJ Int, 1995, 35(6): 737-743.

[7] Brown S B, Kim K H, Anand L. An internal variable constitutive model for hot working of metals [J]. Int J Plasticity, 1989, 5: 95-130.

[8] Lush A M, Weber G, Anand L. An implicit time-integration procedure for a set of internal variable constitutive equatuions for isotropic elasto-viscoplasticity [J]. Int J Plasticity, 1989, 5: 521-549.

[9] Weber G G, Lush A M, Zavaliangos A, Anand L. An objective time-integration procedure for isotropic rate-independent and rate-dependent elastic-plastic constitutive equations [J]. Int J Plasticity, 1990, 6: 701-744.

[10] Xu C, chen G, sakane M. Prediction of stress—strain relationship with an improved anand constitutive model for lead-free solder Sn-3.5Ag [J]. IEEE Transactions on Components and Packaging Technologies, 2005, 28(1): 111-116

(Edited by ZHAO Jun)

Corresponding author: JU Dong-ying; Tel: +81-48-595-6826; Fax: +81-48-585-5928; E-mail: dyju@sit.ac.jp