Transient numerical simulation of annealing process in a conjugate combined radiation conduction heat transfer
来源期刊:中南大学学报(英文版)2020年第9期
论文作者:M. Foruzan NIA S. A. Gandjalikhan NASSAB
文章页码:2662 - 2672
Key words:conjugate; radiation; conduction; transient annealing process
Abstract: The annealing time is an important affecting factor in the performance of many furnaces. The present work deals with the transient simulation of annealing process in a cubic furnace in which a solid element is placed in its center. As the working gas can have some radiating features, a set of governing equations including the energy balance with the radiative transfer equation (RTE) for the gray radiating medium and the conduction equation inside the solid product are numerically solved with progressing in time. Numerical results which are validated against both analytical and theoretical findings in the literature demonstrate that during the starting period, a high rate of radiant energy transfers into the solid body even at small optical thickness. This behavior which hastens the rate of heat transfer at low values of the radiation conduction parameter, causes a fast annealing process in which the solid body warms up to its maximum temperature. Moreover, it is revealed that the rate of heat transfer is an increasing function of radiation-conduction parameter.
Cite this article as: M. Foruzan NIA, S. A. Gandjalikhan NASSAB. Transient numerical simulation of annealing process in a conjugate combined radiation conduction heat transfer problem [J]. Journal of Central South University, 2020, 27(9): 2662-2672. DOI: https://doi.org/10.1007/s11771-020-4489-5.

J. Cent. South Univ. (2020) 27: 2662-2672
DOI: https://doi.org/10.1007/s11771-020-4489-5
M. Foruzan NIA, S. A. Gandjalikhan NASSAB
Mechanical Engineering Department, Shahid Bahonar University of Kerman, Kerman 7616913439, Iran
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020
Abstract: The annealing time is an important affecting factor in the performance of many furnaces. The present work deals with the transient simulation of annealing process in a cubic furnace in which a solid element is placed in its center. As the working gas can have some radiating features, a set of governing equations including the energy balance with the radiative transfer equation (RTE) for the gray radiating medium and the conduction equation inside the solid product are numerically solved with progressing in time. Numerical results which are validated against both analytical and theoretical findings in the literature demonstrate that during the starting period, a high rate of radiant energy transfers into the solid body even at small optical thickness. This behavior which hastens the rate of heat transfer at low values of the radiation conduction parameter, causes a fast annealing process in which the solid body warms up to its maximum temperature. Moreover, it is revealed that the rate of heat transfer is an increasing function of radiation-conduction parameter.
Key words: conjugate; radiation; conduction; transient annealing process
Cite this article as: M. Foruzan NIA, S. A. Gandjalikhan NASSAB. Transient numerical simulation of annealing process in a conjugate combined radiation conduction heat transfer problem [J]. Journal of Central South University, 2020, 27(9): 2662-2672. DOI: https://doi.org/10.1007/s11771-020-4489-5.
1 Introduction
Annealing is a thermal treatment process used for removing residual stress in metals being hardened during one or series of metal shaping, such as rolling, starching and forming. Due to the nature of this heat treatment, the metal should be kept at the proper temperature for a specified time. So, annealing process has a transient heat transfer nature in which the mechanism of heat transfer should be comprehensively analyzed. Due to the design of some annealing furnaces in which the process is done in the absence of forced convection, the investigation can consist of combined radiation and conduction. Besides, many furnaces in laboratories or metal turning workshops have a cubic shape and are heated by electric heating elements (Figure 1). So, all boundaries in terms of heat transfer can be considered in the same condition in 2-D analysis and the effects of natural convection can be ignored because of small Grashof number. In this regard, a significant amount of investigation has been done on combined conduction and radiation heat transfer in steady state condition [1, 2]. A hybrid method was employed by MAHAPATRA et al [3] to scrutinize the thermal characteristics of combined radiation and conduction heat transfer in a participating medium. There are several research works regarding numerical simulation of combined radiation and conduction heat transfer in two- and three-dimensional enclosures with complex geometries [4, 5].
As far as transient problem is considered, LICK [6] studied the transient radiation-conduction heat transfer in semi-infinite medium and obtained analytical solution by using the Kernel substitution technique. CHANG et al [7] considered a medium between two concentric cylinders, and they studied the combination of radiation and conduction heat transfer in both transient and steady state condition. LII et al [8] studied the radiation and conduction heat transfer in participating slab in transient condition. Using collected grid to solve radiation transfer equation and implicit finite difference scheme for conduction part, TSAI et al [9] analyzed transient heat transfer in a solid sphere. Transient conduction-radiation heat transfer in a slab was solved by TSENG et al [10]. In this case, thermal conductivity was a function of temperature, and their method was based on deferential discrete ordinate method for RTE and fully implicit time marching algorithm for energy equation. LIU et al [11] studied transient conduction and radiation in 2-D semitransparent cylinder subjected to pulse irradiation at boundary based on implicit central difference scheme and discrete ordinate method for energy and RTE equations, respectively. Coupled radiation and conduction in infinite cylinders with isothermal black wall was investigated by LIU [12] in transient condition. The RTE was solved by radiative transfer coefficient and the energy equation by implicit finite difference scheme. Combined radiation and conduction heat transfer in fibrous medium that can be attributed to anisotropic optical properties was investigated by ASLLANJ et al [13]. In that case, the RTE was numerically solved by applying the collapsed dimension method, and energy equation was estimated by direction implicit scheme. In a 2D rectangular enclosure, MISHRA et al [14] studied the effect of variable thermal conductivity in transient conduction-radiation heat transfer. MISHRA et al [15] considered transient conduction and radiation heat transfer in 1D planar and 2D rectangular medium. They studied the efficiency of combining finite volume method and the Lattice Boltzmann method for solving RTE and energy equation, respectively. Hybrid ray tracing method was used by YI et al [16] to study heat transfer in transient condition for plane parallel participating medium. Transient modeling of combined conduction and radiation heat transfer in wood wool insulation, which is used in buildings, was developed by KAEMMERLEN et al [17]. A parametric study of transient conduction radiation in two-dimensional enclosure was done by CHAOBAN et al [18]. Their method was based on combination of control volume finite element and the Lattice Boltzmann. They also studied transient conduction radiation heat transfer in a two dimensional rectangular and cylindrical medium using the Lattice Boltzmann method [19, 20]. Study of combined radiation and conduction in transient mode, which is mentioned and cited, is limited to cavities filled with radiating gases, but annealing furnaces also contain some solid body under heat treatment process. It is worthwhile noting that in many types of annealing furnaces, a special filling gas, such as nitrogen or combination of gases is charged, because oxygen leads to oxide formation in the annealed body, which is detrimental. Therefore, the mechanisms of heat transfer in the working gas are conduction, radiation and free convection, of which the last mechanism is ignored in the simulation because of small convection coefficient; moreover, as the radiation heat transfer is dominant, the effects of gas radiation in term of scattering, emission and absorption are an important issue.
In order to study the effects of the working gas on these kinds of annealing furnaces, a cavity filled with a working gas and a solid body at its center is considered. Although the geometry of this study is simple, the main contribution is investigating the effects of the gas radiation on the time of heating and the temperature distribution. Toward this end, the radiative transfer equation and energy equation are solved in the transient condition by the finite difference technique and discrete ordinate method, respectively. Heat transfer in the solid body is pure conduction while cavity is occupied by an absorbing, emitting and scattering medium, where heat is transferred by conduction and radiation, simultaneously. By applying the aforementioned technique, the temperature distribution inside the solid body, which is desired to be annealed, can be obtained at each time step. In order to check the accuracy of the calculations, the numerical results are validated with findings in several relevant previous investigations.
2 Mathematical modeling
In this paper, the transient heat transfer process from the surrounding heated walls toward the solid element located at center of furnace via the radiating gas is numerically simulated. Owing to the geometric features of the furnace, a two- dimensional model is considered. A simple schematic of the computational domain is shown in Figure 1(b), in which L=4LRef, L′=LRef. Also, all boundaries are considered to be opaque, diffuse and gray.

Figure 1 Schematic of annealing furnace:
The unsteady form of energy equation for 2-D combined conduction and radiation heat transfer and in the absence of convection and heat generation can be written as follows:
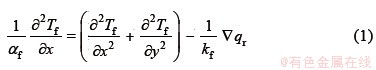
In the solid medium, the energy equation is as follows:
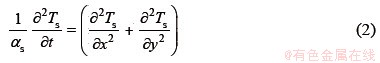
where αs and αf are the thermal diffusivities for solid and participating media, respectively.
The radiative term in the energy equation i.e.,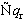 can be computed by the following equation [21]:
can be computed by the following equation [21]:
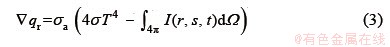
In Eq. (3), I(r, s, t) is the radiation intensity at position r, in direction s and at time t.
The radiative term presented in the gas energy equation,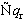 is a function of radiant intensity field. Therefore, it is needed to solve the radiative transfer equation at first. This integro-differential equation for an emitting, absorbing and scattering medium has the following form [22]:
is a function of radiant intensity field. Therefore, it is needed to solve the radiative transfer equation at first. This integro-differential equation for an emitting, absorbing and scattering medium has the following form [22]:
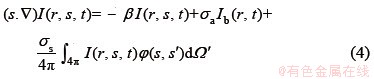
The boundary condition for a diffusely emitting and reflecting gray wall is:
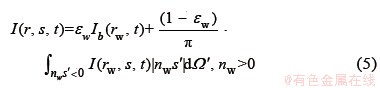
In Eq. (5), εwis the wall emissivity which is considered to be 0.8, Ib(rw, t) is the black body radiation intensity at the temperature of the boundary surface at any time, and nw is the outward unit vector normal to the surface. Since the RTE depends on the temperature fields through the emission term Ib(rw, t), it must be solved simultaneously with energy equation. RTE is an integro-differential equation which can be solved in quasi steady form with discrete ordinates method (DOM). The detail of this method was reported in the previous work by the first author and is omitted here for space saving [22].
3 Non-dimensional forms of energy equations
To obtain the non-dimensional equation, the following dimensionless parameters are introduced and used in simulation:
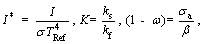
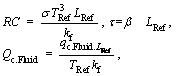
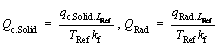 (6)
(6)
where RC is the radiation conduction parameter.
By using the aforementioned parameters, the non-dimensional forms of the energy equations in participating and solid media can be presented as follows:
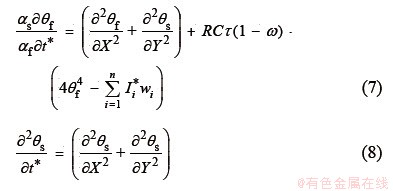
3.1 Boundary and initial conditions in non- dimensional forms
The Dirichlet boundary conditions are considered for the isotherm furnace walls as follows:
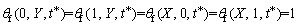 (9)
(9)
On the interface surface between the participating medium and solid element, the following criteria based on the continuity of temperature and heat flux must be imposed [23]:
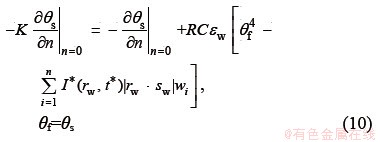
where n is the normal vector to the solid walls.
The solution procedure starts from t*=0 inside the computational domain while θf(X, Y, 0)=θs(X, Y, 0)=0.5 and is continued until steady state condition is reached. Obviously, at this state, the temperatures of radiating medium inside the furnace and also the solid body reach to the furnace wall temperature, i.e.,θf(X, Y, ∞)=θs(X, Y, ∞)=1.
3.2 Main physical quantities
In this work, the transient thermal characteristics of the annealing furnace are under study, and the effects of optical thickness and radiation-conduction (RC) parameter which are two important radiation factors, have been investigated. A focus is made on verifying the temperature distribution inside the solid element from starting time toward the steady state position. The average total heat flux to solid body in the furnace is obtained with the following relation:

where n is the normal vector to the boundary surfaces and
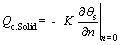 (12)
(12)
At t*, the radiation heat flux from the walls is calculated as follows:
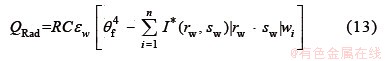
and its conduction counterpart is:
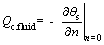 (14)
(14)
So, the total of heat flux from the heated walls of the furnace can be calculated as:
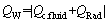 (15)
(15)
4 Numerical method
The finite difference method is used for discretizing the energy equations and the time linearization technique is employed for handling the nonlinear terms [14]. The linearized forms of Eq. (7) for calculating the gas temperature at time steps m+1 and m+2 obtained based on the ADI technique are formulated as [24]:
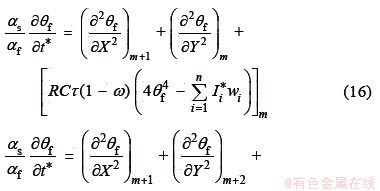
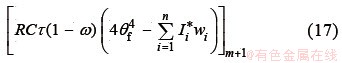
The discretized forms of Eqs. (16) and (17) are solved by using the tridiagonal matrix algorithm (TDMA) and the successive over-relaxation (SOR) technique.
In the calculation of solid temperature, similar method is used, in a way that the linearized forms of the conduction equation along with the ADI technique are formulated as follows:
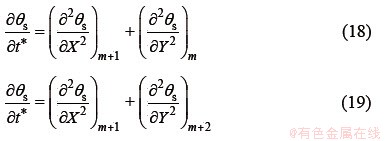
Accordingly, the following equation is imposed at the interface:
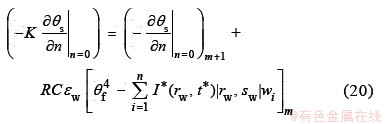
Numerical calculations are carried out by a computer program in FORTRAN. In order to obtain the grid-independent solutions, several discretized computational domains with different grid sizes and also different time steps were examined and the results of grid test are shown in Figure 2. This figure depicts the total heat flux distribution along the furnace wall at different mesh sizes. It should be mentioned that because of the symmetry, all of the furnace walls have the similar boundary and thermal behavior. According to Figure 2, the grid size of 120×120 is chosen as the optimal one for obtaining the grid independent solutions. Accordingly, the optimum time step equal to 10-4 is used. In order to simulate the solid phase in the computational domain, the blocked off method was employed. The detail of this method was described in the previous work by the second author [25]. Numerical solutions are obtained iteratively, in a way that iterations repeat until the sum of the absolute residuals become less than 10-5 for energy equations in the solid and gas phases at each time step. Whereas, in the numerical solution of RTE, the maximum difference between the radiative intensities is calculated during two consecutive iteration levels do not exceed 10-6 at each nodal point for the converged solution To check the accuracy of presented numerical method, two benchmark problems have been solved and the results were compared to exact and numerical findings reported in the literature.
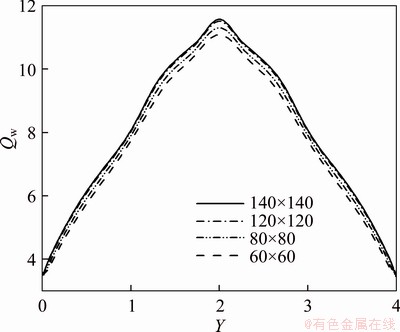
Figure 2 Total heat flux distributions along annealing furnace wall for different grid sizes t*=1, RC=100, ω=0.5, τ=1
Regarding first test case, the transient conduction in a square domain with L=π mm is simulated. All temperature of the surroundings walls are kept at zero, and the initial temperature is considered as:
T(x, y, 0)=10sinxsiny (21)
The analytical solution of the transient conduction equation leads to the following temperature distribution [26]:
T(x, y, t)=10sinxsiny-e-2t (22)
In Figure 3, the present numerical results are compared to exact solution which was reported in Ref. [26]. This figure shows the temperature distributions along the horizontal mid-line inside the square domain at different time steps. It is clear that because of the imposed boundary and initial conditions, the problem has two symmetries regarding both vertical (x=L/2) and horizontal (y=L/2) mid-lines. As seen in Figure 3, the results have good agreement with the exact solution.
With regard to the second case, combined transient conduction and radiation heat transfer is simulated. It is a 2D cavity considered by MISHRA et al [14]. The non-dimensional temperature distributions across the vertical mid line at different non-dimensional times are presented in Figure 4.
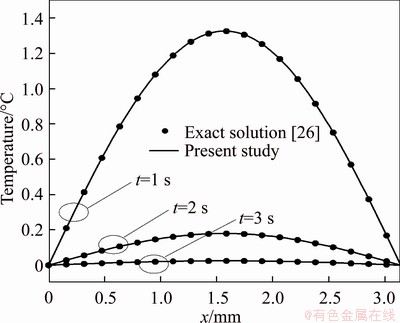
Figure 3 Temperature distribution along horizontal symmetry line
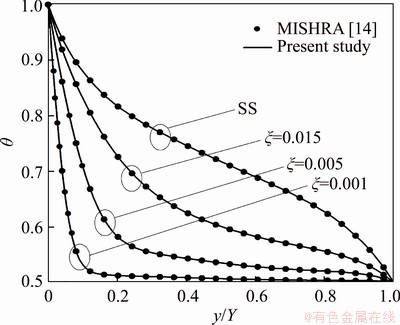
Figure 4 Temperature distribution across vertical symmetry line for different values of non-dimensional time and comparison to theoretical finding in Ref. [14] (SS means steady state and ξ is non-dimensional time)
This figure shows a good consistency between the present numerical results with those reported in Ref. [14].
5 Results and discussion
In this paper, a conjugate problem of combined radiation and conduction heat transfer in a furnace with isothermal heated walls is numerically solved. This problem can simulate the annealing process in a rectangular furnace in which a solid block is inserted in its center. For this purpose, the numerical solutions of energy equations inside the gas medium and solid element in their transient forms are carried out. The main focus is made on the solid body heating process and the effects of different important factors on this subject are examined.
First, to demonstrate the temperature pattern inside the computational domain including both radiating medium and solid element, the isotherm plots are drawn at t*=1 in Figure 5. In the computation of Figure 5, four different values of the optical thickness are used to study the effect on this parameter on the thermal characteristics of system. This figure shows how the solid element is heated inside the furnace during the annealing process, in a way that the temperature decrease from the surrounding heated walls toward the center of solid block can be clearly seen. If one compares the temperature fields at four different values of the optical thickness with each other, it can be found that the temperature distribution is much affected by this radiative parameter. Figure 5 shows that transferred thermal energy is a decreasing function of optical thickness τ. To elaborate, the penetration of radiative heat flux form boundaries to the product is inversely proportioned to the optical thickness. This is due to the fact that for optically thin media, the ability of absorbing radiant intensity by the participating medium decrease and more radiant energy transfers from the heated wall towards the solid element and finally thermal equilibrium happens in a short time period during the annealing process.
For more study regarding the effect of optical thickness in Figure 6, the total thermal energy entering the solid element including both radiation and conduction is drawn as a function of time for different values of the gas optical thickness. As expected, the decreasing trend of energy transfer into the body with time is observed, in a way that the rate of heat transfer approaches to zero at steady condition while all parts of the system reach to the cavity heated wall temperature and thermal equilibrium governs to the system. It is displayed in Figure 6 that low value of optical thickness causes higher rate of heat transfer from the furnace heated walls into the solid body. This behavior hastens annealing process in which the solid body warms up to its maximum temperature. For example, Figure 6 shows the dimensional times for achieving to steady state condition in the cases of τ=1, 0.5 equal to t*=10, 20, respectively.
In Figure 7, the distributions of total heat flux along the furnace walls are plotted at different optical thickness. This figure demonstrates that the maximum outgoing heat flux takes place at the middle of the furnace walls and the minimum value at the corners. This figure also confirms that the rate of heat transfer from the heated walls toward the solid body is a decreasing function of optical thickness.
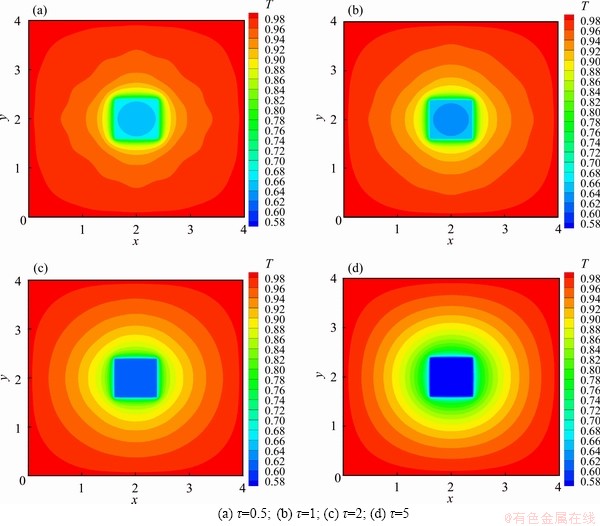
Figure 5 Temperature distribution inside furnace for different values of optical thickness RC=50, ω=0.5, t*=1:

Figure 6 Total rate of heat transfer to solid body versus non dimensional time, RC=50, ω=0.5
In order to study the effects of optical thickness on the temperature, at an arbitrary time,t*=1, the temperature distribution along the vertical centerline in the furnace is shown in Figure 8. A sharp temperature decrease is seen from the heated wall toward the solid body and a nearly constant temperature along the solid element takes place after which temperature increases as it moves toward the opposite heated wall. This figure also depicts that high temperature takes place inside the participating medium and also in solid element for small values of the optical thickness as it was observed in previous figures.
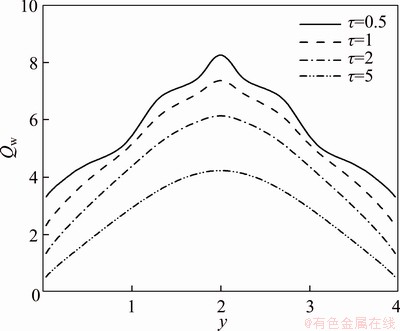
Figure 7 Effect of optical thickness on distribution of total heat flux along furnace wall (t*=1, RC=50, ω=0.5)
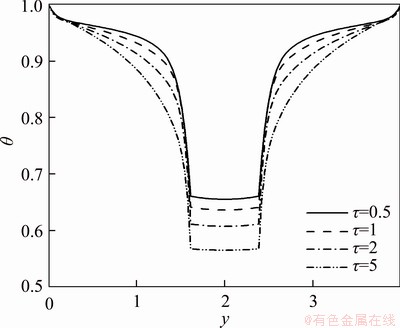
Figure 8 Temperature distribution along vertical symmetry line in the furnace (t*=1, RC=50, ω=0.5)
A quick glance at the effect of optical thickness on the behavior of the annealing process reveals that more time is needed to transfer a defined value of thermal energy to the solid body as the optical thickness increases. To elaborate,the data obtained from total transferred energy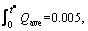 is shown in Table 1.
is shown in Table 1.
Table 1 Time required for transferring a specified value of heat (0.005) to solid body versus optical thickness

One of the most important thermal parameters, which has considerable influence on the combined radiation conduction heat transfer, is the radiation conduction parameter (RC). Its value shows the ratio of radiation heat transfer in a participating medium to the conduction counterpart. In Figure 9, the effect of RC on the total heat flux distribution along the furnace heated wall is studied. This figure shows that when the dominant mechanism of heat transfer is radiation, more heat transfer is released from the heated wall towards the solid body.
Figure 10 shows the isotherm plots inside the furnace at different values of RC. If one compares the temperature fields in the cases of RC=25 and 100 with each other, it can be noticed that the annealing process reaches rapidly thermal equilibrium in the cases having high values of radiation conduction parameter for the participating medium.
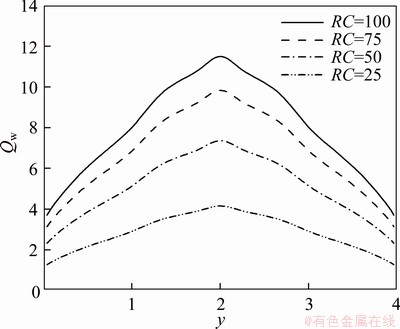
Figure 9 Effect of radiation conduction parameter on total heat flux distribution along furnace’s heated wall (t*=1, τ=1.0, ω=0.5)
This behavior can also be seen in Figure 11 in which the temperature distributions along the vertical symmetry line inside the furnace are drawn. It is observed that under the condition of high values of the RC parameter, high rate of heat transfer happens from the cavity heated wall into the product, and its temperature rapidly becomes more close to the hated wall temperature.
In Table 2, the amounts of time periods during the annealing process up to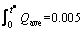 for the solid element are given. This table also confirms this fact that high radiation conduction parameter leads to fast annealing process and finally small transient time period.
for the solid element are given. This table also confirms this fact that high radiation conduction parameter leads to fast annealing process and finally small transient time period.
6 Conclusions
This work deals with the simulation of combined radiation and conduction heat transfer in a conjugate problem. The main purpose is to find the thermal characteristics of annealing furnace in which thermal energy is released from the boundary heated walls towards the annealed block by the radiating medium. The transient forms of governing equations including the conservations of energy for both gas and solid phases and also the RTE are solved, simultaneously, in computation of temperature field. It is revealed that high rate of energy transfer into the solid body is more intensive for small value of the gas optical thickness during the transient time period. The radiation-conduction parameter is also found as an important factor, in a way that the energy transfer into the annealed bock gets higher values by decreasing this parameter. Consequently, the non-radiating gases are suggested for using in such annealing furnaces in order to improve the performance of thermal system.
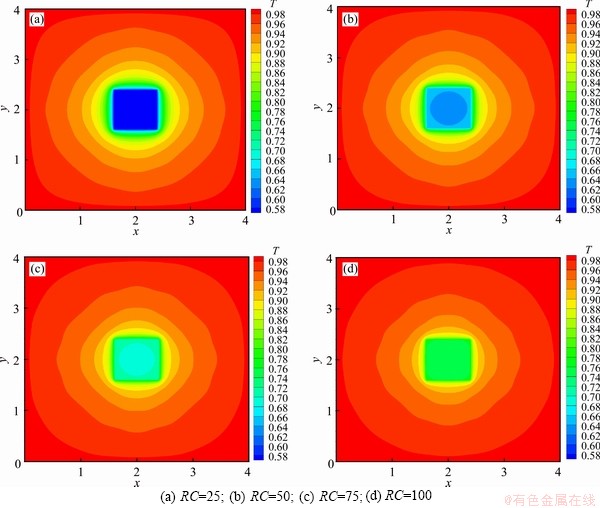
Figure 10 Temperature contours for different values of RC (t*=1, τ=1.0, ω=0.5):
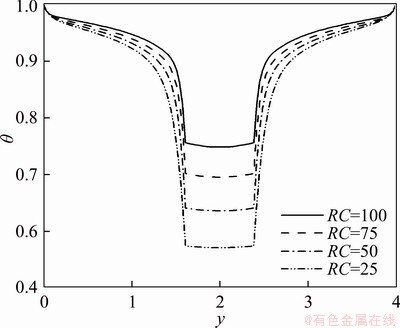
Figure 11 Temperature distribution along vertical symmetry line inside furnace (t*=1, τ=1.0, ω=0.5)
Table 2 Time required for transferring a specified value of heat (0.005) to solid body versus RC

Nomenclatures
I
Radiation intensity, W·m-2
k
Thermal conductivity, W·m-1·K-1
K
Non-dimensional thermal conductivity
L
Length, m
n
Unit vector normal to surface
q
Heat flux, W·m-2
Q
Dimensionless heat flux
RC
Radiation conduction parameter
r
Position vector, m
s
Direction
t
Time, s
T
Temperature, K
w
Quadrature weight
x, y
Coordinate system, m
X, Y
Dimensionless axes
Greek symbols
α
Thermal diffusivity
β
Extinction coefficient, m-1
φ
Scattering phase function
ξ, η
x- and y-direction cosines
Ω
Solid angle
σ
Stefan–Boltzmann constant
σa
Absorption coefficient, m-1
σs
Scattering coefficient, m-1
ε
Emissivity coefficient
ω
Albedo coefficient
τ
Optical thickness
θ
Dimensionless temperature
Subscripts
b
Black body
c
Conductive
f
Fluid
s
Solid
r
Radiative
ref
Reference
w
Wall
t
Total
Superscripts
*
Non-dimensional parameter
Contributors
M. Foruzan NIA carried out conceptualization, methodology, software, investigation, visualization, validation, writing-original draft, and S. A. Gandjalikhan NASSAB established conceptualization, and methodology, and finished writing-review, editing and supervision.
Conflict of interest
M. Foruzan NIA, and S. A. Gandjalikhan NASSAB declare that they have no conflict of interest.
References
[1] RAZZAQUE M M, HOWELL J R, KLEIN D E. Coupled radiative and conductive heat transfer in a two-dimensional rectangular enclosure with gray participating media using finite elements [J]. Journal of Heat Transfer, 1984, 106(3): 613-619.
[2] KIM T Y, BAEK S W. Analysis of combined conductive and radiative heat transfer in a two-dimensional rectangular enclosure using the discrete ordinates method [J]. International Journal of Heat and Mass Transfer, 1991, 34(9): 2265-2273.
[3] MAHAPATRA S K, NANDA P, SARKAR A. Analysis of coupled conduction and radiation heat transfer in presence of participating medium-using a hybrid method [J]. Heat and Mass Transfer, 2005, 41(10): 890-898.
[4] LARI K, GANDJALIKHAN N A S. Modeling of the conjugate radiation and conduction problem in a 3D complex multi-burner furnace [J]. Thermal Science, 2012, 16(4): 1187-1200.
[5] ZARE M, NASSAB S A G. Combined radiative-conductive heat transfer in two-dimensional complex geometries with variable thermal conductivity [J]. Iranian Journal of Science and Technology, Transactions of Mechanical Engineering, 2014, 38: 275-288.
[6] LICK W. Transient energy transfer by radiation and conduction [J]. International Journal of Heat and Mass Transfer, 1965, 8(1): 119-127.
[7] CHANG Y P, SMITH R S Jr. Steady and transient heat transfer by radiation and conduction in a medium bounded by two coaxial cylindrical surfaces [J]. International Journal of Heat and Mass Transfer, 1970, 13(1): 69-80.
[8] LII C C, OZISIK M N. Transient radiation and conduction in an absorbing, emitting, scattering slab with reflective boundaries [J]. International Journal of Heat and Mass Transfer, 1972, 15(5): 1175-1179.
[9] TSAI J R, OZISIK M N. Transient, combined conduction and radiation in an absorbing, emitting, and isotropically scattering solid sphere [J]. Journal of Quantitative Spectroscopy and Radiative Transfer, 1987, 38(4): 243-251.
[10] TSENG C J, CHU H S. Transient combined conduction and radiation in an absorbing, emitting and anisotropically- scattering medium with variable thermal conductivity [J]. International Journal of Heat and Mass Transfer, 1992, 35(7): 1844-1847.
[11] LIU Lin-hua, TAN He-ping. Transient radiation and conduction in a two-dimensional participating cylinder subjected to a pulse irradiation [J].International Journal of Thermal Sciences, 2001, 40(10): 877-889.
[12] LIU L H. Transient coupled radiation–conduction in infinite semitransparent cylinders [J]. Journal of Quantitative Spectroscopy and Radiative Transfer, 2002, 74(1): 97-114.
[13] ASLLANAJ F, JEANDEL G, ROCHE J R, LACROIX D. Transient combined radiation and conduction heat transfer in fibrous media with temperature and flux boundary conditions [J]. International Journal of Thermal Sciences, 2004, 43(10): 939-950.
[14] MISHRA S C, TALUKDAR P, TRIMIS D, DURST F. Two-dimensional transient conduction and radiation heat transfer with temperature dependent thermal conductivity [J]. International Communications in Heat and Mass Transfer, 2005, 32(3, 4): 305-314.
[15] MISHRA S C, ROY H K. Solving transient conduction and radiation heat transfer problems using the lattice Boltzmann method and the finite volume method [J]. Journal of Computational Physics, 2007, 223(1): 89-107.
[16] YI Hong-liang, ZHANG Hao-chun, TAN He-ping. Transient radiation and conduction heat transfer inside a plane-parallel participating gray medium with boundaries having different reflecting characteristics [J]. Journal of Quantitative Spectroscopy and Radiative Transfer, 2009, 110(18): 1978-1992.
[17] KAEMMERLEN A, ASLLANAJ F, SALLEE H, BAILLIS D, JEANDEL G. Transient modeling of combined conduction and radiation in wood fibre insulation and comparison with experimental data [J]. International Journal of Thermal Sciences, 2010, 49(11): 2169-2176.
[18] CHAABANE R, ASKRI F, NASRALLAH S B. Parametric study of simultaneous transient conduction and radiation in a two-dimensional participating medium [J]. Communications in Nonlinear Science and Numerical Simulation, 2011, 16(10): 4006-4020.
[19] CHAABANE R, ASKRI F, NASRALLAH S B. Analysis of two-dimensional transient conduction–radiation problems in an anisotropically scattering participating enclosure using the lattice Boltzmann method and the control volume finite element method [J]. Computer Physics Communications, 2011, 182(7): 1402-1413.
[20] CHAABANE R, ASKRI F, NASRALLAH S B. Application of the lattice Boltzmann method to transient conduction and radiation heat transfer in cylindrical media [J]. Journal of Quantitative Spectroscopy and Radiative Transfer, 2011, 112(12): 2013-2027.
[21] MODEST M F. Radiative heat transfer [M]. Academic Press, 2013.
[22] LARI K, BANESHI M, GANDJALIKHAN N, SEYYED A, KOMIYA A, MARUYAMA S. Combined heat transfer of radiation and natural convection in a square cavity containing participating gases [J]. International Journal of Heat and Mass Transfer, 2011, 54(23, 24): 5087-5099.
[23] NIA M F, NASSAB S A G. Conjugate heat transfer study of combined radiation and forced convection turbulent separated flow [J]. International Journal of Nonlinear Sciences and Numerical Simulation, 2017, 18(1): 29-39.
[24] FERZIGER J H. Numerical methods for engineering application [M]. New York: Wiley, 1981.
[25] NIA M F, NASSAB S A G, ANSARI A B. Transient combined natural convection and radiation in a double space cavity with conducting walls [J]. International Journal of Thermal Sciences, 2018, 128: 94-104.
[26] DAI B D, ZHENG B, LIANG Q, WANG L. Numerical solution of transient heat conduction problems using improved meshless local Petrov– Galerkin method [J]. Applied Mathematics and Computation, 2013, 219(19): 10044-10052.
[27] LI Qing-hua, CHEN Shen-shen, KOU Guang-xiao. Transient heat conduction analysis using the MLPG method and modified precise time step integration method [J]. Journal of Computational Physics, 2011, 230(7): 2736-2750.
(Edited by FANG Jing-hua)
中文导读
共轭联合辐射传导传热中退火过程的瞬态数值模拟
摘要:退火时间是许多炉性能的重要影响因素。本文研究了立方炉中放置固体元素的退火过程的瞬态模拟。由于工作气体具有一定的辐射特性,数值求解了一组随时间推移的控制方程,包括灰色辐射介质的能量平衡与辐射传递方程(RTE)和固体积内的传导方程。 数值结果与文献中的分析和理论分析吻合,结果表明,在起始阶段,即使在较小的光学厚度下,高辐射能量也会转移到固体中, 这加快了低辐射传导参数时的传热速率,导致了一个快速的退火过程,在这个过程中,固体加热到最高温度。此外,还发现传热速率是辐射传导参数的增函数。
关键词:共轭;辐射;传导;瞬态退火过程
Received date: 2020-01-25; Accepted date: 2020-06-12
Corresponding author: S. A. Gandjalikhan NASSAB, PhD, Professor; Tel: +98-9131407549; E-mail: ganj110@uk.ac.ir; ORCID: https:// orcid.org/0000-0003-0783-3155

