高钛6061铝合金的热变形行为
来源期刊:中国有色金属学报(英文版)2016年第2期
论文作者:陈微 官英平 王振华
文章页码:369 - 377
关键词:6061铝合金;热变形;动态回复;热加工图;位错
Key words:6061 Al alloy; hot deformation; dynamic recovery; hot processing map; dislocation
摘 要:利用应力应变曲线、热加工图,结合电子透射电子显微镜和背散射衍射技术研究在变形温度为350~510 °C、应变速率为0.001~10 s-1时高钛6061铝合金的热变形行为。结果表明,该合金的热压缩变形流变峰值应力随变形温度的升高和应变速率的降低而降低;在实验参数范围内平均热变形激活能为185 kJ/mol;建立了流变应力模型;该合金热变形时主要的软化机制为动态回复;根据材料动态模型获得了高钛6061铝合金的热加工图,最佳的热加工窗口温度为400~440 °C,应变速率为0.001~0.1 s-1。
Abstract: The hot deformation behavior of a high Ti 6061 aluminum alloy in the temperature range from 350 to 510 °C and strain rate range from 0.001 to 10 s-1 was investigated using stress-strain curve analysis, processing map, transmission electron microscopy and electron backscatter diffraction analysis. The results show that the peak stress decreases with increasing deformation temperatures and decreasing strain rate. The average deformation activation energy is 185 kJ/mol in the parameter range investigated. The flow stress model was constructed. The main softening mechanism is dynamic recovery. The processing map was obtained using dynamic material model, and the suggested processing window is 400-440 °C and 0.001-0.1 s-1.
Trans. Nonferrous Met. Soc. China 26(2016) 369-377
Wei CHEN, Ying-ping GUAN, Zhen-hua WANG
Key Laboratory of Advanced Forging & Stamping Technology and Science, Ministry of Education, Yanshan University, Qinhuangdao 066004, China
Received 30 March 2015; accepted 31 September 2015
Abstract: The hot deformation behavior of a high Ti 6061 aluminum alloy in the temperature range from 350 to 510 °C and strain rate range from 0.001 to 10 s-1 was investigated using stress-strain curve analysis, processing map, transmission electron microscopy and electron backscatter diffraction analysis. The results show that the peak stress decreases with increasing deformation temperatures and decreasing strain rate. The average deformation activation energy is 185 kJ/mol in the parameter range investigated. The flow stress model was constructed. The main softening mechanism is dynamic recovery. The processing map was obtained using dynamic material model, and the suggested processing window is 400-440 °C and 0.001-0.1 s-1.
Key words: 6061 Al alloy; hot deformation; dynamic recovery; hot processing map; dislocation
1 Introduction
6061 aluminum alloy is a typical Al-Mg-Si alloy. By adding Ti to common 6061 alloy, i.e., high Ti 6061 alloy, the strength, toughness, fatigue properties and wear resistance will be dramatically improved. Furthermore, Ti addition can also restrain the formation of cracks during casting [1]. When Ti is added to aluminum alloy melts, TiAl3 particles form and serve as the nucleation sites of alloys. Grain refinement relies on efficient nucleation and restricts growth of TiAl3 particles [2]. Normally, the TiAl3 particles are dissolved within a minute or less [3] and then lose refinement effect, but this is not valid for a Ti concentration about 0.15% (mass fraction) [4]. This wrought aluminum alloy is widely used in aerospace, automotive and other industries.
There are many reports [5-7] about high temperature deformation behavior of aluminum alloys. EZATPOUR et al [8] suggested that dynamic recovery occurred in 6061 aluminum alloy at high temperature and low strain rate, but HUANG et al [9] reported that the deformed microstructures were mainly with a type of dynamic recovery at low temperature and low strain rate. GOURDET and MONTHEILLET [10,11] demonstrated that new grains were formed through the progressive increase of low angle boundary (LAB) during continuous dynamic recrystallization in alloys with high stacking fault energy. Moreover, according to the reports of McQUEEN and BLUM [12] and STRAUB and BLUM [13], it should be pointed out that an increase/decrease in the fraction of HAB/LAB cannot be considered as the evidence of continuous dynamic recrystallization which is the mechanism for the steady state deformation. There is no solid concerted conclusion about the evolution of boundary misorientations during high temperature deformation of aluminum alloys, and the stacking fault energy is not the decisive factor of softening mechanism. Hot deformation resistance is one of the basic properties of aluminum alloy. Establishing a flow stress model can provide material with theoretical and experimental basis about hot processing. Microstructure evolution has important influences on the properties of materials. Some reports [14,15] showed that dynamic recrystallization occurs only under very low Zener-Hollomon parameter conditions. By changing thermo-parameters, various Z values can be obtained and the aluminum alloy microstructure could be predicted and controlled during hot deformation.
Though many researches have been conducted on 6061 aluminum alloy, such as hot deformation mechanism of coarse grains in high Ti 6061 aluminum alloy suggested by GUAN et al [16], researches on hot deformation characteristics of high Ti aluminum alloy were few, which greatly limit the application of high Ti 6061 alloy. In this study, a high Ti 6061 aluminum alloy was hot compressed using Gleeble-3500 thermal/ mechanical simulator. The flow behavior, microstructure evolution and the dynamic softening mechanism were studied using transmission electron microscopy (TEM) and electron backscatter diffraction (EBSD) technique. The present work aims to provide theoretic guidance for establishing the hot working process of high Ti 6061 aluminum alloy.
2 Experimental
The tested high Ti 6061 aluminum alloy was continuously cast bar with a diameter of 200 mm. Its chemical composition was shown as follows (mass fraction): 1.1% Mg, 0.8% Si, 0.15% Ti, 0.35% Cu, 0.25% Cr, 0.1% Zn, 0.2% Fe, balance Al. Solution heat treatment was conducted at 560 °C for 120 min and then quenched into water immediately. Compression specimens (10 mm in diameter and 16 mm in height) were cut from the heat treated bar. Hot compression was performed on a Gleeble-3500 thermal/mechanical simulator. Specimen was heated at a rate of 10 °C/s to 350-510 °C. The strain rate range was 0.001-10 s-1 and the true strain was 0.8. After deformation, specimens were quenched in water to freeze the deformed microstructure.
Deformed specimens were sectioned parallel to the compression axis. EBSD specimens were mechanically polished and then electrically polished using a solution of 10% HClO4 acids in alcohol at -30 °C and a voltage of 30 V. The crystallographic information was analyzed using a HKL Channel 5 software. The deformation microstructures were also examined using a JEM-2010 TEM. The TEM specimens were ground mechanically to a thickness of ~30 μm and then twin-jet electropolished in a solution consisting of 20 mL HNO3 and 80 mL methanol at 20 V and -30 °C.
3 Results and discussion
3.1 Flow behavior
The stress-strain curves of high Ti 6061 aluminum alloy under different temperatures and strain rates are shown in Fig. l. It can be seen that the stress increased rapidly to a peak and then decreased with increasing strain at 0.001 s-1 and 0.01 s-1. However, plateaus appeared under higher strain rate conditions. For a given deformation condition, the flow stress of high Ti 6061 aluminum alloy was higher than that of common 6061 alloy without Ti [17]. Obviously, Ti addition increased the hot deformation resistance.
Both peak stress and steady-state stress decreased with increasing deformation temperatures or decreasing strain rates. When the true strain rate was fixed, the dynamic softening effect leading to the decrease of stress was more pronounced at higher deformation temperatures. At a given temperature, the higher the strain rate, the higher the peak stress. It means that high Ti 6061 aluminum alloy is a strain rate sensitive material and the strain rate effect is positive.
3.2 Constitutive equation and Z parameter
A thermally activated process presents during the thermal deformation of metal material, which is similar to high-temperature creep [18]. SELLAR and MCG TEGART [19] proposed a modified hyperbolic sine relation with Arrhenius activation energy (Q) and temperature (T) to describe steady-state flow stress as Eq. (1). The relationships could also be described by Eqs. (2) and (3) at low stress level and high stress level, respectively.
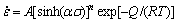 (1)
(1)
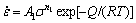 (2)
(2)
 (3)
(3)
where A, A1, A2, α, β, n and n1 are material constants and R is mole gas constant. α is an adjustable constant, α=β/n1. Equation (1) can be conveniently expressed in terms of a temperature compensated strain rate factor, the Zener-Hollomon parameter Z [20]:
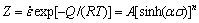 (4)
(4)
Taking the logarithm of both sides of Eqs. (2) and (3), respectively, gives
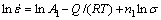 (5)
(5)
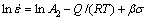 (6)
(6)
The slope of the plot of 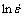 against ln σ and the slope of the plot of
against ln σ and the slope of the plot of 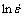 against σ can be used for obtaining the values of n1 and β, respectively, as shown in Fig. 2. Because the slopes of these lines are similar, the values of n1 and β can be obtained for different deformation temperatures by liner fitting method, and the mean values of n1 and β can be calculated as 9.6825 and 0.2435 MPa-1, respectively. Therefore, α=β/n1= 0.0251 MPa-1.
against σ can be used for obtaining the values of n1 and β, respectively, as shown in Fig. 2. Because the slopes of these lines are similar, the values of n1 and β can be obtained for different deformation temperatures by liner fitting method, and the mean values of n1 and β can be calculated as 9.6825 and 0.2435 MPa-1, respectively. Therefore, α=β/n1= 0.0251 MPa-1.
By taking natural logarithm of Eq. (1), the following expression can be derived:
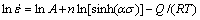 (7)
(7)
The slope of the plot of 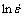 against ln[sinh(ασ)] can be used for obtaining the value of n, and the plot is shown in Fig. 3(a).
against ln[sinh(ασ)] can be used for obtaining the value of n, and the plot is shown in Fig. 3(a).
For the given strain rate conditions, differentiating Eq. (7) gives
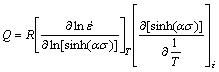 (8)
(8)
By substituting the values of temperature and flow stress under different strain rates into Eq. (8), the relationship between ln[sinh(ασ)] and temperature can be obtained as Fig. 3(b).
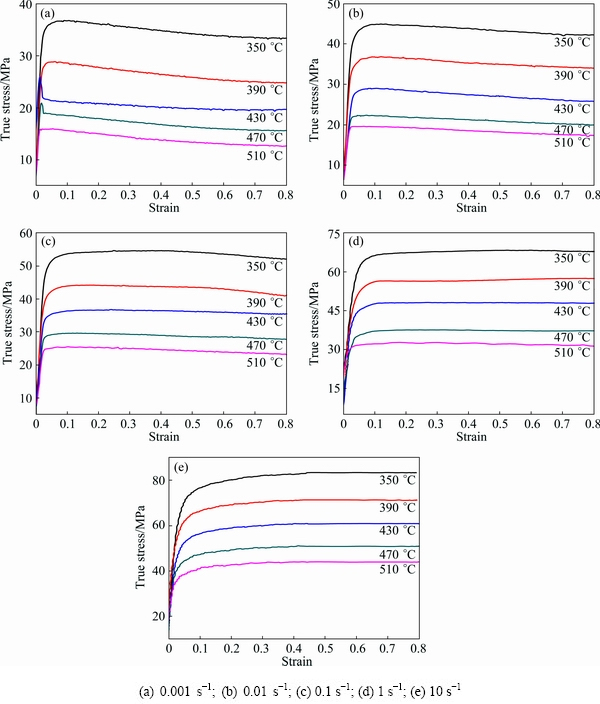
Fig. 1 True stress-strain curves for high Ti 6061 aluminum alloy deformed at different strain rates
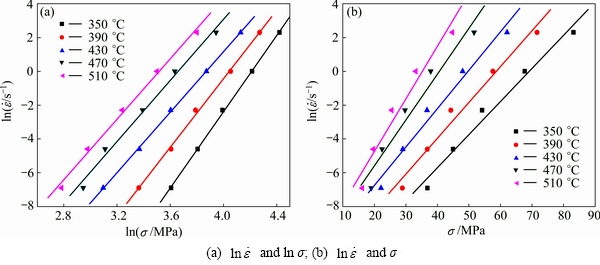
Fig. 2 Relationships between strain rate and flow stress of high Ti 6061 aluminum alloy
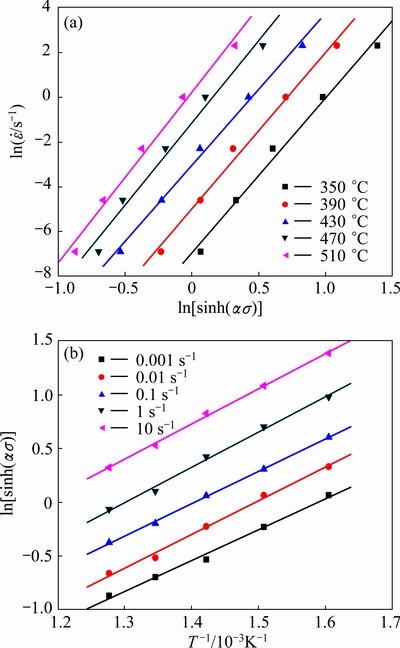
Fig. 3 Relationships between 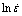 and ln[sinh(ασ)] (a) and relation between of ln[sinh(ασ)] and 1/T (b)
and ln[sinh(ασ)] (a) and relation between of ln[sinh(ασ)] and 1/T (b)
The hot deformation activation energy derived from Arrhenius plots of ln[sinh(ασ)] versus 1/T shown in Fig. 3 is 185 kJ/mol, which is lower than that reported by EZATPOUR et al [8] (274 kJ/mol). It should be noted that deformation activation energy is an average value and depends on the experimental parameters. In this study, much lower strain rates (0.001 and 0.01 s-1) and higher deformation temperature (510 °C) were used. This can explain why the value of Q is lower than that in Ref. [8]. SHERBY et al [21] proposed that the activation energy for self-diffusion of pure aluminum is 142 kJ/mol. A Q value of 185 kJ/mol suggests that the softening mechanism of high Ti 6061 aluminum alloy is dominated by dynamic recrystallization or dynamic recovery instead of diffusion. This value is close to that (181 kJ/mol or more) for a solution of Al-Mg-Si aluminum alloy proposed by GAN et al [22]. Otherwise, some deviation of deformation activation energy value is permissive because the property of linear regression method was used to gain deformation activation energy value, as reported by WHITTENBERGER et al [23].
By logarithmic transformation of Eq. (4), the following expression can be expressed
ln Z=ln A+nln[sinh(ασ)] (9)
The relationship between ln Z and ln[sinh(ασ)] is shown in Fig. 4. The values of A and n can be obtained as 2.65×1012 and 7.1369, respectively. The correlation coefficient is 0.99, revealing a good linear relation between ln Z and ln[sinh(ασ)].
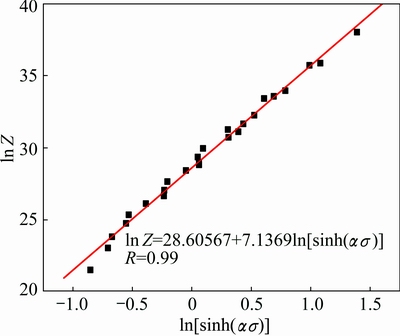
Fig. 4 Relationship between ln Z and ln[sinh(ασ)]
Finally, substituting the values of α, n, A and Q into Eq. (1), the flow stress for high Ti 6061 aluminum alloy during high temperature compression can be represented by a hyperbolic sine equation shown as follows:
 (10)
(10)
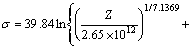
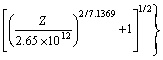 (11)
(11)
Z values are shown in Table 1. It is clear that Z value increases with strain rate increasing and deformation temperature decreasing.
Table 1 ln Z values of high Ti 6061 aluminum alloy

3.3 Processing map
The dynamic materials model (DMM) was used to construct processing map for high Ti 6061 aluminum alloy. The workpiece during hot deformation is a dissipater of power and the power might be instantaneously dissipated into two complementary parts:
G content and J co-content [24]:
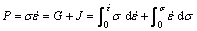 (12)
(12)
where G represents the power dissipated by plastic work, most of which is converted into viscoplastic heat, the little remaining power is stored as lattice defects. The co-content J is related to the power dissipated by metallurgical processes, such as recovery, recrystallization, phase transformation and wedge cracking. The power partitioning between G and J is controlled by the constitutive flow behavior of the material and is decided by the strain rate sensitivity (m):
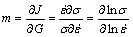 (13)
(13)
For an ideal linear dissipater, m=1 and
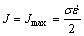 (14)
(14)
The efficiency of power dissipation of a nonlinear dissipater may be expressed as a dimensionless parameter:
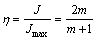 (15)
(15)
The parameter η is a dimensionless parameter, indicating how efficiently the material dissipates energy by microstructural changes. Therefore, η is an important parameter for the determination of optimum conditions for thermo-mechanical processing [25]. To evaluate the flow stability of high Ti 6061 alloy, the DMM stability criterium proposed by MURTY [26] was used.
2m<η or m<0 (16)
The processing map of high Ti 6061 aluminum alloy at a strain of 0.8 is presented in Fig. 5. There are four domains with different dissipative states. The contour number represents power dissipation efficiency, which characterizes the rate of microstructure evolution in hot working process. In this study, no flow instability region appears.
3.4 Microstructure evolution
Figure 6 shows the microstructure of the specimens corresponding to the four domains in Fig. 5. The morphology and content of dislocation are influenced by the Z value. At higher Z (390 °C, 1 s-1), subgrains with larger size formed. The dislocation wall can be seen in the subgrain, which divides the subgrain into many small regions, indicating the formation of new subgrains (Fig. 6(a)). With the decrease of Z value, i.e., temperature increases and/or strain rate decreases, the dislocation structure distributes uniformly, subgrains and cells are rarely found (Figs. 6(b) and (c)). At 510 °C and 0.01 s-1, i.e., Domain 3 in Fig. 5, although the atomic diffusion and dislocation movement ability are strengthened, dislocations in cell wall and other position have enough time to react attributed to the low strain rate. A large number of dislocations in the vicinity of the cell wall are formed to low-energy state by a polygonal pile effect (Fig. 6(d)). Moreover, in high stacking fault energy metallic materials, the maximum efficiency of power dissipation for dynamic recrystallization is about 50% while it is 35% in low stacking fault material [27]. In this study, the peak efficiency is only 23.6% and is not high enough to induce dynamic recrystallization. Form above results, it can be concluded that dynamic recovery represents the main softening mechanism of high Ti 6061 aluminum alloy.
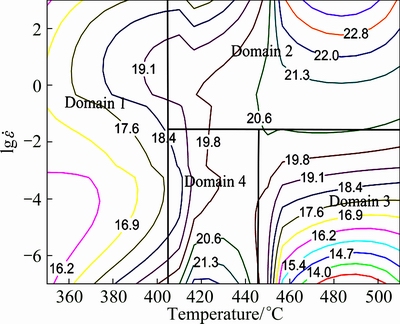
Fig. 5 Processing map of high Ti 6061 aluminum alloy developed at strain of 0.8
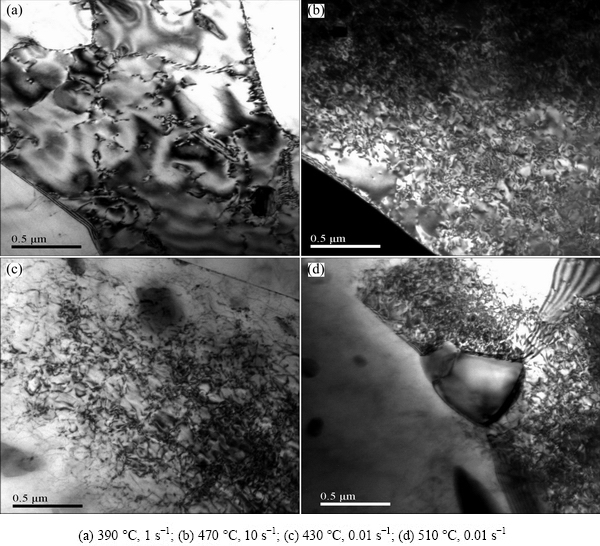
Fig. 6 TEM images of high Ti 6061 aluminum alloy deformed under different deformation conditions
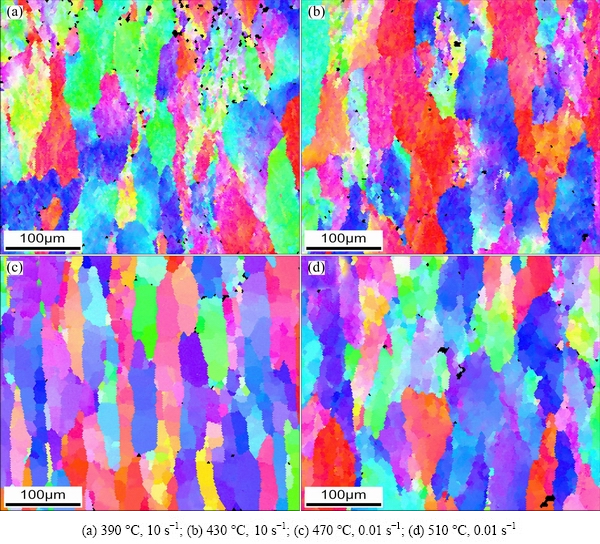
Fig. 7 Orientation maps of specimens deformed at different temperatures and compressive strain rates
It is difficult to get a lot of statistical information about the structure characteristics by TEM. Therefore, EBSD was also used to analyze the microstructure of high Ti 6061 aluminum alloy. Orientation maps are shown in Fig. 7. When deformed under high strain rate conditions (Figs. 7(a) and (b)), a large number of subgrain structures [28,29] form. The percentage of low angle grain boundaries, i.e., less than 15°, is more than 85%. With increasing the deformation temperature from 390 to 430 °C, there is almost no change in grain size and the evolution of subgrain size is also obvious. In Figs. 7(c) and (d), the percentage of low angle grain boundaries increases from 55% to 74%, as shown in Fig. 8. In the specimen deformed at 470 °C and 0.01 s-1 (Fig. 7(c)), it seems that local geometric dynamic recrystallization appears, but only few. The essence of geometric dynamic recrystallization also belongs to the scope of dynamic recovery [30-32]. Under high temperature and low strain rate conditions, the dislocations offset each other and restructure more thoroughly. With the increasing polygonization effect, recrystallization nucleation is restrained. Moreover, TiAl3 may dissolve at high temperatures, and its heterogeneous nucleation effect will be weaken [33], which could lead to grain coarsening (Fig. 7(d)). This condition corresponds to the Domain 3 in Fig. 5. In this domain, power dissipation efficiency reduces fast and this region is a potential dangerous region of deformation.
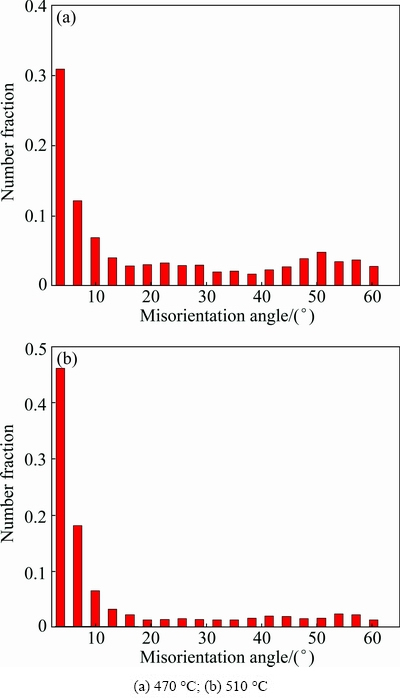
Fig. 8 Histograms of variation of misorientation angle at strain rate of 0.01 s-1 and different deformation temperatures
Figure 9 shows the variation of average grain size and efficiency with temperature. At a strain rate of 10 s-1, both grain size and efficiency of power dissipation increase continuously, especially above 440 °C (Fig. 9(a)). However, at a lower strain rate of 0.01 s-1 (Fig. 9(b)), a very high power dissipation and finer grains are obtained in the temperature range of 400-440 °C due to geometric dynamic recrystallization. To get better microstructure and processing performance, the suggested hot processing window is 400-440 °C at lower strain rates.

Fig. 9 Variation of average grain size and efficiency of power dissipation
4 Conclusions
1) The peak stress of high Ti 6061 Al alloy decreases with increasing deformation temperatures and decreasing strain rate.
2) The average deformation activation energy is 185 kJ/mol in the test parameter range.
3) The flow stress model for high Ti 6061 aluminum alloy during high temperature compression was constructed.
4) The main softening mechanism is dynamic recovery.
5) The processing maps were obtained using dynamic material model. The suggested processing window is 400-440 °C and 0.001-0.1 s-1.
References
[1] MCCARTENY D G. Grain refining of aluminium and its alloys using inoculants [J]. International Materials Reviews, 1989, 34(5): 247-260.
[2] WANG Jian-hua, YI Dan-qing, WANG Bin. Microstructure and properties of 2618-Ti heat resistant aluminum alloy [J]. Transactions of Nonferrous Metals Society of China, 2003, 13(3): 590-594.
[3] JOHNSSON M, BACKERUD L, SIGWORTH G K. Study of the mechanism of grain refinement of aluminum after additions of Ti- and B-containing master alloys [J]. Metallurgical Transactions A, 1993, 24(2): 481-491.
[4] MAJED J, TORBJOM C. Effect of titanium additions on the microstructure of DC-cast aluminium alloys [J]. Materials Science and Engineering A, 2005, 413-414: 277-282.
[5] WANG Ming-liang, JIN Pei-peng, WANG Jin-hui, HAN Li. Hot deformation behavior of as-quenched 7005 aluminum alloy [J]. Transactions of Nonferrous Metals Society of China, 2014, 24(9): 2796-2804.
[6] FENG Di, ZHANG Xin-ming, LIU Sheng-dan, WU Ze-zheng, TAN Qi. Rate controlling mechanisms in hot deformation of 7A55 aluminum alloy [J]. Transactions of Nonferrous Metals Society of China, 2014, 24(1): 28-35.
[7] POLETTI C, RODRIGUEZ-HORTALA M, HAUSER M, SOMMITSCH C. Microstructure development in hot deformed AA6082 [J]. Materials Science and Engineering A, 2011, 528: 2423-2430.
[8] EZATPOUR H R, HADDAD SABZEVAR M, SAJJADI S A, HUANG Y Z. Investigation of work softening mechanisms and texture in a hot deformed 6061 aluminum alloy at high temperature [J]. Materials Science and Engineering A, 2014, 606: 240-247.
[9] HUANG Chang-qing, DIAO Jin-peng, DENG Hua, LI Bing-ji, HU Xing-hua. Microstructure evolution of 6016 aluminum alloy during compression at elevated temperatures by hot rolling emulation [J]. Transactions of Nonferrous Metals Society of China, 2013, 23(6): 1576-1582.
[10] GOURDET S, MONTHEILLET F. A model of continuous dynamic recrystallization [J]. Acta Materialia, 2003, 51: 2685-2699.
[11] GOURDET S, MONTHEILLET F. An experimental study of the recrystallization mechanism during hot deformation of aluminium [J]. Materials Science and Engineering A, 2000, 283: 274-288.
[12] McQUEEN H J, BLUM W. Dynamic recovery: Sufficient mechanism in the hot deformation of Al (<99.99) [J]. Materials Science and Engineering A, 2009, 290(1-2): 95-107.
[13] STRAUB S, BLUM W. Does the “natural” third power law of steady state creep hold for pure aluminum [J]. Scripta Metallurgica et Materialia, 1990, 24(10): 1837-1842.
[14] LIU Xiao-yan, PAN Qing-lin, HE Yun-bin, LI Wen-bin, LIANG Wen-jie, YIN Zhi-min. Flow behavior and microstructural evolution of Al-Cu-Mg-Ag alloy during hot compression deformation [J]. Materials Science and Engineering A, 2009, 500(1-2): 150-154.
[15] HU Hui-e, ZHEN Liang, YANG Li, SHAO Wen-zhu, ZHANG Bao-you. Deformation behavior and microstructure evolution of 7050 aluminum alloy during high temperature deformation [J]. Materials Science and Engineering A, 2008, 488: 64-71.
[16] GUAN Ying-ping, WANG Zhen-hua, WU Bin, WANG Wei-xin, FU Wang-tang. Mechanism and inhibition of grain coarsening of Al-Mg-Si alloy in hot forming [J]. Journal of Harbin Institute of Technology, 2013, 20(3): 67-74.
[17] KWON Y N, LEE Y S, LEE J H. Deformation behavior of Al-Mg-Si alloy at the elevated temperature [J]. Journal of Materials Processing Technology, 2007, 188: 533-536.
[18] JONAS J J, SELLARS C M, MCG TEGART W J. Strength and structure under hot working conditions [J]. Metallurgical Review, 1969, 130(14): 1-24.
[19] SELLARS C M, MCG TEGART W J. On the mechanism of hot deformation [J]. Acta Metallurgica, 1966, 14(9): 1136-1138.
[20] ZENER C, HOLLOMON J H. Effect of strain rate upon the plastic flow of steel [J]. Journal of Applied Physics, 1944, 15(1): 22-32.
[21] SHERBY D, KLUNDT H, MILLER K. Flow stress, subgrain size, and subgrain stability at elevated temperature [J]. Metallurgical Transactions A, 1977, 8(6): 843-850.
[22] GAN Chun-lei, ZHENG Kai-hong, QI Wen-jun, WANG Meng-jun. Constitutive equations for high temperature flow stress prediction of 6063 Al alloy considering compensation of strain [J]. Transactions of Nonferrous Metals Society of China, 2014, 24(11): 3486-3491.
[23] WHITTENBERGER J D, NOEBE R D, DAROLIA R. Elevated temperature creep deformation in solid solution strengthened <001> NiAl-3.6Ti single crystals [J]. Materials Science and Engineering A, 2004, 367: 143-151.
[24] PRASAD Y V R K, GEGEL H L, DORIVELU S M,MALAS J C, MORGAN J T, LARK K A, BARKER D R. Modeling of dynamic material behavior in hot deformation: Forging of Ti-6242 [J]. Metallurgical and Materials Transactions A, 1984, 15: 1883-1892.
[25] PRASAD Y V R K, RAO K P. Processing maps and rate controlling mechanisms of hot deformation of electrolytic tough pitch copper in the temperature range 300-950 °C [J]. Materials Science and Engineering A, 2005, 391: 141-150.
[26] MURTY G S, KOCZAK M J. Superplastic behavior of an Al-4wt%Ti alloy processed by the powder metallurgy route [J]. Materials Science and Engineering A, 1988, 100: 37-43.
[27] PRASAD Y V R K, SESHACHAYULU T. Processing maps for hot working of titanium alloys [J]. Materials Science and Engineering A, 1998, 243: 82-88.
[28] SHEN Jian, GOTTSTEIN G. Modeling of microstructural evolution of aluminium alloy during thermomechanical processing [J]. Materials Science and Technology, 2001, 9(3): 329-335.
[29] PENG Da-shu, ZHANG Hui, YANG Li-bin, MENG Li-ping. Simulation on microstructural evolution during hot-rolling of aluminum alloys [J]. Materials Review, 2000, 12(14): 12-17.
[30] DOHERTY R D, HUGHES D A, HUMPHREYS F J, JONAS J J, JUUL JENSEN D, KASSNER M E, KING W E, McNELLEY T R, McQUEEN H J, ROLLETT A D. Current issues in recrystallization: A review [J]. Materials Science and Engineering A, 1997, 238: 219-274.
[31] McQUEEN H J, KNUSTAD O, RYUM N, SOLBERG J K. Microstructural evolution in Al deformed to strain of 60 at 400 degree C [J]. Scripta Materialia, 1985, 19: 73-78.
[32] BLUM W, ZHU Q, MERKEL R, McQUEEN H J. Geometric dynamic recrystallization in hot torsion of Al-5Mg-0.6Mn (AA5083) [J]. Materials Science and Engineering A, 1996, 205: 23-30.
[33] LIMMANEEVICHITR C, EIDHED W. Fading mechanism of grain refinement of aluminum-silicon alloy with Al-Ti-B grain refiners [J]. Materials Science and Engineering A, 2003, 349: 197-206.
陈 微,官英平,王振华
燕山大学 先进锻压成形技术与科学教育部重点实验室,秦皇岛 066004
摘 要:利用应力应变曲线、热加工图,结合电子透射电子显微镜和背散射衍射技术研究在变形温度为350~510 °C、应变速率为0.001~10 s-1时高钛6061铝合金的热变形行为。结果表明,该合金的热压缩变形流变峰值应力随变形温度的升高和应变速率的降低而降低;在实验参数范围内平均热变形激活能为185 kJ/mol;建立了流变应力模型;该合金热变形时主要的软化机制为动态回复;根据材料动态模型获得了高钛6061铝合金的热加工图,最佳的热加工窗口温度为400~440 °C,应变速率为0.001~0.1 s-1。
关键词:6061铝合金;热变形;动态回复;热加工图;位错
(Edited by Yun-bin HE)
Corresponding author: Ying-ping GUAN; Tel: +86-335-8074686; Fax: +86-335-8074686; E-mail: gyp@ysu.edu.cn
DOI: 10.1016/S1003-6326(16)64129-8

