
An accurate theoretical study on intrinsic defect energetics in rutile TiO2
MA Xin-guo(马新国), JIANG Jian-jun(江建军), LIANG Pei(梁培), WANG Juan(王娟)
Department of Electronic Science and Technology, Huazhong University of Science and Technology,
Wuhan 430074, China
Received 15 July 2007; accepted 10 September 2007
Abstract: An accurate theoretical study on the intrinsic point defects in rutile TiO2 was carried out by first-principles calculations using plane-wave pseudopotential method. The structural parameters of defect-free bulk rutile TiO2 were calculated, which are close to experimental data. And the effects of point defects on the geometry structures were analyzed. To get accurate value of formation energy and charge transfer levels, several technical details must be considered, such as the position of EVBM originating from supercell size and electrostatic interactions between the charged defects, and band-gap error etc. The formation energies of the point defects in various charge states were given as a function of Fermi level for the two limiting values of extreme O-rich conditions and extreme Ti-rich conditions. Under extreme Ti-rich conditions, Ti4+ interstitial and VO2+ have very low formation energy, and wound thus exist in significant quantities, namely, producing the intrinsic n-type TiO2. The stability of these point defects is traced back to the multivalence of titanium. Under extreme reducing condition, Frenkel defect comprised of Tii4+ and VTi4- would be formed in TiO2.
Key words: first-principles; TiO2; point defect; formation energy
1 Introduction
Titanium dioxide has received a lot of attention as a promising material in several applications, including white pigment, photocatalysis[1], dye-sensitized solar cells[2], and nanoscale electronic devices[3-4]. Rutile has the simplest and best known structure and point defects easily yield in a perfect rutile matrix after sputtering and annealing by varying temperatures and oxygen partial pressures, which directly determine the physical and chemical behavior of TiO2. For example, regular rutile TiO2 is an insulator in nature and can not dissociate H2O directly, but becomes semi-conductive with oxygen-deficiency and can adsorb H2O dissociatively on the defect site[5]. In another example, leakage currents in the TiO2 insulator are often explained on the basis of gap states originating from various point defects.
Recently, there have been many studies of the electronic and structural properties of point defect TiO2, both of experimental[6-11] and theoretical aspects [12-13], the question which type of defect is dominant in region of oxygen deficiencies is still subject to debate. YAGI et al[8] and LEE et al[9] reported that defects are mostly Ti interstitial ions and ionization of Ti interstitial donors takes place at lower oxygen partial pressures (p(O2)). The thermoelectric power data reported by NOWOTNY et al[10] confirmed that oxygen vacancies are the predominant ionic defects that are compensated by electrons. BAUMARD and TANI[11] have mentioned that Ti vacancies may also be
present in TiO2. The more source of information can be taken by first-principles calculations using accurate theoretical method, such as KOUDRIACHOVA[12] simply presented the investigations of the geometry structure of point defects in TiO2-x, and CHO et al[13] examined atomic relaxations around the point defects and related features in the electronic structure. They seem to have focused on neutral charge states. However, their studies did not consider the chemical potential and Fermi level as controlling variables for defect formation energies. Thus, we argue that a theoretical investigation covering simultaneously charged defects of rutile TiO2 is still in need, with particular emphasis placed on the technical aspects of accurate formation energy calculations.
2 Calculation models and methods
First-principles calculations were performed in the framework of density functional theory (DFT) within the generalized gradient approximation (GGA) using the Perdew-Burke-Ernzerhof (PBE) exchange correlation potential[14], and utilizing the plane-wave total energy pseudopotential method as implemented in castep code[15]. The ion-electron interaction is modeled by ultrasoft pseudopotentials in the Vanderbilt form. The valence atomic configurations are 3s23p63d24s2 for Ti, 2s22p4 for O atom. We use a 5×7×2 k-point set and cut-off 380 eV for the calculations of the stoichiometric and intrinsic point defects structure in rutile TiO2. The convergence criteria for the structure optimization and energy calculation were set to (a) a SCF tolerance of 2.0×10-6 eV/atom, (b) an energy tolerance of 2.0×10-5 eV/atom, (c) a maximum force tolerance of 0.5 eV/nm, and (d) a maximum displacement tolerance of 2.0×10-4 nm. Using above-mentioned methods can yield satisfactory results in our recent studies[16-17].
To calculate the formation energies of point defects, a supercell consisting of a 2×2×2 periodic repetition of the primitive unit cell was used. In our calculations, the defects considered included oxygen vacancies and interstitials, and titanium vacancies and interstitials. To introduce an isolated vacancy, an interior Ti or O atom is removed from the supercell. In the case of an isolated interstitial, an atoms of Ti or O atom is put into the supercell, where the normally vacant octahedral site is considered as a possible interstitial site. Due to the large cation-anion size mismatch and strong ionicity of TiO2, antisites are unlikely to form in TiO2. For calculations of the defective supercells, the nearest and next-nearest atoms located within a radius of 0.38 nm from defects were allowed to relax.
3 Results and discussion
We begin with the experimental lattice parameters to get the crystal cell, and optimizing the bulk structure of rutile TiO2. The calculated results using Monkhorst k-points 5×7×2 and Ecut of 380 eV show that the lattice constants are slightly overestimated using a typical feature of GGA methods. But the overestimated is always less than 2%. The calculated crystal parameters shown in Table 1 are in good agreement with the experimental values[18]. The PBE functional is usually the most reliable, since it performs well for both small and extended systems[19].
We analyzed the effects of point defects on the geometry structures. For an isolated O vacancy, after relaxation, the total energy is lowered by 0.6 eV and each of the nearest-neighbor Ti atoms moves 0.01-0.015 nm away from the vacancy toward its remaining O neighbors. This is due to the effectively positive charges of the O vacancy site which interact repulsively with nearby cations. As a Ti atom is missing here, the surrounding O atoms relax outward, leading to increase the Coulomb binding by reducing the apical Ti-O distances (dap) from the 0.199 8 nm to 0.184 0 nm, and the equatorial Ti-O distances (deq) from the 0.195 5 nm to 0.179 9 nm. The results also show that the interstitial Ti has little effect on the host lattice, and the interstitial O would spontaneously bind to the lattice oxygen, resulting in an O2 dimer substituting on one O site. Our results are qualitatively similar to those of Ref.[13], although some differences were evident in the interpretation of those results. To the extent that quantitative differences occur they don’t consider the charge states of point defects, referring to the ion radius and electrostatic interactions between charged defects.
In thermodynamic equilibrium the concentration c of point defects is given by the expression c = NsiteNconfig? exp[-Ef/(kT)][20]. Here, Ef is the defect formation energy, and lower Ef means to higher defect concentration c, which directly determines the physical and chemical behavior of TiO2. In other words, defects with lower formation energies are more likely to form. The formation energy of a defect in charge state is define as:
Ef(Dq) = Etot(Dq)-Etot(perfect)+nTi μTi+nQ μQ+q(Ef +EqVBM) (1)
where Etot(Dq) is a total energy of a supercell with the
Table 1 Calculated structural parameters for crystalline TiO2 compared to experimental values of Ref.[18], and theoretical values of Ref.[19]

defect, Etot(perfect) is the total energy for the equivalent supercell containing only bulk TiO2. Here nAl and nO are the numbers of Ti and O atoms removed from or added to the perfect supercell to introduce a vacancy or interstitial. For example, nAl=0 and nO=-1 for an O vacancy, and nAl=0 and nO=+1 for an O interstitial. For each defect species, its charge q varying from neutral to fully ionized states was considered.
In order to succeed, a certain number of difficult problems had to be solved. Firstly,  values of defective supercells were obtained from the one of the perfect supercell and a difference ΔV in average potentials (Vav) between the perfect supercell and a bulk like environment in defective supercells as follow:
values of defective supercells were obtained from the one of the perfect supercell and a difference ΔV in average potentials (Vav) between the perfect supercell and a bulk like environment in defective supercells as follow:
 (2)
(2)
The first term of the right-hand side of Eqn.(2) can be contained by
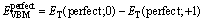
where ET(perfect; 0) is the total energy of the neutral perfect supercell. ET(perfect; +1) is that of the +1 charged perfect supercell, which corresponds to the situation that one electron is removed from the VBM of the neutral perfect supercell.
Secondly, the calculated band gap (Eg) is in much smaller than the experimental one of 3.0 eV. The difference between theory and experiment (ΔEg=0.85 eV) could affect formation energies of intrinsic defects in TiO2. When a defect induces extra occupied levels below the CBM, which are composed of cation orbitals similar to the conduction band, its formation energy will be underestimated since the energy position of the CBM itself is underestimated. This situation corresponds to oxygen vacancy and Ti interstitial in TiO2. In such case, it is assumed as crude correction that the conduction band is rigidly shifted upward to match the experimental Eg. Then formation energies were corrected by adding a value of mΔEg, where m is the number of electrons at defect-induced levels in Eg.
Thirdly, the chemical potentials depend on the experimental growth conditions, which can be Ti-rich or O-rich (or anything in between). Under extreme Ti-rich conditions, μTi = μTi[bulk]. Similarly, extreme O-rich conditions place an upper limit on μO given by μO= 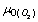 . Using the following expression:
. Using the following expression:
μTi+2μO = Etot(TiO2) (3)
The upper limit on μTi then results in a lower limit on μO:
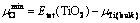 (4)
(4)
Similarly, the upper limit on μO results in a lower limit on μTi:
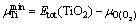 (5)
(5)
Also, the formation enthalpy ΔHf[TiO2] can be obtained by
 =
= (6)
(6)
From Eqns.(3), (4) and (6), the range of μO is represented as
 +
+ ≤
≤ ≤
≤ (7)
(7)
Our calculated value for ΔHf[TiO2] obtained from Eqn.(6) is -9.63 eV per TiO2 or 3.2 eV per atom, which is comparable to the experimental value of (9.6±0.8) eV per TiO2[21]. The formation energy of point defects in various charge states at the VBM (EF=0 eV) under
extreme O-rich conditions (μO= ) and extreme
) and extreme
T-rich conditions (μTi = μTi(bulk)) are given in Table 2 under several corrections.
Table 2 Point defect formation energies in TiO2 with Fermi level at VBM. Both O-rich (μO=0) and Ti-rich (μO=-4.814 eV) conditions are shown
conditions are shown

Fig.1 shows the formation energies of various point defects in TiO2 as a function of the Fermi energy under the O-rich and Ti-rich growth conditions, respectively. Filled circles denote the position of thermodynamic transition levels ε(q1/q2), which is defined as the Fermi-level position where charge states q1 and q2 have equal energy. As the name implies, the level would be observed in experiments where the final charge state can fully relax to its equilibrium configuration after the transition.

Fig.1 Formation energies of intrinsic point defects as function of Fermi level under Ti-rich (μO=-4.814 eV) (a) and O-rich (μO=0 eV) growth conditions(b), respectively. For each defect species, only lowest-energy charge states with respect to EF are shown. Zero energy of EF corresponds to valence-band minimum, while 3 eV indicates conduction-band minimum using experimental Eg value
From Fig.1(a), we see that when EF is near the VBM (i.e., p-type material), EF(Tii4+) and Ff(VO2+) are both negative, so Tii and VO will form spontaneously. On the other hand, when EF is the midgap (intrinsic material and /or at high temperature), EF(Tii,+4) still negative while EF(VO2+) has positive small value about 0.5 eV, so Tii will be more abundant under these conditions. Tii is not only easy to form, but also produces a donor levels:
Its lowest defect transition level is  (+4/+3) = ECBM- 0.41 being inside the band gap, so it is ionized only when EF is below this position. Hence, Tii have two charge states +4 and +3. Similarly, VO has a donor level below the CBM at
(+4/+3) = ECBM- 0.41 being inside the band gap, so it is ionized only when EF is below this position. Hence, Tii have two charge states +4 and +3. Similarly, VO has a donor level below the CBM at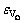 (+2/0)=ECBM-0.36 eV.
(+2/0)=ECBM-0.36 eV.
The donor level of Tii in TiO2 is deeper than Sni in SnO2 because the outer electrons of the Ti atom are more strongly bound than that of the Sn atom, which indicate TiO2 has more strongly covalence than SnO2. They will play significant role in increasing absorbance in the visible region and electron traps, resulting in the improvement in photocatalytic activity under visible- light irradiation. As EF moves towards the CBM the formation energy of acceptlike (negatively charged) intrinsic defects such as VTi and Oi is decreased. Were these “electron killers” to form spontaneously, they would compensate the electron-producing intrinsic donor defects, i.e., Tii and VO. However, this does not happen since VTi and Oi hardly form as they have high formation energies, the high formation energies of VTi and Oi result from the large electrostatic repulsion between the negatively charged oxygen atoms at the vertices of Ti-centered octahedron.
To understand why Ti interstitial has a low formation energy we investigate the structural changes in the lattice of TiO2 upon introducing this defect. In the rutile phase of TiO2, each Ti4+ center is surrounded with six O2- ions in octahedral coordination, and each O2- ion is surrounded by three Ti4+ ions in trigonal-planar coordination. Like the substitutional Ti site in TiO2, the interstitial site is also coordinated by six oxygens. This ion Tii of +4 and/or +3 charged state is small enough to fit in the space around the interstitial site so as to make it almost octahedrally coordinated, similar to the situation for host Ti atoms. Another reason for the ease of forming Tii in TiO2 is that Titanium has two stable oxidation states, Ti(IV) in TiO2 and Ti(II) in TiO. Introduction of Ti ion makes the oxygen coordination in TiO2 become similar to that in TiO. It is well known that in this structure, each titanium atom is surrounded by an oxygen octahedron, because of the highly symmetric distribution of titanium atoms, no distortion takes place, leading to an fcc lattice. Because both oxides are stable, the formation of Tii is not energetically costly, so Tii can form easily.
From the Fig.1(b), we see that the thermodynamic transition levels ε(q1/q2) are similar to that of Fig.1(a). On the other hand, when EF is near the VBM, the formation energies of VTi and Tii is low. However, VTi and Tii have equal energy at EF=0.21 eV, indicating that the Frenkel defect comprised of Tii4+ and VTi would form in TiO2. In fact, VTi hardly form as Tii would compensate the electron-producing intrinsic accept defects, i.e., VTi and Oi. On O-rich conditions, the concentration of VTi and Oi would be very low, namely, producing the intrinsic p-type TiO2 is quite difficult.
4 Conclusions
1) First-principles plane-wave pseudopotential calculations were performed to study the formation energies of intrinsic point defects in TiO2. Various charge states for individual point defects were considered, and their formation energies were calculated.
2) Under the Ti-rich conditions, the fully ionized states of Tii and VO has low formation energy, so Tii and VO will be more abundant and will coexist and dominate in the defect structure of TiO2 under these conditions. Tii has a donor level below the CBM at 0.41 eV, so Tii have two charge states +4 and +3.
3) On O-rich conditions, the Frenkel defect comprised of Tii4+ and VTi would form in TiO2. However, the concentration of VTi and Oi would be very low.
References
[1] LINSEBIGLER A L, LU G, YATES J T. Photocatalysis on TiO2 surfaces: Principles, mechanisms, and selected results[J]. Chem Rev, 1995, 95: 735-758.
[2] O’REGAN B, GR?TZEL M. A low-cost, high-efficiency solar cell based on dye-sensitized colloidal TiO2 films[J]. Nature, 1991, 353: 737-740.
[3] MAITI C K, SAMANTA S K, DALAPATI G K, NANDI S K, CHATTERJEE S. Electrical characterization of TiO2 gate oxides on strained-Si[J]. Microelectron Eng, 2004, 72: 253-256.
[4] KATAYAMA M, IKESAKA S, KUWANO J, YAMAMMOTO Y, KOINUMA H, MATSUMOTO Y. Field-effect transistor based on atomically flat rutile TiO2[J]. Appl Phys Lett, 2006, 89: 242103-242105.
[5] DIEBOLD U. The surface science of titanium dioxide[J]. Surf Sci Rep, 2003, 48: 53-229.
[6] LISACHENKO A A, MIKHA?LOV R V. Point defects as the centers of titanium dioxide sensitization in the visible spectral range[J]. Tech Phys Lett, 2005, 31(1): 21-24.
[7] LEE D K, YOO H I. Unusual oxygen re-equilibration kinetics of TiO2-δ [J]. Solid State Ionics, 2006, 177: 1-9.
[8] YAGI E, HASIGUTI R, AONO M. Electronic conduction above 4 K of slightly reduced oxygen-deficient rutile TiO2-x[J]. Phys Rev B, 1996, 54: 7945-7956.
[9] LEE, D K, JEON J I, KIM M H, CHOI W, YOO H I. Oxygen nonstoichiometry (δ) of TiO2-δ-revisited[J]. Journal of Solid State Chem, 2005, 178: 185-193.
[10] NOWOTNY M K, BAK T, NOWOTNY J. Electrical properties and defect chemistry of TiO2 single crystal. II. thermoelectric power[J]. J Phys Chem B, 2006, 110: 16283-16291.
[11] BAUMARD J F, TANI E. Electrical conductivity and charge compensation in Nb doped TiO2 rutile[J]. J Chem Phys, 1977, 67: 857-860. [12] KOUDRIACHOVA M. Geometry and ordering of defects in non-stoichiometric rutile[J]. Phys Stat Sol(C), 2007, 4(3): 1205-1208.
[13] CHO E, HAN S, AHN H S, LEE K R, KIM S K, HWANG C S. First-principles study of point defects in rutile TiO2-x[J]. Phys Rev B, 2006, 73: 193202-193205.
[14] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple[J]. Phys Rev Lett, 1996, 77: 3865-3868.
[15] SEGALL M D,LINDAN PHILIP J D, PROBERT M J, PICKARD C J, HASNIP P J, CLARK S J, PAYNE M C. First-principles simulation: ideas, illustrations and the CASTEP code[J]. J Phys: Condens Matter, 2002, 14: 2717-2744.
[16] MA X G, TANG C Q, YANG X H. Effect of relaxation on the energetics and structure of anatase TiO2 (101) surface[J]. Surface Review and Letters, 2006, 13(6): 825-831.
[17] MA X G, TANG C Q, YANG X H. Electronic structures of S-doped anatase and rutile TiO2[J]. Journal of Theoretical and Computational Chemistry, 2007, 6(1): 23-32.
[18] BURDETT J K, HUGHBANKS T, MILLER G J, RICHARDSON J W, SMITH J V. Structural-electronic relationships in inorganic solids: powder neutron diffraction studies of the rutile and anatase polymorphs of titanium dioxide at 15 and 295 K[J]. J Am Chem Soc, 1987, 109: 3639-3646.
[19] LAZZERI M, VITTADINI A, SELLONI A. Structure and energetics of stoichiometric TiO2 anatase surfaces[J]. Phys Rev B, 2001, 63: 155409-155418.
[20] VAN D E WALLE C G, NEUGEBAUER J. First-principles calculations for defects and impurities: Applications to Ⅲ-nitrides[J]. J Appl Phys, 2004, 95: 3851-3879.
[21] KNAUTH P, TULLER H L. Electrical and defect thermodynamic properties of nanocrystalline titanium dioxide[J]. J Appl Phys, 1999, 85: 897-902.
Foundation item: Project(NCET-04-0702) supported by the New Century Excellent Talents in University; Project(50771047) supported by the National Natural Science Foundation of China
Corresponding author: JIANG Jian-jun; E-mail: jiangjj@mail.hust.edu.cn
(Edited by YANG Hua)