Trans. Nonferrous Met. Soc. China 24(2014) 1531-1537

Ultimate pit optimization with ecological cost for open pit metal mines

Xiao-chuan XU¹, Xiao-wei GU¹, Qing WANG¹, Jian-ping LIU^1,2, Jun WANG^1,3

1. School of Resource and Civil Engineering, Northeastern University, Shenyang 110819, China;

2. Dongmei Foundation Company of Shenyang, Shenyang 110016, China;

3. School of Economics and Management, Henan Polytechnic University, Jiaozuo 454000, China

Received 30 October 2013; accepted 27 February 2014

Abstract: The ecological costs of open pit metal mining are quantified, which include lost value of direct eco-services, lost value of indirect eco-services, prevention and restoration costs, and cost of carbon emission from energy consumption. These ecological costs are incorporated in an iterative ultimate pit optimization algorithm. A case study is presented to demonstrate the influence of ecological costs on pit design outcome. The results show that it is possible to internalize ecological costs in mine designs. The pit optimization outcome shifts considerably to the conservative side and the profitability decreases substantially when ecological costs are accounted for.

Key words: ultimate pit; optimization; ecological cost; open pit metal mine

1 Introduction

The first step in planning an open pit operation is generally designing the ultimate pit. Many algorithms have been developed for this purpose, such as the moving cone algorithm, the graph theory algorithm, the improved graph theory algorithm, and the network-flow algorithm [1-4]. In the past decade, research on the pit design problem has largely focused on two subjects. One is the incorporation of various practical conditions and different approaches have been proposed. For example, FRIMPONG et al [5] incorporated structural, hydrological, and geotechnical conditions in an artificial intelligence algorithm for ultimate pit optimization. JALALI et al [6] considered uncertainties of pit geometries and used Markev chains to solve the problem. LATORRE and GOLOSINSKI [7] considered the time value of money in designing the pit. Another subject of extensive research is the consideration of uncertainties in market prices and resources [8-11].

With growing awareness of sustainable development, the mineral industry is under increasing pressure to operate mines not only based on economic and engineering principles, but also in accordance with sustainability principles. Regulations have been enacted in various countries requiring environmental impact assessment and reclamation planning for mining projects. Best practice guidelines have been adopted in some countries to promote proactive attention to sustainability concerns. Academic research efforts have also been directed at addressing environmental issues associated with mining. Life cycle assessment (LCA) is one of the widely used approaches for assessing environmental impacts of mining, mineral processing, smelting, and fabrication [12-15]. In most LCA studies, the mining system is largely simplified and mining process details affecting environmental impacts are rarely taken into account. DURUCAN et al [16] presented an LCA model that presents the mining system in a more comprehensive and detailed way. There are also studies with broader scopes which encompass not only the environmental but also the social issues of mining [17,18].

It is obvious that ecological impacts are closely related to mine design for a specific mining project. In the case of an open pit operation, the impacts vary with the size of the ultimate pit, its average strip ratio, and the mine life. However, as briefly reviewed above, studies addressing the pit optimization problem generally do not include environmental issues in their optimization scheme, while studies addressing the environmental issues of mining are rarely concerned with concrete design alternatives. The objective of this work is to quantify the ecological costs of open pit metal mining and to incorporate them in a pit optimization algorithm to demonstrate the influence of ecological costs on pit optimization outcome.

2 Ecological cost of open pit metal mining

Ecosystems provide a wide range of services to human society. Damage to ecosystems either causes a total loss of all or some of the eco-services that they provide or causes degradation in their service-providing capabilities. Therefore, the ecological costs of mining may be viewed as the lost values of eco-services caused by mining activities and the costs required for restoring the damaged ecosystems. The ecological costs of a mining operation are defined here to include four major components: lost value of direct eco-services, lost value of indirect eco-services, prevention and restoration costs, and cost of carbon emission from energy consumption. The first three components are directly related to the type and area of land damaged by mining. Since metal mines are generally located in hilly regions, where the land type is usually forest, the ecological cost estimation in this section is for forest land.

2.1 Land area estimation

The land areas damaged by an open pit operation include pit excavation, waste dump, tailings pond, roads, and facility sites. The land areas damaged by roads and facility sites are not considered in this study because they are negligibly small compared with those damaged by the waste dump, pit, and tailings pond. For a given pit design, the land area of the pit is directly obtained by delineating its surface outline. The land areas occupied by the waste dump and tailings pond, A_w and A_t are estimated by

                          (1)

                      (2)

where Q_w and Q_o are the total quantities of material in the pit mined as waste and ore, respectively; f_s is the average swelling factor of waste rock in the dump; ρ_w and ρ_t are the densities of in-situ waste and tailings in pond, respectively; H_w and H_t are the planned height of the waste dump and depth of the tailings pond, respectively; f_w and f_t are the shape factors of the waste dump and tailings pond, which have a value from 1.0 (for cylinder and prism like shapes) to 3.0 (for cone like shapes); g_o and g_p are the average grades of mined ore and concentrate, respectively; r is the metal recovery of ore processing.

2.2 Lost value of direct ecological services

Providing bio-products is one of the most important ecosystem services from which human society directly benefits. Such services are herein termed direct eco-services. The direct eco-service provided by forest is producing timber and its value depends on the productivity, type, and price of timber. In many cases, the land price is a good reflection of the capitalization of the expected net gains from the products produced by the land in the future. The current price of forest land acquisition is used in the case study.

2.3 Lost value of indirect ecological services

Apart from providing bio-products, ecosystems provide a wide range of other services that have indirect value to human welfare. Such services are termed indirect eco-services. However, human knowledge of these eco-services and their functions in relation to the welfare of human society is far from complete. Quantifying the values of all indirect eco-services is a very difficult, if not impossible task. Based on the availability of relevant data, the indirect eco-services of forest considered in this study include soil erosion control, air pollutant absorption, oxygen release, rain runoff control, and soil nutrient formation.

2.3.1 Value of soil erosion control

The amount of soil retained due to the soil erosion control function of forest is calculated using the soil retaining capacity of the type of forest destroyed by mining. The soil retaining capacity is the difference between the amount of soil loss without any vegetation and that with forest cover. The economic value of the retained soil may be estimated as the lost net revenue if the soil was used to form an equivalent area of crop land. Thus, the value of soil erosion control, V_s, is calculated as

                              (3)

where s is the soil retaining capacity of forest; ρ_s is the soil density; h_m is the minimum soil thickness required for crop land; v is the annual net revenue of local crop land.

2.3.2 Value of air pollutant absorption

Forest can absorb certain air pollutants, such as CO₂, SO₂, and NO_X and suppress dust. NO_X is not considered in this study due to availability of relevant data. The amount of absorbed CO₂ is calculated based on the net primary productivity (NPP) of the type of forest destroyed by mining, in terms of mass of dry matter, and the photosynthesis equation giving the CO₂ absorption factor, which is the mass of CO₂ absorbed per unit mass of NPP. The economic value of CO₂ absorption is estimated using carbon capture and storage cost. The amounts of absorbed SO₂ and suppressed dust are calculated based on the capacity of forest of SO₂ absorption and dust suppression. The economic values of SO₂ absorption and dust suppression are estimated using the costs of SO₂ scrubbing and dust control, such as those in a coal-fired power plant. Thus, the value of air pollutant absorption, V_a, is

                     (4)

where q is the forest’s NPP; 1.62 is the CO₂ absorption factor; f_C is the mass conversion factor from C to CO₂; c_C is the carbon capture and storage cost; y_S and y_d are the forest’s capacities to absorb SO₂ and suppress dust, respectively; and c_S and c_d are the SO₂ scrubbing and dust control costs, respectively.

2.3.3 Value of oxygen release

The amount of released oxygen is calculated based on the forest’s NPP and the oxygen release factor given by the photosynthesis equation. The economic value of oxygen release is estimated as the industrial cost of producing the same amount of oxygen. Thus, the value of oxygen release, V_O, is

                                 (5)

where c_O is the cost of oxygen production; and 1.2 is the oxygen release factor.

2.3.4 Value of rain runoff control

The amount of rain water retained due to lower runoff is calculated based on the runoff reduction coefficient of the type of forest destroyed by mining, the annual rainfall, and the proportion of rainfall that causes runoff flow. The economic value of the retained water is estimated by the cost of storing the same water amount in a reservoir. Thus, the value of rain runoff control, V_r, is

                                (6)

where p is the average rainfall; k is the proportion of rainfall causing runoff flow; f_r is the runoff reduction coefficient of forest; and c_r is the cost of storing water in a reservoir.

2.3.5 Value of soil nutrient formation

The amount of soil nutrients, mainly nitrogen (N), phosphorus (P), and potassium (K), formed through the nutrient circulation mechanism of forest land is calculated based on the NPP and the N, P, K contents of NPP of the destroyed forest. The economic value is estimated using the price of fertilizer. Thus, the value of soil nutrient formation, V_n, is

                (7)

where k_N, k_P and k_K are the N, P and K contents in q, respectively; p_N, p_P and p_K are the prices of N, P and K fertilizers, respectively; and f_P is the mass conversion factor from P to P₂O₅.

2.3.6 Total lost value of indirect eco-services

The above indirect eco-services are provided by the forest on a yearly basis and are lost for each of the years beginning when the forest is destroyed and ending when vegetation is fully restored. Thus, the total cost due to loss of indirect eco-services, C_i, is

            (8)

where P_o is the planned annual ore production rate; N is the time length for reclamation and vegetation recovery.

2.4 Prevention and restoration costs

Prevention costs are those spent on environmental protection measures, such as waste water treatment and toxic waste handling. These costs are treated as ecological costs because they are used to protect the environment and thus the ecosystems from degradation. Restoration costs are those spent on restoring the damaged ecosystems to their original or expected conditions, including mainly the reclamation cost. Only reclamation cost is considered for the case study.

2.5 Ecological cost associated with damaged forest land

The total ecological cost per hectare of forest land damaged by any mining activity, C_E, is equal to the sum of the above three components:

                           (9)

where C_d and C_r are the lost value of direct eco-services and reclamation cost, respectively.

2.6 Cost of carbon emission from energy consumption

The major environmental impact of energy consumption is global warming associated with greenhouse gas emissions (mainly CO₂). Directly estimating the cost of global warming is hardly practical at present due to limited knowledge and data. One of the approaches to combat global warming is carbon capture and storage, for which pilot tests are carried out in several countries [19]. Therefore, the cost of carbon emission from energy consumption of a mining operation is estimated based on the emitted carbon amount and an assumed carbon capture and storage cost. The amount of carbon emitted from direct consumption of fossil fuels (diesel and gasoline) is calculated by their carbon emission factors, which is the mass of carbon emitted from burning unit mass of fuel. Since around 80% of total electricity is generated from coal in China, electricity consumption is converted to an equivalent amount of coal and the amount of emitted carbon is calculated by applying the carbon emission factor of coal. Thus, the cost of carbon emission from energy consumption, c_e, of mined or processed material, is

                 (10)

where e_d and e_g are the average diesel and gasoline consumptions, respectively, per ton of mined ore or waste, equal to zero for ore processing; e_e is the average electricity consumption per unit mass of mined or processed material, which is denoted as e_em for ore or waste mining and e_ep for ore processing in Table 1 in the case study; f_d, f_g, and f_c are the carbon emission factors for diesel, gasoline, and coal, respectively; b is the proportion of total electricity generated from coal; and a_c is the amount of coal required to generate a unit of electricity.

3 Ultimate pit optimization with ecological costs

A moving cone elimination algorithm is used for pit optimization. A cross section of a hypothetical block model and the moving cone elimination process is shown in Fig. 1. An initial pit is first obtained by projecting from a sufficiently large surface boundary outline down to the lowest level of the block model, according to the specified pit slope angles. Partial blocks are used at the pit wall and the ground surface to accurately represent the slope angles and the topography.

Table 1 Parameter values used in the case study

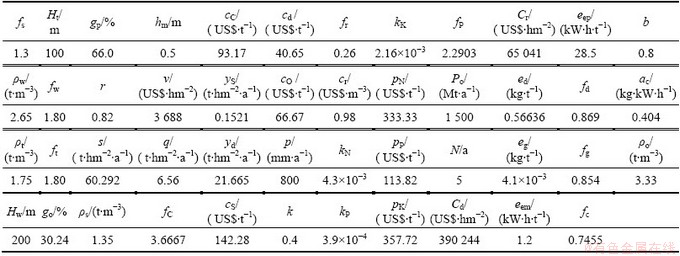
Fig. 1 Cross section of hypothetical block model and moving cone elimination process

The moving cone elimination process starts with the initial pit. The apex of the cone, with its shell slopes equal to the pit slopes in all directions, is placed at the center of a block at the central level of the lowest block layer in the pit, as shown by Cone A in Fig. 1. The profit of the cone is calculated based on the quantities of ore and waste and ore grade in the cone, and the cost, price, and recovery parameters. The cone is eliminated from the pit if its profit is negative or zero. The cone apex is then moved to all the blocks along the same horizontal block layer and the ones with negative or zero profits are eliminated. The same cone-moving, evaluation and elimination process is then repeated by moving the cone along the next higher layer of blocks. A round of cone-scanning is completed after all the block layers are considered from layer 1 to layer M. The cone-scanning process is repeated round after round until no cone is found in a round with a negative or zero profit, and the remaining portion is the optimum pit.

A problem arises when ecological costs are to be incorporated in the above pit optimization algorithm. The ecological costs cannot be calculated without knowing the quantities of materials mined and the land areas damaged, while the quantities and areas are unknown before the pit is obtained. Therefore, an iteration process is needed as described below.

Step 1: Optimize the pit using the moving cone elimination algorithm without considering ecological costs. Three different unit costs for ore mining, waste removal, and ore processing are used in the optimization, which are referred to as the “original unit costs” when ecological costs are not included and denoted by c_o, c_w, and c_p, in US$/t, respectively.

Step 2: Determine the damaged land areas associated with the pit obtained in Step 1. The mined area of the pit, A_m, is directly obtained by delineating its surface outline. The areas occupied by the waste dump and tailings pond, A_w and A_t, are estimated using Eqs. (1) and (2).

Step 3: Calculate ecological costs and attribute them to ore mining, waste removal, and ore processing. The total ecological cost associated with the mined area of the pit is converted into two unit costs, Δc_mo and Δc_mw in US$/t, which are attributed to the unit costs of ore mining and waste removal, respectively, according to the ore and waste volumes in the pit.

                         (11)

                         (12)

where ρ_o is the average density of in-situ ore.

The total ecological cost associated with the land area of the waste dump is converted into a unit cost, Δc_ww, which is attributed to the unit cost of waste removal.

                              (13)

The total ecological cost associated with the land area of the tailings pond is converted into a unit cost, Δc_tp, which is attributed to the unit cost of ore processing.

                               (14)

Let Δc_o, Δc_w, and Δc_p be the increase in the unit costs of ore mining, waste removal, and ore processing, respectively, due to ecological costs associated with damaged forest land and energy consumption. Then, we have

Δc_o=Δc_mo+c_eo                            (15)

Δc_w=Δc_mw+Δc_ww+c_ew                        (16)

Δc_p=Δc_tp+c_ep                             (17)

where c_eo, c_ew, and c_ep are the unit ecological costs of carbon emission from energy consumption in ore mining, waste removal, and ore processing, respectively, calculated using Eq. (10) based on their respective energy consumption data.

Step 4: Add Δc_o, Δc_w, and Δc_p to the corresponding original unit costs, c_o, c_w, and c_p, and optimize the pit again using the increased unit costs. Ecological costs are recalculated for the new pit and the iteration continues until the resulting pit converges, that is, when the pit obtained in a given round is the same as or sufficiently close to the one obtained in the preceding round, the final pit is the optimum pit with ecological costs.

4 Case study

The procedure outlined above is applied to a big iron ore mine in Northeastern China. The deposit has been mined for many years using open pit mining and has some 500 Mt of ore remaining. The type of the ecosystem damaged by the mining operation is forest. The current topography of the mine is taken as the starting point to design a pit for the remaining portion of the deposit. The original unit costs of ore mining, waste removal, and ore processing are 3.90, 2.44 and 21.95 US$/t, respectively. The price of concentrate is 105.69 US$/t. The pit slope angles are 34.8°, 34.5°, 51°, 42°, 48.1°, 47.5°, and 34.8°, respectively, in orientations at 21°, 41.5°, 119°, 200.5°, 224.5°, 291°, and 352.5° measured counter-clock wise from the east. The values of parameters in the equations are listed in Table 1. The parameter values related to indirect eco-services of forest are mainly from ZHAO et al [20]. The carbon emission factors for diesel, gasoline, and coal are from ZHANG [21]. All monetary values are converted to US dollars using an exchange rate of 6.15 RMB to a 1 dollar.

The pit optimization process converges after five iterations. The quantities of materials mined as ore and waste, as well as the total profits, for the optimum pits with or without ecological costs are given in Table 2. The contours of the two pits are shown in Fig. 2.

Fig. 2 Ultimate pit contours without (a) or with (b) consideration of ecological costs

Table 2 Ore and waste quantities and profits for pits with or without ecological costs

The optimum pit with ecological costs is much smaller than the optimum pit without ecological costs, with the former shrinking mainly on the left-hand side and much shallower. The quantities of ore and waste mined from the pit with ecological costs are 26.0% and 49.3% less than those from the pit without ecological costs, respectively. The total material mass decreases by 43.4% when ecological costs are considered. For the optimum pit with ecological costs, the unit ecological costs attributed to ore mining, waste removal, and ore processing are 0.237, 0.512, and 0.997 US$/t, respectively, which represent 6.1%, 21.0%, and 4.5% of the respective original unit costs. The total profit of the optimum pit with ecological costs is 25.8% less than that of the optimum pit without ecological costs. Therefore, ecological costs do make a difference.

5 Conclusions

1) Estimation of the ecological costs of open pit metal mining is outlined and a procedure is given for ultimate pit optimization with internalized ecological costs. Ecosystem services may be viewed from quite different angles and their economic values evaluated through different approaches. Therefore, the ecological costs of a given mining operation may differ to a large extent. Our intention is not to present a definitive method of ecological cost calculation, but to show that meaningful estimation of a variety of ecological costs is possible for a mining operation.

2) Though the case study is preliminary, it is possible to internalize ecological costs in mine designs. It also indicates that the pit optimization outcome shifts considerably to the conservative side and the profitability decreases substantially when ecological costs are accounted for.

3) The total damaged land areas are used in the ecological cost estimation for the case study. However, land areas are damaged gradually as the mining operation proceeds. Therefore, a more realistic approach to study the influence of ecological costs on mine planning and profitability is to internalize the ecological costs in a procedure of mine production scheduling, so that these costs are distributed over the time horizons of different schedule alternatives. We are working on an algorithm to optimize simultaneously the production schedule and the ultimate pit with internalized ecological costs with an objective of maximizing the total net present value.

References

[1] DOWD P A, ONUR A H. Open-pit optimization—Part 1: Optimal open-pit design [J]. Transactions of the Institution of Mining and Metallurgy (Section A: Mining Technology), 1993, 102: 95-104.

[2] LERCHS H, GROSSMANN I F. Optimum design of open-pit mines [J]. CIM Bulletin, 1965, 58: 47-54.

[3] ZHAO Y, KIM Y C. A new optimum pit limit design algorithm [C]// Proceedings of the 23rd International Symposium on Application of Computers and Operations Research in the Minerals Industry. Richardson: SPE of AIME, 1992: 423-434.

[4] YEGULALP T M, ARIAS J A. A fast algorithm to solve the ultimate pit limit problem [C]//Proceedings of the 23rd International Symposium on Application of Computers and Operations Research in the Minerals Industry. Richardson: SPE of AIME, 1992: 391-398.

[5] FRIMPONG S, ASA E, SZYMANSKI J. Intelligent modeling: Advances in open pit mine design and optimization research [J]. International Journal of Surface Mining, Reclamation and Environment, 2002, 16(2): 134-143.

[6] JALALI S E, ATAEE-POUR M, SHAHRIAR K. Pit limits optimization using a stochastic process [J]. Canadian Institute of Mining Magazine, 2006, 1(6): 90-94.

[7] LATORRE E, GOLOSINSKI T S. Definition of economic limits taking into consideration of time value of money [J]. Canadian Institute of Mining, Metallurgy and Petroleum (CIM) Journal, 2011, 2(3): 162-170.

[8] AKBARI A D, OSANLOO M, SHIRAZI M A. Ultimate pit limit determination through minimizing risk costs associated with price uncertainty [J]. Gospodarka Surowcami Mineralnymi-mineral Resources Management, 2008, 24(4): 157-170.

[9] SABOUR S A, DIMITRAKOPOULOS R. Incorporating geological and market uncertainties and operational flexibility into open pit mine design [J]. Journal of Mining Science, 2011, 47(2): 191-201.

[10] GROBLER F, ELKINGTON T, RENDU J M. Robust decision making—Application to mine planning under price uncertainty [C]// Proceedings of the 35th International Symposium on Application of Computers and Operations Research in the Minerals Industry. Carlton: Australasian Institute of Mining and Metallurgy, 2011: 371-380.

[11] DIMITRAKOPOULOS R, MARTINEZ L A, RAMAZAN S. A maximum upside/downside approach to the traditional optimization of open pit mine design [J]. Journal of Mining Science, 2007, 43(1): 73-82.

[12] HONG Jian-ping, WANG Jun, CHEN Hai-yan, SUN Bao-de, LI Jia-jing, CHEN Chong. Process of aluminum dross recycling and life cycle assessment for Al-Si alloys and brown fused alumina [J]. Transactions of Nonferrous Metals Society of China, 2010, 11: 2155-2161.

[13] HAKE J F, LANDWEHR U, SCHREIBER A. Integrated analysis of metallic resource flows [J]. The International Journal of Life Cycle Assessment, 1998, 3(4): 182-183.

[14] AZAPAGIC A, CLIFT R. Life cycle assessment as a tool for improving process performance: A case study on boron products [J]. The International Journal of Life Cycle Assessment, 1999, 4(3): 133-142.

[15] LI G, NIE Z, ZHOU H, DI X, LIU Y, ZUO T. An accumulative model for the comparative life cycle assessment case study: Iron and steel process [J]. The International Journal of Life Cycle Assessment, 2002, 7(4): 225-229.

[16] DURUCAN S, KORRE A, MUNOZ-MELENDEZ G. Mining life cycle modeling: A cradle-to-gate approach to environmental management in the minerals industry [J]. Journal of Cleaner Production, 2006, 14(12): 1057-1070.

[17] VEIGA M M, SCOBLE M, MACALISTAIR M L. Mining with communities [J]. Natural Resources Forum, 2001, 25(3): 191-202.

[18] ODELL C J. Integration of sustainability into the mine design process [D]. Vancouver: University of British Columbia, 2004: 86-98.

[19] FINKENRATH M. Cost and performance of carbon dioxide capture from power generation [EB/OL]. http://www.egcfe.ewg.apec.org/ projects/ EWG052010A/References/IEA_costperf_ccs_powergen.pdf, [2013-09-08].

[20] ZHAO Tong-qian, OUYANG Zhi-yun, ZHENG Hua, WANG Xiao, MIAO Hong. Forest ecosystem services and their valuation in China [J]. Journal of Natural Resources, 2004, 19(4): 480-491. (in Chinese)

[21] ZHANG Hong-wu. Energy consumption and CO₂ emission in China [J]. Journal of Shanxi Normal University, 2001, 15(4): 64-69. (in Chinese).

考虑生态成本的露天金属矿最终境界优化

胥孝川¹，顾晓薇¹，王 青¹，刘剑平^1,2，王 俊^1,3

1. 东北大学 资源与土木工程学院，沈阳 110819；

2. 东煤沈阳基础工程公司，沈阳 110016；

3. 河南理工大学 经济管理学院，焦作 454000

摘  要：量化了金属矿露天开采的生态成本，包括直接生态服务价值损失、间接生态服务价值损失、预防和恢复成本以及能耗产生的碳排放成本。通过迭代算法把这些生态成本纳入境界优化，并以实例应用说明生态成本对境界设计结果的影响。结果表明，在矿山设计中内生化生态成本是可能的。考虑生态成本使境界优化结果变得很保守，盈利也大幅度降低。

关键词：最终境界；优化；生态成本；露天金属矿

(Edited by Xiang-qun LI)

Foundation item: Project (50974041) supported by the National Natural Science Foundation of China; Project (NCET-11-0073) supported by Program for New Century Excellent Talents in University of Ministry of Education of China; Project (201102065) supported by the Natural Science Foundation of Liaoning Province, China; Project (2012921075) supported by the Ten Million Talent Project of Liaoning Province, China

Corresponding author: Xiao-wei GU; Tel: +86-24-83678400; Fax: +86-24-83680388; E-mail: guxiaowei@mail.neu.edu.cn

DOI: 10.1016/S1003-6326(14)63222-2