Numerical and experimental investigation of rock breaking method under free surface by TBM disc cutter
来源期刊:中南大学学报(英文版)2018年第9期
论文作者:夏毅敏 张旭辉 曾桂英 谭青 郭犇
文章页码:2107 - 2118
Key words:free surface; tunnel boring machine; disc cutter; rock breaking method
Abstract: To study the rock breaking method under the free surface induced by disc cutter, the rock breaking simulations were first conducted based on the discrete element method, and the dynamic process of rock breaking under the free surface was studied including stressed zone, crush zone, crack initiation and propagation. Then the crack propagation conditions, specific energy, etc. under different free surface distance (S) were also investigated combined with linear cutting experiments. The results show that the rock breaking process under the free surface induced by disc cutter is dominated by tension failure mode. There exists a critical S to promote crack propagation to free surface effectively. And this rock breaking method can improve the rock breaking force and breaking efficiency significantly when proper.
Cite this article as: ZHANG Xu-hui, XIA Yi-min, ZENG Gui-ying, TAN Qing, GUO Ben. Numerical and experimental investigation of rock breaking method under free surface by TBM disc cutter [J]. Journal of Central South University, 2018, 25(9): 2107–2118. DOI: https://doi.org/10.1007/s11771-018-3900-y.

J. Cent. South Univ. (2018) 25: 2107-2118
DOI: https://doi.org/10.1007/s11771-018-3900-y
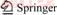
ZHANG Xu-hui(张旭辉)1, 2, XIA Yi-min(夏毅敏)1, 2, ZENG Gui-ying(曾桂英)1, 2, TAN Qing(谭青)1, 2, GUO Ben(郭犇)1, 2
1. State Key Laboratory of High Performance Complex Manufacturing (Central South University),Changsha 410083, China;
2. School of Mechanical and Electrical Engineering, Central South University, Changsha 410083, China
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2018
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2018
Abstract: To study the rock breaking method under the free surface induced by disc cutter, the rock breaking simulations were first conducted based on the discrete element method, and the dynamic process of rock breaking under the free surface was studied including stressed zone, crush zone, crack initiation and propagation. Then the crack propagation conditions, specific energy, etc. under different free surface distance (S) were also investigated combined with linear cutting experiments. The results show that the rock breaking process under the free surface induced by disc cutter is dominated by tension failure mode. There exists a critical S to promote crack propagation to free surface effectively. And this rock breaking method can improve the rock breaking force and breaking efficiency significantly when proper.
Key words: free surface; tunnel boring machine; disc cutter; rock breaking method
Cite this article as: ZHANG Xu-hui, XIA Yi-min, ZENG Gui-ying, TAN Qing, GUO Ben. Numerical and experimental investigation of rock breaking method under free surface by TBM disc cutter [J]. Journal of Central South University, 2018, 25(9): 2107–2118. DOI: https://doi.org/10.1007/s11771-018-3900-y.
1 Introduction
With the rapid development of tunnel engineering, tunnel boring machines (TBMs) are widely used in tunnel excavation due to their high excavation efficiency, excellent safety and less ground disturbance [1, 2]. TBM disc cutters are the most efficient and most popular cutting tool to cutting rock in hard rock grounds. And the TBM performance and efficiency are heavily dependent on the rock breaking characteristics induced by disc cutter.
In the past years, the rock breaking process induced by disc cutter has been investigated by many researchers by experimental method. GERTSCH et al [3] conducted a series of cutting tests with a single disc cutter to study cutting forces and cutting efficiency based on the liner cutting machine (LCM). BALCI et al [4] and TUMAC et al [5] investigated the rock cutting characteristics induced by two kinds of disc cutters by using LCM cutting tests. CHO et al [6] performed a series of rock cutting tests to study the optimum cutting condition based on the LCM. MA et al [7] and YIN et al [8] studied the influence of confining stress and joint on rock fragmentation by conducting LCM tests, respectively. GENG et al [9, 10] studied the rock cutting process of normal and gage disc cutters by conducting rotary cutting machine (RCM) tests.
Numerical methods have also been employed to study the rock breaking process induced by disc cutter due to their high efficiency and high reliability. GONG et al [11, 12] simulated the crack initiation and propagation induced by the disc cutter under different joint spacing and orientations based on the UDEC. CHO et al [13] used AUTODYN to investigate the optimum cutting condition of the disc cutter. MA et al [14] studied the rock fragmentation under different confining stress induced by disc cutters based RFPA. LIU et al [15–17] used PFC to simulate the dynamic process of crack initiation and propagation under different joint conditions. HUO et al [18] and LIU et al [19] used DYNA and ABAQUS respectively to study the influence of cutting mode and cutting order on the rock cutting efficiency and obtain the optimal cutter spacing. Based on above-mentioned researches, numerical simulation can simulate the rock fragmentation process effectively and provide better understanding of rock fragmentation mechanism induced by disc cutters.
Improving rock breaking efficiency of TBM has been paid more and more attention for hard rock grounds. Many promising new rock cutting methods induced by disc cutter have been introduced gradually to improve the rock breaking efficiency in past years. For example, CICCU et al [20] investigated the disc cutter performance assisted with water jet. HASSANI et al [21] proposed a microwave-irradiation-assisted rock breaking method and studied the influence of microwave irradiation on rock fragmentation. GENG et al [22] proposed a new rock breaking method induced by disc cutter assisted with free surface.
For the rock breaking method under the free surface of TBM, as shown in Figure 1, its cutterhead is divided into two parts including the first cutterhead and the second cutterhead, and they can be driven separately during excavating process. The small first cutterhead digs a small hole to create the free surface first. Then, the second cutterhead enlarges the hole based on the existing free surface with the disc cutters. Thus, the thrust and torque of the TBM are distributed into two stages of the cutterhead. Furthermore, the rock cutting forces of the multi-stage cutterhead applied to the main bearing and the main beam are also reduced since the rock cutting forces are also divided into two parts compared with the conventional cutterhead. GENG et al [22] designed a general structure of a small two-stage cutterhead (4 mm in diameter) and introduced the rock breaking method under the free surface by disc cutter. Unfortunately, the rock breaking mechanism and the crack propagation process induced by disc cutter under the free surface are still unclear. To understand the rock breaking method more clearly and promote the application of this method, the PFC-2D is adopted for simulating the rock breaking process induced by disc cutter under the free surface. Furthermore, the rock breaking tests under the free surface were also carried out based on the LCM.
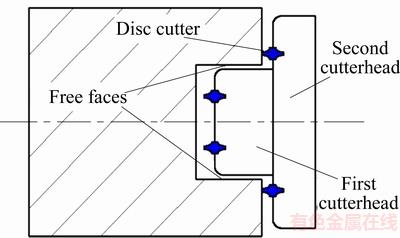
Figure 1 Multi-stage cutterhead TBM
2 Model calibration and boundary conditions
2.1 Model calibration
The PFC detailed by POTYONDY et al [23] is a discrete element simulation method that can simulate the mechanical behavior of rock or soil. And it has been widely used to solve lots of geotechnical engineering problems, such as mining and slope stability. In order to simulate a kind of rock in PFC, the macroscopic parameters of the rock should be obtained first through the laboratory tests. In this paper, the granite was chosen as the simulated rock whose major macroscopic properties are shown in Table 1. Then the microscopic parameters of particles should be decided to represent the corresponding rock in PFC. Unfortunately, there is no straightforward method to calibrate the macroscopic parameters and the microscopic parameters. To simplify the calibration procedure, MOON et al [24] evaluated the relationship between the microscopic and macroscopic properties by simulating uniaxial compressive strength (UCS) and Brazilian strength (BTS) tests. On this basis, a series of simulation tests including UCS and BTS tests are conducted to select the appropriate microscopic properties of the rock sample, as shown in Figure 2. In these UCS and BTS samples, the height and diameter of the sample are 100 and 50 mm, respectively. The proper microscopic parameters of particles corresponding to the granite are obtained through a series of simulation tests, as shown in Table 2.
Table 1 Macroscopic parameters of rock sample

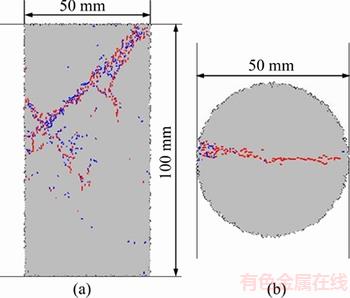
Figure 2 Numerical tests of UCS (a) and BTS (b)
Table 2 Microscopic parameters of rock sample in PFC
2.2 Numerical model establishment
The configuration of the numerical model is shown in Figure 3. In this model, the rock breaking process induced by disc cutter is taken as a penetration process without considering the rolling process of the disc cutter. Many researchers [12, 25, 26] have verified the appropriateness of 2-D equivalent model. The dimensions of the model are 500 mm×300 mm which consists of about 65922 particles. The disc cutter is simulated by walls with infinite stiffness whose cutter tip width is 20 mm and wedge angle is 20°. The rock sample is constrained by the left wall and the bottom wall. The top side of the rock sample is defined as working surface which is contacted by the disc cutter and the right side of the rock sample is defined as free surface. The distance between the center of the disc cutter and the free surface is defined as the free surface distance (S). The penetration tests with six different values of S, namely 20, 40, 60, 80, 100 and 120 mm are investigated, respectively. The crack depth (l) denotes the distance between the working surface and crack end position.
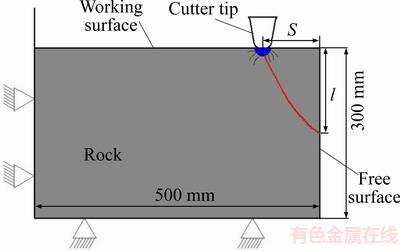
Figure 3 Numerical model of rock breaking induced by disc cutter under free surface
3 Numerical results
3.1 Dynamic process of rock breaking under free surface
3.1.1 Crack initiation and propagation
Figures 4 and 5 show the dynamic processes of internal reaction forces and crack propagation induced by the disc cutter when S is 40 mm. The compressive and tensile reaction forces are implied by black and red colors, as shown in Figure 4. The shear and tensile cracks are implied by blue and red lines respectively, as shown in Figure 5. During the beginning staging, the rock deforms elastically with the increase of the penetration. When the penetration is increased to 1.2 mm, a highly stressed zone is formed under the disc cutter, as shown in Figure 4(a). Meanwhile, a small amount of micro cracks are produced under the disc cutter,as shown in Figure 5(a). When the stressed zone develops to a certain extent, a crush zone is formed and many micro cracks are also produced under the disc cutter, as shown in Figure 5(b). But it still has no obvious tensile force under the disc cutter as shown in Figure 4(b). When the penetration is increased to 1.6 mm, the micro cracks will gather together and form a macro crack as shown in Figures 5(c) and (d). At this time, there is no reaction force around the macro crack, as shown in Figures 4(c) and (d). Then, the macro crack is gradually propagated to the free surface which is driven by tensile cracks and a complete chip will also be produced, as shown in Figures 5(e) and (f). Moreover, the tensile force can be found at the macro crack tip, as shown in Figures 4(e) and (f). It is indicated that the rock breaking method under the free surface is dominated by tension failure mode.

Figure 4 Internal reaction forces under different penetrations:
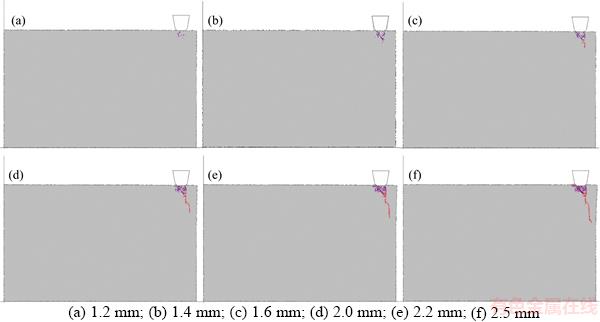
Figure 5 Crack propagation under different penetrations:
3.1.2 Number of cracks
The number of the cracks with the increase of penetration is shown in Figure 6. When the penetration is less than 1.2 mm, no cracks are produced in the rock due to the elastic deformation of the rock sample. With the increase of the penetration, the shear and tensile cracks appear simultaneously and the number of these two kinds of cracks is similar. It can be concluded that there exists shear and tensile failure in the early stages of rock fragmentation under the free surface. However, when the penetration is more than 1.5 mm, the tension crack grows rapidly and the number of the tensile crack exceeds that of the shear crack. It indicates the crack propagation is dominated by tensile failure mode under the free surface. This is consistent with the above conclusions in section 3.1.1. It should be noted that the crack growth rate remains almost constant at the crack propagation stage, as shown in Figure 6, from line a to line b. When the penetration is more than 2.5 mm, the crack growth rate decreased significantly and the number of crack changes little. In this sense, a complete rock breaking process by the disc cutter under the free surface will be over.
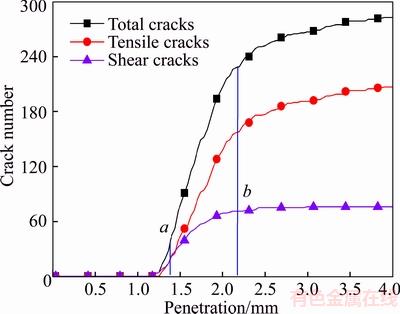
Figure 6 Variation of number of cracks with penetration
3.1.3 Cutting forces
Figure 7 shows the changes in the cutting forces (including normal force and side force) with the increase of penetration. At the very beginning of the penetration test, the normal force of the disc cutter increases linearly corresponding to the elastic deformation of the rock sample, and the side fore of the disc cutter changes little at this stage. When the penetration is increased to about 1.4 mm, the normal force achieves the peak value corresponding to a big crush zone, as shown in Figure 5(b). After the peak value, the normal force drops significantly and the side force begins to increase with the increase of the penetration. Meanwhile, the macro crack will also be formed and propagated, corresponding to Figures 5(c) and (d). When the penetration is increased to 2.5 mm, the normal force features certain volatility. And the side force still shows an increasing trend with the increase of the penetration. It implies that the side force plays an important role to the macro crack propagation when rock is broken by the disc cutter under the free surface.
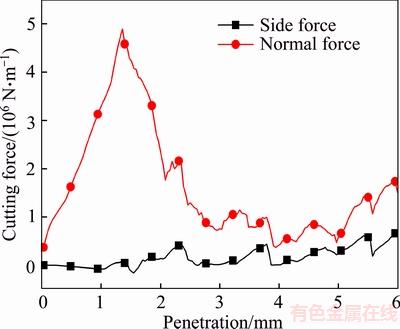
Figure 7 Variation of cutting force with penetration
3.1.4 Energy consumption
The energy-penetration response induced by the disc cutter is shown in Figure 8. Four kinds of energy are recorded in PFC including total consumption energy, friction energy, kinetic energy and strain energy which refer to the total work produced by disc cutter, the friction heat produced by particles or cutter, the energy of particle motion and the energy produced by rock elastic deformation, respectively. Based on the law of conservation of energy, the other energy including radiation energy, etc is also calculated. When the penetration is less than 1.4 mm, the total consumption energy is similar to strain energy with the increase of the penetration, and the difference between these two kinds of energy is very small. It indicates that total consumption energy of the disc cutter is mainly converted to strain energy at the initial stage of the penetration test. When the strain energy arrives its peak value, the macro crack will be initiated and propagated dramatically corresponding to Figures 5(c) and (d). Then, the strain energy is decreased dramatically and converted into friction energy or in other forms of energy with the crack propagation. It suggests that the rock broken by the disc cutter under the free surface is actually a process of energy accumulation and release.
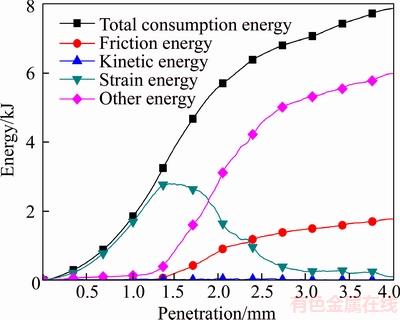
Figure 8 Energy dissipation process with penetration
3.2 Rock breaking characteristics under different S
3.2.1 Crack propagation conditions
The crack propagation under different S when the penetration is 6 mm is illustrated in Figure 9. When S is less than 80 mm, the macro crack is inclined to be propagated to the free surface and can be reached to the free surface. Moreover, a complete rock chip can also be formed, as shown in Figures 9(a)–(d). However, when S is more than 80 mm, the macro crack is inclined to be propagated along the penetration direction, as shown in Figures 9(e) and (f). In other words, for a given penetration, only when S is less than a certain value, can the free surface be used to promote the rock breaking effectively by disc cutter.
3.2.2 Crack depth and chip area
The crack depth and chip area under different S are presented in Figure 10. The crack depth increases with the increase of S, but the increase speed slows down when S is more than 80 mm. When S is less than 80 mm, the macro crack can be propagated to the free surface easily. And the bigger S is, the later the crack reaches the free surface, resulting in a increase in crack depth. When S is more than 80 mm, the macro crack can not be propagated to the free surface. In other words, the crack propagation condition is basically the same when S is more than 80 mm, resulting in little difference in crack depth. The chip area increases and then decreases with the increase of the S. This can also be explained with the crack propagation. When S is less than 80 mm, the crack depth increases with the increase of S, resulting in the increase of the rock chip. When S is more than 80 mm, the crack can not be propagated to the free surface. Thus, the rock chip area just appears around the disc cutter, resulting in a decrease of rock chip area.
3.2.3 Peak force and peak strain energy under different S
Figure 11 shows the changes in the peak force and peak strain energy induced by the disc cutter with different S. And the peak force denotes the peak value of the normal force in this work. Both the peak force and peak strain energy increase with the increase of S. In other words, it needs greater cutting force and more energy to promote rock breaking with the increase of S. In fact, when S is more than 80 mm, the rock breaking method by disc cutter under the free surface is similar to that under the conventional condition. In this sense, it shows that the rock breaking method under the free surface can improve the rock breaking force and breaking energy.

Figure 9 Crack propagation under different S:

Figure 10 Crack depth and chip area under different S
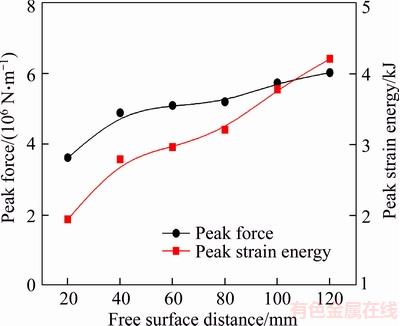
Figure 11 Peak force and peak strain energy under different S
3.2.4 Specific energy under different values of S
Specific energy (Es) refers to the energy consumed by breaking unit volume of rock [27], and the lower the Es is, the higher the rock breaking efficiency is. The Es can be calculated by the following equation:
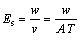 (1)
(1)
where w denotes the cutting energy; v denotes the rock broken volume; A denotes the rock broken area; and T denotes the unit thickness in PFC-2D.Figure 12 illustrates the changes in Es with different S. When S is less than 100 mm, Es decreases and then increases with the increase of S. And the peak value of Es is achieved when S is 80 mm. When S is more than 100 mm, Es changes little due to the same crack propagation mode with the conventional rock breaking method by disc cutter. It can be concluded that the rock breaking method by disc cutter under the free surface can improve rock breaking efficiency significantly when S is less than a critical value compared with the conventional rock breaking method.
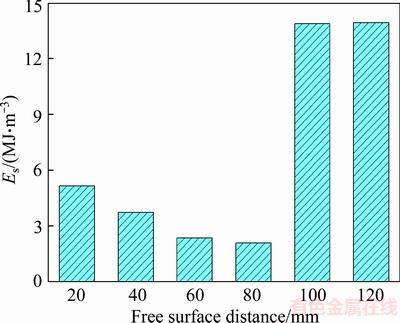
Figure 12 Specific energy under different S
4 Experiment
4.1 Experiment equipment
In order to observe the macroscopic phenomena of rock fragmentation under the free surface, the rock breaking experiments by disc cutter were conducted based on the LCM in central south university whose overall size is about 5 m× 3.2 m×3.5 m. The LCM is shown in Figure 13(a), which includes three parts: hydraulic system, testing system and mechanical cutting system. The hydraulic system can control three moving directions including longitudinal direction, vertical direction and lateral direction with the aid of the three hydraulic cylinder. In addition, the limit loads of the longitudinal, the vertical, and the lateral hydraulic cylinder are 450, 600 and 70 kN, respectively. The testing system can record the cutting force of the disc cutter during cutting process. And the mechanical cutting system with a disc cutter is mainly responsible for cutting rock sample. The cutter tip width and wedge angle of the disc cutter are about 20 mm and 20°, respectively, corresponding to the simulation cutter. The rock sample whose macro parameters are shown in Table 1 was cast in a steel box to mount its position, as shown in Figure 13(b). The rock breaking tests with six different values of S and one penetration corresponding to the simulation conditions were performed, respectively.
4.2 Dynamic process of rock fragmentation
Figure 14 illustrates the dynamic rock breaking process by disc cutter when S is 80 mm. When the cutting time (t) was less than 2.5 s, there was no obvious phenomenon for rock fragmentation due to the elastic deformation of the rock sample. When t is reached to 2.5 s, a lot of dust was produced around the disc cutter, as shown in Figure 12(a). Then, the macro crack began to be propagated and a big rock chip was also formed, as shown in Figure 12(b). Furthermore, the rock chip flew away from the rock sample quickly, as shown in Figures 12(c) and (d). This rock fragmentation process was also observed in simulation, as stated in section 3.1.1.
4.3 Rock fragmentation conditions
The rock breaking conditions under different S are shown in Figure 15. Over the range of S from 20 to 80 mm, the crack produced by disc cutter could be propagated to free surface and many big rock chips were formed, as shown in Figures 15(a)–(d). Moreover, both the crack depth and the size of the rock chip increase with the increase of S. For S is 100 or 120 mm, only one cutting groove induced by disc cutter can be observed and no crack can be propagated to the free surface, which is consistent with the rock breaking phenomenon induced by the conventional rock breaking method, as shown in Figures 15(e) and (f). It indicated that the critical value of S is 80 mm to promote the rock breaking with the free surface by disc cutter when the penetration is 6 mm. This is also consistent with the numerical results.
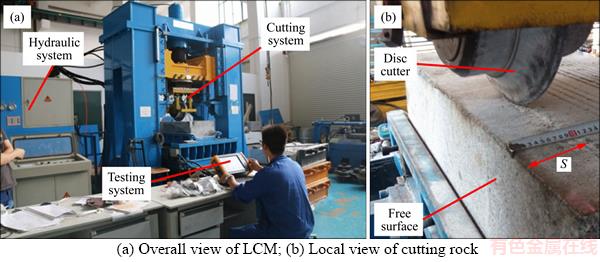
Figure 13 Linear cutting machine:

Figure 14 Rock breaking process:

Figure 15 Rock breaking conditions under different S:
4.4 Specific energy under different S
The experimental and numerical results of Es under different S are shown in Figure 16. Both the numerical and experimental Es decrease and then increase with the increase of S. In other words, the change trend of the two kinds of results about the Es is basically the same with the increase of S. Over the range of S from 20 to 80 mm, the Es values obtained from simulations are close to that obtained from experiments. However, when S is more than 80 mm, Es values obtained from the rock breaking experiments show large discrepancy to those from the numerical simulations. It can be explained that the rock chips produced in experiment can not be collected completely since the rock chips are shown like powder and no big rock chips produced when S is more than 80 mm. It should be noted that Es values obtained are far lower when S is 20, 40, 60 and 80 mm than those when S is 100 and 120 mm. As stated in section 3.2.4, when S is less than a critical value, such as 80 mm in this work, the rock breaking method by disc cutter under the free surface can improve rock breaking efficiency significantly.

Figure 16 Comparison between experimental and numerical Es under different S
4.5 Application
According to the above research, the rock breaking method by disc cutter under the free surface can improve the rock breaking force and breaking efficiency significantly when S is less than a critical value. These research results can strengthen the confidence to apply this rock breaking method to the multi-stage cutterhead TBM. To use the free surface to promote rock fragmentation effectively, S should be controlled within 80 mm when the penetration of the disc cutter is 6 mm.
It should be pointed out that the multi-stage cutterhead still have some problems to be solved although it has more advantages compared with the conventional cutterhead, such as its deslagging problem and the possible structure. To slag smoothly, both the first cutterhead and the second cutterhead should have their slag holes, which can be distributed on the edge of the first cutterhead and the second cutterhead based on the conventional cutterhead. Meanwhile, the layout of the slag holes should be studied deeply to improve the deslagging efficiency. In addition, GENG et al [22] designed a general structure of a small two-stage cutterhead preliminarily. And the diameter of the first cutterhead and the second cutterhead is 2310 and 4000 mm, respectively. Meanwhile, the distance between the two stage cutterhead surfaces is 300 mm, which will produce a 300 mm-height free face. But it is still far from practical application regarding manufacturing requirements and detail design. These problems should be studied and solved in future study.
5 Conclusions
1) With the increase of the penetration of the disc cutter, a highly stressed zone is produced first under the disc cutter, then a crush zone is formed which contains a few micro cracks, and finally a macro crack driven by tension failure mode begins to be propagated to the free surface when S is proper.
2) For a given penetration with 6 mm, the crack can be propagated to the free surface when S is less than 80 mm. However, when S is more than 80 mm, the free surface can not be used to promote rock fragmentation by the disc cutter. Meanwhile, the crack depth, peak force and peak strain energy all increase with the increase of S.
3) The Es first decreases and then increases with the increase of S, and the Es values obtained are far lower when S is 20, 40, 60 and 80 mm than those when S is 100 and 120 mm based on the numerical and experimental results.
4) The rock breaking method under the free surface by the disc cutter can improve the rock breaking force and breaking efficiency significantly, and this method can be used to the multi-stage cutterhead TBM.
References
[1] HUO Jun-zhou, SUN Xiao-long, LI Guang-qin, LI Tao, SUN Wei. Multi-degree-of-freedom coupling dynamic characteristic of TBM disc cutter under shock excitation [J]. Journal of Central South University, 2015, 22(9): 3326–3337. DOI: 10.1007/s11771-015-2873-3.
[2] ZHANG Zhao-huang, MENG Liang, SUN Fei. Rock deformation equations and application to the study on slantingly installed disc cutter [J]. Acta Mechanica Sinica, 2014, 30(4): 540–546. DOI: 10.1007/s10409-014-0056-3.
[3] GERTSCH R, GERTSCH L, ROSTAMI J. Disc cutting tests in colorado red granite: Implications for TBM performance prediction [J]. International Journal of Rock Mechanics and Mining Sciences, 2007, 44(2): 238–246. DOI: 10.1016/ j.ijrmms.2006.07.007.
[4] BALCI C, TUMAC D. Investigation into the effects of different rocks on rock cuttability by a V-type disc cutter [J]. Tunnelling and Underground Space Technology, 2012, 30(4): 183–193. DOI: 10.1016/j.tust.2012.02.018.
[5] TUMAC D, BALCI C. Investigations into the cutting characteristics of CCS type disc cutters and the comparison between experimental, theoretical and empirical force estimations [J]. Tunnelling and Underground Space Technology, 2012, 45: 84–98. DOI: 10.1016/j.tust. 2014.09.009.
[6] CHO J W, JEON S, JEONG H Y, CHANG S H. Evaluation of cutting efficiency during TBM disc cutter excavation within a Korean granitic rock using linear-cutting-machine testing and photogrammetric measurement [J]. Tunnelling and Underground Space Technology, 2013, 35(4): 37–54. DOI: 10.1016/j.tust.2012.08.006.
[7] MA Hong-su, GONG Qiu-ming, WANG Jun, YIN Li-jun, ZHAO Xiao-bao. Study on the influence of confining stress on TBM performance in granite rock by linear cutting test [J]. Tunnelling and Underground Space Technology, 2016, 57(2): 145–150. DOI: 10.1016/j.tust.2016.02.020.
[8] YIN Li-jun, MIAO Chong-tong, HE Guan-wen, DAI Fu-chu, GONG Qiu-ming. Study on the influence of joint spacing on rock fragmentation under TBM cutter by linear cutting test. Tunnelling and underground space technology [J]. Tunnelling and Underground Space Technology, 2016, 57(2): 137–144. DOI: 10.1016/j.tust.2016.02.018.
[9] GENG Qi, WEI Zheng-ying, MENG Hao. An experimental research on the rock cutting process of the gage cutters for rock tunnel boring machine (TBM) [J]. Tunnelling and Underground Space Technology, 2016, 52(12): 182–191. DOI: 10.1016/j.tust.2015.12.008.
[10] GENG Qi, WEI Zheng-ying, MENG Hao, CHEN Qiao. Numerical and experimental research on the rock-breaking process of tunnel boring machine normal disc cutters [J]. Journal of Mechanical Science and Technology, 2016, 30(4): 1733–1745. DOI: 10.1007/s12206-016-0329-9.
[11] GONG Qiu-ming, JIAO Yi, ZHAO Jian. Numerical modelling of the effects of joint spacing on rock fragmentation by TBM cutters [J]. Tunnelling and Underground Space Technology, 2006, 21(1): 46–55. DOI: 10.1016/j.tust.2005.06.004.
[12] GONG Qiu-ming, ZHAO Jian, JIAO Yi. Numerical modeling of the effects of joint orientation on rock fragmentation by TBM cutters [J]. Tunnelling and Underground Space Technology, 2005, 20(1): 183–191. DOI: 10.1016/j.tust.2004.08.006.
[13] CHO J, JEON S, YU S, CHANG S. Optimum spacing of TBM disc cutters: A numerical simulation using the three-dimensional dynamic fracturing method [J]. Tunnelling and Underground Space Technology, 2010, 25(3): 230–244. DOI: 10.1016/j.tust.2009.11.007.
[14] MA Hong-su, YIN Li-jun, JI Hong-guang. Numerical study of the effect of confining stress on rock fragmentation by TBM cutters [J]. International Journal of Rock Mechanics and Mining Sciences, 2011, 48(6): 1021–1033. DOI: 10.1016/j.ijrmms.2011.05.002.
[15] LIU Jie, CAO Ping, LI Kai-hui. A study on isotropic rock breaking with tbm cutters under different confining stresses [J]. Geotechnical and Geological Engineering, 2015, 33(6): 1379–1394. DOI: 10.1007/s10706-015-9907-3.
[16] LIU Jie, CAO Ping, JIANG Zhe, ZHAO Yan-lin, CAO Ri-hong. Numerical simulation on effects of embedded crack on rock fragmentation by a tunnel boring machine cutter [J]. Journal of Central South University, 2014, 21(8): 3302–3308. DOI: 10.1007/s11771-014-2303-y.
[17] LIU Jie, CAO Ping, DU Chun-huang, JIANG Zhe, LIU Jing-shuo. Effects of discontinuities on penetration of TBM cutters [J]. Journal of Central South University, 2015, 22(9): 3624–3632. DOI: 10.1007/s11771-015-2903-1.
[18] HUO Jun-zhou, YANG Jing, SUN Wei, LI Qing-yu. Simulation and optimization design of three-dimensional rotating cutting action of TBM cutter group with different modes [J]. Journal of Harbin Engineering University, 2014, 35(11): 1403–1408. DOI: 10.3969/ j.issn.1006-7043. 201307062. (in Chinese)
[19] LIU Jian-qin, LIU Meng-meng, GUO Wei. Research on the simulation of cutting rock rotary by hard rock tunnel boring machine disc cutters [J]. Journal of Mechanical Engineering, 2015, 51(9): 199–205. DOI: 10.3901/JME. 2015.09.199. (in Chinese)
[20] CICCU R, GROSSO B. Improvement of disc cutter performance by water jet assistance [J]. Rock Mechanics and Rock Engineering, 2014, 47(2): 733–744. DOI: 10.1007/ s00603-013-0433-4.
[21] HASSANI F, NEKOOVAGHT P M, GHARIB N. The influence of microwave irradiation on rocks for microwave-assisted underground excavation [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2016, 8(1): 1–15. DOI: 10.1016/j.jrmge.2015.10.004.
[22] GENG Qi, WEI Zheng-ying, MENG Hao, MACIAS F, BRULAND A. Free-face-assisted rock breaking method based on the multi-stage tunnel boring machine (TBM) cutterhead [J]. Rock Mechanics and Rock Engineering, 2016, 49(11): 4459–4472. DOI: 10.1007/s00603-016-1053-6.
[23] POTYONDY D, CUNDALL P. A bonded-particle model for rock [J]. International Journal of Rock Mechanics and Mining Sciences, 2004, 41(8): 1329–64. DOI: 10.1016/ j.ijrmms.2004.09.011.
[24] MOON T, OH J. A study of optimal rock-cutting conditions for hard rock tbm using the discrete element method [J]. Rock Mechanics and Rock Engineering, 2012, 45(5): 837–849. DOI: 10.1007/s00603-011-0180-3.
[25] HUANG Hai-ying, DETOURNAY E. Discrete element modeling of tool-rock interaction II: Rockindentation [J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2013, 37(13): 1913–1929. DOI: 10.1002/ nag.2114.
[26] INNAURATO N, OGGERI C, ORESTE P P, VINAI R. Experimental and numerical studies on rock breaking with TBM tools under high stress confinement [J]. Rock Mechanics and Rock Engineering, 2007, 40(5): 429–451. DOI: 10.1007/s00603-006-0109-4.
[27] TEALE R. The concept of specific energy in rock drilling [J]. International Journal of Rock Mechanics and Mining Science and Geomechanics Abstracts, 1965, 2(1): 57–73. DOI: 10.1016/0148-9062(65)90022-7.
(Edited by FANG Jing-hua)
中文导读
临空面下滚刀破岩方法数值模拟及试验研究
摘要:为了研究临空面下滚刀新型破岩方法,采用离散元法建立了临空面下滚刀破岩模型,模拟了临空面下滚刀作用后密实核形成、裂纹起裂、裂纹扩展等动态过程,并结合线性切割实验研究了不同临空距下裂纹扩展模式、比能耗等变化规律。研究结果表明:临空面下滚刀破碎岩石过程以张拉破坏为主,存在一个临界临空距促使裂纹能有效扩展到临空面。当临空距合理时,临空面下滚刀新型破岩方法能显著降低破岩载荷并提升破岩效率。
关键词:临空面;隧道掘进机;滚刀;破岩方法
Foundation item: Project(2013CB035401) supported by the National Basic Research Program of China; Project(2012AA041803) supported by the National High-Technology Research and Development Program of China; Project(51475478) supported by the National Natural Science Foundation of China; Project(2015GK1029) supported by the Science and Technology Project of Strategic Emerging Industry in Hunan Province, China; Project(CX2017B048) supported by the Hunan Provincial Innovation Foundation For Postgraduate, China
Received date: 2017-07-01; Accepted date: 2017-10-16
Corresponding author: XIA Yi-min, PhD, Professor; Tel: +86-731-88876926; E-mail: xiaymj@csu.edu.cn; ORCID: 0000-0001- 6174-0377

