
Influence of two-step cooling method on magnetic properties of Fe82Mo7B10Cu1 nanocrystalline alloy
PENG Kun(彭 坤), ZHOU Ling-ping(周灵平), HU Ai-ping (胡爱平), ZHU Jia-jun(朱家俊), LI De-yi(李德意)
College of Materials Science and Engineering, Hunan University, Changsha 410082, China
Received 24 September 2007; accepted 28 March 2008
Abstract: Soft magnetic properties of Fe82Mo7B10Cu1 nanocrystalline alloy were studied as a function of cooling condition. The results show that higher permeability and relaxation frequency can be obtained by the two-step cooling method, and the pinning field of the sample obtained by this method is smaller than that of the furnace-cooled and water-quenched samples. This phenomenon was interpreted in terms of internal stress and the magnetic ordering of the residual amorphous phase. The two-step cooling treatment is an effective way to improve the soft magnetic properties of Fe82Mo7B10Cu1 nanocrystalline alloy.
Key words: Fe-M-B alloy; nanocrystalline alloy; magnetic properties; two-step cooling method
1 Introduction
Nanocrystalline soft magnetic materials are of great interest because of their promising technological applications. Among the Fe-based alloys, considerable attention is devoted to the Fe-M-B (M=Zr, Nb, Hf) type alloys, which is called Nanoperm. The nanocrystalline microstructure is usually obtained by controlling the crystallization process of the as-quenched amorphous ribbons. The resultant microstructure is composed of nanograin of α-Fe and an M and B-rich amorphous phase and shows excellent soft magnetic properties. The excellent soft magnetic properties are dependent on the microstructure of the sample. The application of nanocrystalline soft magnetic materials in high-frequency range requires the optimization of their dynamic properties such as the complex permeability[1]. Factors affecting the complex permeability have been received great attention. The influences of B content and magnetic field annealing on the soft magnetic properties of Fe-M-B nanocrystalline materials were investigated[2-3]. Refs.[4-7] revealed that the corrosion, nitridation and oxidation of the surface of nanocrystalline soft magnetic materials lead to the changes of the initial permeability μi, the relaxation frequency f0 and the product μi?f0. And the effect of cooling condition on soft magnetic properties was investigated in Co-based amorphous alloys[8] and nanocrystalline alloy[1-9]. On one hand, slow cooling below the Curie temperature leads to the ordering of magnetic pairs[10], which increases anisotropy and decreases permeability. On the other hand, rapid cooling induces internal thermal stress, which is accompanied by poor soft magnetism. However, the effect of cooling condition after annealing on soft magnetic properties for Fe-based nanocrystalline alloys has not been examined in detail. The effects of two-step cooling method after crystallization on the magnetic properties are studied for Fe82Mo7B10Cu1 alloy in this work.
2 Experimental
Ingots of Fe82Mo7B10Cu1 (mole fraction, %) alloys were prepared by arc melting in pure argon atmosphere and the Fe82Mo7B10Cu1 amorphous ribbons with 1 mm in width and about 20 μm in thickness were obtained by the single-roller melt spinning method. And these ribbons were wound into toroidal cores of 5 mm in inner diameter and 6 mm in outer diameter for the permeability measurements. The microstructure was examined by X-ray diffractometry(XRD) with Cu Kα radiation. The complex permeability was measured under applied field by an impedance analyzer HP4284A.
In order to investigate the influence of cooling condition on the magnetic properties of Fe82Mo7B10Cu1 nanocrystalline alloy, the as-quenched ribbons were wounded into a torodial core, and the cores were placed in a hermetical vacuum quartz glass pipe. The cores were subsequently annealed at 873 K for 30 min, and then cooled to room temperature under various cooling conditions, in which the samples (A, B, C, D and E) were cooled to various temperature(TF) in furnace and then by water quenching. Fig.1 schematically describes the process of cooling conditions.

Fig.1 Process of heat treatment with various cooling conditions
3 Results and discussion
The as-quenched ribbons are confirmed to be amorphous by XRD analysis. The nanocrystalline alloys can be obtained by annealing at 873 K for 30 min, as shown in Fig.2. The cooling conditions have no obvious effects on the microstructure, and the average grain sizes evaluated by the Scherrer’s equation are 23-28 nm.

Fig.2 XRD spectra of as-quenched ribbons and annealed sample
The magnetic moments of the sample is varied with the structure and magnetic phase transformation during the thermocycle and can be sensitively detected by the thermomagnetic analysis (TMA). Fig.3 shows the temperature dependence of magnetization for water quenched and furnace cooled samples. The M—T curves show the features of a two-phase system, i.e. a two-stage fall to zero in the magnetization, which is associated with the Curie temperatures of the residual amorphous phase and the bcc nanocrystalline phase. The cooling conditions have no obvious effect on the Curie temperature and the volume fraction of the residual amorphous phase, indicating that the cooling conditions have no obvious effect on the composition of the crystalline and residual amorphous phase, which is in agreement with the HASIAK et al ’s results[9]. Therefore, the Curie temperatures of the residual amorphous (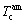 ) in the samples obtained by different cooling method can be considered to be equivalent.
) in the samples obtained by different cooling method can be considered to be equivalent.

Fig.3 Temperature dependence of magnetization for water quenched and furnace cooled samples
Fig.4 shows the μ′—H curves of samples A, C and E, disclosing the amplitude dependence of the real part of the permeability on the applied fields. The regularities of the changes of μ′ with the applied field amplitude in all samples exhibit a qualitatively similar behavior. However, the extents of μ′ varying with field are different. The extent of μ′ varying with applied magnetic field in sample C is obviously smaller than that of samples A and E, and the maximum values of μ′ for all samples are approximately equivalent. It is clear that the permeability μ′ exhibits an almost constant behavior at low fields, increases with the field increasing, and then decreases after a maximum. These three stages correspond to three magnetization stages, that is, reversible domain wall bulging, irreversible domain wall displacement and saturation. As the amplitude of the applied magnetic field increases, the μ′ increases. This indicates that very small applied field only causes the domain wall to bulge in the direction of applied magnetic field and the domain walls remains to be pinned at the original sites. There is a so-called pinning field, below which the fixed domain walls cannot be unpinned from their initial sites, and this magnetization process is reversible. However, when the applied field is larger than the pinning field, the domain wall will be unpinned, which leads to the irreversible magnetization process. It is clear that when TF>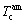 , the internal stress in the sample decreases with the decreasing of TF, which results in the decrease of the extent of domain wall pinning, thus, the extent of μ′ increasing with applied magnetic field decreases with the decreasing of TF. In the Nanoperm alloys, since there is a number of residual amorphous phases in the materials, the nucleation of short-range atomic ordering in domain wall in the process of cooling also affects the extent of domain wall pinning. Since the domain walls act as nucleation sites of short-range atomic ordering[8], the slower cooling rate will result in the more short-range ordering configurations forming in the domain walls. This will make the extent of domain wall pinning increase. When TF is lower than
, the internal stress in the sample decreases with the decreasing of TF, which results in the decrease of the extent of domain wall pinning, thus, the extent of μ′ increasing with applied magnetic field decreases with the decreasing of TF. In the Nanoperm alloys, since there is a number of residual amorphous phases in the materials, the nucleation of short-range atomic ordering in domain wall in the process of cooling also affects the extent of domain wall pinning. Since the domain walls act as nucleation sites of short-range atomic ordering[8], the slower cooling rate will result in the more short-range ordering configurations forming in the domain walls. This will make the extent of domain wall pinning increase. When TF is lower than 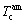 , the appearance of short-range atomic ordering leads to the increase of domain wall pinning, and then leads to the extent of the μ′ increasing with applied magnetic field increase with decreasing of TF.
, the appearance of short-range atomic ordering leads to the increase of domain wall pinning, and then leads to the extent of the μ′ increasing with applied magnetic field increase with decreasing of TF.

Fig.4 μ′-H curves of samples A, C and E
Fig.5 shows the permeability spectra (0.4 A/m applied field) of Fe82Mo7B10Cu1 nanocrystalline alloy as a function of cooling conditions. The permeability spectra show a typical relaxation dispersion. The real part of the permeability is independent of frequency at low frequencies and decreases at higher frequencies. The relaxation frequency f0 is determined from the imaginary permeability spectra which correspond to the maximum of μ′. For samples under different cooling conditions, the real part of the permeability of sample with furnace cooling is higher than that with water quenching. This high permeability is due to the release of internal thermal stress by slow cooling. On the other hand, the slow cooling through Curie temperature can lead to magnetic ordering[8], resulting in the decrease of soft magnetic properties. In order to enhance the soft magnetic properties by co-reducing internal thermal stress and magnetic ordering, the furnace cooling (cooling to temperature TF in furnace) and then water-quenching methods (two-step cooling method) were applied. As expected, the samples obtained by two-step cooling method show higher permeability. When TF is higher than the Curie temperature of the residual amorphous phase 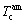 , the permeability increases with the decreasing of TF, and the permeability reaches the maximum value when TF is closed to
, the permeability increases with the decreasing of TF, and the permeability reaches the maximum value when TF is closed to 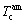 , which is the result of the decreasing of internal stress. When TF is below the Curie temperature of the residual amorphous phase, the permeability decreases, which is the result of magnetic ordering. This superior soft magnetic property obtained by two-step cooling method is consumed to be due to the combination of two associated effects: the relief of internal thermal stress by slow cooling to
, which is the result of the decreasing of internal stress. When TF is below the Curie temperature of the residual amorphous phase, the permeability decreases, which is the result of magnetic ordering. This superior soft magnetic property obtained by two-step cooling method is consumed to be due to the combination of two associated effects: the relief of internal thermal stress by slow cooling to 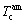 and the suppression of magnetic ordering by passing
and the suppression of magnetic ordering by passing 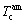 with high cooling rate. Water quenching may induce internal stress in the sample. The internal stress acts as pinning sites of the domain walls, which causes a decrease of the magnetic permeability and an increase of the stabilization field[9], and this will lead to high relaxation frequency. Therefore, the relaxation frequency of samples A, B, C and D is higher than that of sample E, as shown in Fig.5. In addition, the relaxation frequency of samples B, C and D is higher than that of sample A, which is the result of ordering of magnetic pairs.
with high cooling rate. Water quenching may induce internal stress in the sample. The internal stress acts as pinning sites of the domain walls, which causes a decrease of the magnetic permeability and an increase of the stabilization field[9], and this will lead to high relaxation frequency. Therefore, the relaxation frequency of samples A, B, C and D is higher than that of sample E, as shown in Fig.5. In addition, the relaxation frequency of samples B, C and D is higher than that of sample A, which is the result of ordering of magnetic pairs.

Fig.5 Influence of cooling conditions on complex permeability spectra of Fe82Mo7B10Cu1 nanocrystalline alloy: (a) Real part of permeability; (b) Imaginary part of permeability
The magnetization under a small applied magnetic field is accompanied by the domain wall motion impeded by the defects or impurities. Domain wall dynamics in soft magnetic materials can be described by the following equation [10-11]:
 (1)
(1)
where m is the effective mass of the domain wall, x is the domain wall displacement, β is the damping term, α is the restoring term, Ms is the saturation magnetization, H(t) is the time-dependent excitation field and t is the time. Eqn.(1) can be solved for the stationary[12], forced oscillation condition of a driving field H=H0 exp(-iωt), in terms of the real and imaginary parts of the susceptibility, and the real and imaginary parts of the permeability can be obtained. H0 is the amplitude of the applied field. The inertia term md2x/dt2 is much smaller than the damping term βdx/dt and can be neglected, therefore, the solution to this equation for the relaxation frequency is ω0=α/β(ω=2πf is the angular frequency), thus the relaxation frequency f0 can be obtained.
 (2)
(2)
For high permeability materials, the initial permeability can also be approximately expressed as [10-13]
 (3)
(3)
where l is the distance between pinning edges and γ is the wall energy. Take the expression of the restoring coefficient α[12,13] and damping coefficient β[13-15] into account, then the following equation can be obtained:
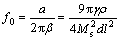 (4)
(4)
where ρ is the electrical resistivity and d is the ribbon thickness.
It is clear that the permeability is proportional to the distance between pinning edges. So larger permeability can be obtained in sample C. And the relaxation frequency is proportional to the resistivity of materials and inversely proportional to the distance between pinning edges. With the decreasing of TF, the residual stress will relax, which leads to small resistivity and domain wall pinning. In addition, the increase of the ordering of magnetic pairs will reduce the amount of magnetic polarization and then result in small resistivity in the residual amorphous layer[16], and the character of residual amorphous layer has an important influence on magnetic properties of sample[17]. The electrical resistivity ρ of samples C, D and E are about 12.1×10-8, 11.5×10-8 and 8.5×10-8 Ω/m, respectively. The electrical resistivity of the sample cooled in furnace is obviously small than others. The ordering of magnetic pairs will also enhance the pinning and reduce the distance between pinning edges. These factors will influence the value of relaxation frequency, which depends on the results of the co-operation of internal stress and magnetic order as discussed above. Therefore, the higher relaxation frequency can be obtained in sample C.
4 Conclusions
The two-step cooling method can improve the soft magnetic properties. The pinning field of the sample obtained by this method is smaller than that of the furnace-cooled and water-quenched sample, but the cooling method has no obvious effect on the maximum values of μ′. This phenomenon is interpreted in terms of internal stress and the magnetic ordering of the residual amorphous phase. The two-step cooling treatment is an effective way to improve the soft magnetic properties of Fe82Mo7B10Cu1 nanocrystalline alloy.
References
[1] QIN W, XU F PENG K, GAO W L, DU Y W. Effect of cooling rate on dynamic magnetization of Fe86Zr7B6Cu1 nanocrystalline alloy [J]. Mater Res Bull, 2002, 37: 1393-1399.
[2] CONDE C F, BLAZQUEZ J S, FRANCO V, CONDE A, ?VEC P JANI?KOVI? D. Microstructure and magnetic properties of FeMoBCu alloys: Influence of B content [J]. Acta Materialia, 2007, 55: 5675-5683.
[3] ITO N, MICHELS A, KOHLBRECHER J. Effect of magnetic field annealing on the soft magnetic properties of nanocrystalline materials [J]. J Magn Magn Mater, 2007, 316: 458-461.
[4] XU F, QIN W, PENG K, GAO W L, DU Y W. Effects of slight oxidation on the dynamic magnetization of nanocrystalline FeNbBCu ribbons [J]. Mater Letters, 2003, 57: 4227-4231.
[5] PENG K, TANG Y H, ZHOU L P, TAMG J C, XU F, DU Y W. Influence of corrosion on the magnetic properties of amorphous and nanocrystalline FeZrNbBCu alloy [J]. Physica B, 2005, 366: 110-115.
[6] QIN W, GAO W L, XU F, PENG K, YANG S, GU B X, DU Y W. The effects of nitridation on the permeability-frequency spectra in nanocrystalline Fe88Zr7B4Cu alloys [J]. J Phys D: Appl Phys, 2003, 36: 63-67.
[7] TANG J C, LIU W S, HE Y H, XUE X. Effect of biasing magnetic field on the complex permeability of nanocrystalline Fe86Zr7B6Cu1 alloy [J]. Physica B, 2007, 389: 343-346.
[8] YANG J H, KIM Y B, RYU K S, KIM M J, CHUNG Y C, KIM T K. Enhancement of permeability of Co-based amorphous alloy by two-step cooling method [J]. J Magn Magn Mater, 2000, 222: 65-69.
[9] HASIAK M, ZBROSZCZYK J, OLSZEWSKI J, CIURZY?SKA W H, WYSTOCKI B, BLACHOWICZ A. Effect of cooling rate on magnetic properties of amorphous and nanocrystalline Fe73.5Cu1Nb3Si15.5B7 alloy [J]. J Magn Magn Mater, 2000, 215-216: 410-412.
[10] GYORGY E M. Treatise in solids state chemistry: Defects in solids [M]. Vol.2. HANNAY N B, ed. New York: Plenum, 1975: 395.
[11] CULLITY B D. Introduction to magnetic materials [M]. Amsterdam: Addison-Wesley, 1972: 447.
[12] VELLA-COLEIRO G P, SMITH D H, VAN UITERT L G. MA. Resonant motion of domain walls in yttrium gadolinium iron garnets [J]. J Appl Phys, 1972, 43: 2428-2430.
[13] AGUILAR-SAHAGUN G, QUINTANA P, AMANO E, IRVINE J T S, VALENZUELA R. Equation of motion of domain walls and equivalent circuits in soft ferromagnetic materials [J]. J Appl Phys, 1994, 75: 7000-7004.
[14] TURETLLI R S, GR?SSINGER R, KUSSBACH C, SINNECKER J P. Study of frequency dependencies of the complex magnetic ac-permeability after effect in amorphous Fe64Co21B15 and Fe8Co77B15 alloys [J]. J Appl Phys, 1998: 83(3): 1581-1587.
[15] ALLIA P, BEATRICE C, VINAI F, MAZZETTI P. Evidence for a magnetic permeability relaxation of dissipative type in amorphous ferromagnetic alloys [J]. Appl Phys Lett, 1987, 51: 142-144.
[16] JU H L, K WON C, LI Q, GREENE R L, VENKATESAN T. Giant magnetoresistance in La1-xSrxMnOz films near room temperature [J]. Appl Phys Lett, 1994, 65(16): 2108-2110.
[17] PENG K, LIU F S, FU D F, HUANG Z G, XU F, DU Y W. High-temperature magnetic properties of Fe-based nanocrystalline alloy[J]. Mater Chem & Phys, 2005, 89: 138-142.
Foundation item: Project(50501008) supported by the National Natural Science Foundation of China
Corresponding author: PENG Kun; Tel: +86-731-8821778; E-mail: kpeng@hnu.cn
(Edited by YUAN Sai-qian)