Review of aerodynamics of high-speed train-bridge system in crosswinds
来源期刊:中南大学学报(英文版)2020年第4期
论文作者:何旭辉 李欢
文章页码:1054 - 1073
Key words:high-speed railway; train-bridge system; wind barrier; crosswinds; aerodynamic assessment; wind tunnel test
Abstract: Serviceability and running safety of the high-speed train on/through a bridge are of major concern in China. Due to the uncertainty chain of the train dynamic analysis in crosswinds originating mainly from the aerodynamic assessment, this paper primarily reviews five meaningful progresses on the aerodynamics of the train-bridge system done by Wind Tunnel Laboratory of Central South University in the past several years. Firstly, the flow around the train and the uncertainty origin of the aerodynamic assessment are described from the fluid mechanism point of view. After a brief introduction of the current aerodynamic assessment methods with their strengths and weaknesses, a new-developed TRAIN-INFRASTRUCTURE rig with the maximum launch speed of 35 m/s is introduced. Then, several benchmark studies are presented, including the statistic results of the characterized geometry parameters of the currently utilized bridge-decks, the aerodynamics of the train, and the aerodynamics of the flat box/truss bridge-decks. Upon compared with the foregoing mentioned benchmarks, this paper highlights the aerodynamic interference of the train-bridge system associated with its physical natures. Finally, a porosity- and orientation-adjustable novel wind barrier with its effects on the aerodynamics of the train-bridge system is discussed.
Cite this article as: HE Xu-hui, LI Huan. Review of aerodynamics of high-speed train-bridge system in crosswinds [J]. Journal of Central South University, 2020, 27(4): 1054-1073. DOI: https://doi.org/10.1007/s11771-020-4351-9.

J. Cent. South Univ. (2020) 27: 1054-1073
DOI: https://doi.org/10.1007/s11771-020-4351-9
HE Xu-hui(何旭辉)1, 2, 3, LI Huan(李欢)1, 2, 3
1. School of Civil Engineering, Central South University, Changsha 410075, China;
2. National Engineering Laboratory for High Speed Railway Construction, Central South University, Changsha 410075, China;
3. Joint International Research Laboratory of Key Technology for Rail Traffic Safety, Central South University, Changsha 410075, China
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020
Abstract: Serviceability and running safety of the high-speed train on/through a bridge are of major concern in China. Due to the uncertainty chain of the train dynamic analysis in crosswinds originating mainly from the aerodynamic assessment, this paper primarily reviews five meaningful progresses on the aerodynamics of the train-bridge system done by Wind Tunnel Laboratory of Central South University in the past several years. Firstly, the flow around the train and the uncertainty origin of the aerodynamic assessment are described from the fluid mechanism point of view. After a brief introduction of the current aerodynamic assessment methods with their strengths and weaknesses, a new-developed TRAIN-INFRASTRUCTURE rig with the maximum launch speed of 35 m/s is introduced. Then, several benchmark studies are presented, including the statistic results of the characterized geometry parameters of the currently utilized bridge-decks, the aerodynamics of the train, and the aerodynamics of the flat box/truss bridge-decks. Upon compared with the foregoing mentioned benchmarks, this paper highlights the aerodynamic interference of the train-bridge system associated with its physical natures. Finally, a porosity- and orientation-adjustable novel wind barrier with its effects on the aerodynamics of the train-bridge system is discussed.
Key words: high-speed railway; train-bridge system; wind barrier; crosswinds; aerodynamic assessment; wind tunnel test
Cite this article as: HE Xu-hui, LI Huan. Review of aerodynamics of high-speed train-bridge system in crosswinds [J]. Journal of Central South University, 2020, 27(4): 1054-1073. DOI: https://doi.org/10.1007/s11771-020-4351-9.
1 Introduction
By the end of 2019, the total track mileage of the Chinese high-speed railway (HSR) is over 35000 km, already finishing the 2004 program, i.e., the “four vertical and horizontal” corridors. In order to fulfill the further revised Medium- and Long-term Railway Plan in 2016, the Chinese HSR is expanding to southeast coastal and southwest mountainous areas where are characterized by adverse geological conditions. In order to meet the stringent requirements of the track regularity, numerous railway bridges are in use, under construction, or planned to be constructed in these regions to go across wide rivers, grand valleys, and even straits [1-4]. From the infrastructure point of view, a bridge is usually the vital point of a railway segment [3, 5], especially for the long-span bridge. Relative to other infrastructure scenarios, such as the flat ground and embankment, the bridge scenario is featured by smaller deck-stiffness, which is susceptible to crosswinds [5]. From the rolling stock point of view, the three major features,i.e. large slenderness ratio (length to height ratio), light weightiness, and high running speed, together entail the high-speed train being very sensitive to crosswinds [6-17]. Owing to the bridge and the train both suffering seriously from crosswinds, the serviceability and running safety of the train-bridge system are of major concern in China.
To assess the serviceability and running safety of the train-bridge system in crosswinds, several risk-analysis frameworks have been developed by previous researches [18-35]. Generally, a crosswind risk-analysis framework is consisted of two components, i.e., wind loads and dynamic models. As pointed out by BAKER [23], the levels of complexity and uncertainty involved in the above two components are inconsistent with each other. In other words, the uncertainty chain of the crosswind risk-analysis framework is originated mainly from the aerodynamic assessment.
In crosswinds, the aerodynamics of the train-bridge system can be categized into three aspects, i.e., static wind loads, buffeting loads, and self-excited loads. The static wind loads are major due to the mean component of the oncoming flow, which experiences significant effects of the aerodynamic interference between the train and the bridge [1, 21, 22, 36]. The buffeting loads are caused basically by the fluctuating component of the oncoming flow [37] and by vortex shedding from the train and the bridge bodies [1]. Moreover, the turbulence induced by the aerodynamic interference between the train and the bridge also plays a very important role in the buffeting loads [1]. The self-excited loads are originated from the interaction between flow and elastic structure. Because the self-excited loads on the train are limited, as pointed out by LI et al [21], they are neglected usually in the risk-analysis [22, 29]. However, the self-excited loads of the long-span bridge deck are indispensable. At the current juncture, the static wind loads tested on the train model on ground scenarios (flat ground and embankment) by static model wind tunnel test still have errors in the level of 20% [12, 37-41]. Let alone the static wind loads, buffeting loads, and self-excited loads of the train-bridge system. Thus, further validations and improvements in the aerodynamic assessment are required urgently.
Motivated by the above requirements both in reality and theory, several improvements carried out by Wind Tunnel Laboratory of Central South University are introduced, which can be classified roughly into five aspects as follows:
1) Based on many previous studies, flow around the train and uncertainty origin of the aerodynamic assessment by wind tunnel test were revealed from the fluid mechanism point of view.
2) A new TRAIN-INFRASTRUCTURE rig with the maximum launch speed of 35 m/s was developed. Effects of the train slipstream on the aerodynamic interference between the train and the bridge-deck will be highlighted in near future.
3) The characterized geometry parameters of the currently utilized bridge-decks in long-span railway bridges were counted. Based on these statistic results, the effects of geometry parameters on the aerodynamics of the flat-box/truss bridge-decks were investigated, which could be served as benchmarks to deepen the aerodynamic interference between the train and flat box/truss bridge-decks. More importantly, these studies can provide important information in the initial design stage of a new long-span railway bridge.
4) The aerodynamic interference between the train and the flat box/truss bridge-decks was well studied via static model test. Five underlying mechanisms were summarized. Moreover, these mechanisms were verified by moving model tests.
5) Effects of the wind-resistant protective measures on the aerodynamics of the train- infrastructure system were studied. Based on previous studies, a novel and efficient wind barrier, namely the louver-type wind barrier, was designed. When this kind of wind barrier is mounted on a bridge-deck, both the velocity and orientation of the approaching flow of the train and of the near wake of the bridge-deck can be adjusted. The wind loads on the bi-component of the train-bridge system can be well balanced.
2 Flow around train on/through scenarios of different types
This section describes the flow around the train on/through infrastructure scenarios of different types in crosswinds from the fluid mechanism point of view.
In crosswinds, the flow is assumed normal to the track [7]. Figure 1 sketches the flow around the train after several previous studies [11, 17, 42-45].
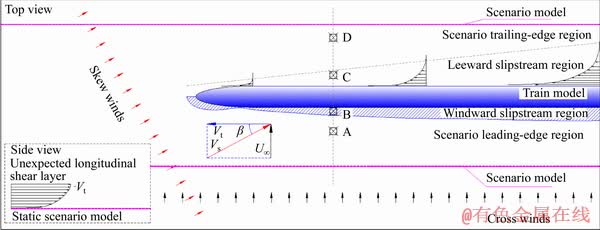
Figure 1 Flow around train
Due to the motion of the train, yaw angles (the angle between a structure centerline and its horizontal approaching flow) of the train and of the infrastructure scenario are not consisted with each other, namely, the yaw angle of the infrastructure scenario is β=90°, whereas the train experiences an effective yaw angle β=arctan(U∞/Vt) (Figure 1), which is far less than β=90°, as established already by a couple of researchers [7, 12, 41, 46]. The above inconsistency leads to a plenty of difficulties in the aerodynamic assessment process.
As shown in Figure 1, the flow around the train is categorized into four regions, i.e., scenario leading-edge region, windward slipstream region, leeward slipstream region, and scenario trailing- edge region. Due to the symmetry of the train model, only half slipstream is sketched in Figure 1. As discussed by many previous studies [17, 43, 45, 47, 48], the slipstream shall have an obvious boundary. For a train running on the flat ground scenario without crosswinds, points A and D in the scenario leading- and trailing-edge regions are out of the slipstream boundary layer. They are not subjected to the train slipstream, while points B and C in the windward and leeward slipstream regions suffer seriously from the train slipstream. The effects of this slipstream on people and overhead structures have been well studied by GAWTHORPE [49] and BAKER [25, 26]. In practice, train usually travels in crosswinds. The above-mentioned windward slipstream shall be pushed towards the train upstream surface by the lateral approaching flow, resulting in a thinner slipstream boundary layer, while the leeward slipstream shall be changed substantially by the shedding vortices from the roof and bottom of the train. Several previous studies have already shown that the slipstream is very complex but still has a boundary in this situation [17, 43, 45]. For windward slipstream, the boundary may be still clear. But for the leeward slipstream, the boundary may be indescribable. For clarifying representation, the authors utilize a grey dash line to just donate the boundary of the leeward slipstream.
When the train is running on other scenarios, such as embankment, viaduct, and long-span bridge, the effects on the train caused by these scenarios should be taken into account, such as the well-known overspeed effect on the top of the embankment observed by BAKER [50] and the quasi-Reynold number effect on the train shoulder resulted from the speed-up turbulent flow separated from the leading-edge of flat box/truss bridge-decks [1]. In this circumstance, point A suffers only from the leading-edge shear layer of the scenario; point B bears both the leading-edge shear layer of the scenario and the slipstream; point C is subjected to an interaction between the slipstream and shedding vortices from the train; and point D experiences both shedding vortices and the trailing-edge shear layer of the scenario.
Based on the above discussion, the aerodynamic interference between the train and scenarios of different types mainly involves three components, i.e., the crosswinds, the slipstream, and their coupling effect. If one wants to get an in-deep understanding of the mechanisms of the flow around the train on/through scenarios of different types, several benchmark studies should be carried out in advance with the aim of separating the above three components. For example, a fundamental investigation on a stationary train on a bridge-deck in crosswinds. A comprehensive understanding of the above physical features would play an important role in improving the resolution of the current aerodynamic assessment [43]. Even though the effects of embankment, viaduct, and long-span bridge on the aerodynamics of the train have already been revealed by BOCCIOLONE et al [37], CHELI et al [5], and LI et al [1], further studies are still needed, especially for the effects of the train on wind-sensitive long-span bridge deck.
3 Assessment methods
So far, there are three approaches to assess the aerodynamics of the train-infrastructure system, i.e., full-scale measurement, computational fluid dynamic (CFD) simulation, and wind tunnel test, which are described systematically in this section. The new-developed TRAIN-INFRASTRUCTURE rig is also introduced herein.
3.1 Full scale measurement
The full-scale measurement is carried out on full-scale train in natural wind field to provide the real data, which can be served as the calibration and verification for all other tests. In the past few decades, there are a number of full-scale measurements, i.e., the Pendine experiment performed on the Advanced Passenger Train by COOPER [51], the TRANSAERO experiment carried on the Inter-Reggio train by MATSCHKE and HEINE [52], the recent experiment on the Class 43 New measurement train [53], and the experiments on the aerodynamics of the train in tunnel [54-56]. Due to the uncertainty of the natural wind, the full-scale measurement is very different to be carried out. Moreover, multiple test cases and the “ensemble average” technique [57] are required to gain a satisfactory experiment result, which leads the full-scale measurement to be an expensive and complex undertaking [46].
3.2 Computational fluid dynamic (CFD) simulation
The CFD simulation is a promising method to assess both the aerodynamic forces and the flow field of the train-infrastructure system. In crosswinds, the slipstream boundary layer of the train is thick enough, thus DES and LES turbulence models are widely being utilized in the recent studies [58-60]. Using the above two turbulence models, the nose length effects on the aerodynamics of the train in large yaw angle and on its slipstream in small yaw angle are well studied [59, 60]. The influences caused by other factors, such as the coupler in double-unit train, the moving flat ground, the rotating wheels, the embankment are also investigated [41, 61-63]. However, due to the large slenderness ratio (length to height ratio) and irregular geometric shape of the lead and tail train, high resolution grid is required to capture predominate flow structures to reproduce the real aerodynamic behaviors of the train. Generally, the chief challenge of the CFD is the huge number of computational grids [7, 25]. Moreover, the CFD results always need the results of other methods for validation.
3.3 Wind tunnel test
3.3.1 Static model test
So far, the static model test is the most widely used to acquire the crosswind aerodynamics of the train-infrastructure system. As shown in Figure 1, the train and infrastructure scenario models are usually fixed together in the skew winds within β=[0°, 90°] [11, 12, 37, 39, 41, 43, 46, 51, 58, 64-66]. The skew winds may be smooth flow [9] or atmosphere boundary layer [37]. Both steady and unsteady aerodynamic forces can be tested, and a basic experimental results database has been established accumulatively. For the train, the lateral and longitudinal components of the skew winds can well simulate its motion and the crosswinds, respectively. However, the skew winds cannot reproduce the real crosswinds for the non-moving scenario, despite several remedies having been developed [11, 12, 67, 68]. The non-moving scenario and the longitudinal components of the skew winds together result in an unexpected longitudinal shear layer, as highlighted in a side view subfigure in Figure 1. Such unexpected longitudinal shear layer is the origin of the forgoing mentioned aerodynamic assessment uncertainty. For scenarios of different types, the effects of the unexpected longitudinal shear layer are distinct.
For the flat ground scenario, the unexpected longitudinal shear layer would be thin in smooth skew winds. Due to the gap between the train and the ground is 10%-15% of the train height [1], the effects of the unexpected longitudinal shear layer on the lead car may be limited, whereas such effects on the interior and tail cars shall be non-ignorable [7]. In order to diminish the effects of the unexpected longitudinal shear layer, the thickness of the boundary layer of the skew winds at where the train model is fixed shall be less than 30% of the train height, as documented in CEN [9]. The enough thin boundary layer of the skew winds should be the chief reason that the static model results show a good agreement with those of the moving model tests on side force, lift force, and rolling moment [37, 39, 51]. Moreover, as pointed out by DORRIGATTI et al [39], PREMOLI et al [41], and ZHANG et al [62], the major discrepancy between the moving and static experiments is focused on the underbody flow of the train. This observation can also be explained reasonably by the unexpected longitudinal shear layer.
For the embankment scenario, the boundary layer of this scenario shall be much thicker than that of the flat ground in skew winds, as evidenced by the embankment overspeed effect mentioned before [41, 46, 50]. Thus, the effects of the unexpected longitudinal shear layer shall be more serious. The flow around (Figure 1) the train shall be changed substantially. By flow visualization, SCHETZ [46] found out a strong but real-world- non-exist vortex, which alters dramatically the aerodynamics of the train and embankment system, along the leeward embankment. Note that the embankment model in SCHOBER et al [69] has an end layout. Based on an experimental study of a train on three typical embankment models, i.e., infinite model (wall-to-wall), finite models with and without end layout, TOMASINI et al [12] summarized that the embankment end layout plays an important role in determining the aerodynamics of the train. The above two investigations together suggest that the unexpected longitudinal shear layer has a sensibly negative effect on the aerodynamic assessment of the train on the embankment scenario, which results in a pseudo approaching flow to the train.
For the viaduct and long-span bridge scenarios, the effects of the unexpected shear layer on the train and upper-half scenario model are similar to those of the embankment. Besides, the flow on the lower- half scenario model is also changed completely by the unexpected shear layer. Relative to the flat ground, embankment, and viaduct scenarios, the long-span bridge scenario itself is very sensitive to the crosswinds for its smaller deck-stiffness. Moreover, all the wind loads of the train-bridge system are carried by the bridge-deck scenario, and the wind loads on a bridge-deck scenario would be underestimated in skew winds. Based on the above consensuses, almost all the studies on the aerodynamic assessment of the train-bridge system by static model test are performed only at β=90° [1, 65, 70-75]. In this circumstance, the effects of the unexpected longitudinal shear layer both on the train and on the long-span bridge can be eliminated. However, the aerodynamic forces of the train shall be overestimated by this method. But for an interior car, the above test results can be scaled by a factor sin2β to estimate the aerodynamic forces at a certain yaw angle β [43]. Indeed, this method has several shortcomings. For example, the sin2β scaled law shall be invalid for the lead and tail cars. But at this juncture, it is acceptable for practical considerations as it is widely utilized to examine the dynamics of the long-span bridge-train system in crosswinds [21, 22, 29, 76]. As discussed in Section 2, the train-bridge system tested results only at β=90° can reveal the aerodynamics in crosswinds without the interference of the slipstream and of the coupling effect of the slipstream and crosswinds. Compared with the moving model test, these studies could be served as a benchmark to deepen the understanding of the physical natures of the long-span bridge-train system in crosswinds.
3.3.2 Moving model test
The moving model test is an effective way to study the aerodynamics of the train, which can overcome the aforementioned inconsistency of the yaw angles of the bicomponent of the train-infrastructure system. Some innovative test systems have been developed, such as the TRAIN rig system [39, 53, 57], the synchronous driving device by LI et al [36], the conveyor belt driving device by XIANG et al [72, 73], the sliding devices developed by LI et al [77], and the gravity launching ramp by BOCCIOLONE et al [37]. However, numerous practical and technical difficulties remain unsolved in the moving test [39], which can be summarized into four aspects: vibration of the moving train model, instability of the running speed of the train model, short sampling duration, and nonconformity of the train-to-wind speed ratio between the model and the real world due to the limitation on the running speed of the train model. Therefore, the testing results of the moving train experiments have some limitations and sources of uncertainties, as documented in CEN [9].
For the sake of addressing several difficulties listed above, a new TRAIN-INFRASTRUCTURE rig system was developed by Wind Tunnel Laboratory of Central South University, as shown in Figure 2. The maximum launch speed of the train model is 35 m/s, which is larger than most of the current moving model devices. Because the wind tunnel is 12 m in width, the minimum sampling duration is about 0.34 s. The predominant features of the aerodynamics of the train-infrastructure system can still be detected. Compared with the results of the static model test, the effects of the train slipstream on the aerodynamic interference between the train and the bridge-deck will be highlighted in near future, as described in Section 2.
4 Aerodynamic characteristics
This section is focused on the aerodynamic characteristics of the train-bridge system. For the sake of highlighting the underlying mechanisms of the aerodynamic interference between the train and the bridge, several benchmark studies, i.e., the aerodynamics of the train-only and bridge-only models, are described in Sections 4.1 and 4.2, respectively. Finally, the recent core findings of the aerodynamic interference of the train-bridge system are summarized in Sections 4.3.
4.1 Aerodynamics of train
The flow around a train on the flat ground scenario experiences significant yaw angle effect [7, 43, 46, 78], as shown in Figures 3(a)-(c). With an increase in β=[0°, 90°], the flow around the train transits from steady slender body flow to unsteady vortex shedding flow. Within small yaw angle range of β=[0°, 40°-50°], a system of the longitudinal vortices dominates the leeward side of the train resembling the flow around a missile [7], which results in an almost sinusoidal variation of the aerodynamic forces of the lead vehicle, as shown in Figures 3(d), (e) and (f). In this range of β, the effects of the lead vehicle’s geometry are limited, as witnessed by the almost coincident curves of the aerodynamic coefficients of three different trains.
Within large yaw angle range of β=[60°-80°, 90°], the flow around the train likes that of a square cylinder with around corners, excepted for a very small region near the lead and tail noses [43, 58]. In this circumstance, the aerodynamic forces of the lead vehicle display a geometry-dependent behavior, especially for the lift coefficient as shown in Figure 3(e). Within moderate yaw angle range of β=[40°-50°, 60°-80°], the above two flow patterns can be detected concurrently at an intermediate distance from the train’s lead nose [7, 8, 58, 78]. while the above flow transition issue has limited effect on the aerodynamic forces of the interior vehicle, as depicted in Figure 4. Generally, the aerodynamic force curves in Figure 4 behave in a sin2β law which is unsensitive to the variation of the scenario. That’s the reason why this core finding by CHIU and SQUIRE [43] is utilized widely in the dynamic analysis of the train-bridge system, as described in Section 3.3.2.

Figure 2 TRAIN-INFRASTRUCTURE rig system of Wind Tunnel Laboratory of Central South University
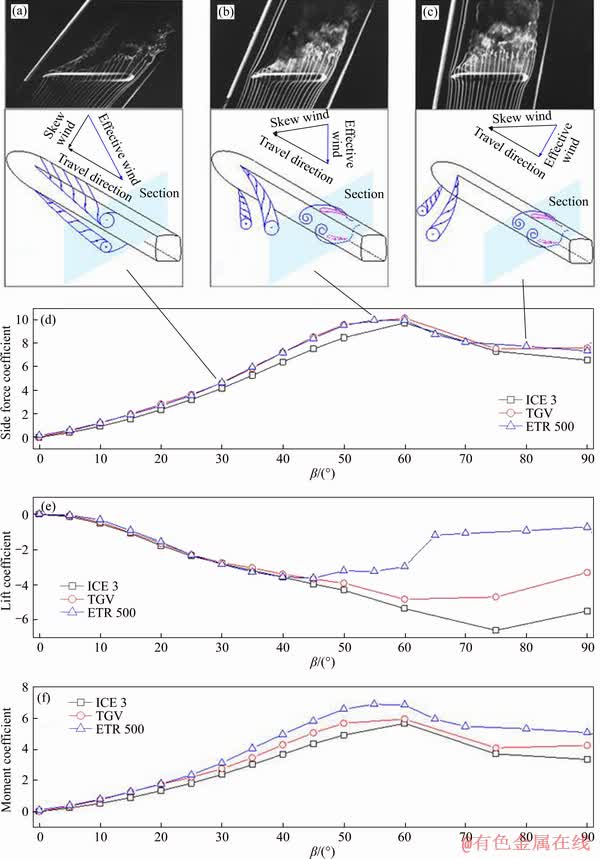
Figure 3 Aerodynamics of lead vehicle on flat ground in uniform flow (Smoke-wire visualization results and aerodynamic coefficients are taken from CHIU and SQUIRE [43] and CEN [9], respectively)
On the other hand, when a train runs on an elastic long-span bridge, its approaching flow may have an incidence, namely, the wind angle of attack α. In bridge engineering, the most concern range of α is [-12°, 12°]. If taken the effect of the bridge- deck into consideration, the α of the train would be even larger. Thus, the authors carried out an investigation to find out the aerodynamics of a 2D bluff body with the cross-section of a train at incidence. With a variation in α=[-20°, 20°], four typical flow patterns as shown in Figure 5 are identified. When the flow around the train is dominated by a full reattachment either on the upper or lower half train, the aerodynamics is characterized by an alteration between large- and small-amplitude fluctuations with a frequency about 1/10 of the predominant vortex shedding. This alteration phenomenon has a strong negative effect on the span-wise correlation of the aerodynamics.
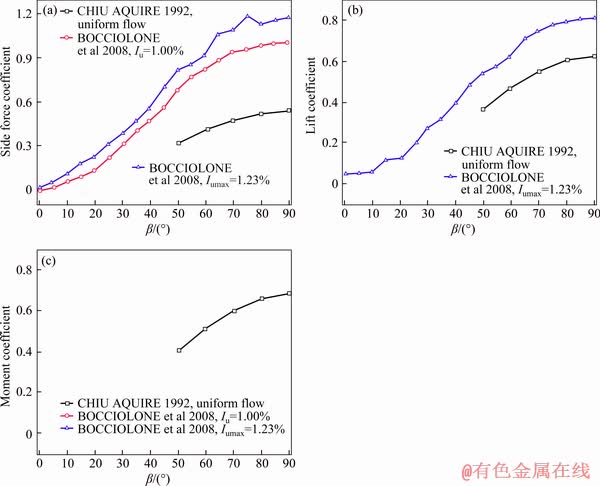
Figure 4 Mean aerodynamic forces of interior train on flat ground/viaduct scenarios in uniform/low turbulence flows (Aerodynamic coefficients are taken from CHIU and SQUIRE [43] and BOCCIOLONE et al [37])
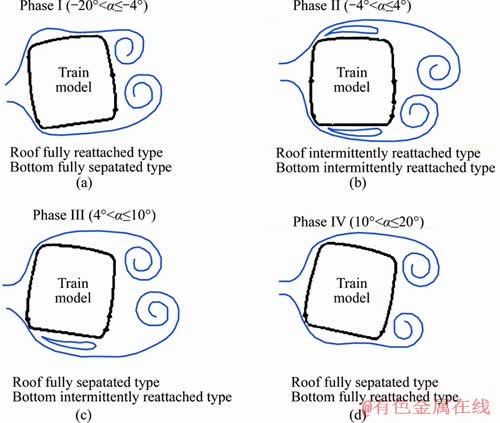
Figure 5 Flow around a 2D bluff body with cross section of a train at incidence
As the two upper shoulders of almost all the trains are smoothed by round corners with radius ratio r/d=0.10-0.15 (r and d are the corner radius and characteristic height of the train normal to the oncoming flow, respectively), the Reynolds number effects were also examined by the authors. Experimental results demonstrate that the aerodynamics of the train experiences significant Reynolds number effects resembling a prism with rounded-corners, as shown in Figure 6. The critical Reynolds number, corresponding to the drag crisis and the transition from subcritical to critical regimes, is around 1.56×105. The above findings would be conducive to reveal the ground effects mentioned by CHIU and SQUIRE [43], SCHETZ [46], and CHOI et al [78].
4.2 Aerodynamics of bridge-deck
Considering the protection of arable lands, the demand of economic development, and the rapid construction of new infrastructure, Chinese railway is characterized by a relatively high-percentage of bridges in the total mileage [83]. Because the small- and medium-span railway bridges are insensitive to crosswinds, the corresponding studies are focused mainly on their effects on the train, such as BOCCIOLONE et al [37] and HE et al [75]. However, the aerodynamics of the long-span railway bridge itself is of concern in crosswinds. In railway engineering, the most widely utilized bridge-decks in the long-span bridge are flat box/truss bridge-decks, especially in China [3, 83]. Thus, this section exhibits chiefly the aerodynamics of the above two kinds of railway bridge decks.
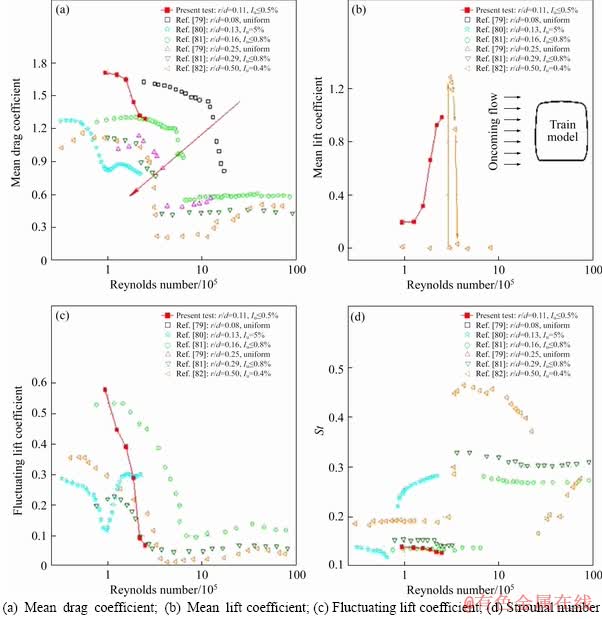
Figure 6 Variation of aerodynamic properties of train with Re at α=0°:(This figure is redrawn from LI et al [1])
Starting from Ganjiang and Oujiang Railway Bridges both with the main span of 300 m built in 2018, the flat box bridge-deck has been used widely in Chinese high-speed railway, such as Lingang Yangtze River Bridge, Bianyuzhou Bridge, Quanzhou Bay Bridge, Taoyaomen Railway Bridge and Xiangjiaomen Railway Bridge. For the sake of gaining universal aerodynamic characteristics of this kind of bridge-deck, the non-dimensionalized geometry parameters used in reality are depicted in HE et al [83] together with several highway bridges. Generally, the aspect ratio B/D (width to height ratio), wind fairing angle θ, and relative height of the wind fairing nose H/h (h and H are the distances from the wind fairing nose to the upper and lower surfaces of the bridge-deck, respectively) range from 5 to 18, 40° to 80°, and 1 to 4, respectively. Based on the above statistic results, the aerodynamics of 22 flat box bridge-decks with B/D=6, 7, 9 and 12, θ=45°, 55°, 65° and 75°, and H/h=1, 2, 3 and 4 were examined in a wind tunnel. Experimental results show that stall is an inherent characteristic of the flat box bridge-deck resembling the airfoil, and the minimum stall angle of all the 22 flat box bridge-decks is α=-2°. These observations suggest that the stall phenomenon of the flat box bridge-deck shall be paid close attention to. It is well known that stall is a very dangerous behavior for the airfoil, which is characterized by an abrupt-decease lift and by a rapid-increase drag. Numerous studies show that the stall of the airfoil is caused by large scale flow separation [84, 85]. But for the flat box bridge-deck, the stall is due to the impinging leading-edge vortices (vortices generated from the unstable leading-edge shear layer are transported by the boundary layer along the lateral surface of the bridge-deck producing regular or irregular vortex street), which was first named by NAUDASCHER and WANG [86]. After that, it is utilized frequently by DENIZ and STAUBLI [87], MANNINI et al [88, 89], PAIDOUSSIS et al [90], and TAYLOR et al [91]. Similar to the airfoil, the stall is also unfavorite to the stability of the flat box bridge-deck, which results in three dramatically enhanced fluctuation aerodynamic forces [83].
HE et al [83] also revealed that there were three typical flow patterns, i.e., trailing-edge vortex shedding (TEVS), impinging leading-edge vortices (ILEV), and alternate-edge vortex shedding (AEVS), around the flat box bridge-deck with an increase in α=[-12°, 12°], as shown in Figure 7. In TEVS and AEVS flow patterns regime, two St numbers can be identified from the lift power spectrum density, as shown in Figure 8. Proper orthogonal decomposition (POD) analysis presents that the almost constant St-c is attributed to vortices shedding from the leading-edge of the deck, whereas the variable St-v is due to vortices shedding from the trailing-edge. On the other hand, only one St number can be detected when the bridge-deck is dominated by the ILEV flow pattern. Similar to the study results of ITO et al [92], the ILEV and AEVS flow patterns without steady flow-reattachment correspond a strong spanwise coherence of the deck aerodynamics, while the TEVS flow pattern with steady flow-reattachment results in a weak spanwise coherence.
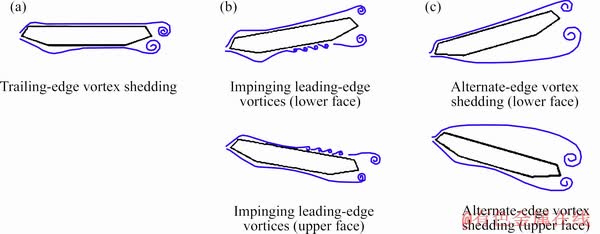
Figure 7 Flow patterns around flat box bridge-deck with an increase in |α|
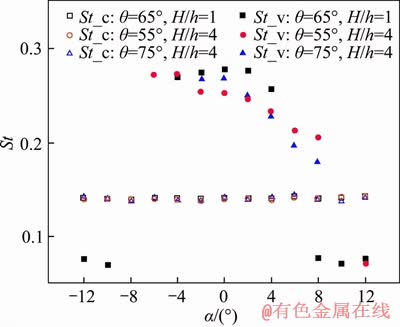
Figure 8 St number of flat box bridge-decks with B/D=9 (This figure is taken from HE et al [79])
Truss is another frequently encountered bridge-deck in Chinese high-speed railway, which has numerous members and various construction forms. Thus, to quantify the aerodynamics of such bridge-girder is quite difficult. From the wind engineering point of view, the authors utilize three parameters, i.e., aspect ratio B/D, solidity ratio Φ (the ratio of projected to envelope areas of the planar truss), and truss type (Warren and Pratt) to describe the predominate geometric features of the truss bridge-girder. Generally, the ranges of B/D and Φ used in engineering are 1.0-2.2 and 0.2-0.4, respectively. Wind tunnel tests show that the mean aerodynamic forces of the truss bridge-girder are B/D- and Φ-dependent but are insensitive to the truss type.
4.3 Aerodynamic interference between train and bridge-deck
This section highlights the recent investigations on the aerodynamic interference between the train and the bridge-deck in crosswinds via static and moving model tests.
4.3.1 Static model test
By static model test, the above aerodynamic interference was well studied by LI et al [1] and HE et al [75] on train-flat box deck, train-box deck, and train-truss girder systems, as shown in Figure 9. It is manifested primarily by five basic mechanisms: 1) flow featuring quasi-Reynolds number effect happening near the upstream shoulder of the train, 2) suppressing effect on the underbody vortex- shedding of the train, 3) shielding effect of the deck leading-edge and upstream truss on the train, 4) promoting effect on the flow transition of the bridge-deck, and 5) intensifying effect on the trailing-edge vortex-shedding of the bridge-deck.
1) Flow featuring quasi-Reynolds number effect
When the train is on the downstream track of a flat box bridge-deck, its aerodynamics experiences a flow featuring quasi-Reynolds number effect depending on the attack angle and velocity of the oncoming flow [1]. When α increases beyond zero, the mean drag and lift coefficients of the train begin to decrease or increase rapidly associated with three enhanced fluctuating aerodynamic forces at Re=1.25×105 (based on the free oncoming flow velocity U∞ and the train height d), as described by Figure 10. This observation is attributed to the variation in the flow regime around the train from subcritical to critical although the approaching flow Re remains unchanged. The separated shear flow from the leading-edge of the bridge-deck impinges on the upstream shoulder of the train on the downstream track at a critical orientation of the approach flow, as shown in Figure 11. The resulting accelerated flow in the shear layer, characterized by higher turbulence intensity, prompts the flow regime transition near the upstream shoulder of the train without a change in the inflow Re. This change in the pressure field over the upstream shoulder of the train when it is on the downstream track on the bridge-deck bears strong similarity to the changes experienced by circular cross-sections or cross- sections with rounded corners with a change in the inflow Reynolds number. The similar phenomenon was also observed by the authors on the same train but inside a truss bridge-deck.
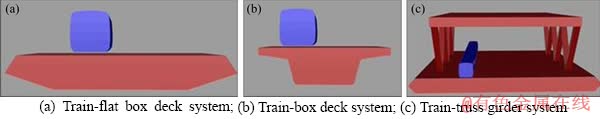
Figure 9 Most encountered train-bridge systems in China High-speed Railway:
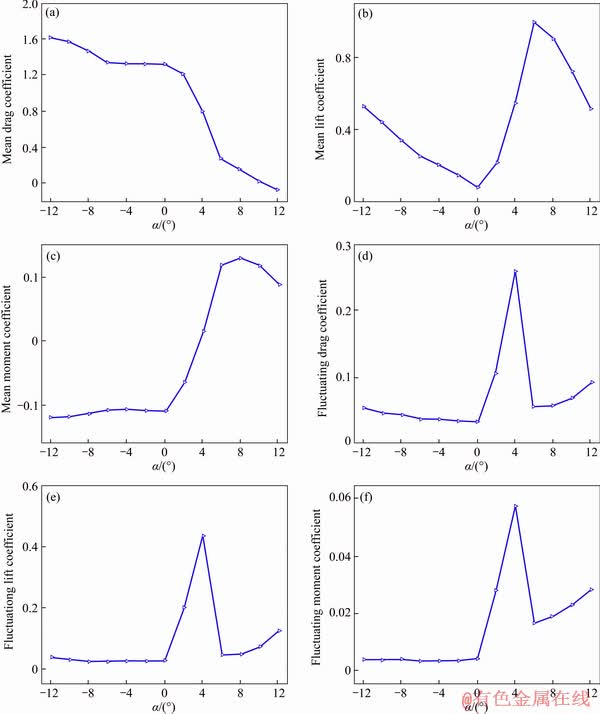
Figure 10 Aerodynamic force coefficients of train on downstream track of flat box bridge-deck
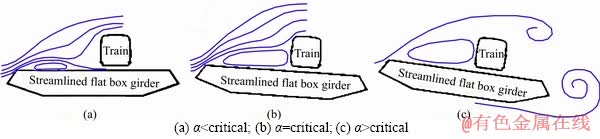
Figure 11 Flow patterns around train model on downstream track of a flat box bridge-deck:(This figure is redrawn from LI et al [1])
2) Suppressing effect on the underbody vortex-shedding of the train
The lower half train model resembles that of a square cylinder. Without the infrastructure scenario, its underbody flow is characterized by strong flow separation-reattachment, as shown in Figure 5. However, such underbody flow is suppressed substantially in the presence of the bridge-deck, which results in three reduced fluctuation forces.
3) Shielding effect of the deck leading-edge and upstream truss on the train
With an increase in α, the train on the flat box bridge-deck is submerged gradually by its leading- edge separated flow, as shown in Figure 11. If the α is large enough, the leading-edge separated flow shall have a shielding effect on the train. This shielding effect leads to a small drag to the train together with a weaken spanwise coherence due to the high-level turbulence intensity of the leading-edge separated flow. Obviously, this shielding effect is more noticeable for a train inside a truss bridge-deck owing to the upstream planer truss. Moreover, with an increase in the solidity ratio Φ of the upstream planer truss, this shielding effect intensifies significantly.
4) Promoting effect on the flow transition of the bridge-deck
In the presence of the train, the global centerline of the aerodynamics of the flat box bridge-deck is shifted parallel about 4° to the positive direction of α. The results of the pressure field, smoke-wire visualization, and near wake flow profile together assume that the above parallel shift of the aerodynamics centerline is due to the bridge-deck flow transition from TEVS to ILVS, and even to LEVS occurring at smaller critical angles of attack relative to that of the bridge-deck-only case. In other words, the presence of the train has a promoting effect on flow transition of the bridge-deck.
5) Intensifying effect on the trailing-edge vortex-shedding of the bridge-deck
Due to the additional vortices shedding from the roof of the upstream or downstream train, the bridge-deck trailing-edge vortex shedding intensifies substantially, which brings about three enlarged fluctuation force coefficients, a widened near wake, and a strengthened spanwise correlation of the aerodynamics of the bridge-deck.
In addition, the aerodynamic interference on the buffeting forces of train on a truss bridge-deck was studied by MA et al [65]. Several empirical formulas are suggested for engineering applications.
4.3.2 Moving model test
The aerodynamics of the train-bridge system was also studied by ZOU et al [94] and HE and ZOU [95] on moving train models launched by a U-shape ramp, as shown in Figure 12. Experimental results show that the above-mentioned five effects, revealed by the static model test, can also be detected herein, especially for the shielding effect of the bridge-deck leading-edge. Moving model tests on the train-bridge system in crosswinds carried out in other wind tunnels [36, 77, 93] have also reached similar conclusions, even though they are expressed in a different way.
5 Wind barriers
In strong crosswind area, wind-resistant protective measures should be taken to ensure the running safety of the train. Currently, two approaches are utilized around the world. One is to restrict the instantaneous speed of the train based on the wind warning system along the track [96-98]. The other one is to install wind barrier. The later one is more popular for it can diminish effectively the slowdown and suspending events of the train operation [98-100]. In this section, recent studies on the conventional wind barriers and a novel wind barrier are introduced.

Figure 12 A U-shape launching ramp
5.1 Conventional wind barriers
Wind barrier can reduce significantly the wind load of a train running in its near wake [70, 71, 96, 100-104]. Generally, the shielding efficiency of the wind barrier highly depends on its height, porosity, and distance to the train. For infrastructure scenarios with enough stiffness, such as the flat ground and embankment, solid wind barrier is used widely [103]. However, the wind barrier also has negative effect on aeroelastic infrastructure scenarios (viaduct and long-span bridge). For example, the dynamic analyses carried out by ZHANG et al [99] and GUO et al [100] show that the deflection of the viaduct with wind barriers are enhanced obviously. For the sake of balancing the effects both on the train and the bridge, several porous wind barriers are adopted in reality.
5.2 Novel wind barrier
In the last decade, the above mentioned solid and porous wind barriers are well studied. However, the porosity and height of these conventional wind barriers cannot be adjusted based on the oncoming flow properties to reach a better protective effect for the train-bridge system after being installed. Motivated by this problem, a novel and efficient wind barrier was developed by Wind Tunnel Laboratory of Central South University, as shown in Figure 13. By rotating the wind blade of this kind of barrier, the velocity and orientation of the approaching flow of the train and of the near wake of the bridge-deck can be adjusted.
Effects of this novel wind barrier on the aerodynamics of the train-bridge system were examined by wind tunnel test and CFD [75, 98]. Upon compared to the conventional grid-type wind barrier with the same porosity, height, and distance to the train, the louver-type wind barrier can lead to larger decreases of the lift and moment coefficients for both the train and bridge-deck, as shown in Figure 14. The mechanism underlying this observation is orientation variation of the flows upstream the train and downstream the bridge-deck trailing-edge, the rotation angle of the wind barrier blade (Figure 13) and the rotation form (the rotation directions of the upstream and downstream wind barriers) were also discussed by HE et al [75].
The wind load on the louver-type wind barrier itself is also important for the train-bridge system. The blade of this wind barrier is a kind of shallow bluff body, as shown in Figure 13. In large rotation angle, intense flow separation-reattachment will govern the aerodynamics this wind barrier, which is unfavorable to the stability of the whole system. Thus, a wind tunnel test with the aim of optimizing the parameters of the louver-type wind barrier was carried out by HE et al [102]. Specially, a sharp barrier blade is recommended.
6 Concluding remarks
This paper has described mainly the core findings on the aerodynamics of the high-speed train-bridge system in crosswinds by Wind Tunnel Laboratory of Central South University in the past five years. These original results will help the high-speed railway community to gain a deeper understanding of their research area and to trigger more advanced improvements.
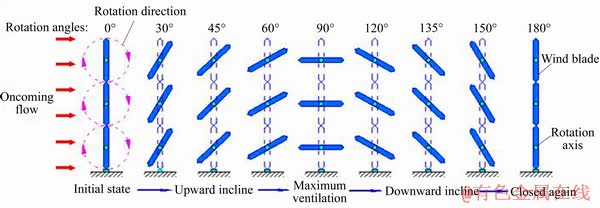
Figure 13 Louver-type wind barrier (This figure is taken from HE et al [102])
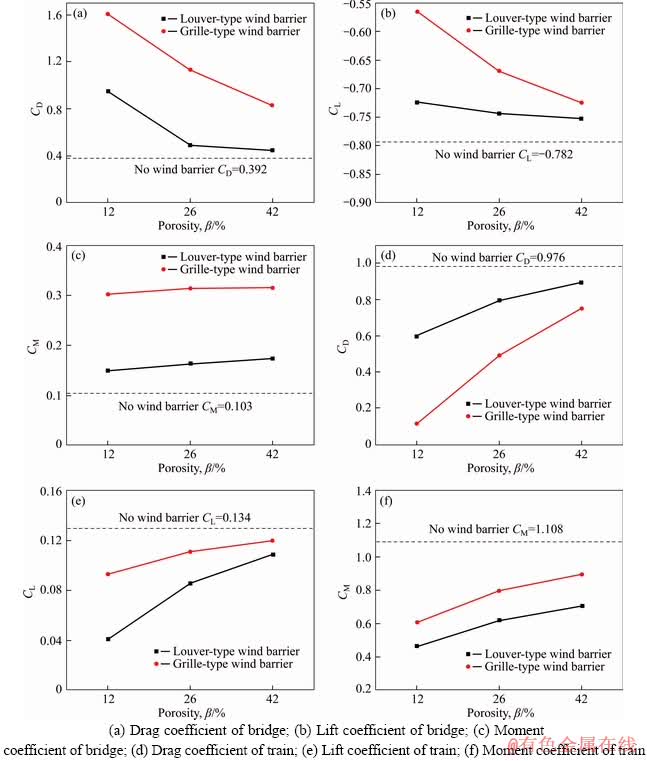
Figure 14 Comparison of aerodynamic coefficients of bridge and train between louver-type and grid-type wind barriers (This figure is taken from HE et al [75]):
References
[1] LI Huan, HE Xu-hui, WANG Han-feng, KAREEM A. Aerodynamics of a scale model of a high-speed train on a streamlined deck in cross winds [J]. Journal of Fluids and Structures, 2019, 91: 102717. DOI: https://doi.org/10.1016/ j.jfluidstructs.2019.102717.
[2] HU Nan, DAI Gong-lian, YAN Bin, LIU Ke. Recent development of design and construction of medium and long span high-speed railway bridges in China [J]. Engineering Structures, 2014, 74: 233-241. DOI: https://doi.org/ 10.1016/j.engstruct.2014.05.052.
[3] HE Xu-hui, WU Teng, ZOU Yun-feng, CHEN Frank Y., GUO Hui, YU Zhi-wu. Recent developments of high-speed railway bridges in China [J]. Structure and Infrastructure Engineering, 2017a, 13(12): 1584-1595. DOI: https:// doi.org/10.1080/15732479.2017.1304429.
[4] LI Yong-le, HU Peng, XU Xin-yu, QIU Jun-jie. Wind characteristics at bridge site in a deep-cutting gorge by wind tunnel test [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2017, 160: 30-46. DOI: https://doi.org/ 10.1016/j.jweia.2016.11.002.
[5] CHELI F, CORRADI R, ROCCHI D, MAESTRINI E. Wind tunnel tests on train scale models to investigate the effect of infrastructure scenario [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2010a, 98(6, 7): 353-362. DOI: https://doi.org/10.1016/j.jweia.2010.01.001.
[6] TSI, EC. Technical specification for interoperability of the trans-european high speed rail system. european law [S]. Official Journal of the European Communities, 2008.
[7] BAKER C. The flow around high speed trains [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2010a, 98(6, 7): 277-298. DOI: https://doi.org/10.1016/j.jweia.2009. 11.002.
[8] BAKER C. The simulation of unsteady aerodynamic cross wind forces on trains [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2010b, 98(2): 88-99. DOI: https:// doi.org/10.1016/j.jweia.2009.09.006.
[9] CEN. Railway applications–aerodynamics–part 6: Requirements and test procedures for cross wind assessment [S]. 2018.
[10] CHELI F, RIPAMONTI F, ROCCHI D, TOMASINI G. Aerodynamic behaviour investigation of the new EMUV250 train to cross wind [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2010b, 98(4, 5): 189-201. DOI: https://doi.org/10.1016/j.jweia.2009.10.015.
[11] NOGUCHI Y, SUZUKI M, BAKER C, NAKADE K. Numerical and experimental study on the aerodynamic force coefficients of railway vehicles on an embankment in crosswind [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2019, 184: 90-105. DOI: https://doi.org/ 10.1016/j.jweia.2018.11.019.
[12] TOMASINI G, GIAPPINO S, CORRADI R. Experimental investigation of the effects of embankment scenario on railway vehicle aerodynamic coefficients [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2014, 131: 59-71. DOI: https://doi.org/10.1016/j.jweia.2014.05.004.
[13] TIAN Hong-qi. Review of research on high-speed railway aerodynamics in China [J]. Transportation Safety and Environment, 2019, 1: 1-21. DOI: https://doi.org/10.1093/ tse/tdz014.
[14] LU Chun-fang. A discussion on technologies for improving the operational speed of high-speed railway networks [J]. Transportation Safety and Environment, 2019, 1: 22-36. DOI: https://doi.org/10.1093/tse/tdz003.
[15] TAO Yu, YANG Ming-zhi, QIAN B, WU Fan, WANG Tian-tian. Numerical and experimental study on ventilation panel models in a subway passenger compartment [J]. Engineering, 2019, 5(2): 329-336. DOI: https://doi.org/ 10.1016/j.eng.2018.12.007.
[16] WANG Tian-tian, WU Fan, YANG Ming-zhi, JI Peng, QIAN B. Reduction of pressure transients of high-speed train passing through a tunnel by cross-section increase [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2018, 183: 235-242. DOI: https://doi.org/10.1016/j.jweia. 2018.11.001.
[17] CHEN Zheng-wei, LIU Tang-hong, YAN Chun-guang, YU Miao, GUO Zi-jian, WANG Tian-tian. Numerical simulation and comparison of the slipstreams of trains with different nose lengths under crosswind [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2019, 190: 256-272. DOI: https://doi.org/10.1016/j.jweia.2019.05.005.
[18] BAKER C. Ground vehicles in high cross winds, part I: steady aerodynamic forces [J]. Journal of Fluids and Structures, 1991a, 5(1): 69-90. DOI: https://doi.org/10.1016/ 0889-9746(91)80012-3.
[19] BAKER C. Ground vehicles in high cross winds part II: unsteady aerodynamic forces [J]. Journal of Fluids and Structures, 1991b, 5(1): 91-111. DOI: https://doi.org/10. 1016/0889-9746(91)80013-4.
[20] BAKER C. Ground vehicles in high cross winds part III: The interaction of aerodynamic forces and the vehicle system [J]. Journal of Fluids and Structures, 1991c, 5(2): 221-241. DOI: https://doi.org/10.1016/0889-9746(91)90478-8.
[21] LI Yong-le, QIANG Shi-zhong, LIAO Hai-li, XU You-lin. Dynamics of wind–rail vehicle–bridge systems [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2005, 93(6): 483-507. DOI: https://doi.org/10.1016/j.jweia.2005. 04.001.
[22] XU You-lin, DING Quan-shun. Interaction of railway vehicles with track in cross-winds [J]. Journal of Fluids and Structures, 2006, 22(3): 295-314. DOI: https://doi.org/10. 1016/j.jfluidstructs.2005.11.003
[23] BAKER C. A framework for the consideration of the effects of crosswinds on trains [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2013, 123: 130-142. DOI: https://doi.org/10.1016/j.jweia.2013.09.015.
[24] BAKER C, CHELI F, ORELLANO A, PARADOT N, PROPPE C, ROCCHI D. Cross-wind effects on road and rail vehicles [J]. Vehicle System Dynamics, 2009, 47(8): 983-1022. DOI: https://doi.org/10.1080/004231109030787 94.
[25] BAKER C. A review of train aerodynamics Part 1–Fundamentals [J]. The Aeronautical Journal, 2014a, 118(1201): 201-228. DOI: https://doi.org/10.1017/S00019 2400000909X.
[26] BAKER C. A review of train aerodynamics, Part 2–Applications [J]. The Aeronautical Journal, 2014b, 118(1202): 345-382. DOI: https://doi.org/10.1017/S0001924 000009179.
[27] DING Y, STERLINGS M, BAKER C. An alternative approach to modelling train stability in high cross winds [J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2008, 222(1): 85-97. DOI: https://doi.org/10.1243/09544097JRRT138.
[28] GIAPPINO S, ROCCHI D, SCHITO P, TOMASINI G. Cross wind and rollover risk on lightweight railway vehicles [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2016, 153: 106-112. DOI: https://doi.org/10.1016/j.jweia. 2016.03.013.
[29] OLMOS J, ASTIZ M. Improvement of the lateral dynamic response of a high pier viaduct under turbulent wind during the high-speed train travel [J]. Engineering Structures, 2018, 165: 368-385. DOI: https://doi.org/10.1016/j.engstruct. 2018.03.054.
[30] YU Meng-ge, ZHANG Ji-ye, ZHANG Ke-yue, ZHANG Wei-hua. Crosswind stability analysis of a high-speed train based on fuzzy random reliability [J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2015, 229(8): 875-887. DOI: https://doi.org/10.1177/0954409714524548.
[31] XU You-lin, XIA He, YAN Quan-sheng. Dynamic response of suspension bridge to high wind and running train [J]. Journal of Bridge Engineering, 2003, 8: 46-55. DOI: https://doi.org/10.1061/(ASCE)1084-0702(2003)8:1(46).
[32] XU You-lin, ZHANG Nan, XIA He. Vibration of coupled train and cable-stayed bridge system in cross wind [J]. Journal of Engineering Structure, 2004, 26: 1389-1406. DOI: https://doi.org/10.1016/j.engstruct.2004.05.005.
[33] XU You-lin, GUO Wei-wei, CHEN Jun, SHUM K, XIA He. Dynamic response of suspension bridge to typhoon and trains. I: Field measurement results [J]. Journal of Structural Engineering, 2007, 133(1): 3-11. DOI: https://doi.org/10. 1061/(ASCE)0733-9445(2007)133:1(3).
[34] GUO Wei-wei, XU You-lin, XIA He, ZHANG Wen-shou, SHUM K. Dynamic response of suspension bridge to typhoon and trains. II: Numerical results [J]. Journal of Structural Engineering, 2007, 133(1): 12-21. DOI: https:// doi.org/10.1061/(ASCE)0733-9445(2007)133:1(12).
[35] XIA He, GUO Wei-wei, ZHANG Nan, SUN Guo-jun. Dynamic analysis of a train-bridge system under wind action [J]. Computer and Structures, 2008, 86: 1845-1855. DOI: https://doi.org/10.1016/j.compstruc.2008.04.007.
[36] LI Xiao-zhen, WANG Ming, XIAO Jun, ZOU Qi-yang, LIU De-jun. Experimental study on aerodynamic characteristics of high-speed train on a truss bridge: A moving model test [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2018, 179: 26-38. DOI: https://doi.org/10.1016/j.jweia.2018. 05.012.
[37] BOCCIOLONE M, CHELI F, CORRADI R, MUGGIASCA S, TOMASINI G. Crosswind action on rail vehicles: Wind tunnel experimental analyses [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2008, 96(5): 584-610. DOI: https://doi.org/10.1016/j.jweia.2008.02.030.
[38] CATANZARO C, CHELI F, ROCCHI D, SCHITO P, TOMASINI G. High-speed train crosswind analysis: CFD study and validation with wind-tunnel tests [C]// International Conference on Engineering Conferences International. Springer, 2010: 99-112. DOI: https:// doi.org/10.1007/978-3-319-20122-1_6.
[39] DORIGATTI F, STERLING M, BAKER C, QUINN A. Crosswind effects on the stability of a model passenger train—A comparison of static and moving experiments [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2015, 138: 36-51. DOI: https://doi.org/10.1016/j.jweia. 2014.11.009.
[40] KIKUCHI K, SUZUKI M. Study of aerodynamic coefficients used to estimate critical wind speed for vehicle overturning [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2015, 147: 1-17. DOI: https://doi.org/10. 1016/j.jweia.2015.09.003.
[41] PREMOLI A, ROCCHI D, SCHITO P, TOMASINI G. Comparison between steady and moving railway vehicles subjected to crosswind by CFD analysis [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2016, 156: 29-40. DOI: https://doi.org/10.1016/j.jweia.2016.07.006.
[42] COPLEY J. The three-dimensional flow around railway trains [J]. Journal of Wind Engineering and Industrial Aerodynamics, 1987, 26(1): 21-52. DOI: https://doi.org/10. 1016/0167-6105(87)90034-1.
[43] CHIU T, SQUIRE L. An experimental study of the flow over a train in a crosswind at large yaw angles up to 90° [J]. Journal of Wind Engineering and Industrial Aerodynamics, 1992, 45(1): 47-74. DOI: https://doi.org/10.1016/0167- 6105(92)90005-U.
[44] HEMIDA H, KRAJNOVIC S. Exploring flow structures around a simplified ICE2 train subjected to a 30 side wind using LES [J]. Engineering Applications of Computational Fluid Mechanics, 2009, 3(1): 28-41. DOI: https://doi.org/ 10.1080/19942060.2009.11015252.
[45] BELL J, BURTON D, THOMPSON M, HERBST A, SHERIDAN J. Wind tunnel analysis of the slipstream and wake of a high-speed train [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2014, 134: 122-138. DOI: https://doi.org/10.1016/j.jweia.2014.09.004.
[46] SCHETZ J. Aerodynamics of high-speed trains [J]. Annual Review of Fluid Mechanics, 2001, 33(1): 371-414. DOI: https://doi.org/10.1146/annurev.fluid.33.1.371.
[47] NIU Ji-qiang, ZHOU Dan, LIANG Xi-feng. Numerical investigation of the aerodynamic characteristics of high- speed trains of different lengths under crosswind with or without windbreaks [J]. Engineering Applications of Computational Fluid Mechanics, 2018, 12(1): 195-215. DOI: https://doi.org/10.1080/19942060.2017.1390786.
[48] XIA Chao, WANG Han-feng, BAO Di, YANG Zhi-yang. Unsteady flow structures in the wake of a high-speed train [J]. Experimental Thermal and Fluid Science, 2018, 98: 381-396. DOI: https://doi.org/10.1016/j.expthermflusci. 2018.06.010.
[49] GAWTHORPE R. Aerodynamics of trains in the open air [J]. Railway Engineer International, 1978, 3(3): 7-12. DOI: http://pascal-francis.inist.fr/vibad/index.php?action=getRecordDetail&idt=PASCAL7980168430.
[50] BAKER C. The determination of topographical exposure factors for railway embankments [J]. Journal of Wind Engineering and Industrial Aerodynamics, 1985, 21(1): 89-99. DOI: https://doi.org/10.1016/0167-6105(85)90035-2.
[51] COOPER R. The effect of cross-winds on trains [J]. Journal of Fluids Engineering, 1981, 103(1): 170-178. DOI: https://doi. org/10.1115/1.3240768.
[52] MATSCHKE G, HEINE C. Full scale tests on side wind effects on trains. Evaluation of aerodynamic coefficients and efficiency of wind breaking devices [C]// TRANSAERO-A European Initiative on Transient Aerodynamics for Railway System Optimisation. Berlin, Heidelberg: Springer, 2002: 27-38. DOI: https://doi.org/ 10.1007/978-3-540-45854-8_3.
[53] GALLAGHER M, MORDEN J, BAKER C, SOPER D, QUINN A, HEMIDA H, STERLING M. Trains in crosswinds–Comparison of full-scale on-train measurements, physical model tests and CFD calculations [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2018, 175: 428-444. DOI: https://doi.org/10.1016/j.jweia.2018.03.002.
[54] KO Y, CHEN C, HOE T, WANG S. Field measurements of aerodynamic pressures in tunnels induced by high speed trains [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2012, 100(1): 19-29. DOI: https://doi.org/ 10.1016/j.jweia.2011.10.008.
[55] SUZUKI M, IDO A, SAKUMA Y, KAJIYAMA H. Full-scale measurement and numerical simulation of flow around high-speed train in tunnel [J]. Journal of Mechanical Systems for Transportation and Logistics, 2008, 1(3): 281-292. DOI: https://doi.org/10.1299/jmtl.1.281.
[56] LIU Tang-hong, TIAN Hong-qi, LIANG Xi-feng. Aerodynamic effects caused by trains entering tunnels [J]. Journal of Transportation Engineering, 2010, 136(9): 846-853. DOI: https://doi.org/10.1061/(ASCE)TE.1943- 5436.0000146.
[57] BAKER C, DALLEY S, JOHNSON T, QUINN A, WRIGHT N. The slipstream and wake of a high-speed train [J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2001, 215(2): 83-99. DOI: https://doi.org/10.1243/0954409011531422.
[58] HEMIDA H, KRAJNOVIC S. LES study of the influence of a train-nose shape on the flow structures under cross-wind conditions [J]. Journal of Fluids Engineering, 2008, 130(9). DOI: https://doi.org/10.1115/1.2953228.
[59] MORDEN J, HEMIDA H, BAKER C. Comparison of RANS and detached eddy simulation results to wind-tunnel data for the surface pressures upon a class 43 high-speed train [J]. Journal of Fluids Engineering, 2015, 137(4): 041108. DOI: https://doi.org/10.1115/1.4029261.
[60] CHEN Zheng-wei, LIU Tang-hong, JIANG Zhen-hua, GUO Zi-jian, ZHANG Jie. Comparative analysis of the effect of different nose lengths on train aerodynamic performance under crosswind [J]. Journal of Fluids and Structures, 2018, 78: 69-85. DOI: https://doi.org/10.1016/j.jfluidstructs.2017. 12.016.
[61] NIU Ji-qiang, ZHOU Dan, LIU Tang-hong, LIANG Xi-feng. Numerical simulation of aerodynamic performance of a couple multiple units high-speed train [J]. Vehicle System Dynamics, 2017, 55(5): 681-703. DOI: https://doi.org/ 10.1080/00423114.2016.1277769.
[62] ZHANG Jie, LI Jing-juan, TIAN Hong-qi, GAO Guan-jun, SHERIDAN J. Impact of ground and wheel boundary conditions on numerical simulation of the high-speed train aerodynamic performance [J]. Journal of Fluids and Structures, 2016, 61: 249-261. DOI: https://doi.org/10.1016/ j.jfluidstructs.2015.10.006.
[63] GUO Zi-jian, LIU Tang-hong, YU Miao, CHEN Zheng-wei, LI Wen-hui, HUO Xiao-shuai, LIU Hong-kang. Numerical study for the aerodynamic performance of double unit train under crosswind [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2019, 191: 203-214. DOI: https://doi.org/10.1016/j.jweia.2019.06.014.
[64] KHIER W, BREUER M, DURST F. Flow structure around trains under side wind conditions: A numerical study [J]. Computers & Fluids, 2000, 29(2): 179-195. DOI: https://doi.org/10.1016/S0045-7930(99)00008-0.
[65] MA Cun-ming, DUAN Qing-song, LI Qiu-sheng, CHEN Ke-jian, LIAO Hai-li. Buffeting forces on static trains on a truss girder in turbulent crosswinds [J]. Journal of Bridge Engineering, 2018, 23(11): 04018086. DOI: https://doi.org/ 10.1061/(ASCE)BE.1943-5592.0001305.
[66] HE Xu-hui, ZOU Yun-feng, WANG Han-feng, HAN Yan, SHI Kang. Aerodynamic characteristics of a trailing rail vehicles on viaduct based on still wind tunnel experiments [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2014, 135: 22-33. DOI: https://doi.org/10. 1016/j.jweia.2014.10.004.
[67] KWON H, PARK Y, LEE D, KIM M. Wind tunnel experiments on Korean high-speed trains using various ground simulation techniques [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2001, 89(13): 1179-1195. DOI: https://doi.org/10.1016/S0167-6105(01) 00107-6.
[68] SUZUKI M, TANEMOTO K, MAEDA T. Aerodynamic characteristics of train/vehicles under cross winds [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2003, 91(1, 2): 209-218. DOI: https://doi.org/10.1016/ S0167-6105(02)00346-X.
[69] SCHOBER M, WEISE M, ORELLANO A, DEEG P, WETZEL W. Wind tunnel investigation of an ICE 3 endcar on three standard ground scenarios [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2010, 98(6, 7): 345-352. DOI: https://doi.org/10.1016/j.jweia.2009.12.004.
[70] BARCALA M, MESEGUER J. An experimental study of the influence of parapets on the aerodynamic loads under cross wind on a two-dimensional model of a railway vehicle on a bridge [J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2007, 221(4): 487-494. DOI: https://doi.org/10.1243/09544097 JRRT53.
[71] BARCALA M, MESEGUER J. Visualization study of the influence of parapets on the flow around a train vehicle under cross winds [J]. WIT Transactions on The Built Environment, 2008, 103: 797-806. DOI: https://doi.org/ 10.2495/CR080771.
[72] XIANG Huo-yue, LI Yong-le, LIAO Hai-li, LI Cui-juan. An adaptive surrogate model based on support vector regression and its application to the optimization of railway wind barriers [J]. Structural and Multidisciplinary Optimization, 2017, 55(2): 701-713. DOI: https://doi.org/10.1007/s00158- 016-1528-9.
[73] XIANG Huo-yue, LI Yong-le, WANG Bin. Aerodynamic interaction between static vehicles and wind barriers on railway bridges exposed to crosswinds [J]. Wind and Structures, 2015, 20(2): 237-247. DOI: https://doi.org/10. 12989/was.2015.20.2.237.
[74] OGUETA M, FRANCHINI S, ALONSO G. Effects of bird protection barriers on the aerodynamic and aeroelastic behaviour of high speed train bridges [J]. Engineering Structures, 2014, 81: 22-34. DOI: https://doi.org/10.1016/ j.engstruct.2014.09.035.
[75] HE Xu-hui, SHI Kang, WU Teng, ZOU Yun-feng, WANG Han-feng, QIN Hong-xi. Aerodynamic performance of a novel wind barrier for train-bridge system [J]. Wind and Structures, 2016, 23(3): 171-189. DOI: https://doi.org/ 10.12989/was.2016.23.3.171.
[76] SAYYAADI H, SHOKOUHI N. A new model in rail–vehicles dynamics considering nonlinear suspension components behavior [J]. International Journal of Mechanical Sciences, 2009, 51(3): 222-232. DOI: https://doi.org/ 10.1016/j.ijmecsci.2009.01.003.
[77] LI Yong-le, HU Peng, XU You-lin, ZHANG, Ming-jin, LIAO Hai-li. Wind loads on a moving vehicle-bridge deck system by wind-tunnel model test [J]. Wind and Structures, 2014, 19(2): 145-167. DOI: https://doi.org/10.12989/was. 2014.19.2.145.
[78] CHOI H, LEE J, PARK H. Aerodynamics of heavy vehicles [J]. Annual Review of Fluid Mechanics, 2014, 46: 441-468. DOI: https://doi.org/10.1146/annurev-fluid-011212-140616.
[79] POLHAMUSL E. C. Effect of flow incidence and Reynolds number on low-speed aerodynamic characteristics of several noncircular cylinders with applications to directional stability and spinning [R]. Technical Report Archive & Image Library, 1985. DOI: https://ntrs.nasa.gov/search.jsp?R=19930085064.
[80] CARASSALE L, FREDA A, MARRE-BRUNENGHI M. Experimental investigation on the aerodynamic behavior of square cylinders with rounded corners [J]. Journal of Fluids & Structures, 2014, 44(7): 195-204. DOI: https://doi.org/ 10.1016/j.jfluidstructs.2013.10.010
[81] HINSBERG N P, SCHEWE G, JACOBS M. Experiments on the aerodynamic behaviour of square cylinders with rounded corners at Reynolds numbers up to 12 million [J]. Journal of Fluids and Structures, 2017, 74: 214-233. DOI: https://doi.org/ 10.1016/j.jfluidstructs.2017.08.002
[82] SCHEWE G. On the force fluctuations acting on a circular cylinder in crossflow from subcritical up to transcritical Reynolds numbers [J]. Journal of Fluid Mechanics, 1983, 133: 265-285. DOI: https://doi.org/10.1017/ S0022112083001913
[83] HE Xu-hui, LI Huan, WANG Han-feng, FANG Dong-xu, LIU Meng-ting. Effects of geometrical parameters on the aerodynamic characteristics of a streamlined flat box bridge-deck [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2017, 170: 56-67. DOI: https://doi.org/ 10.1016/j.jweia.2017.08.009.
[84] ERICKSON G. High angle-of-attack aerodynamics [J]. Annual Review of Fluid Mechanics, 1995, 27(1): 45-88. DOI: https://doi.org/10.1146/annurev.fl.27.010195.000401.
[85] ERICSSON L, REDING J. Fluid mechanics of dynamic stall part I. Unsteady flow concepts [J]. Journal of Fluids and Structures, 1988, 2(1): 1-33. DOI: https://doi.org/10.1016/ S0889-9746(88)90116-8.
[86] NAUDASCHER E, WANG Yi-nan. Flow-induced vibrations of prismatic bodies and grids of prisms [J]. Journal of Fluids & Structures, 1993, 7(4): 341-373. DOI: https://doi.org/ 10.1006/jfls.1993.1021.
[87] DENIZ S, STAUBLI T. Oscillating rectangular and octagonal profiles: Interaction of leading-and trailing-edge vortex formation [J]. Journal of Fluids and Structures, 1997, 11(1): 3-31. DOI: https://doi.org/10.1006/jfls.1996.0065.
[88] MANNINI C, MARRA A, PIGOLOTTI L, BARTOLI G. The effects of free-stream turbulence and angle of attack on the aerodynamics of a cylinder with rectangular 5: 1 cross section [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2017, 161: 42-58. DOI: https://doi.org/ 10.1016/j.jweia.2016.12.001.
[89] MANNINI C, SODA A, SCHEWE G. Numerical investigation on the three-dimensional unsteady flow past a 5: 1 rectangular cylinder [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2011, 99(4): 469-482. DOI: https://doi.org/10.1016/j.jweia.2010.12.016.
[90] PAIDOUSSIS M, PRICE S, de LANGRE E. Fluid-structure interactions: Cross-flow-induced instabilities [M]. Cambridge: Cambridge University Press, 2010.
[91] TAYLOR Z, PALOMBI E, GURKA R, KOPP G. Features of the turbulent flow around symmetric elongated bluff bodies [J]. Journal of Fluids and Structures, 2011, 27(2): 250-265. DOI: https://doi.org/10.1016/j.jfluidstructs.2010.10.004.
[92] ITO Y, SHIRATO H, MATSUMOTO M. Coherence characteristics of fluctuating lift forces for rectangular shape with various fairing decks [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2014, 135: 34-45. DOI: https://doi.org/10.1016/j.jweia.2014.10.003.
[93] WANG Ming, LI Xiao-zhen, XIAO Jun, ZOU Qi-yang, SHA Hai-qing. An experimental analysis of the aerodynamic characteristics of a high-speed train on a bridge under crosswinds [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2018, 177: 92-100. DOI: https://doi.org/ 10.1016/j.jweia.2018.03.021.
[94] ZOU Yun-feng, HE Xu-hui, ZOU Si-min, HUANG Yong-ming, ZUO Tai-hui. A U-shape launching ramp and method for aerodynamic characteristic testing of running train-bridge system [J]. Journal of Railway Science and Engineering, 2018, 15: 1747-1753. DOI: https://doi.org/ 10.19713/j.cnki.43-1423/u.2018.07.016.
[95] HE Xu-hui, ZOU Si-min. Wind tunnel test on aerodynamic characteristics of high-speed train running on bridge under crosswind [C]// Word Transport Convention. Beijing, 2019: 13-16.
[96] FUJII T, MAEDA T, ISHIDA H, IMAI T, TANEMOTO K, SUZUKI M. Wind-induced accidents of train/vehicles and their measures in Japan [J]. Quarterly Report of RTRI, 1999, 40(1): 50-55. DOI: https://doi.org/10.2219/rtriqr.40.50.
[97] HOPPMANN U, KOENIG S, TIELKES T, MATSCHKE G. A short-term strong wind prediction model for railway application: design and verification [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2002, 90(10): 1127-1134. DOI: https://doi.org/10.1016/S0167-6105(02) 00226-X.
[98] IMAI T, FUJII T, TANEMOTO K, SHIMAMURA T, MAEDA T, ISHIDA H, HIBINO Y. New train regulation method based on wind direction and velocity of natural wind against strong winds [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2002, 90(12-15): 1601-1610. DOI: https://doi.org/10.1016/S0167-6105(02)00273-8.
[99] ZHANG Tian, XIA He, GUO Wei-wei. Analysis on running safety of train on bridge with wind barriers subjected to cross wind [J]. Wind Structures, 2013, 17(3): 203-225. DOI: https://doi.org/10.12989/was.2013.17.2.203.
[100] GUO Wei-wei, XIA He, KAROUMI R, ZHANG Tian, LI Xiao-zhen. Aerodynamic effect of wind barriers and running safety of trains on high-speed railway bridges under cross winds [J]. Wind Structures, 2015, 20(2): 213-236. DOI: https://doi.org/10.12989/was.2015.20.2.213.
[101] AVILA-SANCHEZ S, LOPEZ-GARCIA O, CUERVA A, MESEGUER J. Characterisation of cross-flow above a railway bridge equipped with solid windbreaks [J]. Engineering Structures, 2016, 126: 133-146. DOI: https://doi.org/10.1016/j.engstruct.2016.07.035.
[102] HE Xu-hui, ZHOU Lei, CHEN Zheng-wei, JING Hai-quan, ZOU Yun-feng, WU Teng. Effect of wind barriers on the flow field and aerodynamic forces of a train–bridge system [J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2019, 233(3): 283-297. DOI: https://doi.org/10.1177/0954409718793220.
[103] HASHMI S, HEMIDA H, SOPER D. Wind tunnel testing on a train model subjected to crosswinds with different windbreak walls [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2019, 195: 104013. DOI: https://doi.org/10.1016/j.jweia.2019.104013.
[104] MOHEBBI M, REZVANI M. Analysis of the effects of lateral wind on a high speed train on a double routed railway track with porous shelters [J]. Journal of Wind Engineering and Industrial Aerodynamics, 2019, 184: 116-127. DOI: https://doi.org/10.1016/j.jweia.2018.11.011.
(Edited by HE Yun-bin)
中文导读
高速铁路桥梁-列车系统的横风气动特性研究综述
摘要:本文主要综述了中南大学工程研究中心在桥梁-列车系统横风气动特性研究领域的重要成果。首先,从流体力学的角度分析了列车周围的流场特性,从而揭示了目前高速列车横风气动力评估方法的主要误差来源。然后,介绍了现场实测,数值模拟,动、静模型风洞实验等四种列车气动力评估方法及其优缺点。同时介绍了中南大学风工程研究中心最新开发的列车-线路系统动模型实验装置。为深化研究桥梁和列车之间的气动干扰规律。本文首先详细论述了与桥梁-列车系统横风气动特性相关的基础研究成果,主要包括既有高速铁路桥梁特征几何参数的统计结果,简化列车的气动特性,以及大跨度扁平箱梁和桁架主梁的气动特性。在上述研究的基础上,重点讨论了扁平箱梁和桁架主梁与列车之间的气动干扰规律。最后,对中南大学风工程研究中心开发的可调风向和透风率的新型风屏障及其相关研究结果进行了详细介绍。
关键词:高速铁路;车-桥系统;风屏障;横风;气动特性;风洞试验
Foundation item: Project(2017YFB1201204) supported by National Key R & D Program of China; Projects(51925808, U1934209) supported by the National Natural Science Foundation of China
Received date: 2019-12-28; Accepted date: 2020-03-13
Corresponding author: HE Xu-hui, PhD, Professor; Tel: +86-731-82655366; E-mail: xuhuihe@csu.edu.cn; ORCID: 0000-0003-2746- 182X

