
Thermodynamic analysis of separating lead and antimony in chloride system
CHEN Jin-zhong(陈进中)1, CAO Hua-zhen(曹华珍)2, LI Bo(李 波)2,
YUAN Hai-jun(袁海军)2, ZHENG Guo-qu(郑国渠)2, YANG Tian-zu(杨天足)1
1. School of Metallurgical Science and Engineering, Central South University, Changsha 410083, China;
2. School of Chemical Engineering and Materials, Zhejiang University of Technology,
Hangzhou 310014, China
Received 13 October 2008; accepted 18 February 2009
Abstract: In chloride system, thermodynamic analysis is a useful guide to separate lead and antimony as well as to understand the separation mechanism. An efficient and feasible way for separating lead and antimony was discussed. The relationships of [Pb2+][Cl-]2—lg[Cl]T and E—lg[Cl]T in Pb-Sb-Cl-H2O system were studied, and the solubilities of lead chloride at different antimony concentrations were calculated based on principle of simultaneous equilibrium. The results show that insoluble salt PbCl2 will only exist stably in a certain concentration range of chlorine ion. This concentration range of chlorine ion expands a little with increasing the concentration of antimony in the system while narrows as the system acidity increases. The solubility of Pb2+ in solution decreases with increasing the concentration of antimony in the system, whereas increases with increasing the concentration of total chlorine. The concentration range of total chlorine causing lead solubility less than 0.005 mol/L increases monotonically.
Key words: lead; antimony; separation; chloride system; thermodynamic analysis
1 Introduction
It is difficult to separate lead and antimony from jamesonite by mineral process because they exist as sulfide solid solutions and disseminate each other[1]. In order to utilize comprehensively the jamesonite, it must be treated by metallurgy method. The pyrometallurgy process to separate the lead and antimony is described as follows. Firstly, the lead and antimony alloy is produced by fluidized roasting desulfurization, calcine sintering mixture and reduction smelting in blast furnace; then, the antimony oxide powder and crude lead are produced by converting the lead and antimony alloy in reverberatory furnace; finally, lead and antimony are separated. But there are some problems in the pyrometallurgy process, such as lots of recycle materials, long process, difficulty in separating lead and antimony, low recovery of lead and antimony[2].
It is easy to separate lead and antimony by using the solubility of PbCl2 while the jamesonite is treated by leaching in chloride system. TANG and WANG[3] used SbCl5 as oxidant to separate the lead and antimony by chloridization-leaching the jamesonite. The mass ratio of lead to antimony is 3×10-5. WANG et al[4] used the slurry electrolysis process to treat complex antimony lead concentrate, and proposed that antimony can be separated from lead in one step, and metallic antimony can be achieved in cathode directly at the same time. The recovery of antimony is above 98%. The lead in residue of slurry electrolysis process is transformed into carbonate from chloride with ammonium carbonate. The total recoveries of lead and silver are over 95% and 80%, respectively.
In various media, such as NaCl, HCl and CaCl2, the solubility of PbCl2 as a function of temperature has been studied[5-7]. In chloride system[8-9], the thermo- dynamic analysis of the PbCl2 solubility has been initially studied. But the thermodynamic analysis of PbCl2 solubility in antimony trichloride solution has not been reported yet.
This work focused on the principle of simultaneous equilibrium[10-11] to describe the thermodynamic details of the PbCl2 solubility in antimony trichloride system. The feasibility and rationality of the separation of lead and antimony in chloride system was justified in theory and the important theory base for the study and manufacture was also raised.
2 Equilibrium equations in solution
The divalent lead ion and trivalent antimony ion can react with chlorine ion to create a series of complex ions in the chloride solution. The complex reactions and equilibrium constants are shown as follows[12]:
Sb3++iCl-=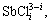
 i=1, 2, 3, 4, 5,6 (1)
i=1, 2, 3, 4, 5,6 (1)
Sb3++H2O=SbO++2H+,  (2)
(2)
Pb2++jCl-=
 j=1, 2, 3, 4 (3)
j=1, 2, 3, 4 (3)
where Ki and Kj are cumulative stability constants of complex ion at all levels. The thermodynamic data are from Ref.[13].
We attempt to study the equilibrium in high acidity condition, so the complex of lead and antimony with hydroxide can be ignored. The total concentrations of antimony, [Sb]T, lead, [Pb]T, and chlorine, [Cl]T, in the solution can be calculated by Eqs.(4)-(6):
 (4)
(4)
 (5)
(5)
 (6)
(6)
where [M]T denotes the total concentration of M and [M] is the concentration of free M.
[Sb]T, [Pb]T, [Cl]T and [H+] in Eqs.(4)-(6) can be controlled during practical work. The concentrations of free Sb3+, Pb2+ and Cl- can be calculated at the four given values.
When the solution coexists with insoluble chloride (PbCl2), the relationship between [Pb2+] and [Cl-] is determined by the solubility product (Ksp) of lead chloride:
 (7)
(7)
Moreover, when the solution is in a equilibrium condition, only one electrode potential exists based on
the principle of simultaneous equilibrium: 
 The above
The above
value can be calculated by the following equation:
 (8)
(8)
Using the total concentration of lead, [Pb]T, to take the place of the concentration of free lead ion, [Pb2+], in Eq.(8), Eq.(9) is formed. And the relationship between the lead electrode potential and [Cl-] at given total concentration of lead, [Pb]T, is represented by Eq.(10):
 (9)
(9)
 (10)
(10)
Then, E—lg[Cl]T diagram at a given temperature and a total lead concentration is plotted based on the relationship between [Cl-] and [Cl]T, Eq.(10). In order to demonstrate the effect of [Sb]T, [Pb]T and [H+] on lead electrode potential, E—lg[Cl]T diagram of lead is plotted.
In E—lg[Cl]T diagram, two intersection points of the two curves are ascertained by Eqs.(9) and (10), which are marked as α and β, respectively. And the range of [Cl]T corresponding to the two points is the concentration range of total chlorine, in which the precipitation PbCl2 exists stably.
The calculation of points α and β could be educed based on the relationship among the point potentials,  and
and  Also, they could be calculated by Eq.(11) based on the principle of solubility product:
Also, they could be calculated by Eq.(11) based on the principle of solubility product:
 (11)
(11)
It is concluded that [Cl-] and the corresponding [Cl]T are gained with the help of Eq.(11) and Eq.(6), respectively. The total chlorine concentration corresponding to α and β changes as the total lead concentration changes. So, the practical solubility curves of solid PbCl2 at different temperatures are gained according to [Pb]T–[Cl]T diagram.
3 Thermodynamic analysis of Pb-Sb-Cl- H2O system
3.1 Relationship between solubility product and lg[Cl]T at different [Sb]T
To elucidate the separating mechanism of Pb and Sb in Pb-Sb-Cl-H2O system, the relationship between solubility product and lg[Cl]T at different [Sb]T is firstly studied. Fig.1 shows the curves of [Pb2+][Cl-]2 vs lg[Cl]T in Pb-Sb-Cl-H2O system at different [Sb]T. When there is no antimony in the system, the limiting point (α) appears with [Cl]T reaching a certain amount. PbCl2 precipitation is just observed at this point, corresponding to the value of [Pb2+][Cl-]2 in the solution larger than the solubility of the PbCl2. However, when [Cl]T reaches the limiting point β, the precipitated PbCl2 will dissolve into the solution again because the value of [Pb2+][Cl-] in the solution is smaller than the solubility of PbCl2. That is to say, the insoluble salt PbCl2 will only exist stably in a certain concentration range of the chlorine ion (between α and β). The points α and β move to right with increasing antimony concentration in the system and the concentration range of the chlorine ion expands a little as well. The total chlorine concentration that can dissolve the PbCl2 is about 20 mol/L, when the concentration of antimony increases to 2.5 mol/L. However, the total chlorine concentration is usually less than 20 mol/L in practical antimony trichloride leaching system. Thus, the separation of lead and antimony becomes feasible because the insoluble salt PbCl2 will exist stably in the certain concentration range of chlorine ion, while antimony can be soluble in this concentration range.
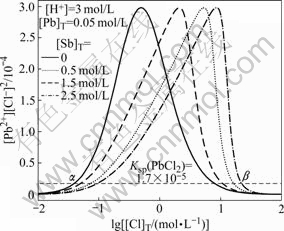
Fig.1 [Pb2+][Cl-]2 as function of lg[Cl]T in Pb-Sb-Cl-H2O system at 25 ℃
3.2 E—lg[Cl]T diagram at different [Sb]T
Fig.2 shows E—lg[Cl]T diagram of Pb-Sb-Cl-H2O system at different [Sb]T. It is clear that the equilibrium concentration ranges of chlorine ion ascertained by points α and β are similar to those in Fig.1. Moreover, the areas in which metal lead exists stably expand with increasing antimony concentration. The line crossing α and β is linear when no antimony exists in the system while it is curve when antimony exists in the system. The reason may be that the Sb3+ reacts with chlorine ion more easily than Pb2+ to create more complexes. The difference of equilibrium constants can also be found in Table 1. Thus, the concentration of free chlorine ion is affected, in turn changing the areas in which metal lead exists stably.
Table 1 Stability constants of ions in Pb-Sb-Cl-H2O solution at 25 ℃
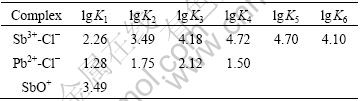
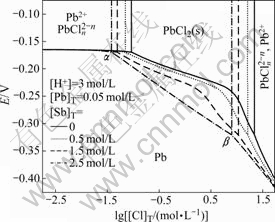
Fig.2 E—lg[Cl]T diagram of Pb-Sb-Cl-H2O system at different [Sb]T
3.3 E—lg[Cl]T diagram at different [Pb]T
To elucidate more clearly, the changes of E with [Cl]T at different [Pb]T are studied as well. Fig.3 shows the E—lg[Cl]T diagram at different [Pb]T. The areas ascertained by points α and β in which solid lead chloride exists stably at given conditions expand a little with increasing the total lead concentration. Point α moves to top left corner while point β moves to bottom right corner, thus the ascertained areas in which metal lead exists stably also expand a little with increasing the total lead concentration. Moreover, the corresponding potentials  and
and  increase with increasing the total lead concentration. In summary, when the total antimony concentration and acidity of system are given, [Cl]T corresponding to points α and β changes with increasing the total lead concentration, as well as changes with increasing the lead solubility in the solution, demonstrating easy separation Sb from Pb at a higher [Pb2+].
increase with increasing the total lead concentration. In summary, when the total antimony concentration and acidity of system are given, [Cl]T corresponding to points α and β changes with increasing the total lead concentration, as well as changes with increasing the lead solubility in the solution, demonstrating easy separation Sb from Pb at a higher [Pb2+].
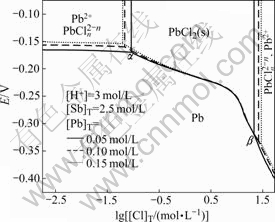
Fig.3 E—lg[Cl]T diagram of Pb-Sb-Cl-H2O system at different [Pb]T
3.4 E—lg[Cl]T diagram at different [H+]
Fig.4 shows E—lg[Cl]T diagram of Pb-Sb-Cl-H2O system at different [H+]. It can be found that point α moves to right while point β stays in original place and thus the chlorine concentration range between α and β narrows with increasing the system acidity. The reason may be that there is SbO+ in the Pb-Sb-Cl-H2O system and it has a proportional relation with [Sb3+] and [H+]. Thus, [H+] influences [Sb3+] indirectly by influencing [Cl-] and finally the total chlorine concentration range in which precipitated PbCl2 exists stably is also influenced.
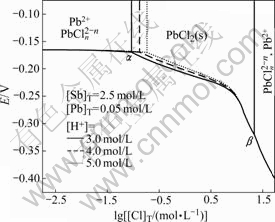
Fig.4 E—lg[Cl]T diagram of Pb-Sb-Cl-H2O system at different [H+]
3.5 Solubility curve of PbCl2(s) at different [Sb]T
Fig.5 shows the solubility of PbCl2(s) as a function of [Cl]T at different [Sb]T in Pb-Sb-Cl-H2O system at 25 ℃. It is clear that the lead concentration reaches the lowest value (0.003 5 mol/L) in solutions with [Cl]T reaching 0.3 mol/L when there is no antimony in the system. That is to say, when the total chlorine concentration is less than 0.3 mol/L, the solubility of lead decreases with increasing the total chlorine concentration. However, when the concentration is more than 0.3 mol/L, the solubility of lead rises sharply because increasing chloride activity favors the formation of soluble lead chloride complexes  where i=1, 2, 3, 4). The same phenomena were reported by other researchers [14-15].
where i=1, 2, 3, 4). The same phenomena were reported by other researchers [14-15].
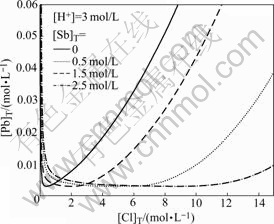
Fig.5 Solubility curve of PbCl2(s) in Pb-Sb-Cl-H2O system at different [Sb]T
The solubility of lead ion reaches the lowest value when the total chlorine concentration is 2.0 mol/L and the concentration of antimony in the system is 0.5 mol/L. And the solubility of lead ion is less than 0.005 mol/L when the total chlorine concentration ranges from 0.3 mol/L to 0.5 mol/L. The range of [Cl]T corresponding to the solubility of lead less than 0.005 mol/L also continually extends with the increase in total chlorine concentration. [Cl]T range is 1.0-8.0 mol/L when the concentration of antimony is 1.5 mol/L, whereas the chlorine concentration range is 2.0-13.0 mol/L when the concentration of antimony increases to 2.5 mol/L (here two different concentrations have comparability). It is sort well in the practical leaching system with a high concentration of antimony. The solubility of lead decreases with increasing the antimony concentration in the leaching solution. So, the favorable effect on the separation of lead and antimony is better.
Over the certain [Cl]T, the solubility of Pb upgrades markedly with the increase in [Cl]T, but the change tendency becomes weaker as [Sb]T increases.
4 Conclusions
1) The thermodynamic analysis of Pb-Sb-Cl-H2O system is introduced here based on the principle of simultaneous equilibrium.
2) The E—lg[Cl]T diagram, [Pb2+][Cl-]2—lg[Cl]T diagram and the solubility curves of lead chloride at different antimony concentrations are plotted, respectively.
3) The insoluble salt PbCl2 only exists stably in a certain concentration range of chlorine ion. This concentration range of chlorine ion in which insoluble salt PbCl2 exists stably expands a little with increasing the concentration of antimony in the system. The solubility of Pb in solution decreases with increasing the concentration of antimony in the system. And the concentration range of total chlorine corresponding to lead solubility less than 0.005 mol/L expands continually, meaning that separating the lead and antimony is feasible. The solubility of lead increases with the increasing of the concentration of total chlorine in the system, while the concentration range of chlorine ion in which insoluble salt PbCl2 exists stably narrows with the increasing of the system acidity.
References
[1] ZHAO Tian-cong. Antimony [M]. Beijing: Metallurgical Industry Press, 1987. (in Chinese)
[2] SLOBODSKA Y Y. Pyrometallurgical treatment of lead-antimony ores [J]. Tsvetn Metally, 1932, 7: 108-111.
[3] TANG Mo-tang, WANG Ling. A new process for treating lead solution of jamesonite concentrate by a neutralization hydrolization method [J]. Journal of Central South University: Science and Technology, 1997, 28(3): 226-228. (in Chinese)
[4] WANG Cheng-yan, QIU Ding-fan, JIANG Pei-hai, DENG Chun-long. Study on slurry electrolysis process for treating complex antimony-lead concentrate [J]. Mining and Metallurgy, 2002, 11(3): 55-58. (in Chinese)
[5] WINAND R. Chloride hydrometallurgy [J]. Hydrometallurgy, 1991, 27(3): 285-316.
[6] HOLDICH R G, LAWSON G J. The solubility of aqueous lead chloride solution [J]. Hydrometallurgy, 1987, 19(2): 199-208.
[7] LIU Yun-xia. Study on PbCl2 solubility under different conditions of HCl concentration, Sb3+ concentration and temperature [J]. Chinese Journal of Rare Metals, 2000, 24(4): 270-273. (in Chinese)
[8] LINKE W F, SEIDELL A. Solubility of inorganic and metal organic compounds [M]. New York: Am Chem Soc, 1965.
[9] PETERS E. Applications of chloride hydrometallurgy to treatment of sulphide minerals [C]// Proc Symposium on Chloride Hydrometallurgy. Brussels: Benelux Metallurgie, 1977: 1-37.
[10] XIAO Wen-de, ZHU Kai-hong, YUAN Wei-kang, CHIEN H H. An algorithm for simultaneous chemical and phase equilibrium calculation [J]. American Institute of Chemical Engineers, 1989, 35(11): 1813-1820.
[11] ZHENG Guo-qu, CAO Hua-zhen, ZHANG Li-feng. Influence of ammonia concentration on anodic deposition of nickel oxide [J]. J Appl Electrochem, 2007, 37(7): 799-803.
[12] POTER S K, JACOBSON R A. The crystal structure of pyridinium tetrachloroantimonate(Ⅲ) [J]. Journal of the Chemical Society A: Inorganic, Physical, Theoretical, 1970, 31(1): 1356-1359
[13] KOTRLY S, SUCHA L. Handbook of chemical equilibria in analytical chemistry [M]. Chichester: Ellis Horwood, 1985.
[14] SILLEN L G, MARTELL A E. Stability constants of metal-ion complexes [M]. London: The Chemical Society, 1971.
[15] MUIR D M, GALE D C, PARDER A J, GILES D E. Leaching of McArthur river lead-zinc sulphide concentrate in aqueous chloride and chloride systems [J]. Proc Australian Inst Min Metall, 1976, 259: 23-25.
Foundation item: Project(2006BAB02B04-4-1)supported by the National Key Technology R&D Program of China
Corresponding author: ZHENG Guo-qu; Tel: +86-571-88320429; E-mail: zhenggq@zjut.edu.cn
DOI: 10.1016/S1003-6326(08)60341-6
(Edited by YANG Hua)