Magnetocrystalline anisotropy and spin reorientation transition of

DyMn₆Sn₆ compound

GUO Guang-hua(郭光华), QIN Jiang(秦 江), ZHANG Hai-bei(张海贝)

School of Physics Science and Technology, Central South University, Changsha 410083, China

Received 5 September 2006; accepted 4 December 2006

Abstract: The spin-reorientation transition of intermetallic compound DyMn₆Sn₆ was investigated by applying the molecular field theory. The temperature dependence of easy magnetization direction of compound and the magnetic moment directions of Dy and Mn ions were theoretically calculated and they have good agreement with the experimental data. In the framework of single ion model, the temperature dependence of magnetocrystalline anisotropic constants K_1R and K_2R of Dy ion were also calculated. The results show that the fourth-order crystal field parameter,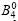, and the corresponding second-order magnetocrystalline anisotropic constant, K_2R, of Dy ion must be taken into account in order to explain the spin-reorientation transition satisfactorily. The competition between K_2R and K_1R plays a key role in the spin-reorientation transition of DyMn₆Sn₆.

Key words: rare earth-transition metal compound; spin-reorientation transition; magnetocrystalline anisotropy

1 Introduction Recently, the rare earth-transition metal compounds RM₆X₆ (R=rare-earth elements, M=transition metal and X=Sn and Ge) have attracted much attention due to their abundant magnetic properties[1-15]. RMn₆Sn₆ with heavy rare earth element crystallizes into HfFe₆Ge₆-type structure (space group is P₆/mmm), which is composed of hexagonal R layers comprising Sn atoms and Mn Kagomé nets, stacked in the sequence -Mn-R-Mn-Mn-R-Mn- along the c-axis[1]. From the magnetic point of view, RMn₆Sn₆ have two different magnetic subsystems: R- and Mn-subsystems. Neutron diffraction studies and magnetic measurements[1-3] indicate that Mn- and R-subsystems simultaneous transfer from paramagnetic state into ferromagnetic state for compounds with Tb, Dy and Ho below Curie temperature T_C (where T_C=423, 393 and 376 K for Tb, Dy and Ho, respectively) due to the strong R-Mn exchange interaction. Mn-subsystem and R-subsystem are antiferromagnetically coupled with each other. When temperature drops to T_SR, spin reorientation transition occurs in the compounds and the easy magnetization direction transfers from c-plane to c-axis. The spin reorientation temperatures T_SR are 330, 300 and 200 K for Tb, Dy and Ho compounds, respectively. For TbMn₆Sn₆, when temperature is below 250 K, the easy magnetization direction completely transfers to c-axis. While for DyMn₆Sn₆ and HoMn₆Sn₆, the directions do not completely transfer to c-axis. The angles of easy direction with c-axis are 45? for Dy at 220 K and 48? for Ho at 100 K and keep constants until 2 K. Many researches[2,4-5] show that the mechanism of spin reorientation transition is different for three compounds. The spin reorientation transition in TbMn₆Sn₆ comes from the competition of magnetocrystalline anisotropy energies of Tb- and Mn-subsystems. While for DyMn₆Sn₆ and HoMn₆Sn₆, the competition between the first- and the second-order magnetocrystalline anisotropy constants K₁ and K₂ plays a very important role (which means the fourth-order crystalline field parameter of rare earth ions cannot be ignored). In this paper, the mechanism of spin reorientation transition of DyMn₆Sn₆ compound was quantitatively investigated in the frame work of molecular field theory. The temperature depend- ences of magnetocrystalline anisotropy constants of Dy ion were also calculated based on the single ion model.

2 Theoretical model

DyMn₆Sn₆ is composed of two magnetic subsystems: Dy- and Mn-subsystems. According to the molecular field theory, the Hamiltonians of Dy and Mn ions have the following forms:

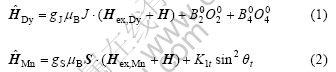
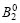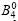where  g_J and g_S represent the g-factors of Dy and Mn ions; J and S are the total angular momentum operator of Dy ion and spin momentum operator of Mn ion, respectively; μ_B is Bohr momentum;  and  describe the crystalline field parameters of Dy³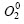⁺;  and 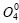 are Stevens operators; K_1t is magnetocrystalline anisotropy constant of Mn ion and θ_t is the angle of Mn ion magnetic moment with respect to c-axis; H is external magnetic field; the molecular fields acting on the Dy and Mn ions are H_{ex, Dy} and H_{ex, Mn}, which can be written as

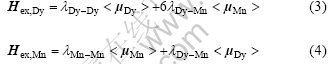
λ_Dy-Dy, λ_Dy-Mn and λ_Mn-Mn represent the molecular field constants of Dy-Dy, Dy-Mn and Mn-Mn exchange interaction, respectively. 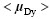 and 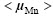 are the thermal average of magnetization of Dy- and Mn-subsystems, respectively.

The free energy of system can be given as

where  k_B is Boltzmann constant; Z_Dy and Z_Mn are partition functions of Dy and Mn ions, respectively.

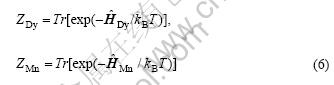
From the stable equilibrium condition the magnitude of 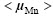and 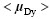, as well as their direction with respect to the c-axis can be evaluated. Consequently, the magnetic structure and the temperature and field dependence of magnetization of compounds can be determined.

In our calculation the values of Dy and Mn magnetic moments at 4.2 K are taken to be 10μ_B and 2.21μ_B, respectively[2]. By fitting the experimental data in Ref.[2], including the spin reorientation temperature and thermal magnetization curves, the other magnetic parameters are estimated as follows: λ_Mn-Mn=162.3T/μ_B, λ_Dy-Mn=-5.8T/μ_B, λ_Dy-Dy=2.1T/μ_B, 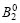=-0.25 K, 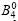= 0.003 K, K_1t0=-1.5 K/Mn (valued at T=4.2 K).

Fig.1 shows the theoretically calculated temperature dependences of easy magnetization direction of DyMn₆Sn₆ and the angles of Dy and Mn magnetic moments with respect to the c-axis. When temperature is above 300 K, as seen from Fig.1, the easy magnetization direction lies in the c-plane. The angles of Dy and Mn magnetic moments are all equal to 90?. At T=300 K the spin reorientation transition occurs and the easy direction begins to turn to c-axis. As temperature reaches 220 K the angle between the easy direction and c-axis is 45? and keeps this value down to 2 K. During the process of spin reorientation the Dy and Mn magnetic moments keep antiparallel. The calculated results are in good agreement with neutron diffraction data[2].

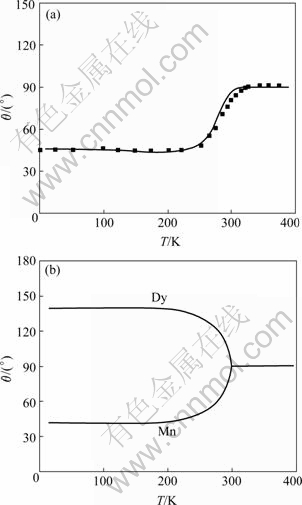
Fig.1 Temperature dependence of easy magnetization direction of DyMn₆Sn₆ (a) and angles of Dy and Mn magnetic moments with respect to c-axis (b) (Solid lines are theoretically calculated curves, dots denote experimental data)

There are many reasons that can lead to the spin reorientation transition. The competition between the magnetocrystalline anisotropy energies of two magnetic subsystems or the competition among the different order magnetic anisotropy constants in the same subsystem may all give rise to the change of easy magnetization direction. The estimated magnetocrystalline anisotropy constant K_1t of Mn-subsystem has negative value, which indicates that its easy magnetization direction lies in the c-plane. Neutron diffraction study also confirms that the easy direction of Mn-subsystem is in the c-plane[2]. The crystal field parameter 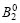 acting on Dy⁺³ is negative (corresponding  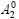is positive), which favors the easy magnetization direction of Dy-sublattice along the c-axis. Investigations in Refs.[2, 4] showed the spin reorientation transition of TbMn₆Sn₆ can be described in the frame work of competition between the magnetocrystalline anisotropy energies of Tb- and Mn-subsystems. For DyMn₆Sn₆ and HoMn₆Sn₆, however, the competition between the first-order and the second-order magnetocrystalline anisotropy constants K₁ and K₂ play an important role in the process of spin reorientation[5-6]. In fact, the estimated  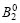is relatively small compared with other rare earth- transitional metal compounds (corresponding  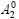equals to 126 K?, while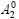 of R₂Fe₁₄B is as large as 983 K?[2]), which indicates that the higher order crystal field parameter cannot be neglected. Our calculations also show that 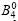 plays a very important role in the spin reorientation transition in DyMn₆Sn₆. Furthermore, below 220 K, the easy magnetization direction of DyMn₆Sn₆ is in cone plane. This requests that K₁ and K₂ must satisfy the condition: 0＜-K₁＜2K₂. Therefore K₂ must be taken into account in order to explain the spin reorientation transition of DyMn₆Sn₆, which mainly comes from the fourth-order crystal field parameter, 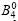, of Dy ion.

In order to explain the spin reorientation transition of DyMn₆Sn₆ quantitatively, we calculated the temperature dependence of magnetocrystalline anisotropy constants of Mn- and Dy-subsystems in the frame work of single ion model. We consider the magnetocrystalline anisotropy constant of Mn-subsystem follows the Akulov-Zener law: 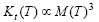. In accordance with single ion model, the magnetocrystalline anisotropy coefficients of rare-earth sublattice can be written as follows:

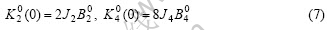
where  J₂=J(J-1/2), J₄=J₂(J-1)(J-3/2).

The temperature dependence of 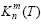is as

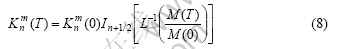
where  I_n+1/2 is hyperbolic Bessel function and L is Langevin function.

The magnetocrystalline anisotropy constants K_1R and K_2R of rare earth subsystem can be calculated from the following formula:

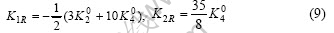
Fig.2 shows the theoretically calculated temperature dependence of K_1R and K_2R of Dy-subsystem. It can be seen from Fig.2 that K_2R keeps positive in the whole temperature range of magnetically ordered state, which indicates that K_2R favors the easy magnetization direction of Dy-subsystem directed along the c-axis. In contrast, K_1R changes its sign from positive to negative with the decrease of temperature. In high temperature range K_1R favors the magnetic moment of Ho⁺³ along the c-axis. Below 145 K, K_1R becomes negative and makes for magnetic moment of Ho⁺³ perpendicular to the c-axis. The total magnetocrystalline anisotropy constants of DyMn₆Sn₆ are K₁=K_1R+6_K1t, K₂=K_2R. In the whole temperature range of magnetically ordered state, K₁ is always negative and K₂ keeps positive.

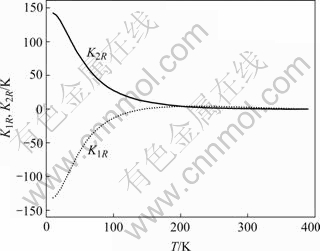
Fig.2 Theoretically calculated temperature dependence of K_1R and K_2R

Our theoretical calculations (Fig.1(b)) and neutron diffraction data[2] all show that the magnetic moments of Dy and Mn ions are always antiparallel in the whole magnetically ordered state. In this case, the free energy correlated to the spin reorientation transition only includes the magnetic anisotropic energy:

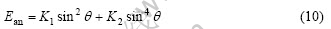
where  θ is the angle of the easy magnetization direction of DyMn₆Sn₆ with respect to the c-axis. The directions of magnetic moments of Dy and Mn ions and the easy magnetization direction of compounds can be decided from equilibrium condition. There are three states: 1) θ₁=π/2 corresponding the c-plane easy magnetization direction; 2) θ₂=0 indicating the c-axis easy magnetization direction; 3) sin²θ₃=-K₁/2K₂ and sin²θ₃≤1 representing the easy magnetization cone. The free energies corresponding the three solutions are E₁=K₁+K₂, E₂=0 and E₃=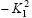/4K₂, respectively. By comparing the values of the three energies we can decide which state is the stablest one. Fig.3 displays the temperature dependences of E₁, E₂ and E_3. As shown in Fig.3, in high temperature range E₁ is the smallest one, which indicates that the easy magnetization direction is in c-plane. When temperature is below T_SR of 300 K, E₃ becomes the smallest one, showing the spin-reorientation transition occurs and the easy magnetization direction begins to transfer to the c-axis (when T＞T_SR, sin²θ₃= -K₁/(2K₂)＞1, therefore θ₃ is not the solution). At T=2 K, sin²θ₃=0.513, θ₃=45?. In the whole temperature range of magnetically ordered state E₂ is not the smallest energy. This means that the easy magnetization direction does not completely transfer to the c-axis. The calculated θ₃ and spin reorientation temperature T_SR are in good agreement with neutron diffraction results[2].

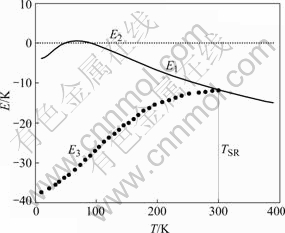
Fig.3 Temperature dependences of free energies E₁, E₂ and E₃

 4 Conclusions

1) The magnetic parameters of DyMn₆Sn₆ were estimated in the framework of molecular field model by fitting the different kinds of experimental data. The temperature dependence of magnetocrystalline anisotropy constants K_1R and K_2R of Dy ion was calculated. It is shown that the K_2R keeps positive in the whole magnetically ordered temperature range, which indicates that K_2R favors the magnetic moment of Dy ion directed along the c-axis. On the contrary, K_1R changes its sign from positive to negative at T=145 K. Above 145 K, K_1R is positive and makes for the magnetic moment of Dy ion directed along the c-axis, and below this temperature it favors the moment direction of Dy ion lying in the c-plane. The fourth order crystal field 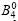 makes great contribution to the magnetocrystalline anisotropy energy. The total magnetocrystalline anisotropy constant K₁ is always negative and K₂ keeps positive in the whole temperature range of magnetically ordered state.

2) The spin reorientation of TbMn₆Sn₆ comes from the competition of magnetocrystalline anisotropies of Tb- and Mn-subsystems. For DyMn₆Sn₆, however, in order to describe the spin reorientation transition satisfactorily, the fourth-order crystal field parameter 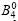 (corresponding to the second-order magneto- crystalline anisotropy constant K_2R) must be taken into account, which plays the key role in the low temperature range. In compound DyMn₆Sn₆ the magnetocrystalline anisotropy energies of Dy- and Mn-subsystems also compete with each other, but the competition between K_2R with K_1R and K_1t are the main cause of spin reorientation in compound DyMn₆Sn₆.

References

VENTURINI G, CHAFIK E L, IDRISSI B, MALAMAN B. Magnetic properties of RMn₆Sn₆ (R=Sc, Y, Gd-Tm, Lu) compounds with HfFe₆Ge₆ type structure [J]. J Magn Magn Mater, 1991, 94: 35-42.

MALAMAN B, VENTURINI G, WELTER R, SANCHEZ J P, VULLIET P, RESSOUCHE E. Magnetic properties of RMn₆Sn₆ (R=Gd-Er) compounds from neutron diffraction and mossbauer measurements [J]. J Magn Magn Mater, 1999, 202: 519-534.

CLATTERBUCK D M, Jr GSCHNEIDNER K A. Magnetic properties of RMn₆Sn₆ (R=Tb, Ho, Er, Tm, Lu) single crystals [J]. J Magn Magn Mater, 1999, 207: 78-94.

SCHOBINGER-PAPAMANTELLOS P, ANDR? G, RODRIGUEZ- CARVAJAL J, DUIJN H G M, BUSCHOW K H J. Magnetic ordering in TbMn_6-xCr_xSn₆ (x=1, 2) compounds studied by neutron diffraction and magnetic measurements [J]. J Alloys Comp, 2000, 306: 47-55.

LEF?VER C, VENTURINI G. A magnetic study of HoMn₆Sn_6-xGa_x single crystals (0.14≤x≤1.89) [J]. J Magn Magn Mater, 2004, 268: 374-379.

SCHOBINGER-PAPAMANTELLOS P, ANDR? G, RODRIGUEZ- CARVAJAL J, DUIJN H G M, BUSCHOW K H J. Magnetic ordering in DyMn_6-xCr_xSn₆ (x=1, 2) compounds studied by neutron diffraction and magnetic measurements [J]. J Magn Magn Mater, 2000, 219: 22-32.

WANG Ru-wu, ZHANG Li-gang, ZHANG Shao-ying, YAO Jin-lei, LIU Bao-dan, SHEN Bao-gen. Magnetism and magnetoresistance of Dy_1-xSm_xMn₆Ge₆ (x=0.2-1.0) compounds [J]. J Phys D: Appl Phys, 2004, 37: 385-388.

CAKIR O, DINCER I, ELMALI A, ELERMAN Y, EHRENBERG H, FUESS H. Investigation of magnetic phase transitions in HfFe₆Ge₆-type Gd_1-xEr_xMn₆Sn₆ intermetallics [J]. J Alloys Comp, 2005, 396: 18-21.

GUO Guang-hua, ZHANG Hai-bei. Magnetocrystalline anisotropy and spin-reorientation transition of HoMn₆Sn₆ compound [J]. J Alloys Compd, 2007, 429: 46-49.

GUO Guang-hua, ZHANG Hai-bei. The magnetic properties and magnetic phase transition of compound TbMn₆Sn₆ [J]. The Chinese Journal of Nonferrous Metals, 2005, 15(10): 1489-1492. (in Chinese)

ZHAO Peng, ZHANG Shao-Ying, CHENG Zhao-Hua, SHEN Bao-Gen. Magnetic properties of TbMn₆Sn_6-xGa_x (x=0.0-1.2) compounds [J]. Chin Phys, 2002, 11: 955-959.

ZHANG Li-gang, WANG Ru-wu, LI Lu, ZHANG Shao-ying, YAO Jin-lei, SHEN Bao-gen. Magnetic properties properties and magnetoresistance of HfFe₆Ge₆-type Dy_1-xGd_xMn₆Ge₆ (x=0.1-0.6) compounds [J]. Chin Phys, 2003, 12: 562-565.

GUO Guang-hua. The exchange interactions and magnetic properties of intermetallic compounds RMn₂Ge₂ [J]. Trans Nonferrous Met Soc China, 2003, 13(1): 1-5.

CAKIR O, DINCER I, ELMALI A, ELERMAN Y, EHRENBERG H, FUESS H. Investigation of magnetic phase transitions in HfFe₆Ge₆-type Gd_1-xEr_xMn₆Sn₆ intermetallics [J]. J Alloys Compd, 2005, 396: 18-21.

ZHANG L, KLAASSE J C P, BR?CK E, BUSCHOW K H J, de BOER F R. Magnetization studies of RMn₆Ge_6-xGa_x single crystals (R=Sc, Y, Gd-Lu; 0.63≤x≤1.00) [J]. Phys Rev B, 2004, 70: 224425.

Foundation item: Project(60571043) supported by the National Natural Science Foundation of China; Project(03JJY4044) supported by the Natural Science Foundation of Hunan Province, China

Corresponding author: GUO Guang-hua; Tel: +86-731-8836443; E-mail: guogh@mail.csu.edu.cn

(Edited by LI Xiang-qun)