J. Cent. South Univ. Technol. (2010) 17: 235-238

DOI: 10.1007/s11771-010-0036-0                                                                                                                 

Longitudinal crack on slab surface at straightening stage during continuous casting using finite element method

YU Hai-liang(喻海良)^{1, 2}, LIU Xiang-hua(刘相华)¹

1. State Key Laboratory of Rolling and Automation, Northeastern University, Shenyang 110004, China;

2. Department of Mechanical Engineering, Tsinghua University, Beijing 100084, China

? Central South University Press and Springer-Verlag Berlin Heidelberg 2010

Abstract: Deformation behavior of slab at the straightening stage during continuous casting was simulated by the explicit dynamic finite element method, and the stress distribution along the width direction of the slab and its change regularity at slab center during continuous casting were obtained. The influence of distribution and change of stress on the propagation of longitudinal cracks on slab surface was discussed. The results show that the tensional stress appears on slab surface at the inner arc side and the compressive stress appears on slab surface at the outer arc side at stages 6-8 in straightening zone during continuous casting. Longitudinal cracks generally appear on slab top surface and do not appear on slab bottom surface, which are also observed in industry.

Key words: continuous casting slab; straightening; longitudinal crack; finite element method

1 Introduction

During continuous casting, the longitudinal cracks might appear on slab surface, which would cause bleed-out in casting and severely affect the quality of slab, and some slabs might be directly rejected for the large crack size [1]. Cracks on continuous casting slab (CCS) surface might inherit to the rolled products [2-4]. And cracks on the surface of slab during rolling severely affect the quality of rolled products [5-6]. Researches on the initiation and propagation of longitudinal cracks on the surface of slab during continuous casting are important for guiding the production in industry.

There are many kinds of factors that might result in longitudinal cracks on slab surface during continuous casting, such as the continuous technologies, the continuous casting equipment, the mechanical properties of steel at high temperature, and the solidification properties [7]. The initiation of longitudinal cracks on slab surface during continuous casting was widely investigated [7-9]. The linear defects on rolled plates were caused by the surface longitudinal cracks on continuous casting slab by ZHOU and LUO [8]. And longitudinal cracks on continuous casting slab surface were caused by defects of crystallizer. The finite element method (FEM) has been widely used to analyze the thermal distribution during continuous casting [10-12] and the behavior of cracks on slab surface during rolling [13-15]. The closure and growth of longitudinal cracks in flat rolling process were simulated by ERVASTI and ST?HIBERG [14] and analyzed under a variety of crack sizes, roll radii and friction coefficients. The crack behavior during rolling can be divided into three steps: crack opening at the entrance to the gap, crack width decreasing within the roll gap, and a slight crack widening at the exit. The evolution behavior of the longitudinal cracks on slab surfaces in rolling process was analyzed by YUKAWA et al [15] using a rigid-plastic finite element (FE) code. In the above reviewed papers, the longitudinal cracks might appear on slab surface during continuous casting, whose evolution behavior during rolling was also simulated by finite element method (FEM). However, researches on the effect of stress distribution on the evolution of the longitudinal cracks on slab surface at the straightening stage during continuous casting using FEM were reported.

Therefore, in this work, the deformation behavior of slab at straightening stage during continuous casting was investigated using the explicit dynamic FEM on the platform of LS-DYNA. The stress (σ_Z) distribution on slab and the stress change curve at center of slab top surface at the straightening stage were obtained. And the influence of the stress distribution on the behavior of longitudinal cracks on slab surface was discussed.

2 Parameters and simulation

The deformation behavior of slab at straightening stage during continuous casting was simulated. In the FE model, the slab was assumed to be solidification in the straightening zone. The dimensions of slab profile (YZ profile) were 2.00 m×0.25 m. The radius of basis arc of casting mill was 10.3 m. The diameters of rollers from normal stage to horizontal stage were 0.145-0.280 m, separately. The length of rollers was 2.66 m. The pulling speed of slab was 0.85 m/min. The Coulomb friction law was employed for analyzing the contact conditions between slab and rollers, and the friction coefficient was 0.35.

During continuous casting, the rigid model was adopted for rollers, and the bilinear isotropic material model was adopted for the slab. In the models, the density of rollers and slab is 7.85 kg/dm³, elastic moduli of rollers and slab are 210 and 117 GPa, respectively, the Poisson ratio of slab is 0.36, and the deformation resistance is calculated by

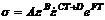                           (1)

where σ is the deformation resistance; ε is the strain;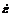 is the strain rate; T is temperature; A, B, C, D and F are material coeffients.

Due to symmetry of continuous casting process, half of the continuous casting model was employed in the FE model. According to the parameters above, the geometrical model was established, as shown in Fig.1(a). The slab and rollers were meshed with 8-noded hexahedral element. And the nodes on the symmetrical surface were constrained and the displacement along slab width direction was zero. In the model, there are 23 376 elements and 47 246 nodes. The meshing of continuous casting model is shown in Fig.1(b).

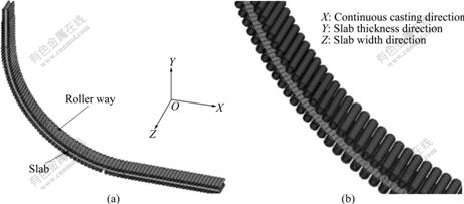
Fig.1 Geometrical and FE meshing models of slab during continuous casting: (a) Complete FE model of continuous casting;(b) Local FE model in straightening zone

3 Results and discussion

During continuous casting, there are 13 stages together, where stage 0 is the vertical stage, stage 1 is bending stage, stages 2-8 are datum arc stages and straightening stages, and stages 9-12 are horizontal stages. In continuous casting process, cracks often appear on the surface of slabs. And the distribution and change of stress on slab surface may play an important role on the behavior of cracks.

Fig.2 shows stress (σ_Z) distribution on slab in the straightening zone. As shown in Fig.2(a), P_A-P_D are the points distributed at the center of slab surface on inner arc side around stages 6-8. The tensional stress will appear in slab center zone on the inner arc side when the slab passes through the zone at stages 6-8 during continuous casting and will not appear when the slab is in the zone at stages 0-5 and stages 9-12. The maximum tensional stress reaches 1.60 MPa at the slab center, which gradually decreases, approaching to the slab edge side. In the same zone, from the outer arc side of slab, the compressive stress appears on slab surface, and the maximum stress reaches 2.22 MPa, as shown in Fig.2(b). So the longitudinal cracks will not propagate in straightening stage on the outer arc side of slab.

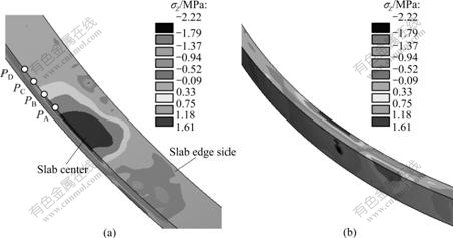
Fig.2 Stress (σ_Z) distribution on slab from inner arc side (a) and outer arc side (b)

Fig.3 shows the change regularity of stress (σ_Z) at slab center. When the slab passes through the zone of stages 6-8, the tensional stress appears and gradually increases to the maximum value of 0.8 MPa, and then gradually decreases until the compressive stress appears. It is obvious that the maximum tensional stress is less than the deformation resistance of slab, so there will be no cracks if the slab surface is of good quality before these stages. However, during continuous casting, micro- cracks might appear on slab surface due to the wear and fish of sheet copper of crystallizer and defective libration. So, if there are cracks deformed before the zone of stages 6-8, they might propagate for the stress concentration at crack tip.

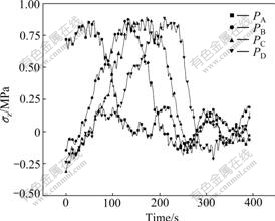
Fig.3 Change of stress (σ_Z) at slab center at stages 6-8 in straightening zone during continuous casting

As shown in Fig.4, the longitudinal crack in the zone of slab center appears on slab surface of the inner arc side during continuous casting.

According to the symmetry of crystallizer, when there exits a crystallizer in the slab, the surface quality of slab will be the same between the inner arc side of the slab and the outer arc side of the slab. So, there are microcracks on both sides of slab surface. Similarly, the evolution behavior of microcracks in the vertical stage will be also the same. However, most longitudinal cracks are observed on the slab top surface (inner arc side) in products. So the deformation behavior of slab at thestraightening stage will directly affect the initiation and propagation of longitudinal cracks on slab surface during continuous casting. From results in Figs.2 and 3, the compressive stress will appear on slab surface when the slab passes through stages 0-5, which will not result in the propagation of longitudinal cracks; and the tensional stress will appear on slab surface on inner arc side when the slab passes through stages 6-8, which might make the cracks propagate. So stages 6-8 are the key stages for the occurrence of longitudinal cracks during continuous casting.

In Fig.4, the longitudinal crack is model-I crack. The local crack increments [16-17] are obtained according to the Paris law:

                          (2)

where da/dN is the local crack growth rate of the crack tip at the depth; a is the crack length at a depth crack; C and m are constants; y is the factor of crack shape.

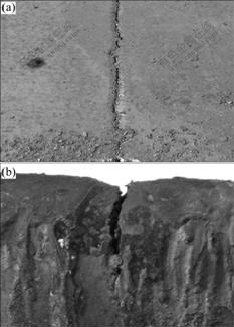
Fig.4 Longitudinal cracks on continuous casting slab from top surface (a) and side surface (b)

From Eq.(2), the local crack increment is in a direct proportion to the stress around crack and the initial crack size. When the slab with micro-longitudinal cracks on slab surface passes through stages 6-8, the tensional stress on the surface of inner arc side of slab will gradually increase. If the tensional stress makes the cracks propagate, the crack intensity factor range  will further increase, and the crack propagation speed will also increase. The propagation of cracks will not stop until the compressive stress appears on the slab surface. So the size of longitudinal cracks on slab top surface is generally large, as shown in Fig.4.

4 Conclusions

(1) The deformation behavior of slab during continuous casting is simulated by finite element method.

(2) During continuous casting, compressive stress (σ_Z) will appear on slab surface when slab passes through stages 0-5 and stages 9-11, and compressive stress will appear on the outer arc side of slab surface when slab passes through stages 6-8, where the longitudinal cracks will not propagate; the tensional stress will appear on the inner arc side of slab surface, and the longitudinal cracks may propagate. The phenomenon is the same as that observed in the industry during continuous casting.

(3) The relationship between the stress and the size of longitudinal cracks still needs to be studied further.

[1] MASTSUMIYA T. Recent topics of research and development in continuous casting [J]. ISIJ International, 2006, 46(12): 1800-1804.

[2] JIAO Guo-hua, WU Guang-liang, SUN Yan-hui, CHEN Xiao-jun, ZHUO Chun-quan. Reason and control of edge cracking of CSP hot-rolled strip [J]. Iron and Steel, 2006, 41(6): 27-31. (in Chinese)

[3] ISAEV O B, EMELYANOV V V, KISLITSA V V, MATROSOV Y I, LEPIKHOV L S. Effect of the carbon content of low-alloy steels on the surface quality of continuous-cast semifinished products and rolled plates [J]. Metallurgist, 2004, 48(5/6): 210-213.

[4] RAY A. Electron-probe microanalysis: Some revelations in the investigation of defects in steel products [J]. Materials Science Forum, 2005, 492/493: 627-634.

[5] YAMAMOTO K. Study on surface defects of coated sheets caused by the surface condition of a hot roughing mill roll [J]. SEAISI (South East Asia Iron and Steel Institute) Quarterly, 2007, 36(3): 54-58.

[6] RIEDEL H, ANDRIEUX F, WALDE T, KARHAUSEN K F. The formation of edge cracks during rolling of metal sheet [J]. Steel Research International, 2007, 78(10/11): 818-824.

[7] MIZUNO H, ESAKA H, SHINOZUKA K, TAMURA M. Analysis of the crystallization of mold flux for continuous casting of steel [J]. ISIJ International, 2008, 48(3): 277-285.

[8] ZHOU You-yu, LUO De-xin. Formation reason for longitudinal crack on surface of continuous casting slab [J]. Journal of Iron and Steel Research, 2004, 16(5): 75-78. (in Chinese)

[9] ZHANG Li, XU Guo-dong, WANG Xin-hua, ZHU Zhi-yuan. Investigation on longitudinal surface cracks of CC slabs for container [J]. Iron and Steel, 2002, 37(1): 19-21, 26. (in Chinese)

[10] LUDWIG A. Thermophysical properties necessary for advanced casting simulation [J]. International Journal of Thermophysics, 2002, 23(5): 1131-1146.

[11] SEYEDEIN S H, HASAN M. A 3-D numerical prediction of turbulent flow, heat transfer and solidification in a continuous slab caster for steel [J]. Canadian Metallurgical Quarterly, 1998, 37(3/4): 213-228.

[12] YANG Jian-wei, DU Yan-ping, SHI Rong, CUI Xiao-chao. Fluid flow and solidification simulation in beam blank continuous casting process with 3D coupled model [J]. Journal of Iron and Steel Research International, 2006, 13(4): 17-21.

[13] YU Hai-liang, LIU Xiang-hua, REN Xiao-jia. Behavior of longitudinal cracks in slab V-H rolling processes [J]. Steel Research International, 2008, 79(7): 537-543.

[14] ERVASTI E, ST?HIBERG U. Behaviour of longitudinal surface cracks in the hot rolling of steel slabs [J]. Journal of Materials Processing Technology, 1999, 94: 141-150.

[15] YUKAWA N, YOSHIDA Y, ISHIKAWA S. Deformation analysis of longitudinal surface micro-defects in flat rolling [J]. Journal of Iron and Steel Institute of Japan, 2006, 92(11): 661-666.

[16] BRANCO R, ANTUNES F V. Finite element modelling and analysis of crack shape evolution in mode-I fatigue middle cracked tension specimens [J]. Engineering Fracture Mechanics, 2008, 75: 3020-3037.

[17] RAVLEHANDRAN K S. Three-dimensional crack-shape effects during the growth of small surface fatigue cracks in a titanium-base alloy [J]. Fatigue and Fracture of Engineering Materials and Structures, 1997, 20(10): 1423-1442.

Foundation item: Project(50634030) supported by the National Natural Science Foundation of China; Project(20090042120005) supported by the Doctorate Foundation of the Ministry of Education of China; Project(2006CB605208-1) supported by the State Basic Research Program of China

Received date: 2009-03-05; Accepted date: 2009-07-11

Corresponding author: YU Hai-liang, PhD; Tel: +86-24-83681803; E-mail: yuhailiang1980@tom.com; yuhl@ral.neu.edu.cn

(Edited by CHEN Wei-ping)