J. Cent. South Univ. (2020) 27: 3839-3851

DOI: https://doi.org/10.1007/s11771-020-4503-y

Torsional dynamic response of tapered pile considering compaction effect and stress diffusion effect

GUAN Wen-jie(官文杰)^{1, 2, 3}, WU Wen-bing(吴文兵)^{1, 2}, JIANG Guo-sheng(蒋国盛)¹,CHIN Jian Leo³, DENG Guo-dong(邓国栋)⁴

1. MOE Engineering Research Center of Rock-Soil Drilling & Excavation and Protection, Faculty of Engineering, China University of Geosciences, Wuhan 430074, China;

2. MOE Guangxi Key Laboratory of Disaster Prevention and Engineering Safety, College of Civil Engineering and Architecture, Guangxi University, Nanning 530004, China;

3. School of Engineering, University of Western Sydney, Locked Bag 1797, Penrith, Sydney,NSW 2751, Australia;

4. School of Civil Engineering, Central South University, Changsha 410075, China

Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020

Abstract: Considering both the compaction effect of pile surrounding soil and the stress diffusion effect of pile end soil, this paper theoretically investigates the torsional vibration characteristics of tapered pile. Utilizing the complex stiffness transfer model to simulate compaction effect and tapered fictitious soil pile model to simulate stress diffusion, the analytical solution for the torsional impedance at tapered pile top is obtained by virtue of Laplace transform technique and impedance transfer method. Based on the present solution, a parametric study is conducted to investigate the rationality of the present solution and the influence of soil and pile properties on the torsional vibration characteristics of tapered pile embedded in layered soil. The results show that, both the compaction effect and stress diffusion effect have significant influence on the torsional vibration characteristics of tapered pile, and these two factors should be considered during the dynamic design of pile foundation.

Key words: tapered pile; compaction effect; stress diffusion effect; complex stiffness transfer model; tapered fictitious soil pile model

Cite this article as: GUAN Wen-jie, WU Wen-bing, JIANG Guo-sheng, CHIN Jian Leo, DENG Guo-dong. Torsional dynamic response of tapered pile considering compaction effect and stress diffusion effect [J]. Journal of Central South University, 2020, 27(12): 3839-3851. DOI: https://doi.org/10.1007/s11771-020-4503-y.

1 Introduction

In recent years, the tapered piles attracted many attentions from researchers due to their good bearing capacity. Because of the existence of the cone angle of tapered pile, the side surface of tapered pile is subjected to the normal force and the tangential force from the pile surrounding soil, which makes the friction between pile and its surrounding soil bigger. However, owing to the fact that the dynamic and static interaction mechanism between tapered pile and soil is still unclear and the construction technology is relatively complex, the tapered pile is not widely used in the engineering project. Over the last 50 years, field and model tests [1-6], theoretical method [7-9] and numerical simulations [10-12] have been used by many scholars to study the bearing capacity of tapered piles. Experimental data have shown that the use of tapered piles can increase the static axial capacity by up to 80% [7]. Compared with the static characteristics of tapered piles, there are few researches on the dynamic characteristics of tapered piles. Nevertheless, the dynamic interaction between a tapered pile and soil around the tapered pile is also an important consideration in the design of pile foundations. GHAZAVI [13] firstly idealized a uniformly tapered pile as a step-tapered pile to analyze the kinematic seismic response of tapered piles. Based on the same conception, GHAZAVI [14] and DEHGHANPOOR [15] studied the response of tapered piles subjected to vertical and lateral dynamic load, respectively. But they did not get the analytical solutions. CAI et al [16] firstly obtained the analytical solution of longitudinal dynamic complex impedance of tapered pile subjected to vertical dynamic load. After CAI’s research, WU et al [17] investigated the influence of the lateral inertial effect on the vertical vibration of tapered pile by the Rayleigh-Love rode model. WANG et al [18] further proposed a new model to simulate the interaction of tapered pile and its surrounding soil considering vertical reaction of pile surrounding soil at the damage site.

However, the soil compaction effect was not taken into consideration in the above researches. Recently, WU et al [19] and GAO et al [20] studied the vertical vibration of tapered piles by complex stiffness transfer model considering the soil compaction effect. Moreover, the pile end soil also has significant influence on the dynamic response of tapered pile. YANG et al [21] originally proposed a new model, namely fictitious soil pile model, to stimulate the stratification of pile end soil. Following the work of YANG et al [21], WU et al [22, 23] utilized the fictitious soil pile model to investigate the vertical and torsional vibration behavior of pile-soil by considering the radial wave effect. Soon later, WANG et al [24] and CUI et al [25] extended the fictitious soil pile model from one-dimensional condition to three-dimensional condition for the vertical vibration of piles embedded in single-phase soil and saturated soil. Then, WANG et al [26] presented the tapered fictious soil pile model that assuming the soil between the pile and bedrock as tapered soil pile, the cone angle of the tapered soil pile reflects the degree of stress diffusion effect. GUAN et al [27] employed the tapered fictious soil pile model to investigate the torsional vibration characteristics of tapered pile.

The previous studies only considered the compaction effect of tapered pile surrounding soil or the stress diffusion effect of tapered pile end soil. However, in practical engineering, these two effects exist simultaneously. In this paper, both the compaction effect of pile surrounding soil and the stress diffusion effect of pile end soil are taken into consideration to study the torsional dynamic response of tapered piles.

2 Mathematical model and general equations

2.1 Computational model and assumptions

The dynamic interaction model of tapered pile-soil system is shown in Figure 1(a). The pile model and the pile-end soil model are the same as those in Ref. [27], and the meanings of the corresponding parameters are also the same.

As shown in Figure 1(b), the pile surrounding soil is divided into two concentric regions to account for the radial inhomogeneity of pile surrounding soil owing to compaction effect [21, 28]; the outer region is semi-infinite and homogeneous; the inner region is disturbed and is subdivided into M concentric annular zones. The radii of the outer and inner boundary of the nth annular zone in the ith soil layer are denoted by 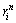and respectively.is the radius between outer and inner region. The complex-valued shear modulus of the inner region, i.e. 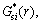is written as:

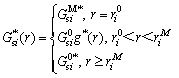               (1)

in which,

                     (2)

where r is the radial distance from the central axis of tapered pile to an arbitrary point; is imaginary unit;anddenote the shear moduli in the outer region and at the soil-pile interface,

respectively; and 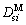represent the material damping coefficients at the corresponding locations, respectively.

Figure 1 Vibration model of pile-soil system:

According to EL NAGGAR’s work[28], the function g^*(r) is given to simulate the variation properties within the inner region:

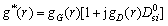                (3)

in which,

           (4)

where is the width of the inner region; p and q are positive-valued constants.

The soil of each annular zone in the ith layer is assumed to be homogeneous with shear modulus;is obtained by substituting Eq. (4) into Eq. (2).

The tapered pile-soil system satisfies the following assumptions:

1) There is no normal stress and shear stress at the free surface of soil, and the soil is infinite in the radial direction. The bottom of the fictitious soil pile is assumed to be rigid.

2) It is assumed that only the tangential displacement occurs during the torsional vibration.

3) The pile and soil keep good contact during the torsional vibration.

4) The tapered pile is assumed to be a viscoelastic, vertical and inverse cone bar.

2.2 Basis dynamic equilibrium equations

The displacement of the nth annular zone in the ith soil layer is denoted by Then, according to the torsional plane strain model by EL NAGGAR [28], the dynamic equation of pile surrounding soil can be expressed as follows:

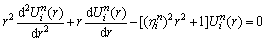   (5)

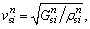
where denote the shear wave velocity, mass density, material damping and shear modulus of the nth annular zone in the ith soil layer, respectively; ω=2πf is the angular frequency, in which f is the natural frequency of soil-pile system. When n equals zero,is the displacement of the outer region in the ith soil layer.

In the ith pile layer,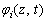represents the twist angle and the dynamic equilibrium equation is expressed as:

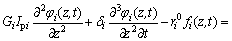
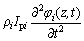                        (6)

where the meanings of corresponding parameters are the same as those in Ref. [27].

2.3 Boundary and initial conditions of pile-soil system

The boundary and initial conditions of the pile-soil system can be obtained as follows:

1) The value of the torque at the tapered pile head is equal to the torsional loading T(t):

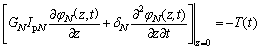    (7)

2) The twist angle at the bottom of pile end soil is zero:

                        (8)

3) The stress and twist angle between pile layers are the same:

      ⁽⁹⁾

4) The initial state of pile-soil system is motionless:

                         (10)

5) The stress and displacement between pile and surrounding soil are the same:

                     (11)

whereis the shear stress of the ith soil layer acting on the wall of the ith pile.

3 Solutions to equations

3.1 Solutions for surrounding soil

The solution of Eq. (5) is expressed as Eq. (12) based on the Bessel function theory:

              (12)

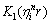where and denote the modified Bessel functions of first order of the first and second kinds, respectively;andrepresent the arbitrary constants which can be obtained by boundary conditions.

3.1.1 Solutions for outer region soil

As shown in Figure 1(b), n equals zero in the outer region. Assuming that the displacements and stresses of the outer region soil approach zero at an infinite horizontal distance from tapered pile, Thus, the displacement of the outer region soil in the ith layer can be expressed as:

                       (13)

The shear stress of the outer region soil in the ith layer can be derived as:

 (14)

The shear stiffness atwhich is described by the shear stress due to harmonic displacement of a unit amplitude in the ith soil layer, can be expressed as:

   (15)

3.1.2 Solutions for inner region soil

In the inner region, the shear stress of the nth annular zone at any point within the ith soil layer can be written as:

     (16)

whereand denote the modified Bessel function of the second order of the first and second kinds, respectively.

The shear stiffness at is described by the shear stress due to harmonic displacement of a unit amplitude in the ith soil layer and can be expressed as:

  (17)

Then, Eq. (17) can be rewritten as:

      (18)

Similarly, the shear stiffness at can be obtained as:

 (19)

Substituting Eq. (18) into Eq. (19) yields:

 (20)

where

According to the impedance transfer method [18], the shear stiffness of the ith soil layer around the ith pile segment can be obtained as:

    (21)

where

3.1.3 Shear stiffness at interface of pile and pile surrounding soil

The shear stiffness of pile surrounding soil around pile segment is calculated by the impedance transfer method. Hence, the shear stiffness of the ith soil layer around the ith pile segment can be written as:

                               (22)

Combining with Eq. (11) and Eq. (22), the resistance f_i(z, t) can be obtained as:

   (23)

3.2 Solutions for tapered pile and fictitious soil pile

Combined with Eq. (10), substituting Eq. (23) into Eq. (6) and employing the Laplace transform on Eq. (6) (two-side) yield:

 (24)

Equation (25) is acquired by solving Eq. (24):

         (25)

The torsional impedance function was defined by MILITANO et al [29] and Eq. (9). By virtue of the impedance transfer method which was employed in Ref. [24], the torsional impedance function at the tapered pile head can be expressed as:

 (26)

The torsional impedance function of the tapered pile head can be decomposed into two parts, namely, the real part and imaginary part, as follows:

                        (27)

where K_k and C_k represent the dynamic stiffness and damping, respectively. The meanings of other parameters are the same as the corresponding parameters in Ref. [27].

4 Parametric study and discussion

The plane deformation assumption is usually adopted when it comes to investigate the stress diffusion characteristics of shallow foundation, that is, the stress diffusion angle remains unchanged as the depth increases. According to the code for design of dynamic machine foundation of China [30], the stress diffusion angle of shallow foundation does generally not exceed 30°. Considering the difference between shallow foundation and pile foundation, the cone angle of tapered fictitious soil pile can vary from 0° to 45° and can be set as β=0°, 15°, 30°, 45°.

In this paper, the basic parameters of the pile-soil system are set as follows: the length of pile is 6 m, and the other properties of pile and pile end soil are the same as the parameters in Ref. [27]. The mass density and material damping of pile surrounding soil are 2000 kg/m³ and 0, respectively; 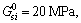GR=2.0,p=q=1.

4.1 Verification of solutions

In this section, the basic parameters of the pile-soil system are the same as those in Ref. [31]. Given β=0° and GR=1, the degenerate solution 1 yields. The degeneration of the present solution indicates that there is no stress diffusion effect in the pile end soil and the pile surrounding soil is homogeneous. Given β=0°, GR=1 and b_i→0, the degeneration solution indicates that the pile surrounding soil is simulated by plane strain model, and called degenerate solution 2. The curve of the degenerate solution 1 matches well with the degenerate solution 2, which reflects that the complex stiffness transfer model is suitable to simulate the pile surrounding soil. Meanwhile, as shown in Figure 2, the variation trend of the curves of the degenerate solution 2 is completely consistent with that of XIE’s solution [31], which verifies the rationality of the fictious soil pile model.

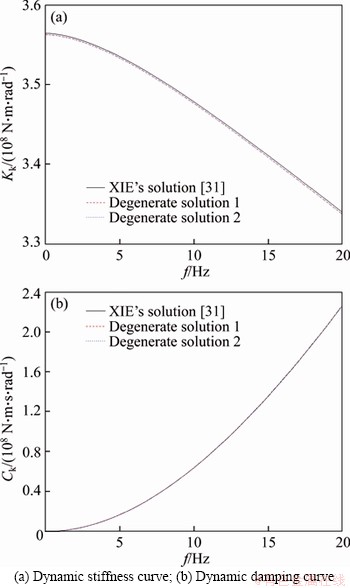
Figure 2 Comparison of the present solution and XIE’s [31] solution:

4.2 Influence of compaction effect of pile surrounding soil on torsional vibration characteristics of tapered pile

In this section, in order to study the influence of compaction of pile surrounding soil clearly, the value of β is set as β=0°, namely, there is no stress diffusion effect in the pile end soil.

4.2.1 Compaction degree of pile surrounding soil

In this section, GR is set as GR=1.0, 1.5, 2.0, 2.5, 3.0. GR=1.0 indicates that there is no compaction effect in the pile surrounding soil. Figure 3 displays the influence of compaction degree of pile surrounding soil on the torsional dynamic stiffness and damping of tapered pile with α=0°, 2°, 4°. It is obviously found that the torsional dynamic stiffness and damping of tapered pile head increase as the compaction degree of pile surrounding soil increases, and that increment gradually increases with the increase of the cone angle of tapered pile. Figure 4 describes the influence of compaction degree of pile surrounding soil on the torsional dynamic stiffness and damping of tapered pile with r₀=0.4, 0.5, 0.6 m. When the radius of tapered pile toe increases, it can be noted that the increment of torsional dynamic stiffness and damping caused by the compaction effect of pile surrounding soil increases gradually.

Figure 3 Influence of compaction degree of pile surrounding soil on torsional vibration characteristics of tapered pile with different cone angle:

The above phenomena indicate that the higher compaction degree of pile surrounding soil leads to the stronger seismic performance of pile-soil system, and when the cone angle and radius of tapered pile are bigger, the influence of compaction degree of pile surrounding soil on the torsional vibration characteristics of tapered pile is more obvious.

4.2.2 Compaction range of pile surrounding soil

In this section, b_i is set as b_i=0.1r_i⁰, 0.5r_i⁰, 1.0r_i⁰, 1.5r_i⁰, 2.0r_i⁰. Figure 5 illustrates the influence of the compaction range of pile surrounding soil on the torsional vibration characteristics of tapered pile with α=0°, 2°, 4°. The torsional dynamic stiffness and damping increase as the width of the inner region increases, and that increment decreases gradually. Moreover, when the cone angle of tapered pile increases, it is found that the increment of torsional dynamic stiffness and damping caused by the compaction range of pile surrounding soil increase.

Figure 4 Influence of compaction degree of pile surrounding soil on torsional vibration characteristics of tapered pile with different radius:

Figure 5 Influence of the compaction range of pile surrounding soil on torsional vibration characteristics of tapered pile with different cone angle:

Figure 6 depicts the influence of compaction range of pile surrounding soil on the torsional dynamic stiffness and damping of tapered pile with r₀=0.4, 0.5, 0.6 m. When the radius of tapered pile toe increases, the increment of torsional dynamic stiffness and damping caused by the compaction range of pile surrounding soil increase gradually.

The above results show that the bigger compaction range of pile surrounding soil leads to the stronger seismic performance of pile-soil system and there is a critical width of the inner region; when the compaction range is bigger than that value, the increase of the width of the inner region has little effect on the torsional vibration characteristics of tapered pile. Meanwhile, the larger the cone angle and radius are, the greater the influence of the soil compaction range on the torsional vibration characteristics of the tapered pile will be.

4.2.3 Compaction location of pile surrounding soil

In this section, the tapered pile and the pile surrounding soil are divided into three parts, namely bottom part, middle part and top part form pile toe to pile top, and the length values of those three parts are 1.8, 2.4 and 1.8 m, respectively.

First, given GR=1, which reflects that there is no compaction in the pile surrounding soil, and in the compaction area, GR changes as that in section 4.2.1. And then, three cases were taken into consideration: compaction effect exists in the bottom part, in the middle part, and in the top part, respectively. The corresponding curves shown in Figure 7 describe the influence of the compaction degree of pile surrounding soil on the torsional vibration characteristics of tapered pile with different location. When the compaction of pile surrounding soil exists at the top part, the influence of compaction degree is the most obvious. When the compaction of pile surrounding soil exists at the bottom part, the influence of compaction degree is the smallest.

Figure 6 Influence of compaction range of pile surrounding soil on torsional vibration characteristics of tapered pile with different radius:

Next, GR is still set as GR=1 and b_i→0. But in the compaction area, GR=2 and the width of inner region soil varies as that in section 4.2.2. Three different conditions are: compaction effect exists in the bottom part, in the middle part and in the top part, respectively. The influence of the compaction range of pile surrounding soil on the torsional vibration characteristics of tapered pile with different location is displayed in Figure 8, and the effect of the compaction range with different location is consistent with that described in Figure 7.

Figure 7 Influence of compaction degree of pile surrounding soil on torsional vibration characteristics of tapered pile with different location:

4.3 Influence of cone angle of tapered fictitious soil pile on torsional vibration characteristics of tapered pile

In this section, the influence of the stress diffusion effect of pile end soil on the torsional dynamic response of tapered pile in layered soil is discussed for different conditions, and the compaction degree and range of pile surrounding soil are set as GR=2 and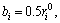 respectively, to make the stress diffusion effect of pile end soil obviously.

4.3.1 For different compaction degree of pile surrounding soil

Figure 9 describes that the influence of the stress diffusion effect of pile end soil on the torsional dynamic characteristics of tapered pile with different compaction degree of pile surrounding soil. The compaction degree of pile surrounding soil is set as GR=1, 2, 3. It is observed that as compaction degree of pile surrounding soil decreases, the influence of the cone angle of tapered fictitious soil pile on the torsional dynamic characteristics of tapered pile becomes more obvious. The above phenomena can be explained as follows: the pile-soil system is in a state of dynamic equilibrium subjected to dynamic load; the upper dynamic load is balanced by the soil resistances at the tapered pile side and tapered pile end. As compaction degree of pile surrounding soil increases, the soil resistance around the pile shaft increases and the soil resistance at the pile end decreases. When compaction degree of pile surrounding soil is big enough, the upper dynamic load is mainly balanced by soil resistance at the pile side, and the resistance at the pile end can be neglected. The smaller compaction degree of pile surrounding soil leads to obviously stress diffusion effect of pile end soil.

Figure 8 Influence of compaction range of pile surrounding soil on torsional vibration characteristics of tapered pile with different location:

Figure 9 Influence of cone angle of tapered fictitious soil pile on torsional vibration characteristics of tapered pile with different compaction degree of pile surrounding soil:

4.3.2 For different cone angle of tapered pile

Figure 10 displays the influence of the stress diffusion effect of pile end soil on the torsional dynamic characteristics of tapered pile with different cone angle. The cone angle of tapered pile is set as α=0°, 2°, 4°[32]. α=0° indicates that the pile is a uniform-section pile. It can be observed that as the cone angle of tapered pile decreases, of the stress diffusion effect of pile end soil on the torsional dynamic characteristics of tapered pile becomes more significant. The phenomenon may be due to the fact that, the existence of cone angle of tapered pile makes the soil resistance around the tapered pile shaft bigger than that of uniform-section pile. It is the same as that in section 4.3.1 that when the cone angle of tapered pile is big enough, the upper dynamic load is mainly balanced by the soil resistance around the pile shaft. Therefore, when the cone angle of tapered pile is set as α≤4° in this case, the diffusion effect in the pile end soil should be considered in pile design.

Figure 10 Influence of cone angle of tapered fictitious soil pile on torsional vibration characteristics of tapered pile head(α=0°, 2°, 4°):

4.3.3 For different radius of tapered pile end

Figure 11 shows the influence of the stress diffusion effect of pile end soil on the torsional dynamic characteristics of tapered pile with different radius of tapered pile end. The radius of tapered pile end is set as r₀=0.5, 0.7, 0.9 m. It can be observed that the influence of the cone angle of tapered fictitious soil pile on the torsional vibration characteristics of tapered pile head becomes more important with the increasing radius of tapered pile end. The result show that the bigger ratio of length to diameter leads to smaller stress diffusion effect in the pile end soil.

Figure 11 Influence of cone angle of tapered fictitious soil pile on torsional vibration characteristics of pile head (r₀=0.5, 0.7, 0.9 m):

4.3.4 For different length of tapered fictitious soil pile

Figures 12 and 13 represent the influence of the stress diffusion effect of pile end soil on the torsional vibration characteristics of tapered pile with different length of pile end soil. The length of tapered fictitious soil pile is set as H_s=2 m and H_s=10 m in Figures 12 and 13, respectively. It can be seen that the influence of the cone angle of tapered fictitious soil pile on the torsional vibration characteristics of tapered pile is getting smaller with the increasing fictitious soil pile length. The result indicates that the stress diffusion effect in pile end soil should be neglected when the length of pile end soil is big enough.

4.3.5 For different shear wave velocity of fictitious soil pile

Figure 14 depicts the influence of the stress diffusion effect of pile end soil on the torsional dynamic characteristics of tapered pile with the different shear wave velocity of tapered fictitious soil pile. The shear wave velocity is set as v_s=100, 200, 300 m/s [33]. It can be observed that the influence of the cone angle of tapered fictitious soil pile on the torsional vibration characteristics of tapered pile head becomes more obvious as the shear wave velocity of tapered fictitious soil pile increases. The result indicates that the stress diffusion effect in pile end soil should be considered when pile end soil property is better.

Figure 12 Influence of cone angle of tapered fictitious soil pile on torsional vibration characteristics of pile head (H_s=2 m):

Figure 13 Influence of cone angle of tapered fictitious soil pile on the torsional vibration characteristics of pile head (H_s=10 m):

Figure 14 Influence of cone angle of tapered fictitious soil pile on torsional vibration characteristics of pile head (v_s=100, 200, 300 m/s):

5 Conclusions

1) The stronger compaction degree and the wider compaction range of pile surrounding soil lead to the stronger seismic performance of pile-soil system. And the bigger radius and cone angle of tapered pile make those effects of compaction more significant.

2) The location of soil compaction also has an important influence on the torsional dynamic response of tapered pile. When the compaction of pile surrounding soil exists at the bottom part, the influence of compaction degree of pile surrounding soil on the torsional vibration characteristics of tapered pile is the smallest.

3) The smaller compaction degree of pile surrounding soil, cone angle of tapered pile, radius of tapered pile end, thickness and larger shear wave velocity of pile end soil lead to the more obvious stress diffusion effect of tapered pile end soil.

Contributors

GUAN Wen-jie conceived and conducted the study, and wrote the manuscript; WU Wen-bing conceived and guided the study, and reviewed the manuscript; JIANG Guo-shenng, CHIN Jian Leo and DENG Guo-dong read and reviewed the manuscript.

Conflict of interest

GUAN Wen-jie, WU Wen-bing, JIANG Guo-shenng, CHIN Jian Leo and DENG Guo-dong declare that they have no conflict of interest.

References

[1] WEI J Q, EL NAGGAR H M. Experimental study of axial behaviour of tapered piles [J]. Canadian Geotechnical Journal, 1998, 35(4): 641-654. DOI:10.1139/t98-033.

[2] LIU Jie, WANG Zhong-hai. Experimental study on bearing capacity of wedge pile [J]. Journal of Tianjian University, 2002, 35(2): 257-260. DOI: 10.3969/j.issn.0493-2137.2002. 02.031. (in Chinese)

[3] RYBNILKOV A M. Experimental investigations of bearing capacity of bored-cast-in-place tapered piles [J]. Soil Mechanics & Foundation Engineering, 1990, 27(2): 48-52. DOI: 10.1007/BF02306100.

[4] EL NAGGAR M H, ELKASABQY M, KHAN M K. Compression testing and analysis of drilled concrete tapered piles in cohesive-frictional soil [J]. Canadian Geotechnical Journal, 2008, 45(3): 377-392. DOI: 10.1139/T07-107.

[5] NAGGAR M H E, SAKR M. Evaluation of axial performance of tapered piles from centrifuge tests [J]. Canadian Geotechnical Journal, 2000, 37(6): 1295-1308. DOI: 10.1139/cgj-37-6-1295.

[6] ZHANG Ke-neng, HE Jie, LIU You-ping, LI Bing. Model experimental research on piling effects of static piling in soft clay ground with tapered pile [J]. Journal of Central South University(Science and Technology), 2012, 43(2): 638-643. http://en.cnki.com.cn/Article_en/CJFDTotal-ZNGD201202039.htm. (in Chinese)

[7] EL NAGGAR M H, WEI J Q. Axial capacity of tapered piles established from model tests [J]. Canadian Geotechnical Journal, 1999, 36(6): 1185-1194. DOI:10.1139/t99-076.

[8] LIU Jie, HE Jie, MIN Chang-qing. Contrast research of bearing behavior for composite foundation with tapered piles and cylindrical pile [J]. Rock & Soil Mechanics, 2010, 31(7): 2202-2206. DOI: 10.3969/j.issn.1000-7598.2010.07.030. (in Chinese)

[9] ZHOU Hang, KONG Gang-qiang, LIU Han-long. Study on pile sinking compaction effect of hydrostatic wedge pile using cavity expansion theory [J]. China Journal of Highway and Transport, 2014, 27(4): 24-30. http://zgglxb.chd.edu. cn/EN/Y2014/V27/I4/24. (in Chinese)

[10] KURIAN N P, SRINIVAS M S. Studies on the behaviour of axially loaded tapered piles by the finite element method [J]. International Journal for Numerical & Analytical Methods in Geomechanics, 2010, 19(12): 869-888. DOI: 10.1002/ nag.1610191204.

[11] YANG Gui, KONG Gan-qiang, CAO Zhao-hu, ZHOU Hang. Comparative numerical analysis on tapered pile and equal section pile driving process [J]. Journal of Railway Science and Engineering, 2016, 13(1): 40-45. DOI: 10.3969/j.issn.1672- 7029.2016.01.007. (in Chinese)

[12] XIONG L X, CHEN H J. A numerical study and simulation of vertical bearing performance of step-tapered pile under vertical and horizontal loads [J]. Indian Geotechnical Journal, 2020, 50(3): 383-409. DOI: 10.1007/s40098-019-00365-7.

[13] GHAZAVI M. Analysis of kinematic seismic response of tapered piles [J]. Geotechnical & Geological Engineering, 2007, 25(1): 37-44. DOI: 10.1007/s10706-006-0004-5.

[14] GHAZAVI M. Response of tapered piles to axial harmonic loading [J]. Revue Canadienne De Géotechnique, 2006, 45(11): 1622-1628. DOI: 10.1139/T08-073.

[15] DEHGHANPOOR A. Response of tapered piles under lateral harmonic vibrations [J]. International Journal of Geomate, 2012, 2(4): 261-265. DOI: 10.21660/2012.4.4c.

[16] CAI Yan-yan, YU Jin, ZHENG Chun-ting, QI Zhi-bo, SONG Bo-xue. Analytical solution for longitudinal dynamic complex impedance of tapered pile [J]. Chinese Journal of Geotechnical Engineering, 2011, 33(S2): 392-398. http://www.cnki.com.cn/Article/CJFDTotal-YTGC2011S2077.htm. (in Chinese)

[17] WU Wen-bing, WANG Kui-hua, WU Deng-hui, MA Bo-ning. Study of dynamic longitudinal impedance of tapered pile considering lateral inertial effect [J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 33(s2): 3618-3625. http:// www.cnki.com.cn/Article/CJFDTotal- YSLX2011S2034.htm. (in Chinese)

[18] WANG Kui-hua, GAO Liu, XIAO Si, WANG Ning. Dynamic characteristic of large diameter tapered pile considering vertical reaction of pile surrounding soil [J]. Rock and Soil Mechanics, 2016, 37(s2): 223-231. DOI: 10.16285/j.rsm. 2016.S2.027. (in Chinese)

[19] WU W B, JIANG G S, DOU B, LEO J C. Vertical dynamic impedance of tapered pile considering compacting effect [J]. Mathematical Problems in Engineering, 2013, 2013:304856. DOI: 10.1155/2013/304856.

[20] GAO L, WANG K H, XIAO S, WU J T, WANG N. Vertical impedance of tapered piles considering the vertical reaction of surrounding soil and construction disturbance [J]. Marine Georesources & Geotechnology, 2017, 35(8): 1068-1076. DOI: 10.1080/ 1064119X.2017.1285379.

[21] YANG Dong-ying, WANG Kui-hua. Vertical vibration of pile based on fictitious soil-pile model in inhomogeneous soil [J]. Journal of Zhejiang University (Engineering Science), 2010, 44(10): 2021-2028. DOI: 10.3785/ j.issn.1008-973X.2010.10.030. (in Chinese)

[22] WU W B, WANG K H, MA S J, LEO C J. Longitudinal dynamic response of pile in layered soil based on virtual soil pile model [J]. Journal of Central South University, 2012, 19(7): 1999-2007. DOI: 10.1007/s11771-012-1237-5.

[23] WU W B, LIU H, EL NAGGAR M H, MEI G X, JIANG G S. Torsional dynamic response of a pile embedded in layered soil based on the fictitious soil pile model [J]. Computers and Geotechnics, 2016, 80: 190-198. DOI: 10.1016/j.compgeo. 2016.06.013.

[24] WANG Kui-hua, WANG Ning, LIU Kai, WU Wen-bing. Longitudinal vibration of piles in 3D axisymmetric soil based on fictitious soil pile method [J]. Chinese Journal of Geotechnical Engineering, 2012, 34(5): 885-892. http:// www.cnki.com.cn/Article/CJFDTotal-YTGC201205018.htm. (in Chinese)

[25] CUI Chun-yi, MENG Kun, LIANG Zhi-mneg, XU Cheng-shun, YANG Gang. Longitudinal vibration characteristics analysis on floating pile in frequency domain based on virtual saturated soil pile model [J]. Journal of Southeast University (Natural Science Edition), 2019, 49(2): 356-361. DOI: 10.3969/j.issn.1001-0505.2019.02.022. (in Chinese)

[26] WANG Kui-hua, LIU Kai, WU Wen-bing, WANG Ning. Effect of cone angle on axial dynamic response of fictitious soil pile [J]. Engineering Mechanics, 2011, 28(9): 129-136. DOI: 10.1111/j.1759-6831.2010.00113.x. (in Chinese)

[27] GUAN W J, WU W B, JIANG G S, LEO J C, LIU H. Torsional vibration solution of tapered pile considering stress diffusion effect of pile end soil [J]. Earth and Environmental Science, 2019, 304: 032095. DOI: 10.1088/1755-1315/ 304/3/032095.

[28] EL NAGGAR M H. Vertical and torsional soil reactions for radially inhomogeneous soil layer [J]. Structural Engineering & Mechanics, 2002, 10(4): 299-312. DOI: 10.12989/sem. 2000.10.4.299.

[29] MILITANO G, RAJAPAKSE R K N D. Dynamic response of a pile in a multi-layered soil to transient torsional and axial loading [J]. Geotechnique, 1999, 49(1): 91-109. DOI: 10.1680/geot.1999.49.1.91.

[30] Ministry of Housing and Urban-Rural Development of China. GB50040-96. Code for design of dynamic machine foundation [S]. Beijing: China Architecture & Building Press, 1997. https://www.renrendoc.com/p-17043364.html. (in Chinese)

[31] XIE B H. Vertical and torsional vibration theory of tapered pile embedded in layered soil and its application [D]. Wuhan: China University of Geosciences (Wuhan), 2014. https:// kns.cnki.net/kns/brief/default_result.aspx. (in Chinese)

[32] LIU Y, ZHANG K N, LIU C, HE J. Test on enhancement coefficient of lateral resistance about CFG micro pile [J]. Journal of Central South University, 2017, 48(1): 184-190. DOI: 10.11817/j.issn.1672-7207.2017.01.026.

[33] WANG Xiao-ning, ZHANG Ming-yi, ZHU Lei, WANG Yong-hong, WANG Jing-jing. In-situ test and fem analysis on bearing characters of rock-socketed short pile for highly weathered granite [J]. Journal of Central South University (Science and Techmology), 2017, 48(2): 512-524. DOI: 10.11817/j.issn.1672-7207.2017.02.032. (in Chinese)

(Edited by YANG Hua)

中文导读

考虑挤土效应和应力扩散效应时楔形桩的扭转动力响应

摘要：同时考虑桩侧土挤土效应及桩端土应力扩散效应，研究了楔形桩的扭转振动特性。采用复刚度传模型和锥形虚土桩模型分别模拟桩侧土的挤土效和桩端土的应力扩散。通过Laplace变换技术和阻抗传递法求解出楔形桩顶部的扭转复阻抗解析解。采用参数分析法分析了本文解的合理性和桩-土性质对成层土中楔形桩扭转动力特性的影响。研究结果表明：桩侧土体的挤土效应及桩端土的应力扩散效应均对楔形桩的扭转振动特性均有明显影响，因此，在桩基动力设计中应考虑以上两种因素的影响。

关键词：楔形桩；挤土效应；应力扩散效应；复刚度传递模型；锥形虚土桩模型

Foundation item: Projects(51578164, 51678547, 51878634, 51878185, 41807262) supported by the National Natural Science Foundation of China

Received date: 2019-08-25; Accepted date: 2020-09-21

Corresponding author: WU Wen-bing, PhD, Professor; Tel: +86-15927208466; E-mail: zjuwwb1126@163.com; ORCID: https://orcid. org/0000-0001-5473-1560