CaSO4-Ca(OH)2-H2O体系相平衡
来源期刊：中国有色金属学报(英文版)2012年第6期
论文作者：王云燕 彭小玉 柴立元 舒余德
文章页码：1478 - 1485
关键词：CaSO4-Ca(OH)2-H2O体系；相平衡；相图；废水
Key words：CaSO4-Ca(OH)2-H2O system; phase equilibrium; phase diagram; wastewater
摘 要：为了给含高浓度硫酸根离子的重金属废水中和法处理提供理论指导，并更好地理解硫酸钙结垢的形成机制，采用Pitzer电解质溶液理论计算298.15 K时CaSO4-Ca(OH)2-H2O三元体系的溶解度，并采用光学法结合XRD测试技术测定该体系的等温平衡溶解度，采用计算和实验方法分别绘制相图。研究了5个区域的物理定义及特征点、线所表达的物理规律，并分析了各区域SO42-浓度对中和水解过程pH调节的影响。中和水解过程pH值的调节取决于体系中SO42-离子的浓度。Ca(OH)2与CaSO4·2H2O在水中溶解时，相互影响的规律体现在相图中的点、线及面上。
Abstract: In order to provide the theoretical guidance for applying the neutralization method to treatment of heavy metals wastewater with high concentration of sulfate, and to better understand the mechanism of calcium sulfate scale formation, the equilibrium solubility data of CaSO4-Ca(OH)2-H2O system at 298.15 K were theoretically calculated via the Pitzer semi-empirical ion-interaction theory, and determined experimentally by the optical method combining with X-ray diffractometry, and the calculated and determined phase diagrams of CaSO4-Ca(OH)2-H2O system were plotted and compared. Physical definition of each area was studied, and the physical law of characteristic point and line was explained in detail. Adjusting the pH value of neutralization-hydrolysis solution depended on the SO42- concentration in the system. And interaction characteristics between the solubilities of CaSO4(s) and Ca(OH)2(s) were found out.
Trans. Nonferrous Met. Soc. China 22(2012) 1478-1485
WANG Yun-yan1, PENG Xiao-yu1,2, CHAI Li-yuan1, SHU Yu-de1
1. School of Metallurgical Science and Engineering, Central South University, Changsha 410083, China;
2. Environmental Monitoring Central Station of Hunan Province, Changsha 410014, China
Received 20 May 2011; accepted 13 October 2011
Abstract: In order to provide the theoretical guidance for applying the neutralization method to treatment of heavy metals wastewater with high concentration of sulfate, and to better understand the mechanism of calcium sulfate scale formation, the equilibrium solubility data of CaSO4-Ca(OH)2-H2O system at 298.15 K were theoretically calculated via the Pitzer semi-empirical ion-interaction theory, and determined experimentally by the optical method combining with X-ray diffractometry, and the calculated and determined phase diagrams of CaSO4-Ca(OH)2-H2O system were plotted and compared. Physical definition of each area was studied, and the physical law of characteristic point and line was explained in detail. Adjusting the pH value of neutralization-hydrolysis solution depended on the SO42- concentration in the system. And interaction characteristics between the solubilities of CaSO4(s) and Ca(OH)2(s) were found out.
Key words: CaSO4-Ca(OH)2-H2O system; phase equilibrium; phase diagram; wastewater
1 Introduction
Large amounts of sulfate wastewater containing heavy metals were discharged annually for the common use of sulfate system in industries, such as electroplating, steel pickling, mining, nonferrous smelting, sulphuric acid production and alkali making [1-4]. Heavy metals belong to persistent pollutants in environment because of their high toxicity. It was considered that sulfate in water would result in a series of problems. For example, water resistance would be decreased, pipeline would be eroded directly, and recycle of sulfate reducing bacteria (SRB) and spread of biology would be influenced indirectly. Higher concentration of sulfate ion in water would lead to soil salinization. Therefore, more and more attentions have been paid to control the content of sulfate ion in water.
Nowadays, lime-milk neutralization process is regarded as a simple, cheap, efficient and sustainable technique, by which heavy metals are removed as hydroxide [5-9]. However, effluent leads to a series of problems. Purification and reutilization of industrial wastewater are limited for high concentration of calcium ion and sulfate ion [10]. Commonly, 
concentration in effluent reaches up to 2000 mg/L, which result in the formation of calcium sulfate scales and pipeline erosion.
Practically, effluent from lime-milk neutralization and precipitation process with high concentration (1800 mg/L) is used to prepare lime milk and then to treat heavy metal wastewater. It was found that pH value of wastewater treatment system could not be adjusted to the expected value, which brought side-effect to removal of heavy metals and increased lime-milk consumption. Thus, the reason of those phenomena should be explained from the view point of thermodynamics [11]. In addition, the related parameters, such as activity, diffusion coefficient and solvent activity, are of practical interest in many environmental and industrial processes. MA et al [12] pointed out that it was feasible to precipitate CaSO4 from Na2SO4-H2O system in the presence of CaO. Therefore, it is necessary to calculate, determine and analyze the phase diagram of CaSO4-Ca(OH)2-H2O system for properly adding Ca(OH)2 and removal of from wastewater.
At present, the most widely used calculation model for aqueous systems over a relatively high range of ionic strength is the Pitzer theory, which allows successful fitting of thermodynamic properties [13]. Pitzer approach permits a description of thermodynamic properties of multi-component systems in terms of both the mixed and the individual parameters of the involved components.
In this work, based on the Pitzer semi-empirical ion-interaction theory for multi-components system, the solubilities of CaSO4(s) and Ca(OH)2(s) in CaSO4- Ca(OH)2-H2O system are calculated, and then calculated phase diagram is plotted. In order to verify the calculation and offer precise guidance for effective removal of sulfate ion and proper addition of Ca(OH)2 in heavy metals wastewater with high concentration, optical method is adopted to determine equilibrium solubility, and the determined phase diagram of CaSO4- Ca(OH)2-H2O system is plotted.
2 Calculation of phase diagrams of CaSO4- Ca(OH)2-H2O system
2.1 Activity coefficient model
The Pitzer activity coefficient model was applied in the calculation process, and the related equations are presented in the following.
The dissociation equilibrium is
(1)
(2)
where Ksp1 is the dissociation constant of CaSO4; Ksp2 is the dissociation constant of Ca(OH)2; 
is the average activity coefficients of CaSO4;
is the average activity coefficients of Ca(OH)2; [Ca2+], [] and [OH-] are the equilibrium concentrations of Ca2+, and OH-, respectively.
The charge conservation is
(3)
where 
and 
are the molality in mol/kg of Ca2+, and OH-, respectively.
The activity coefficient model for single-component points is
(4)
The activity coefficient model for multi-component points is
(5)
where MX represents electrolyte; c represents cations including M ion; a represents anions including X ion; zM is the charge number of cation M; zX is the charge number of anion X; vM is the amount of cation M; vX is the amount of anion X; v is the total amount of M and X which are ionized from electrolyte MX, v=vM+vX; mc and ma represent the molality of cations c and anions a, respectively, mol/kg; θ is the Pitzer mixing parameter between two different ions with the same charge; ψ is the mixing parameter among the three ions with different charges.
where I is the ionic strength.
For water, AФ=0.392 at 25 ℃, 
, 
.
For electrolyte containing at least one univalent ion at 25 ℃,
For electrolyte with bivalent-bivalent style at 25 ℃,
where 
, 
and 
are character parameters of electrolyte.
2.2 Criterion for solid-liquid equilibrium
According to the chemical equilibrium principle, the dissolution equilibrium of any compound can be obtained as compound saturated in solution. The dissolution equilibrium for a given compounds (e.g. 
) is expressed as follows:
(6)
(7)
where 
and 
represent the activities of cation and anion, respectively, mol/L; K is the dissolution equilibrium constant of compound.
The solubility product of CaSO4 is 9.1×10-6 and that of Ca(OH)2 is 5.5×10-6 [12].
2.3 Calculation procedure
According to the Pitzer model of aqueous electrolytes, the mixing parameters were divided into two types: θ(i, j), the parameter of two ions with the same charge, and ψ(i, j, k), the parameter of two ions with the same charge and an ion with opposite charge. i, j denote two definite ions with the same charge.
Calculation procedure can be summarized as follows: 1) Choice of Pitzer parameters of single electrolyte 
, 
, 
and CΦ; 2) Choice of Pitzer ternary parameters {θOH-SO4 and ψCa-OH-SO4}; 3) Calculation of solubility isotherms of three-component solutions. The Pitzer model allows the determination of activity coefficients in saturated and unsaturated electrolyte solutions with an accuracy of 2%-6% [24].
The parameters of single-electrolyte and Pitzer mixing parameters of multi-component systems were determined by many researchers. The values of the Pitzer parameters for CaSO4(aq) and Ca(OH)2(aq), the Pitzer mixing parameters for CaSO4-Ca(OH)2-H2O system and the parameters of single-electrolyte are listed in Table 1 [16].
Table 1 Pitzer parameters of electrolyte and mixing parameters
In CaSO4 saturation area, the product P of ion activity is calculated by:
For Ca(OH)2 saturation area, the product P of ion activity is calculated by:
The values of CΦ for CaSO4 (aq) and Ca(OH)2 (aq) can be neglected because CaSO4 and Ca(OH)2 are compounds with a small solubility according to NIU et al [16].
The calculation procedure is complex that it should be completed through program shown in Fig. 1.
Fig. 1 Calculation procedure of activity coefficient for CaSO4-Ca(OH)2-H2O system
2.4 Calculation results
The calculated activity coefficients of compounds for CaSO4-Ca(OH)2-H2O system are listed in Table 2.
Table 2 Calculated solubility in CaSO4-Ca(OH)2-H2O system
Because the solubilities of CaSO4 and Ca(OH)2 are very small, the values of the calculated solubility are magnified by one hundred times to plot the phase diagram of CaSO4-Ca(OH)2-H2O system at 298.15 K on the top of the triangle (Fig. 2).
Fig. 2 Calculated phase diagram of CaSO4-Ca(OH)2-H2O system in triangle coordinate system
According to the phase rule (F=C-P+2) [16], the CaSO4-Ca(OH)2-H2O system is a ternary system, so the independent component number C is 3. The degree of freedom F is 4 when the system is in single-phase area, which indicates that there are four independent variables, namely temperature, pressure and relative content of two components. If the pressure keeps constantly at 101.325 kPa and temperature is controlled at 298.15 K, there are just two independent variables which are the relative contents of two components, so the rectangular coordinate can be utilized to express the phase equilibrium of CaSO4-Ca(OH)2-H2O system. The original point of coordinate represents pure H2O, the infinite area of abscissa represents pure CaSO4, and the infinite area of ordinate denotes pure Ca(OH)2. The calculated phase diagram of CaSO4-Ca(OH)2-H2O system is shown in Fig. 3.
Fig. 3 Calculated phase diagram of CaSO4-Ca(OH)2-H2O system in rectangular coordinate system
The calculation shows that only the crystallization of simple salts Ca(OH)2 and CaSO4·2H2O is established in CaSO4-Ca(OH)2-H2O system. The solubility of single Ca(OH)2 is 1.841 g/L and the solubility of single CaSO4 is 2.080 g/L. The composition of invariant point is 0.1743% Ca(OH)2 and 0.1385% CaSO4.
3 Experimental
3.1 Chemical reagents
The standardized solution of NaOH (0.02 mol/L) was prepared from analytical grade sodium hydroxide, EDTA standard solution was prepared from analytical grade EDTA-2Na and HCl standard solution (0.1 mol/L) was prepared from concentrated hydrochloric acid in all experiments. Besides, phenolphthalein indicator (1%), calcium carboxylic acid indicator (1%) and benzidine hydrochloride solution (8 g/L) were prepared.
3.2 Experimental procedure
3.2.1 Solution preparation
The accurately weighed calcium hydroxide and concentrated sulfuric acid were put into the iodine measuring flask with the volume of 500 mL, then 500 mL ultra pure water was added to configure a series of system points for CaSO4-Ca(OH)2-H2O system.
3.2.2 Experiment and sampling
The prepared samples were put into water and stirred by a magnetic stirrer. The temperature of the solution was controlled at 298.15 K. When the dissolution reached equilibrium, the solution was stood for 30 min, then the samples of supernatant solution were taken out for analyzing. The precipitate in the solution was detected after filtration and drying [14].
3.3 Principle of solution preparation
According to the calculated results, the saturated solubility of Ca(OH)2 is 1.842 g/L and that of CaSO4 is 2.080 g/L. It is necessary to ensure one of the components excessive for two-phase equilibrium points and two components excessive for co-saturated point [15]. Therefore, the Ca(OH)2 concentration is greater than 1.842 g/L for crystallization area of Ca(OH)2, then the amounts of Ca(OH)2 and ultrapure water are fixed, while the amount of CaSO4 is changed to prepare a series of system points. Similarly, the CaSO4 concentration is higher than 2.080 g/L for crystallization area of CaSO4, then the amounts of CaSO4 and ultrapure water are fixed, while the amount of Ca(OH)2 is altered to prepare a series of system points. The concentrations of Ca(OH)2 and CaSO4 are higher than 1.842 g/L and 2.080 g/L respectively for co-saturated point.
In addition, the active CaSO4 is generated from reaction between Ca(OH)2 and H2SO4 in order to shorten the time of dissolution equilibrium.
3.4 Original composition of solution
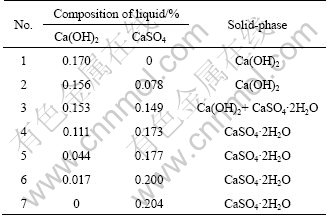
The original composition of solution is listed in Table 3. The samples No.1-3 are composed of fixed dosage of 2.0 g Ca(OH)2 and 500 mL ultrapure water, and altered dosage of CaSO4 of 0, 0.4 and 0.8 g, respectively. The samples No.4-7 are composed of fixed dosage of 3.0 g CaSO4 and 500 mL ultrapure water, and varied dosage of Ca(OH)2 of 0.5, 0.3, 0.1 and 0 g, respectively.
Table 3 Original composition of prepared solution
3.5 Determination of solid-phase composition
The X-ray diffraction (XRD, Rigaku D/max 2550VB+, Japan) was used to detect the solid phase from the solution. The composition of precipitate was confirmed by comparing with the standard patterns [16-18].
3.6 Determination of equilibrium time
The concentrations of calcium ion, sulfate ion and hydroxyl ion were determined at interval of 24 h, and the system was considered to reach equilibrium when the difference of concentration was less than 0.2% for the successive determination [19].
3.7 Determination of ion concentration in equilibrium liquid-phase composition
The sulfate ion, calcium ion and hydroxyl ion were determined by acid-base titration [20,21], sodium EDTA titration [22,23] and HCl titration, respectively.
Each sample was tested in triplicate and the mean value was presented.
4 Results
4.1 Phase composition of precipitates at equilibrium point
The XRD patterns of equilibrium solid-phase for samples No.1-7 are shown in Fig. 4.
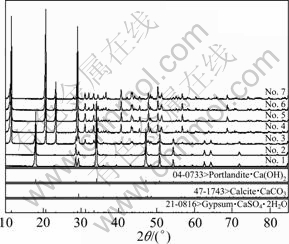
Fig. 4 XRD patterns of equilibrium solid-phase
Comparing with the standard XRD patterns, the main precipitate of samples No.1 and 2 is Ca(OH)2, that of No. 3 is Ca(OH)2 and CaSO4·2H2O, and that of No. 4-7 is CaSO4·2H2O. Besides, there is a few CaCO3 in samples No. 1, 2 and 3, which results from the unavoidable reaction between a little carbon dioxide and Ca(OH)2. The determined equilibrium solubility of CaSO4-Ca(OH)2-H2O system is listed in Table 4.
Table 4 Determined phase equilibrium data of CaSO4- Ca(OH)2-H2O system
4.2 Determined phase diagram of CaSO4-Ca(OH)2- H2O system
According to the phase equilibrium data, the phase diagram of CaSO4-Ca(OH)2-H2O system in triangle was determined as shown in Fig. 5. On the top of the triangle, the values of the calculated solubility was magnified by one hundred times to plot the phase diagram owning to the minor solubility of CaSO4 and Ca(OH)2.
When the rectangular coordinate was used, the phase diagram of CaSO4-Ca(OH)2-H2O system (shown in Fig. 6) was obtained from experimental results listed in Table 4.
There is a liquid area ABCD in Fig. 6, which represents the area of unsaturated solution, in which the concentrations of both CaSO4 and Ca(OH)2 are in instauration, and the scope of this area is restricted by the solubility curves BC and CD. The area EDCF is a two-phase area that is composed of solid Ca(OH)2 and solution containing CaSO4 and Ca(OH)2, in which solid Ca(OH)2 is in equilibrium with solution. Line CD represents the relationship between CaSO4 concentration and Ca(OH)2 solubility. Area BCG is a two-phase area that is composed of solid CaSO4·2H2O and solution containing CaSO4 and Ca(OH)2, in which solid CaSO4·2H2O is in equilibrium with solution. Line BC represents the relationship between Ca(OH)2 concentration and CaSO4 solubility. Point C is a three-phase equilibrium point, named co-saturated point, where the concentrations of Ca(OH)2 and CaSO4 are all saturated. The degree of freedom is zero for co-saturated point, so the composition of each equilibrium phase is constant as evaporation at this point, but the relative amount of each species would change. Area FCGH is a three-phase area composed of co-saturated solution, solid Ca(OH)2 and solid CaSO4·2H2O, where the co-saturated solution is in equilibrium with both solid Ca(OH)2 and solid CaSO4·2H2O. The right area of line HG is the mixed area of three solid phases such as Ca(OH)2, CaSO4·2H2O and CaSO4.
Fig. 5 Determined phase diagram of CaSO4-Ca(OH)2-H2O system in triangle coordinate system
Fig. 6 Determined phase diagram of CaSO4-Ca(OH)2-H2O system in rectangular coordinate system
5 Discussion
The determined phase diagrams of Figs. 5 and 6 are in accordance with the calculated phase diagrams of Figs. 2 and 3, which proves that the calculation procedure is correct and the diagrams could be used to interpret and guide reuse of water containing sulfate ion.
The calculation results show that only the crystallization of simple salts Ca(OH)2 and CaSO4·2H2O is established in ternary CaSO4-Ca(OH)2-H2O system. The solubility of single Ca(OH)2 is 1.841 g/L and that of single CaSO4 is 2.080 g/L. The composition of invariant point is 0.1743% Ca(OH)2 and 0.1385% CaSO4.
The diagrams in the rectangular coordinate (Figs. 3 and 6) consist of five areas: one single-phase area, three two-phase areas and one three-phase area. The single-phase area is an unsaturated solution area, and three two-phase areas include crystallization area of CaSO4·2H2O, crystallization area of Ca(OH)2 and coexisting area of CaSO4·2H2O and Ca(OH)2. Interaction characteristics between the solubility of CaSO4(s) and Ca(OH)2(s) are also obtained, which can be used to guide for treatment and reuse of water containing sulfate ion and calcium ion.
The area FCGH is a three-phase equilibrium area (Fig. 6). Because the degree of freedom for CaSO4- Ca(OH)2-H2O system is zero, the equilibrium composition of liquid is constant and decided by co-saturated point C. So pH value of the neutralization-hydrolysis solution can not be adjusted when concentration of neutralization-hydrolysis solution is in area FCGH, and pH value is constant and decided by the composition of co-saturated solution for point C. The area BCG is a two-phase equilibrium area. Because the degree of freedom is one, the equilibrium composition of liquid is decided by curve BC. Adjustment of pH value of neutralization-hydrolysis solution is decided by line BC when concentration is in the area BCG. The area ABCD is a single-phase equilibrium area. The degree of freedom is two, so pH value of neutralization-hydrolysis solution can be adjusted arbitrarily when concentration is in the area ABCD, and the maximum pH value is decided by curve CD. The area EDCF is a two-phase equilibrium area. The degree of freedom is one, and the equilibrium composition of liquid is decided by curve CD. Adjustment of pH value of neutralization-hydrolysis solution is decided by line CD when concentration of neutralization-hydrolysis solution is in the area EDCF.
6 Conclusions
1) Adjusting the pH value of neutralization- hydrolysis solution depends on concentration of neutralization-hydrolysis solution. The pH value cannot be adjusted as concentration is in the three-phase equilibrium area, and pH value is constant and decided by the composition of co-saturated solution. Adjustment of pH value is decided by the solubility curve of CaSO4 as concentration is in the two-phase equilibrium area of liquor and CaSO4·2H2O. pH value can be adjusted arbitrarily as concentration is in the single-phase equilibrium area, and the maximum pH value is decided by the solubility curve of Ca(OH)2. Adjustment of pH value is decided by the solubility curve of Ca(OH)2 as concentration is in the two-phase equilibrium area of liquor and Ca(OH)2.
2) The interaction characteristics between the solubilities of CaSO4(s) and Ca(OH)2(s) was embodied in the points, lines and areas of phase diagram, which can provide some theoretical guidance for application of neutralization method in treating heavy metal wastewater containing high concentration of sulfate ion and purification of industrial reused water.
References
[1] WANG Guang-xing, GUO Lian-cai. Effective method of control 
concentration [J]. China Chlor-Alkali, 2006(1): 6-7. (in Chinese)
[2] GU Min, FU Bian-hong, YANG Ming-li. Progress in electroplating of copper from sulfate bath [J]. Journal of Materials Protection, 2006(1): 44-48. (in Chinese)
[3] WANG Hua, ZENG Zhen-ou, ZHAO Guo-peng, HU Yao-hong. Study on the process of thick chromium plating and coating performance from trivalent chromium sulfate electrolyte [J]. Electroplating & Finishing, 2007, 26(6): 13-17. (in Chinese)
[4] WU Hui-min, KANG Jian-qiang, ZUO Zheng-zhong, LIANG Guo-dong, ZHAO Guo-peng. Study of electroplating with thrivalent chromium in sulfate system [J]. Journal of Wuhan University, 2004, 50(2): 187-191. (in Chinese)
[5] WANG Xin-wen. Survey on treatment of acidic wastewater from large smelters in china [J]. Mining and Metallurgy, 2000, 9(2): 84-88. (in Chinese)
[6] LI Ying. Theory and practice of heavy metal industrial wastewater treatment recycle [J]. Hunan Nonferrous Metals, 2003, 19(2): 46-48. (in Chinese)
[7] LI Wei-hong. Study on the reuse of heavy metal wastewater for non-ferrous smeltery [J]. Industrial Safety and Environmental Protection, 2002, 28(11): 14-17. (in Chinese)
[8] YIN Zhi-wei. Study on recycle technology for acidic wastewater containing heavy metal ions treated by lime-base process [J]. Mining and Metallurgy, 2002, 11(2): 77-80. (in Chinese)
[9] WANG Liang-zhu. Purification and reutilization of industrial waste water [J]. Metallurgical Power, 2003(3): 58-60. (in Chinese)
[10] WANG Xiang-ping. Application of acidic waste water after treatment in non-ferrous hydrometallurgical enterprise [J]. Energy Saving of Non-ferrous Metallurgy, 2003, 20(4): 34-36. (in Chinese)
[11] DEYHIMI F, KARIMZADEH Z, SALAMAT-AHANGARI R. Thermodynamic investigation of a ternary mixed electrolyte (NaCl/MgCl2/H2O) system using Na+ solvent polymeric membrane ion-selective electrode [J]. Fluid Phase Equilibria, 2007, 254(1-2): 18-27.
[12] MA Hong-wen, WANG Ying-bin, WANG Fang, SU Shuang-qing, LIU Hao, PENG Hui, YU Zi-jian. Chemical equilibrium in silicate system: Part II, reaction thermodynamics [J]. Geoscience, 2006, 20(3): 386-398. (in Chinese)
[13] DEYHIMI F, SALAMAT-AHANGARI R. Potentiometric investigation of the thermodynamic properties of the ternary mixed (NH4Cl+CaCl2+H2O) electrolyte system [J]. Fluid Phase Equilibria, 2008, 264(1-2): 113-121.
[14] GOUNDALI B EL, KADDAMI M. The ternary system H2O-Fe(NO3)3-Co(NO3)2 isotherms 0 and 15 ℃ [J]. Fluid Phase Equilibria, 2007, 260(2): 295-299.
[15] LIANG Bao-min. Theory for phase diagram of salt-water system and its applications [M]. Beijing: Light Industry Press, 1986: 183-195. (in Chinese)
[16] NIU Zi-de, CHENG Fang-qin, LI Bao-cun. Phase diagram of salt-water systems and its application [M]. Tianjin: Tianjin University Press, 2002. (in Chinese)
[17] DONG Y Z, ZHAO Y M, FU P, ZHOU H, HOU X M. Phase relations of Li2O-FeO-B2O3 ternary system and electrochemical properties of LiFeBO3 compound [J]. Journal of Alloys and Compounds, 2008, 461(1-2): 585-590.
[18] BALUN J, ELENO L, INDEN G. Phase equilibria in the Fe-Rh-Ti system I: Experimental results [J]. Intermetallics, 2007, 15(9): 1237-1247.
[19] WANG Yan-jie, TIAN Li-nan. Determination of the phase diagram of the K+, NH4+//Cl-, -H2O system [J]. Journal of Wuhan Institute of Chemical Technology, 2005, 27(1): 11-14. (in Chinese)
[20] WANG Rui-bin. Determination of sulfate radical in intrinsic salt by the benzidine sulfate deposition andalkali titration [J]. Metallurgical Analysis, 2007, 27(4): 62-64. (in Chinese)
[21] WANG Jian-guo, WANG Rui-bin. Study on the determination of sulfate in laboratory wastewater by benzidine hydrochloride separate and acid-base titration [J]. Science Technology and Engineering, 2007, 17(7): 4438-4440. (in Chinese)
[22] WU Ze-hui. Determination of the calcium ion in the rain by complex titration with the indicator ca-reagent carboxylic acid sodium [J]. Journal of Analytical Science, 1999, 15(6): 498-501. (in Chinese)
[23] DENG Chao, WANG Xi-jun, YU Quan. Definition of titration ends of calcium cation contents by using EDTA method [J]. Forest Engineering, 2000, 16(2): 17-18.( in Chinese)
[24] CHRISTOV C,IVANOVA K, VELIKOVA S, TANEV S. Thermodynamic study of aqueous sodium and potassium chloride and chromate systems at the temperature 298.15 K [J]. The Journal of Chemical Thermodynamics, 2002, 34(7): 987-994.
王云燕 1，彭小玉1,2，柴立元1，舒余德1
1. 中南大学 冶金科学与工程学院，长沙 410083；
2. 湖南省环境监测中心站，长沙 410014
摘 要：为了给含高浓度硫酸根离子的重金属废水中和法处理提供理论指导，并更好地理解硫酸钙结垢的形成机制，采用Pitzer电解质溶液理论计算298.15 K时 CaSO4-Ca(OH)2-H2O三元体系的溶解度，并采用光学法结合XRD测试技术测定该体系的等温平衡溶解度，采用计算和实验方法分别绘制相图。研究了5个区域的物理定义及特征点、线所表达的物理规律，并分析了各区域
浓度对中和水解过程pH调节的影响。中和水解过程pH值的调节取决于体系中离子的浓度。Ca(OH)2与CaSO4·2H2O在水中溶解时，相互影响的规律体现在相图中的点、线及面上。
关键词：CaSO4-Ca(OH)2-H2O体系；相平衡；相图；废水
(Edited by YUAN Sai-qian)
Foundation item: Project (50925417) supported by the Funds for Distinguished Young Scientists of China; Project (50830301) supported by the National Natural Science Foundation of China; Project (2009ZX07212-001-01) supported by Major Science and Technology Program for Water Pollution Control and Treatment
Corresponding author: CHAI Li-yuan; Tel: +86-731-88836921; Fax: +86-731-88710171; E-mail: lychai@csu.edu.cn
DOI: 10.1016/S1003-6326(11)61344-7
