Permeability modeling of self-healing due to calcium carbonate precipitation in cement-based materials with mineral additives
来源期刊:中南大学学报(英文版)2019年第3期
论文作者:蒋正武 袁政成 陈庆
文章页码:567 - 576
Key words:cement-based material; self-healing; mineral additive; calcium carbonate; model
Abstract: The permeability modeling of self-healing due to calcium carbonate precipitation in cement-based materials with mineral additives was studied in this work. The parameters of calcium carbonate precipitation during self-healing were simulated. A permeability modeling of self-healing, combined with numerical simulation of calcium carbonate formation, was proposed based on the modified Poiseuille flow model. Moreover, the percentage of calcium carbonate in healing products was measured by TG-DTA. The simulated results show that self-healing can be dramatically promoted with the increase of pH and Ca2+ concentration. The calculated result of permeability is consistent with that measured for cracks appearing in middle or later stages of self-healing, it indicates that this model can be used to predict the self-healing rate to some extent. In addition, TG-DTA results show that the percentage of calcium carbonate in healing products is higher for mortar with only chemical expansion additives or cracks appearing in the later stage, which can more accurately predict the self-healing rate for the model.
Cite this article as: YUAN Zheng-cheng, JIANG Zheng-wu, CHEN Qing. Permeability modeling of self-healing due to calcium carbonate precipitation in cement-based materials with mineral additives [J]. Journal of Central South University, 2019, 26(3): 567–576. DOI: https://doi.org/10.1007/s11771-019-4028-4.

J. Cent. South Univ. (2019) 26: 567-576
DOI: https://doi.org/10.1007/s11771-019-4028-4
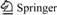
YUAN Zheng-cheng(袁政成), JIANG Zheng-wu(蒋正武), CHEN Qing(陈庆)
Key Laboratory of Advanced Civil Engineering Materials of Ministry of Education, School of Materials Science and Engineering, Tongji University, Shanghai 201804, China
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2019
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2019
Abstract: The permeability modeling of self-healing due to calcium carbonate precipitation in cement-based materials with mineral additives was studied in this work. The parameters of calcium carbonate precipitation during self-healing were simulated. A permeability modeling of self-healing, combined with numerical simulation of calcium carbonate formation, was proposed based on the modified Poiseuille flow model. Moreover, the percentage of calcium carbonate in healing products was measured by TG-DTA. The simulated results show that self-healing can be dramatically promoted with the increase of pH and Ca2+ concentration. The calculated result of permeability is consistent with that measured for cracks appearing in middle or later stages of self-healing, it indicates that this model can be used to predict the self-healing rate to some extent. In addition, TG-DTA results show that the percentage of calcium carbonate in healing products is higher for mortar with only chemical expansion additives or cracks appearing in the later stage, which can more accurately predict the self-healing rate for the model.
Key words: cement-based material; self-healing; mineral additive; calcium carbonate; model
Cite this article as: YUAN Zheng-cheng, JIANG Zheng-wu, CHEN Qing. Permeability modeling of self-healing due to calcium carbonate precipitation in cement-based materials with mineral additives [J]. Journal of Central South University, 2019, 26(3): 567–576. DOI: https://doi.org/10.1007/s11771-019-4028-4.
1 Introduction
Cracks caused by external loading and other factors are unavoidable in cement-based materials [1, 2]. These cracks can have significantly negative effects on permeability, which can help the harmful substances penetrate into cement-based materials and thus reduce the durability of cement-based materials [3, 4]. However, the harmful cracks or unstable cracks are often generated by microcracks accumulation, therefore the security and maintenance costs of cement-based materials will be greatly improved once the initial microcracks are self-healing based on their inherent capability [5, 6].
Self-healing of cracks in concrete is actually an old and well known phenomenon. Concrete in itself possesses some autogenous healing properties due to the continuing hydration of clinker minerals and precipitation of CaCO3 [7, 8]. However, these autogenous healing properties are limited to small cracks [9]. To promote crack self-healing of cement-based materials, some mineral additives can be incorporated into the materials when they are mixed. When healing products or expansion appear in cracks, they can then heal by themselves [10].
The self-healing mechanisms of cement-based materials incorporating mineral additives include the hydration reaction of cementitious [11, 12], chemical expansion or swelling [13, 14], and crystal precipitation [15, 16]. Previous studies by HEIDE [11], HUANG et al [17] and LV et al [18] proposed some self-healing models on the basis of the hydration reaction and chemical expansion. As a typical healing product in cracked cement-based materials, calcium carbonate (mostly calcite) has been observed in almost all laboratory studies and in a number of cracked concretes and masonry mortars [10, 19–21]. It is commonly believed [22] that this precipitation is generated from calcium ions dissolving out of the cement-based materials and carbonate ions from aqueous solution. Most studies on crystallization and precipitation of calcium carbonate during self-healing focus on the experimental stage. However, there are fewer studies involving a numerical modeling. Therefore, the parameters of calcium carbonate precipitation during self-healing were simulated. For the self-healing of surface cracks, a permeability model, combined with numerical simulation of calcium carbonate formation, was proposed and validated in this work, focusing on predicting the rate of the self-healing. Moreover, in order to prove the accuracy of the model, the percentage of calcium carbonate in healing products was measured by TG-DTA.
2 Precipitation of calcium carbonate during self-healing
2.1 Chemical equilibrium equation
In the self-healing of cement-based materials incorporating mineral additives, the reaction between CO2 and Ca2+ and the equilibrium constant are shown in Table 1.
The initial concentration of Ca2+ available to form calcium carbonate in different conditions of the partial pressure of CO2 (PCO2) and pH can be obtained from the equations in Table 1 as follows:
 (1)
(1)
Equation (1) represents the equilibrium relationship between the initial concentration of Ca2+ and external environment in theory, as shown in Figure 1.
Figure 1 shows that the larger the values of pH or PCO2 are, the smaller the number of Ca2+ was to form calcium carbonate. However, the quantitative relationship between the precipitation of calcium carbonate and the main factors is still not clear. More detailed work is done as follows.
2.2 Analysis of parameters of calcium carbonate formation
The influence of different parameters (pH, PCO2 and Ca2+) on calcium carbonate formation was studied. The amount of precipitation was indicated by the ratio of calcium in the precipitate to the total calcium.
2.2.1 pH of crack solution
At 25 °C, Ca2+ concentration of 1×10–3 mol/L and CO2 partial pressure of 1.01325×10–4.5 MPa, the influence of different pH values on precipitation of calcium carbonate is shown in Figure 2.
In general, pH has an important effect on carbonic acid component. As shown in Table 1, the proportion of H2CO3 (aq) is significant at pH 1=6.38, and the proportion of HCO3– is considerably greater at pK1≤pH≤pK2=10.35 and reaches the maximum near pH=8, however, CO32– dominates at pH>pK2=10.35. EDVARDSEN [23] suggested that calcite formation was realized at pH 7.5–8, but calcite was scarce and instable. ALIKO-BEN TEZ et al [24] considered pH>8 as a simplified way of the calcium carbonate formation on the basis. As shown in Figure 2, the results of simulation are essentially the same with them at pH<8. After that calcium carbonate precipitation increases with the pH, which is between 8 and 9 in the above conditions. It is well known that the concentration of HCO3– decreases,the concentration of CO32– increases considerably at pH 8–9, which benefits the precipitation of calcium carbonate. In addition, a greatest contribution of calcite formation is made at pH>9. As a whole, the critical pH value is 8 for calcite formation. So the effect of PCO2 and Ca2+ on calcite formation at pH 8, pH 9, pH 10 and the effect of pH 7, pH 8, pH 9 was investigated respectively as follows.
TEZ et al [24] considered pH>8 as a simplified way of the calcium carbonate formation on the basis. As shown in Figure 2, the results of simulation are essentially the same with them at pH<8. After that calcium carbonate precipitation increases with the pH, which is between 8 and 9 in the above conditions. It is well known that the concentration of HCO3– decreases,the concentration of CO32– increases considerably at pH 8–9, which benefits the precipitation of calcium carbonate. In addition, a greatest contribution of calcite formation is made at pH>9. As a whole, the critical pH value is 8 for calcite formation. So the effect of PCO2 and Ca2+ on calcite formation at pH 8, pH 9, pH 10 and the effect of pH 7, pH 8, pH 9 was investigated respectively as follows.
Table 1 Equilibrium constant and chemical reaction for calcium carbonate precipitation


Figure 1 Relationship between Ca2+, pH and PCO2
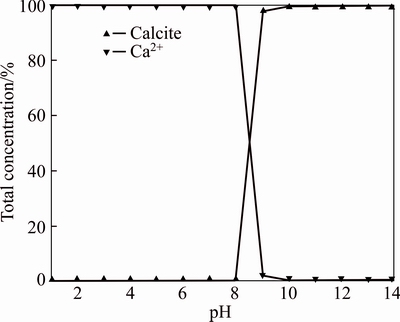
Figure 2 Effect of pH on calcium carbonate precipitation
2.2.2 Partial pressure of CO2 (PCO2)
At 25 °C and a Ca2+ concentration of 1×10-4 mol/L, the influence of different PCO2 on calcium carbonate precipitation is shown in Figure 3.
As shown in Figure 3, at pH 8, the calcium carbonate precipitation is not formed at normal atmospheric pressure. The ratio of calcium in the precipitate to the total calcium is only about 50% when PCO2 is about 100 times higher than the normal atmospheric pressure. In addition, the ratio of calcium in the precipitate to the total calcium is approximately 75% for normal atmospheric pressure at pH 9. After that, when the pH value is more than 9, the formation of calcium carbonate is very easy, the results are consistent well with that of pH above. So it demonstrates that the effect of pH on calcium carbonate precipitation is bigger than that of PCO2 and the proportion of carbonate is mainly affected by pH.
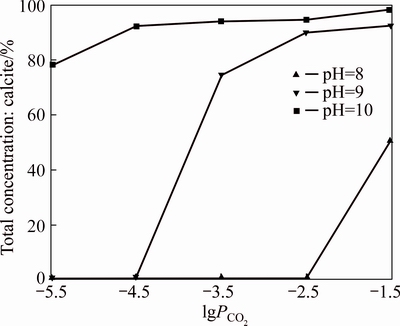
Figure 3 Effect of PCO2 on calcium carbonate precipitation at different pH values
2.2.3 Concentration of Ca2+
At 25 °C and a CO2 partial pressure of 1.01325×10–4.5 MPa, the influence of the concentration of Ca2+ (1×10–3–1×10–2mol/L) on calcium carbonate precipitation is shown in Figure 4.
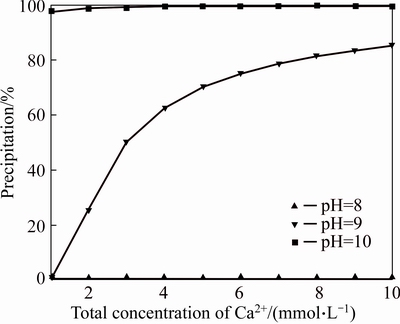
Figure 4 Effect of concentration of Ca2+ on calcium carbonate precipitation at different pH values
Basically, calcite formation is due to the reaction between the calcium ions that are in the cement-based materials and the available bicarbonates and carbonates, the initial concentration of Ca2+ is one to the most important factors for the formation of calcium carbonate. Figure 4 shows that the percentage of calcium carbonate is 0 at pH≤7 when the initial concentration of Ca2+ ranges from 1×10–3 to 1×10–2 mol/L. The amount of calcium carbonate precipitation increases with the total concentration of Ca2+ at pH 8, however, the percentage of calcium carbonate is less than 80% although the initial concentration of Ca2+ is maximum. After that, when the pH value is 9, the percentage of calcite was near 100% with Ca2+ concentration of 3 mmol/L. In a word, the amount of calcium carbonate formation increases with total Ca2+ concentration in a certain pH range, which has a large effect on the self-healing of cracks.
3 Permeability modeling of self-healing
3.1 Model and related parameters
Plummer-Wigley-Parkhurst (PWP) [25] and diffusion boundary layer (DBL) models [26] are used to describe the dissolution of calcite. They are also appropriate models for precipitation [27]. In the model, it is assumed that the reaction time of ions is ignored, the molecular CaCO3 deposition per unit area per unit time can be written as follows:
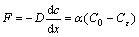 (2)
(2)
where F is the molecular CaCO3 deposition per unit area per unit time; α is the deposition rate, α=D/ε; D is the diffusion coefficient of calcium in crack solutions; and ε is the diffusion layer thickness; C0 is the calcium ion concentration of the crack solution in mol/L; Cε is the calcium ion concentration during the precipitate in mol/L. The linear rate of CaCO3 deposition can be written as follows:
 (3)
(3)
where VM is the molar volume of CaCO3.
As a healing product, CaCO3 forms on both sides of the cracks during self-healing process. The crack width at different healing ages can be written as
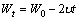 (4)
(4)
where Wt is the crack width at a particular healing age, W0 is the initial crack width, and t is the self-healing age.
The flow of water through cracks in cement-based materials is analyzed by the Poiseuille flow model, which is derived from parallel-plate theory. The modified Poiseuille flow model is given by [28]:
q=740·I·W3·Kt (5)
where q is the water flow per meter visible crack (L/h), I is the hydraulic gradient (m/m), W is the mean crack width at the surface (mm), and Kt is the temperature-coefficient.
Combining Eqs. (2)–(5), the self-healing model of permeability can be written as follows:
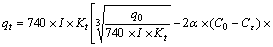
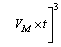 (6)
(6)
where qt and q0 are the water flow of cracks at self-healing age t and 0, respectively, in units of L/h.
At 25 °C water, α=7.92×10–6 m/s with D=0.792×10–9 m2/s and ε=0.01 cm [29]. The diffusion layer ε may lose its actual meaning if the crack width is close to the diffusion layer thickness. However, CaCO3 deposits increase near the opening or outer edge of cracks, and there is also a certain degree of uncertainty in the direction of CaCO3 crystal growth. As a consequence, ε is just used to describe the deposition rate as a parameter in this model during the preliminary study.
The water permeating capacity is affected by temperature-coefficient because the viscosity of water shows a rather pronounced variation with temperature. LI et al [30] has carried out the temperature-coefficient at different temperatures, as shown in Table 2. And the temperature-coefficient can be determined at 25 °C water, and Kt=1.10.
Table 2 Temperature-coefficient at different temperatures

As calcium carbonate mainly consists of calcite, so VM=36.93 cm3/mol, this value is directly taken from the experimental work [31]. In addition, the other parameters, such as I, q0, C0 and Cε, can be obtained by either test or calculation in Section 3.2.2.
3.2 Model validation and analysis
3.2.1 Materials
The cement used in this experiment was P·II Portland cement, with a strength grade of 52.5, produced by Xiaoyetian Cement Group in China. Chemical compositions of the cement determined by X-ray fluorescence(XRF) are listed in Table 3. The fine aggregate was natural river sand with a fineness modulus of 2.2. The water used was tap water. The mineral additives are listed in Table 4.
Table 3 Main chemical composition of cement (mass fraction, %)

Table 4 Types of mineral additives

The optimized mortars proportions are given in Table 5 according to a large number of the previous series experiments. The water of cement ratio is 0.45 and the sand ratio of cement is 2. The control mortar is denoted by M1, without adding any mineral materials. The mortars M2, M3, M4 are partially replaced by minerals additives.
Table 5 Mixture proportion of mortars by mass ratio
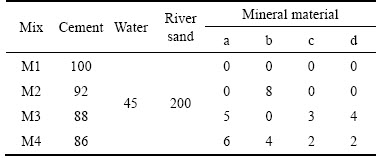
The mortars with dimensions of 70.7 mm× 70.7 mm×70.7 mm were cast in molds. The fresh mortar specimens were demolded after 24 h and then moved out and placed in room at (20±2) °C and (95±5)% RH for certain days.
3.2.2 Test scheme and method
1) Pre-crack: The cracks were produced by the splitting method after 7 or 28 d in the standard curing conditions. A crack with the width of about 0.3 mm was made. After that, the cracked mortar specimens were cured in water at 25 °C for different self-healing ages.
2) Surface crack width test: Four parallel lines were marked in different parts of the crack, the surface crack width (W0) was measured by VMS3023 optical microscope (magnification= 180×). The tests were performed after prefabricating cracks during the initial state.
3) Calcium ion concentration (C0) and pH test of crack solution: the specimens were soaked in deionized water with only the cracks exposed, all the surfaces were sealed with a silicone sealant. The calcium concentration and pH of the solution were measured by inductively coupled plasma atomic emission spectroscopy (ICP-AES) and a pH electrode respectively on the 7th and 28th d. So the calcium ion concentration (C0) and pH test of crack solution in the model can be known, after that setting the CO2 partial pressure at 1.01325×10–4.5 MPa, the initial calcium ion concentration at 1×10–3 mol/L and using different pH values,the calcium ion concentration of the precipitation (Cε) of different test groups (M2, M3, M4) and the control group (M1) can be calculated in Section 2.2.3.
4) Penetration test: The water flow through the cracks under 200 mm hydraulic pressure in 5 min was measured at different self-healing ages (Figure 5) [10], and the volume of water was recorded. The hydraulic gradient (I) also can be known from Figure 5. After that, the initial water permeating capacity (q0) can be determined based on Eq. (5), and the water flow of cracks (qt) can be further calculated in putting all the results or parameters into Eq. (6).
TG-DTA analysis: In order to prove the accuracy of the model, the percentage of calcium carbonate in healing products was measured by TG-DTA [32]. The healing products were carefully scratched off from the cracked mortar surfaces after curing 28 d with tweezers and dried in an oven at 80 °C, then the products were ground into powders for TG-DTA analysis. The tests were performed in argon atmosphere. The heating rate was 10 °C/min and the final temperature was 1000 °C.
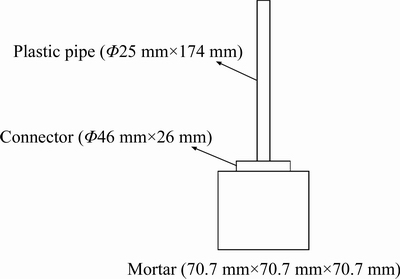
Figure 5 A set-up for testing permeability coefficient
4 Results and discussion
4.1 Surface crack width
The initial cracks width of cracked mortars were measured and recorded at 7 and 28 d, respectively. As shown in Figure 6, the width of initial cracks is mainly 0.25–0.35 mm. The larger the width of initial cracks is, the stronger the permeating capacity is. However, the effect of the width of initial cracks on the measured and calculated results is not significant, so the deviations of initial cracks width among different mortars are acceptable.

Figure 6 Initial cracks width of cracked mortars at different ages
4.2 Calcium ion concentration (C0) and pH test of crack solution
As discussed in Section 2, the pH and calcium ion are the most important factors for calcium carbonate precipitation, so it is necessary to test the calcium ion concentration and pH of crack solution at different pre-cracked ages. The pH and calcium concentration of crack solution at different cracked ages are shown in Figure 7. It can be seen from Figure 7(a) that the solutions in which mortars M2, M3 or M4 are soaked have higher pH values than the solution in which mortar M1 is soaked. In addition, as shown in Figure 7(b), the solution in which mortar M3 is soaked has a much higher calcium ion concentration than that of mortar M1. The calcium ion concentrations of mortars M2 and M4 in the solutions are little higher than the calcium ion concentration of mortar M1.
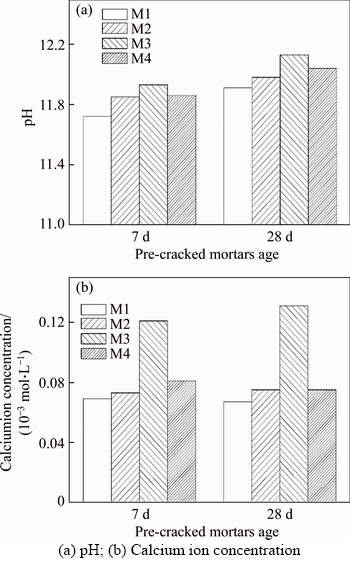
Figure 7 Important factors for calcium carbonate precipitation in crack solution at different cracked ages:
4.3 Model validation and analysis
The measured (referring to “e” in sample number) and calculated (referring to “c” in subscript) water flow rates are shown in Figures 8(a) and (b), where the cracks appeared at 7 d and 28 d, respectively.
As shown in Figure 8, the permeability of mortar M3 is the best among all the mortars, and the pH and calcium ion concentrations of mortar M3 are the highest in Figure 7, so it demonstrates that higher pH values and higher calcium ion contents are found to be the main contributor to precipitation of calcium carbonate, which agrees with the result of numerical simulation analysis as mentioned above. Moreover, the results of measured self-healing rate, namely the drop in the water flow rate, are consistent with the simulation results, especially for mortar M2 or cracks appearing in the later stage of self-healing.

Figure 8 Change of water permeating capacity as a function of time at different cracked ages:
In order to prove the accuracy of the model, the percentages of calcium carbonate in healing products are measured by TG-DTA. Figure 9 shows the results of TG-DTA test for the mortars M1, M2, M3 and M4 cracked at 7 d and the mortar M1 cracked at 7, 28 d respectively. There is remarkable mass loss of all samples at temperature between 660 and 880 °C, which can possibly be contributed by the decomposition of calcite, indicating that the healing products are mainly calcite. The results are well consistent with the previous studies [10] by using XRD and SEM-EDS analysis. It proves that this model can be used to predict the self-healing rate to some extent. Moreover, the percentages of calcium carbonate in healing products for the mortars M1, M2, M3 and M4 are 76.1%, 85.7%, 72.1% and 82.7%, respectively in Figure 9(a), and the percentages of calcium carbonate in healing products for the mortar M1 cracked at 7 and 28 d are 76.1% and 84.8%, respectively, as shown in Figure 9(b). It shows that the percentage of calcium carbonate in healing products is higher in cement- based materials with only chemical expansion additives or cracked later, which can more accurately predict the permeability improvement due to self-healing.

Figure 9 TG-DTA curves of healing products:
However, some deviation of the healing rate between the analysis and the experiments is still found in Figure 8, which can be explained by the reasons below. The test result is better than predicted at the early stages of self-healing (0–3 d), mainly because the self-healing mechanism of cement hydration and expansion are still obvious in this stage, so the reduction in water flow is caused by several types of self-healing products such as C-S-H [33, 34], Aft [35], and calcium carbonate. The permeability of test result is lower than predicted at the later stages of self-healing (>28 d), mainly because in healing process, the self-healing rate is reduced [23].
The crack mouth is mainly deposited by the precipitation of calcium carbonate as many experimental studies suggest [9, 10, 36], considering the complexity of the self-healing of cement-based materials incorporating mineral additives, this model is mainly based on the calcium carbonate precipitation, and the mechanisms of cement hydration and expansion are not included. However, it should be understood that these three mechanisms co-exist in the actual healing process and interact with each other. For instance,further hydration of unhydrated cement will take place in the cracks and generate calcium hydroxide, however, calcium hydroxide reacts with silica- based material and chemical expansion material to form C-S-H and Aft, so the consumed calcium hydroxide resulting unhydrated cement accelerates again and the potential of CaCO3 formation may be reduced [37–40]. Moreover, the model can be used for different conditions though the hydration role of cement or mineral additives is time-dependent or curing condition-dependent, because we find that the precipitation of calcium carbonate is the main contributor to the healing of surface cracks at different ages and curing conditions in our previous study [10]. However, it is worth noting that the accuracy of the model may be affected by different conditions. As mentioned above, some deviation of the healing rate between the analysis and the experiments is still found due to different ages. The percentage of calcium carbonate in healing products may be larger due to higher pH values and higher calcium ion contents, which can more accurately predict the self-healing rate for the model. In addition, we can predict that the permeability will be decreased with the increase of chemical expansion materials or crystallization precipitation materials, because these materials can improve the pH or the concentration of Ca2+. However, it is very important that the properties of cement-based materials cannot be greatly affected by increasing the content of mineral materials. When the adding amount of chemical expansion materials is too much, additional cracking may be induced by restrained deformation. The setting time of cement-based materials will be changed greatly by adding more crystalline precipitated materials. Finally, in practice, in order to consider other ions in the water, such as Cl– and SO42–, they are not clear to precisely predict the self-healing efficiency in this model considering their effect on calcite formation. Further research about this will be carried out.
4 Conclusions
The permeability modeling of self-healing due to calcium carbonate precipitation in cement-based materials with mineral additives is investigated. The following conclusions are drawn:
1) The simulated results show that self- healing can be dramatically promoted by an increase in the pH of the crack solution, the concentration of Ca2+ within a certain range, which is consistent with the experimental results.
2) A permeability model of self-healing was proposed based on calcium carbonate precipitation, and it can be used to predict the self-healing rate to some extent, especially for mortar with only chemical expansion additives or cracks appearing in the later stage due to their higher percentage of calcium carbonate in healing products.
3) At the early stage of self-healing (0–3 d), the cement hydration and expansion are obvious. The reduction of the water flow is caused by several types of self-healing products such as C-S-H, Aft, and calcite, so the actual self-healing rate is faster than that of the model. At the later stage of self-healing (>28 d), the actual transmission of calcium is reduced by the healing process, so the actual self-healing rate is lower than that of the model.
References
[1] HAERI H. Experimental and numerical study on crack propagation in pre-cracked beam specimens under three-point bending [J]. Journal of Central South University, 2016, 23(2): 430–439. DOI: 10.1007/s11771-016-3088-y.
[2] LI Chen, WU Meng-xue, CHEN Qing, JIANG Zheng-wu. Chemical and mineralogical alterations of concrete subjected to chemical attacks in complex underground tunnel environments during 20–36 years [J]. Cement & Concrete Composites, 2018, 86(2): 139–159. DOI: 10.1016/ j.cemconcomp.2017.11.007.
[3] HUANG Hao-liang, YE Guang. Simulation of self-healing by further hydration in cementitious materials [J]. Cement & Concrete Composites, 2012, 34(4): 460–461. DOI: 10.1016/j. cemconcomp.2012.01.003.
[4] SAHMARAN M, YILDIRIM G, ERDEM T K. Self-healing capability of cementitious composites incorporating different supplementary cementitious materials [J]. Cement & Concrete Composites, 2013, 35(1): 89–101. DOI: 10.1016/j.cemconco mp.2012.08.013.
[5] HUANG Hao-liang, YE Guang, SHUI Zhong-he. Feasibility of self-healing in cementitious materials–By using capsules or a vascular system? [J]. Construction and Building Materials, 2014, 63: 108–109. DOI: 10.1016/j.conbuildmat. 2014.04.028.
[6] SANGADJI S. Can self-healing mechanism helps concrete structures sustainable? [J]. Procedia Engineering, 2017, 171: 238–249. DOI: 10.1016/j.proeng.2017.01.331.
[7] HUANG Hao-liang, YE Guang, QIAN Chun-xiang, SCHLANGEN E. Self-healing in cementitious materials: Materials, methods and service conditions [J]. Materials & Design, 2016, 92: 499–511. DOI: 10.1016/j.matdes.2015.12.091.
[8] SAHMARAN M, YILDIRIM G, ERDEM T K. Self-healing capability of cementitious composites incorporating different supplementary cementitious materials [J]. Cement and Concrete Composites, 2013, 35(1): 89–101. DOI: 10.1016/ j.cemconcomp.2012.08.013.
[9] SISOMPHON K, COPUROGLU O, KOENDERS E A B. Self-healing of surface cracks in mortars with expansive additive and crystalline additive [J]. Cement & Concrete Composites, 2012, 34(4): 566–574. DOI: 10.1016/ j.cemconcomp.2012.01.005.
[10] JIANG Zheng-wu, LI Wen-ting, YUAN Zheng-cheng. Influence of mineral additives and environmental conditions on the self-healing capabilities of cementitious materials [J]. Cement & Concrete Composites, 2015, 57(2, 3): 116–127. DOI: 10.1016/j.cemconcomp.2014.11.014.
[11] HEIDE N T. Crack healing in hydrating concrete [D]. Delft: Delft University of Technology, 2005.
[12] HUNG Chung-Chan, SU Yen-Fang, SU Yu-Min. Mechanical properties and self-healing evaluation of strain-hardening cementitious composites with high volumes of hybrid pozzolan materials [J]. Composites Part B–Engineering, 2018, 133: 15–25. DOI: 10.1016/j.compositesb.2017.09.005.
[13] SHERIR M A A, HOSSAIN K M A, LACHEMI M. Self-healing and expansion characteristics of cementitious composites with high volume fly ash and MgO-type expansive agent [J]. Construction & Building Materials, 2016, 127: 80–92. DOI: 10.1016/j.conbuildmat.2016.09.125.
[14] LEE Y S, RYOU J S. Self healing behavior for crack closing of expansive agent via granulation/film coating method [J]. Construction & Building Materials, 2014, 71: 188–193. DOI: 10.1016/j.conbuildmat.20 14.08.045.
[15] ROIG-FLORES M, PIRRITANO F, SERNA P, SCHLANGEN E. Effect of crystalline admixtures on the self-healing capability of early-age concrete studied by means of permeability and crack closing tests [J]. Construction & Building Materials, 2016, 114: 447–457. DOI: 10.1016/j.conbuildmat. 2016.03.196.
[16] ROIG-FLORES M, MOSCATO S, SERNA P, FERRARA L. Self-healing capability of concrete with crystalline admixtures in different environments [J]. Construction & Building Materials, 2015, 86(6): 1–11. DOI: 10.1016/ j.conbuildmat.2015.03.091.
[17] HUANG Hao-liang, YE Guang, DAMIDOT D. Characterization and quantification of self-healing behaviors of microcracks due to further hydration in cement paste [J]. Cement & Concrete Research, 2013, 52(10): 71–81. DOI: 10.1016/j.cemconres.2013.05.003.
[18] LV Zhong, CHEN Hui-su. Modeling of self-healing efficiency for cracks due to unhydrated cement nuclei in hardened cement paste [J]. Procedia Engineering, 2012, 27: 281–290. DOI: 10.1016/j.proeng.2011.12.454.
[19] HERBERT E N, LI V C. Self-healing of microcracks in engineered cementitious composites (ECC) under a natural environment [J]. Materials, 2013, 6(7): 2831. DOI: 10.3390/ma6072831.
[20] TALAIEKHOZAN A, KEYVANFAR A, SHAFAGHAT A, ANDALIB R, MAJID M Z A, FULAZZAKY M A, ZIN R M, LEE C T, HUSSIN M W, HAMZAH N, MARWAR N F, HAIDAR H I. A review of self-healing concrete research development [J]. Journal of Environmental Treatment Techniques, 2014, 2(2): 1–11.
[21] HAN Bao-guo, WANG Yun-yang, DONG Su-fen, ZHANG Li-qing. Smart concretes and structures: A review [J]. Journal of Intelligent Material Systems & Structures, 2015, 26(11): 1–43. DOI: 10.1177/1045389X15586452.
[22] SIAD H, ALYOUSIF A, KESKIN O K, KESKIN S B. Influence of limestone powder on mechanical, physical and self-healing behavior of engineered cementitious composites [J]. Construction & Building Materials, 2015, 99(1): 1–10. DOI: 10.1016/j.conbuildmat.2015.09.007.
[23] EDVARDSEN C. Water permeability and autogenous healing of cracks in concrete [J]. ACI Mater J, 1999, 96(4): 448–454.
[24] ALIKO-BEN TEZ A, DOBLAR
TEZ A, DOBLAR M, SANZ-HERRERA J A. Chemical-diffusive modeling of the self-healing behavior in concrete [J]. International Journal of Solids & Structures, 2015, 69–70(5): 392–402. DOI: 10.1016/j.ijsolstr.2015.05. 011.
M, SANZ-HERRERA J A. Chemical-diffusive modeling of the self-healing behavior in concrete [J]. International Journal of Solids & Structures, 2015, 69–70(5): 392–402. DOI: 10.1016/j.ijsolstr.2015.05. 011.
[25] PLUMMER L N, WIGLEY T M L, PARKHURST D L. The kinetics of calcite dissolution in CO2-water systems at 5– 60 °C and 0.0–1.0 atm CO2 [J]. American Journal of Science, 1978, 278(2): 179–216.
[26] LIU Zai-hua, DREYBRODT W. The DBL model and predication of calcite dissolution/precipitation rates [J]. CarsologicaSinica, 1998, 1(1): 3–9.
[27] DREYBRODT W, BUHMANN D. A mass transfer model for dissolution and precipitation of calcite from turbulent motion [J]. Chem Geol, 1991, 90(1, 2): 107–122. DOI: 10.1016/0009-2541(91)90037-R.
[28] DESMETTRE C, CHARRON J P. Water permeability of reinforced concrete with and without fiber subjected to static and constant tensile loading [J]. Cement and Concrete Research, 2012, 42(7): 945–952. DOI: 10.1016/j.cemconres. 2 012.03.014.
[29] PRESS C. CRC Handbook of chemistry and physics [M]. Taylor & Francis, 2010.
[30] LI Hou-xiang, TANG Chun-an, ZENG San-hai, LI Si-nian. Research on self-healing of concrete cracks [J]. Journal of Wuhan University of Technology, 2004(3): 27–29. (in Chinese)
[31] NIJLAND T G, LARBI J A, HEES R P J V, LUBELLI B, ROOIJ M D. Self healing phenomena in concretes and masonry mortars: A microscopic study [C]// Proceedings of the First International Conference on Self Healing Materials. The Netherlands: Noordwijk aan Zee, 2007: 1–9.
[32] ZHOU Wei, LI Liang, LIU Shu-hua, VINH T N D, LIU Xing-hong. Hydration properties and thermal analysis of cement-based materials containing limestone powder [J]. Journal of Central South University, 2017, 24(12): 2932–2939. DOI: 10.1007/s11771- 017-3707-2.
[33] HUANG Hao-liang, YE Guang, DAMIDOT D. Effect of blast furnace slag on self-healing of microcracks in cementitious materials [J]. Cement & Concrete Research, 2014, 60(2): 68–82. DOI: 10.1016/j.cemconres.20 14.03.010.
[34] HILLOULIN B, HILLOULIN D, GRONDIN F, LOUKILI A, BELIE N D. Mechanical regains due to self-healing in cementitious materials: Experimental measurements and micro- mechanical model [J]. Cement & Concrete Research, 2016, 80(6): 21–32. DOI: 10.1016/j.cemconres.2015.11.005.
[35] LIU He-zhi, ZHANG Qian, GU Chong-shi, SU Huai-zhi, LI V. Self-healing of microcracks in Engineered Cementitious Composites under sulfate and chloride environment [J]. Construction & Building Materials, 2017, 153: 948–956. DOI: 10.1016/j.conbuildmat.20 17.07.126.
[36] WANG Xian-feng, FANG Cheng, LI Da-wang, HAN Ning-xu, XING Feng. A self-healing cementitious composite with mineral admixtures and built-in carbonate [J]. Cement & Concrete Composites, 2018, 92(9): 216–229. DOI: 10.1016/j.cemconcomp.2 018.05.013.
[37] TITTELBOOM K V, GRUYAERT E, RAHIER H, BELIE N D. Influence of mix composition on the extent of autogenous crack healing by continued hydration or calcium carbonate formation [J]. Construction & Building Materials, 2012, 37: 349–359. DOI: 10.1016/j.conbuildmat.2012.07.026.
[38] LI Chen, ZHU Hong-bo, WU Meng-xue, WU Kai-fan, JIANG Zheng-wu. Pozzolanic reaction of fly ash modified by fluidized bed reactor-vapor deposition [J]. Cement & Concrete Research, 2017, 92(2): 98–109. DOI: 10.1016/j.cemconres.201 6.11.016.
[39] LIU Shu-hua, KONG Ya-ning, WANG Lu. Hydration mechanism of low quality fly ash in cement-based materials [J]. Journal of Central South University, 2014, 21(11): 4360–4367. DOI: 10.1007/s11771-014-2436-z.
[40] BA Ming-fang, QIAN Chun-xiang. Hydration evolution of pre-cast concrete with steam and water curing [J]. Journal of Central South University, 2013, 20(10): 2870–2878. DOI: 10.1007/s11771-013-1808-0.
(Edited by FANG Jing-hua)
中文导读
基于碳酸钙沉淀掺矿物外加剂水泥基材料的自愈合渗透性模型
摘要:本文基于碳酸钙沉淀愈合裂缝机理,研究了掺矿物外加剂的水泥基材料裂缝自愈合渗透性模型。模拟了自愈合过程中影响碳酸钙沉淀的主要参数,同时结合改性的泊肃叶渗流模型,提出了自愈合渗透性模型,最后使用热重分析测试了愈合产物中碳酸钙所占的比例。结果表明,在一定范围内,自愈合效果随着裂缝溶液中pH和钙离子浓度的增加而显著提升。中期或后期开裂的砂浆,其自愈合渗透性的计算结果与测试结果一致,这说明在某种程度上自愈合渗透性模型能够预测裂缝的自愈合速率。此外,热重分析结果表明,单掺膨胀剂或晚龄期开裂砂浆的愈合产物中含有更高比例的碳酸钙,有利于模型更准确地预测裂缝的自愈合速率。
关键词:水泥基材料;自愈合;矿物外加剂;碳酸钙;模型
Foundation item: Project(2018YFC0705404) supported by the National Key Technology Research and Development of China; Projects(51878480, 51678442, 51878481, 51878496) supported by the National Natural Science Foundation of China; Project(U1534207) supported by the National High-speed Train Union Fund, China; Project supported by the Fundamental Research Funds for the Central Universities, China
Received date: 2018-03-21; Accepted date: 2018-07-30
Corresponding author: JIANG Zheng-wu, PhD, Professor; Tel: +86-21-69582140; E-mail: jzhw@tongji.edu.cn; ORCID: 0000-0002- 6464-2622

