DOI: 10.11817/j.issn.1672-7207.2015.11.006

含孔功能梯度压电材料板的力电耦合无网格伽辽金法

孟广伟，王晖，周立明，李锋，李霄琳

(吉林大学 机械科学与工程学院，吉林 长春，130022)

摘要：为提高含孔功能梯度压电材料板的计算精度，基于变分原理和功能梯度压电材料的本构关系、几何关系、边界条件等，推导出功能梯度压电材料的无网格方程，提出含孔功能梯度压电材料板的力电耦合无网格伽辽金法。求解含孔功能梯度压电材料板的力学问题，研究孔边环向应力分布及力电集中问题，讨论材料参数按某一方向呈指数函数梯度变化时功能梯度压电材料的力学响应，与ANSYS计算结果进行比较，数值算例结果表明本方法正确可行且具有较高的精度，可求解任意梯度函数的功能梯度压电材料问题。

关键词：功能梯度压电材料；无网格伽辽金法；力电耦合

中图分类号：TB115             文献标志码：A         文章编号：1672-7207(2015)11-4015-06

Electromechanical element-free Galerkin method for functionally graded piezoelectric plate with circular hole

MENG Guangwei, WANG Hui, ZHOU Liming, LI Feng, LI Xiaolin

(School of mechanical Science and Engineering, Jilin University, Changchun 130022, China)

Abstract：For improving the calculation precision of functionally graded piezoelectric plate with a circular hole, the element-free method equations were deducted by using variation principle, constitutive relations, geometrical relations and the boundary conditions of functionally graded piezoelectric plate. Electromechanical-coupling element-free Galerkin method (EEFGM) for functionally graded piezoelectric plate with a circular hole was proposed. The mechanical issues of functionally graded piezoelectric material plate were solved. The problems of hoop stress distribution and electromechanical concentration were studied. The mechanical response of functionally graded piezoelectric material was discussed, with its material parameters changing exponentially in a certain direction. The numerical example results were compared with those of the finite element software ANSYS. Numerical example results show that this method is feasible. Any problem of functionally graded piezoelectric material can be solved.

Key words: functionally graded piezoelectric material; electromechanical element-free Galerkin method; electromechanical- coupling

压电材料以其独特的力电耦合特性既可作为传感元件，又能作为驱动元件，在智能结构和微机电系统中发挥着重要作用，应用范围广阔。近年来，随着科技的发展，功能梯度的概念被引入到压电材料的设计中，研制了功能梯度压电材料。它是将压电材料和功能梯度材料有机结合起来的一种新材料，兼备压电和功能梯度二者的优点，即具有良好的机电耦合特性，同时又能消除层间界面，大大减缓层间界面处的集中应力，充分发挥各组分材料的优良性能。功能梯度压电材料已应用到航空航天、核能、生物医学等高新技术领域。在工程实际中，由于用功能梯度压电材料制作的器件往往以板和壳的结构形式出现，在力电耦合作用下，常常会因制造和使用过程中出现的夹杂和孔洞引起的应力和电场集中而导致功能丧失，因此研究含孔洞的功能梯度压电材料板对提高相关器件性能和使用可靠性是十分必要的^[1-5]。目前，国内外对功能梯度压电材料的研究已取得大量的成果。Zhong等^[6]对功能梯度压电材料平板进行了力电耦合精确分析，Almajid等^[7]基于经典层合板理论，考虑电场作用下的力电耦合效应，分析了功能梯度压电板的离面位移和应力场；Lezgy-Nazargah等^[8]用有限元方法对功能梯度压电材料梁进行了动态分析；Pak^[9-10]用分布位错法计算了有限裂纹镶嵌在无限大的压电介质中的电弹性场和能量释放率，加载方式为力电同时加载。Kogan等^[11]推出了横观各向同性体中含有椭球夹杂的极限情况圆币型裂纹受轴对称加载的强度因子。Chen等^[12]利用Green方程探究了板受法向集中力和集中力偶作用时孔周的应力集中现象。Liu等^[13]用扩展有限元法分析了功能梯度压电材料瞬态热冲击断裂问题。随着功能梯度压电材料的应用领域不断扩大，功能梯度压电材料的非均匀性和一些特殊的应用给功能梯度压电材料结构的常规数值方法分析增加了难度。无网格法是近20多年发展起来的一类数值方法，该方法完全采用基于节点的近似，避免网格再生成的复杂过程，非常适合分析裂纹扩展、大变形、多场耦合问题。在众多无网格方法中，无网格伽辽金法以其方法稳定、精度高、对离散点分布不敏感和适合结构分析等优点受到了较多的关注^[14-19]。但在国内鲜有学者开展该方法在功能梯度压电材料方面的研究。鉴于此，本文作者推导出功能梯度压电材料的无网格方程，提出含孔功能梯度压电材料的力电耦合无网格伽辽金法，采用伽辽金弱形式进行求解，研究孔边环向应力分布及力电集中问题，讨论了材料参数按某一方向呈指数函数梯度变化时功能梯度压电材料的力学响应，与ANSYS软件计算结果进行比较。

1  功能梯度压电材料基本方程

功能梯度压电材料场方程为

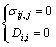
应变和电场相容方程为

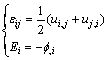
本构方程为

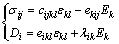
式中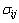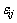：，，D_i和E_i分别为应力、应变、电位移和电场强度；u_i为位移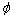；为电势；c_ijkl为弹性模量系数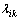；为介电系数；e_kij为压电系数。

在功能梯度压电材料中，材料参数是在某一方向上按某一特定函数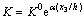连续变化的。文中研究的功能梯度压电材料参数服从指数分布

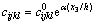
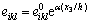
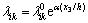
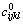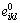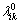其中：，和为板下表面的材料参数；h为板x₃方向的长度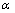；为材料梯度参数，可由平板下表面和上表面的材料参数求得

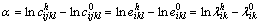
在平面应变情况下，压电材料被看成是横观各向同性的，并将x₁-x₂平面定义为各向同性面。x₂轴为平面问题的厚度方向，x₃轴垂直于该平面，于是研究的是x₁-x₃平面内的问题。本构关系表示矩阵形式为

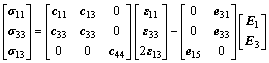
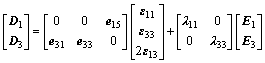
2  力电耦合无网格伽辽金法

采用位移u和电势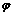为基本场量来进行无网格法求解，在求解域内任一点x处的位移和电势可由如下公式来表示：

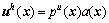
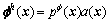
式中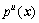：和为基函数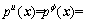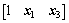， ；a(x)为与空间坐标相关的函数。

下面以位移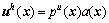为例，说明无网格法推导节点形函数过程。

使场函数的近似在各个节点的误差的加权平方和最小：

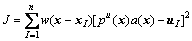
式中：u_I为函数在节点x_I处的值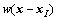；为节点x_I在x处的权函数值，它是以x_I为中心的紧支函数；n为影响域包含的接点数，因此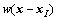，＞0。当J达到最小值时，有如下关系式：

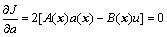
即

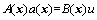
其中：

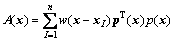
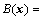
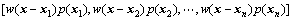
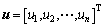
由式(14)得：

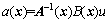
将式(18)代入式(10)，即可得无网格法近似函数：

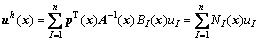
式中：。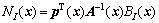

同理

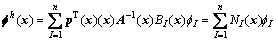
求解域内的势能表达式：

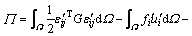
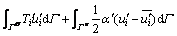
式中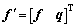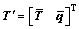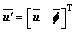：，，和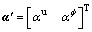分别为广义体积力、给定的广义边界力、广义边界位移和罚函数列阵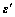；为式(3)中广义应变矩阵；G为广义弹性矩阵。需要说明的是由于力场矩阵系数与电场矩阵系数数量级相差极大，因此罚函数需分别选取。

在系统达到平衡时，系统的势能最小，由式(9)得平衡方程：

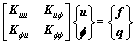
式中：

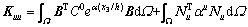
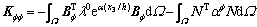
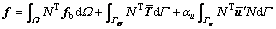
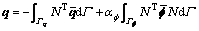
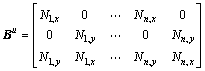
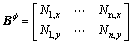
3  数值算例

如图1所示，含圆孔的功能梯度PZT-4压电材料板，边长a=0.4 m，圆孔半径与板边长比r/a=1:10。板底边电势为0 V，上边给定电势V₀=-0.72 MV，板的上下边应力σ=7.5 MPa，设其极化方向为x₃轴正向。材料的梯度变化方向为x₃轴正向，梯度函数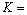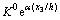，本文中α取为0.5。

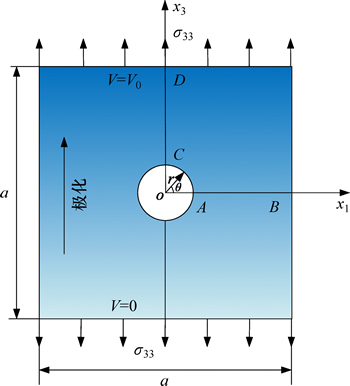
图1  含圆孔功能梯度压电板

Fig. 1  Functionally graded piezoelectric plate with a hole

底边材料参数^[14]为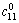：=13.9×10¹⁰ N/m²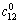，= 7.78×10¹⁰ N/m²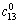，=7.43×10¹⁰ N/m²，=11.5×10¹⁰ N/m²，=2.56×10¹⁰ N/m²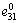，=-5.2 C/m²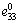，=12.7 C/m²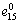，=15.1 C/m²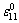，=13.06×10^-9 C×m/V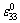，=11.51×10^-9 C×m/V。

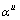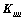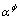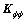在求解域内离散1 656个节点，如图2所示，采用40×40个背景网格，每个网格均采用4×4高斯点进行积分，节点影响域因子为1.7，采用三次样条权函数来进行求解。其中力场罚函数取值为主对角线上最大元素的10⁸倍，电场罚函数为主对角线上最大元素的10⁶倍。

图2  节点分布图

Fig. 2  Node distribution

由力电耦合无网格数值方法求得全场应力分布，图3所示为ANSYS软件计算的结果与无网格法计算的应力云图对比，其中ANSYS采用6 256个网格作为参考解，通过对比发现，EEFGM求解结果与ANSYS软件计算结果吻合地很好。

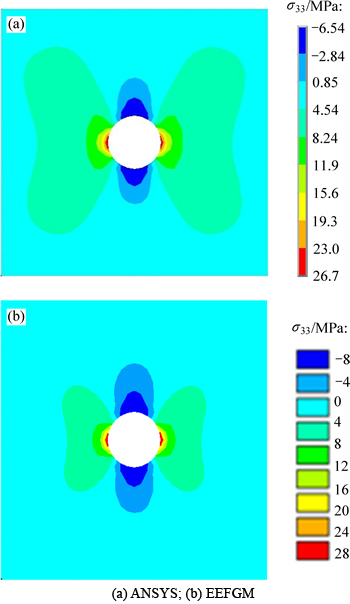
图3  应力σ₃₃分布图

Fig. 3  Distribution of stress σ₃₃

分析AB线和CD线上应力σ₃₃分布分别如图4和图5所示。EEFGM方法的求解结果与划分相同网格数的ANSYS结果进行比较。由图4和图5可知：EEFGM方法与参考解结果吻合得较好，具有更高的精度。

圆孔环向应力σ_θ随角度的变化如图6所示，EEFGM方法的求解结果与ANSYS结果进行比较，依然是EEFGM方法与参考解结果吻合得较好。圆孔环向应力在θ为0°和180°处取得最大值，在θ为90°和270°处取得最小值，与应力分布云图符合，且应力集中现象较明显。

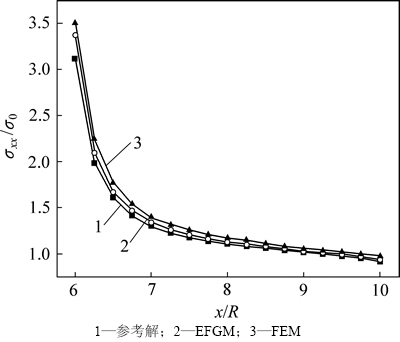
图4  AB线上应力σ₃₃分布

Fig. 4  Stress σ₃₃distribution on line AB

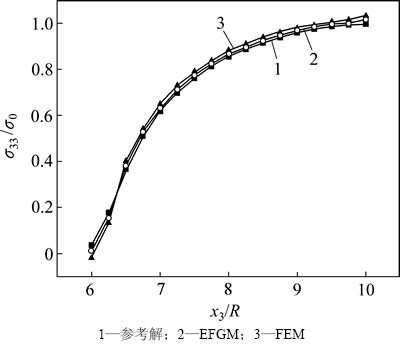
图5  CD线上应力σ₃₃分布

Fig. 5  Stress σ₃₃ distribution on line CD

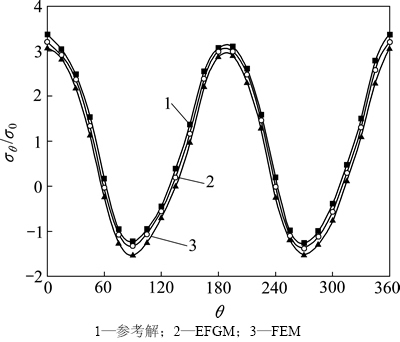
图6  圆孔环向应力σ_θ随角度的变化

Fig. 6  Change of hoop stress σ_θ with angle

4  结论

1) 为提高含孔功能梯度压电材料板的计算精度，基于变分原理和功能梯度压电材料的本构关系、几何关系、边界条件等，推导出功能梯度压电材料的无网格方程，提出了含孔功能梯度压电材料板的力电耦合无网格伽辽金法。

2) 处理了功能梯度材料的力学问题，研究了孔边环向应力分布及力电集中问题，讨论了材料参数按某一方向呈指数函数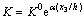梯度变化时功能梯度压电材料的力学响应。

3) 与通用有限元软件ANSYS计算结果进行了比较，数值算例结果表明本方法正确可行且具有较高的精度。通过改变梯度函数能够进一步应用到任意梯度函数的功能梯度压电材料问题，因此应用前景十分广阔。

参考文献：

[1] R, Dineva P, Rangelov T, et al. Anti-plane dynamic hole–crack interaction in a functionally graded piezoelectric media[J]. Archive of Applied Mechanics, 2012, 82(1): 97-110.

[2] Yang Q, Gao C F, Chen W. Stress analysis of a functional graded material plate with a circular hole[J]. Archive of Applied Mechanics, 2010, 80(8): 895-907.

[3] Sosa H. Plane problems in piezoelectric media with defects[J]. International Journal of Solids and Structures, 1991, 28(4): 491-505.

[4] Zhou L M, Meng G W, Li F, et al. Cell-based smoothed finite element method-virtual crack closure technique for a piezoelectric material of crack[J]. Mathematical Problems in Engineering, 2015: 1-10.

[5] Li D M, Zhang L W, Liew K M. A three-dimensional element-free framework for coupled mechanical-diffusion induced nonlinear deformation of polymeric gels using the IMLS-Ritz method[J]. Computer Methods in Applied Mechanics and Engineering, 2015, 296: 232-247.

[6] Zhong Z, Shang E T. Three-dimensional exact analysis of a simply supported functionally gradient piezoelectric plate[J]. International Journal of Solids and Structures, 2003, 40: 5335-5352.

[7] Almajid A, Taya M, Hudnut S. Analysis of out-of-plane displacement and stress field in a piezocomposite plate with functionally graded microstructure[J]. International Journal of Solids and Structures, 2001, 38(19): 3377-3391.

[8] Lezgy-Nazargah M, Vidal P, Polit O. An efficient finite element model for static and dynamic analyses of functionally graded piezoelectric beams[J]. Composite Structures, 2013, 104: 71-84.

[9] Pak Y E. Linear electro-elastic fracture mechanics of piezoelectric materials[J]. International Journal of Fracture, 1992, 54(1): 79-100.

[10] Pak Y E. Crack extension force in a piezoelectric material[J]. Journal of Applied Mechanics, 1990, 57(3): 647-653.

[11] Kogan L, Hui C Y, Molkov V. Stress and induction field of a spheroidal inclusion or a penny-shaped crack in a transversely isotropic piezoelectric material[J]. International Journal of Solids and Structures, 1996, 33(19): 2719-2737.

[12] Chen P, Nie H. Green’s functions for bending problem of a thin anisotropic plate with an elliptic hole[J]. Mechanics Research Communications, 2004, 31(3): 311-320.

[13] Liu P, Yu T, Bui T Q, et al. Transient thermal shock fracture analysis of functionally graded piezoelectric materials by the extended finite element method[J]. International Journal of Solids and Structures, 2014, 51(11): 2167-2182.

[14] 孟广伟，周立明，李锋, 等. 摄动随机局部正交无网格伽辽金法[J]. 吉林大学学报(工学版), 2011, 40(6): 1556-1561.

MENG Guangwei, ZHOU Liming, LI Feng, et al. Perturbation stochastic local orthoganal element-free Galerkin method[J]. Journal of Jilin University (Engineering and Technology Edition), 2011, 40(6): 1556-1561.

[15] Belytschko T, Krongauz Y, Fleming M, et al. Smoothing and accelerated computations in the element free Galerkin method[J]. Journal of Computational and Applied Mathematics, 1996, 74(1): 111-126.

[16] 熊勇刚, 田万鹏, 陈科良, 等. 基于Kriging 插值无网格法的动力弹塑性分析[J]. 中南大学学报(自然科学版), 2014, 45(2): 435-440.

XIONG Yonggang, TIAN Wanpeng, CHEN Keliang, et al. Dynamic elastoplastic analysis by using meshless Kriging interpolation method[J]. Journal of Central South University (Science and Technology), 2014, 45(2): 435-440.

[17] Pathak H, Singh A, Singh I V. Fatigue crack growth simulations of homogeneous and bi-material interfacial cracks using element free Galerkin method[J]. Applied Mathematical Modelling, 2014, 38(13): 3093-3123.

[18] Li D, Bai F, Cheng Y, et al. A novel complex variable element-free Galerkin method for two-dimensional large deformation problems[J]. Computer Methods in Applied Mechanics and Engineering, 2012, 233: 1-10.

[19] Meng G W, Wang H, Zhou L M, et al. Enriched element-free Galerkin method for fracture analysis of functionally graded piezoelectric materials[J]. Mathematical Problems in Engineering, 2015, 2015: 1-13.

[20] Cheng Z Q, Lim C W, Kitipornchai S. Three-dimensional asymptotic approach to inhomogeneous and laminated piezoelectric plates[J]. International Journal of Solids and Structures, 2000, 37(23): 3153-3175.

(编辑  杨幼平)

收稿日期：2015-01-07；修回日期：2015-03-31

基金项目(Foundation item)：国家重大科学仪器开发专项(2012YQ030075)；国家自然科学基金资助项目(51305157)；吉林省科技厅基金资助项目(20130305006GX)；吉林大学研究生创新基金资助项目(2015121) (Project(2012YQ030075) supported by the National Key Scientific Instrument and Equipment Development Projects of China; Project (51305157) supported by the National Natural Science Foundation of China; Project (20130305006GX) supported by Jilin Provincial Department of Science and Technology Fund; Project(2015121) supported by Graduate Innovation Fund of Jilin University)

通信作者：周立明，博士，讲师，从事计算固体力学研究；E-mail: lmzhou@jlu.edu.cn