J. Cent. South Univ. (2015) 22: 413-420
DOI: 10.1007/s11771-016-3086-0
Free vibration analysis of non-homogeneous orthotropic plates resting on Pasternak elastic foundation by Rayleigh-Ritz method
Ahmad Rahbar-Ranji, Arash Shahbaztabar
Department of Ocean Engineering, AmirKabir University of Technology, Hafez Ave., Tehran 15914, Iran
 Central South University Press and Springer-Verlag Berlin Heidelberg 2015
Central South University Press and Springer-Verlag Berlin Heidelberg 2015
Abstract: Free vibration analysis of non-homogeneous orthotropic plates resting on a Pasternak type of elastic foundation is investigated. A set of admissible orthogonal polynomials are generated with Gram-Schmidt orthogonalization procedure and adopted in the Rayleigh-Ritz method. Accuracy and applicability of the method are examined by comparison of the results for different boundary conditions and material types with those available in literature. It is found that this method has good accuracy regardless of type of boundary condition and yields very accurate results even with low number of terms of orthogonal polynomials for the first mode of vibration. For higher modes of vibration, higher terms of orthogonal polynomials should be used. The effects of foundation parameter, density and non-homogeneity parameters on natural frequency are examined. It is concluded that natural frequency of plates are more sensitive to shearing layer coefficient rather than Winkler coefficient and density parameter has weakening effect on natural frequency.
Key words: free vibration; non-homogeneous orthotropic plate; Pasternak foundation; Rayleigh-Ritz method; Gram-Schmidt procedure
1 Introduction
Noise, malfunction and failure of structures are caused by excessive dynamic behavior. Increasing demands for safety and reliability assessment of structures require better understanding of dynamic properties. Modal analysis of structures is carried out to calculate natural frequencies and mode shapes. These parameters are the essential technical information required in the design of structures and determine the dynamic characteristics of existing structures. For simple structures, theoretical approach can be used to predict these parameters; however, for complex structures, numerical or experimental methods should be used.
Plates as a fundamental structural components play an essential role in various engineering fields, such as mechanic, marine, aerospace and civil. Numerous studies have been carried out to analyze dynamic behavior of plates [1]. Plates with non-uniform thickness are used to reduce weight, increase stiffness and modify dynamic characteristics. Orthotropic rectangular plates with non-uniform thickness have great advantages, such as high strength-to-weight ratio, and low cost. Comprehensive understanding of vibration behavior of orthotropic plates with non-uniform thickness is important in many engineering fields.
Many research works have been done to analyze free vibration of orthotropic plates. GUPTA et al [2] studied vibration of orthotropic rectangular plate, in which, the thickness of the plate varies in both directions. LAL et al [3] presented a brief review of the work dealing with the vibrations of plates of nonhomogeneous material. JAFARI and EFTEKHARI [4] used a simple and efficient mixed Ritz-differential quadrature (DQ) method for free vibration and buckling analysis of orthotropic rectangular plates. LUO et al [5] presented a calculation method for fundamental frequency of laminated plates with arbitrary orientation under various boundary conditions. HUANG et al [6] applied a discrete method for analyzing the free vibration problem of orthotropic rectangular plates with variable thickness. XING and LIU [7] presented a new exact solution for thin orthotropic plates for three boundary conditions.
Elastic foundation is a model to represent relatively soft material in contact with plate surface, such as fluid and soil. The oldest model of the soil-structure interaction was proposed by WINKLER. Pasternak is a two-parameter elastic foundation model in which the interaction of adjacent springs is considered by connecting the ends of the springs to a shear layer. In vibration analysis of plates, the effect of elastic foundation must be considered when plates are mounted on elastic springs such as pavement of roads, footing of buildings and bases of machines. Research interest on free vibration of orthotropic plates resting on elastic foundation is still high due to more and more new fields of applications. FERREIRA et al [8] investigated the static and free vibration of rectangular plates resting on Pasternak foundations based on the collocation with radial basis functions. KUMAR and LAL [9] analyzed the transverse vibrations of thin nonhomogeneous non-uniform orthotropic rectangular plates resting on Winkler foundation using two dimensional boundary characteristic orthogonal polynomials in the Rayleigh- Ritz method. SHARMA et al [10] have analyzed free vibration of non-homogeneous orthotropic plates of variable thickness on a Winkler foundation by differential quadrature method. BAHMYARI and RAHBAR-RANJI [11] have investigated free vibration of orthotropic plates with variable thickness resting on a non-uniform Winkler foundation using free Galerkin method. HSU [12] has investigated vibrations of orthotropic rectangular plates on an elastic foundation by using differential quadrature method. LIU et al [13] have analyzed free vibration of orthotropic rectangular plates with tapered varying thickness in one and two directions resting on a Winkler elastic foundation by Galerkin method. YAN et al [14] applied Galerkin method for vibration analysis of isotropic rectangular plate with interior elastic point support and elastically restrained edges.
In this work, free vibration of a non-homogeneous orthotropic plate resting on a Pasternak foundation is examined by Ritz method. A set of orthogonal functions are generated with the aid of Gram-Schmidt orthogonalization procedure as admissible functions. Numerical results are obtained for different boundary conditions, elastic coefficients of foundation, non-homogeneity and density parameters.
2 Governing equations
Consider a non-homogeneous orthotropic thin rectangular plate with length a, width b and uniform thickness h, resting on a Pasternak foundation. Figure 1 shows the geometry of plate with considered Cartesian coordinate system in which the x-y plane lies in the mid plane of the plate.
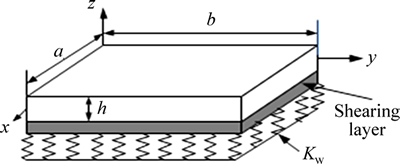
Fig. 1 Geometry and coordinate system of a plate resting on Pasternak foundation
On the basis of classical plate theory, the displacement component of the mid plane is written as
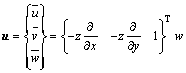 (1)
(1)
where 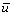 ,
, 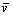 and
and 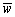 are displacement functions in x, y and z directions, respectively; and w denotes the transverse deflection of the mid plane. Linear components of the engineering strains are calculated as
are displacement functions in x, y and z directions, respectively; and w denotes the transverse deflection of the mid plane. Linear components of the engineering strains are calculated as
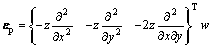 (2)
(2)
The stress–strain relations for an orthotropic elastic plate are expressed as
 (3)
(3)
where
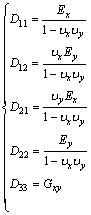 (4)
(4)
where Ex, Ey, υx and υy are the moduli of elasticity and Poisson ratio in x and y directions, respectively; Gxy is the shear modulus. The following relationship exists between these elastic constants:
 (5)
(5)
The strain energy functional of the plate due to bending is expressed as
 (6)
(6)
where εT={εxx εyy γxy} and V denotes the volume of plate. Using stress–strain relation for orthotropic plate, strain energy stored in the plate is determined as
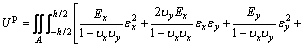
 (7)
(7)
where A is the plate area. For the sake of convenience, the following non-dimensional parameters are introduced as
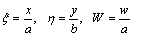 (8)
(8)
Non-homogeneity of the plate is considered by linear varying of the elastic moduli in x direction as
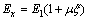 (9a)
(9a)
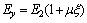 (9b)
(9b)
where E1 and E2 are constants and μ is a parameter designating the non-homogeneity.
Substituting Eqs. (2) and (9) into Eq. (7) and integrating it with respect to z, the strain energy of the plate can be expressed as
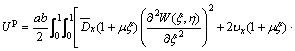
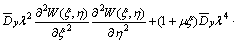
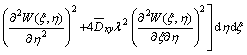 (10)
(10)
where
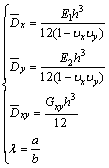 (11)
(11)
The strain energy stored in the Pasternak foundation can be expressed in a non-dimensional form as
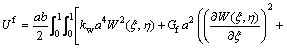
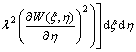 (12)
(12)
where kw and Gf are the Winkler and shearing layer elastic coefficients of foundation, respectively.
The kinetic energy of the vibrating plate is calculated as
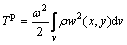 (13)
(13)
where ω is transverse velocity of mid plane, and ρ is the mass density per unit volume which is assumed to vary linearly in x direction as
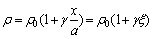 (14)
(14)
where γ is density parameter. Substituting Eq. (14) into Eq. (13) and using non-dimensional parameters, the kinetic energy of the plate is calculated as
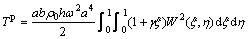 (15)
(15)
Using separation technique, the deflection of the plate can be expressed as
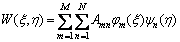 (16)
(16)
where Amn are un-known coefficients; M and N are the total numbers of admissible functions in ζ and η directions, respectively; φm(ζ) and ψn(η) are orthogonal functions which can be constructed by using a well-known procedure known as the Gram–Schmidt orthogonalization process. Implementation of this procedure needs to introduce initial functions that satisfy at least geometrical boundary conditions. These starting functions for different boundary conditions are defined as
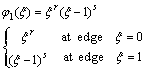 (17)
(17)
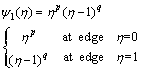 (18)
(18)
where r, s, p, q for simply supported edges are one, for clamped edges are two and for free edges are zero. As an example for SCCF boundary condition, these parameters are 1, 2, 2 and 0, respectively. The other members of the orthogonal set are generated using Gram-Schmidt process as follows [15]:
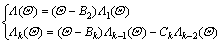 (19)
(19)
where
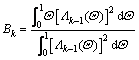 (20)
(20)
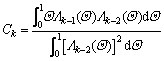 (21)
(21)
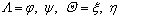
Total energy functional of plate is calculated as
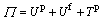 (22)
(22)
Substituting Eqs. (10), (12) and (15) into Eq. (22), the total energy functional would be as
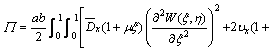
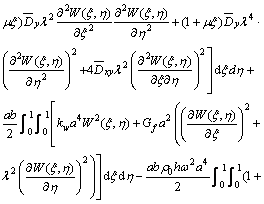
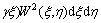 (23)
(23)
Substituting Eq. (16) into Eq. (23) and then minimizing the functional energy with respect to coefficients of admissible function leads to following governing eigenvalue equation:
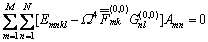 (24)
(24)
where
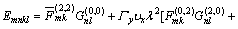
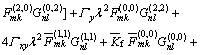
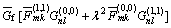 (25)
(25)
where
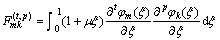 (26)
(26)
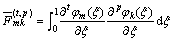 (27)
(27)
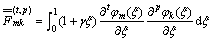 (28)
(28)
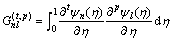 (29)
(29)
 (30)
(30)
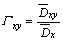 , m, k, n, l=1, 2, 3, …, t, p=0, 1, 2 (31)
, m, k, n, l=1, 2, 3, …, t, p=0, 1, 2 (31)
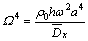 (32)
(32)
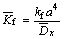 (33)
(33)
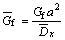 (34)
(34)
3 Numerical results and discussions
To show the accuracy and applicability of the present method, some numerical examples are solved. Table 1 shows the first six natural frequency parameters for homogeneous orthotropic plate obtained by present method and compared with exact solutions in Ref. [7]. Dimensions of plate are 1 m×1.2 m and material properties are assumed as E1=185 GPa, E2=10.5 GPa, vx=0.28 and Gxy=7.3 GPa. As can be seen, very good results are achieved for the first mode of vibration using only four terms of orthogonal polynomials in each direction, regardless of type of boundary condition. For higher mode of vibration, the number of orthogonal polynomial terms should be increased. For example, for third mode M and N equal to 10 give accurate result while for the sixth mode, M and N equal to 12 gives accurate result.
To show the applicability of the present method in determining vibration characteristics of any boundary condition, in Table 2 the first six modes for a homogenous orthotropic square plate with dimensions 1 m×1 m are determined and compared with the results from Ref. [6] and Ref. [11]. In this table, material properties are taken as E1=60.7 GPa, E2=24.8 GPa, vx=0.23 and Gxy=2 GPa. Again it can be concluded that the present method has good accuracy irrespective of boundary conditions and material properties.
Table 3 presents the frequency parameters of a homogeneous orthotropic square plate resting on Pasternak foundation with various boundary conditions and foundation parameters. In Table 2 material properties are the same as Table 1. It can be concluded that natural frequency of plate resting on the Pasternak elastic foundation is more sensitive to shearing layer coefficient rather than Winkler coefficient.
Figure 2 shows the variation of frequency parameter as a function of elastic foundation parameters. As can be seen, when shearing layer coefficient is zero and Winkler coefficient varies from 0 to 1000, frequency parameter increases only by 20%, while when Winkler coefficient is 0 and shearing layer coefficient varies from 0 to 1000, frequency parameter increases by 140%.
Table 1 Frequency parameters 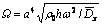 of a homogenous orthotropic plate with dimensions 1 m×1.2 m
of a homogenous orthotropic plate with dimensions 1 m×1.2 m
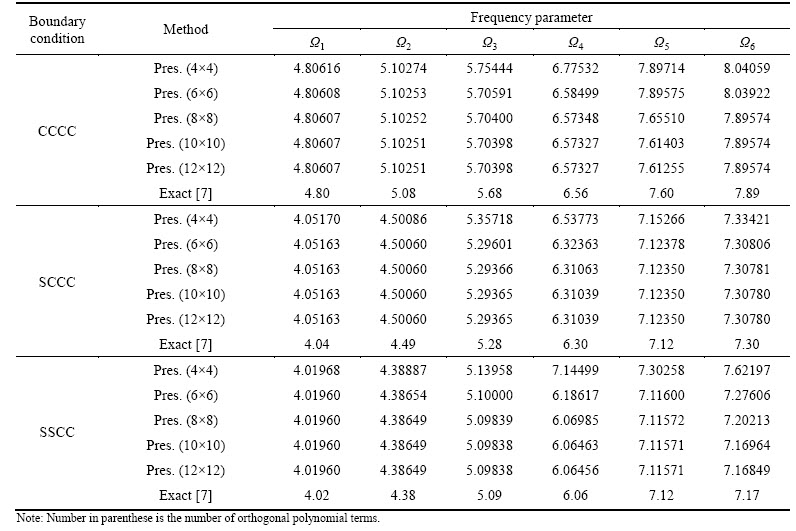
Table 2 Frequency parameter 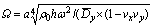 of a homogenous orthotropic plate
of a homogenous orthotropic plate
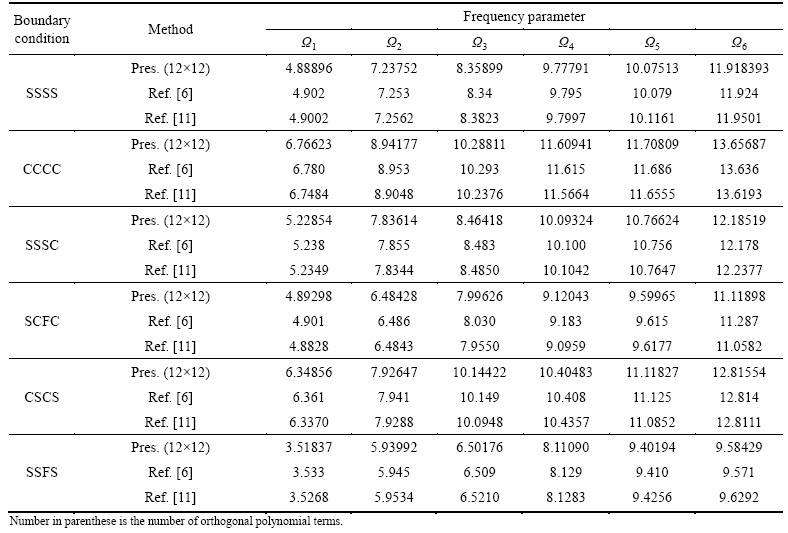
Table 3 Normalized frequency parameters 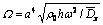 of a homogeneous orthotropic plate resting on Pasternak foundation and λ=1
of a homogeneous orthotropic plate resting on Pasternak foundation and λ=1
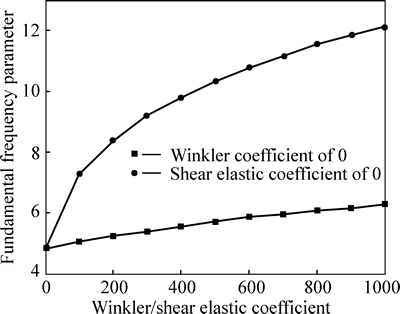
Fig. 2 Variation of frequency parameters by increasing value of foundation parameters for CCCC boundary condition
Table 4 shows the variation of vibration modes with respect to different non-homogeneity and density parameters. In Table 4 square orthotropic plate with material properties the same as Table 1 is considered resting on elastic foundation with parameters 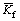 =100 and
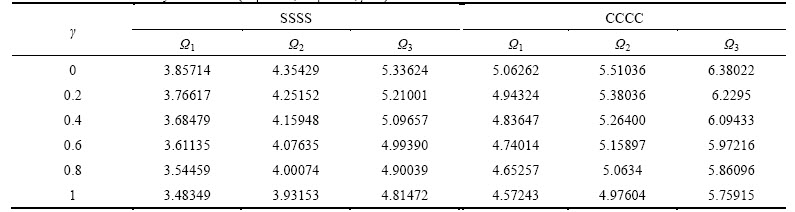
=100 and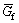 =100. As can be seen, by increasing non- homogeneity parameter, μ, natural frequency increases; however, by increasing density parameter, γ, natural frequency decreases regardless of boundary condition. To examine the effects of μ and γ on natural frequency, in Tables 5 and 6 the first three natural frequencies for a square plate on Winkler type of elastic foundation with SSSS and CCCC boundary conditions are determined as a function of μ and γ. It can be seen that density parameter has weakening effect on natural frequency and non-homogeneity parameter has direct effect on natural frequency.
=100. As can be seen, by increasing non- homogeneity parameter, μ, natural frequency increases; however, by increasing density parameter, γ, natural frequency decreases regardless of boundary condition. To examine the effects of μ and γ on natural frequency, in Tables 5 and 6 the first three natural frequencies for a square plate on Winkler type of elastic foundation with SSSS and CCCC boundary conditions are determined as a function of μ and γ. It can be seen that density parameter has weakening effect on natural frequency and non-homogeneity parameter has direct effect on natural frequency.
Table 4 Frequency parameters 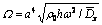 of a non-homogeneous orthotropic plate resting on Pasternak foundation (
of a non-homogeneous orthotropic plate resting on Pasternak foundation (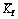 =100,
=100, 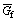 =100)
=100)
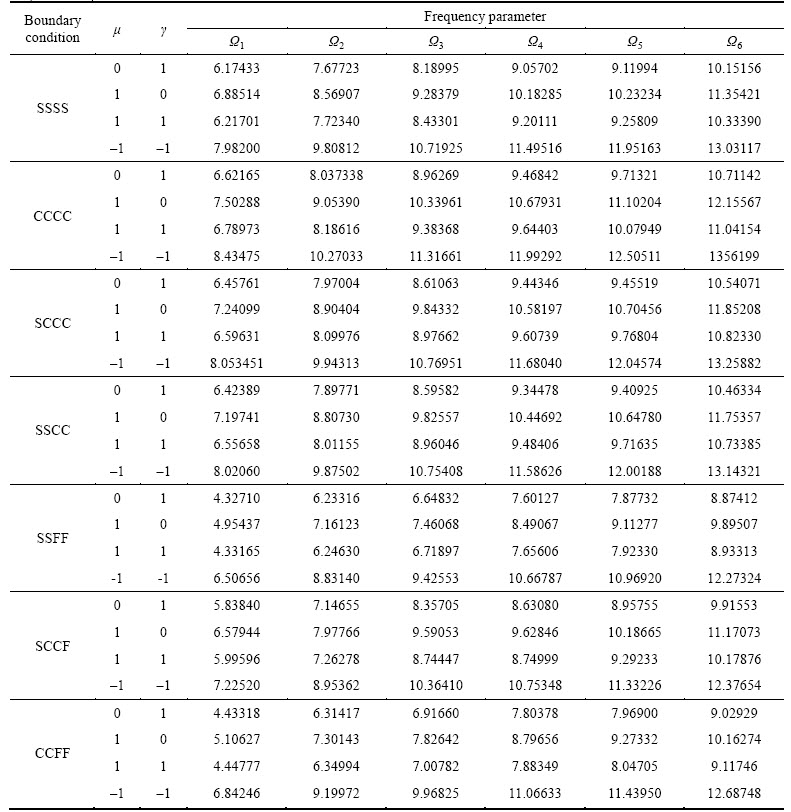
Table 5 First three frequency parameters of a non-homogeneous orthotropic plate resting on Winkler type of elastic foundation with SSSS and CCCC boundary conditions (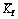 =100,
=100, 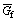 =100, γ=0)
=100, γ=0)
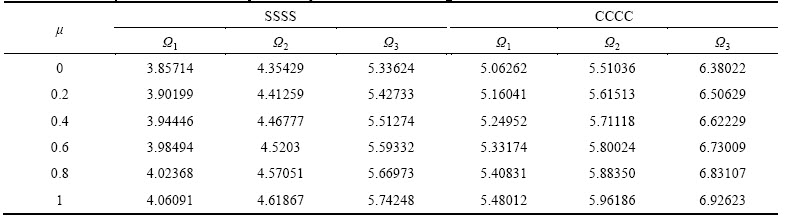
Table 6 First three frequency parameters of a non-homogeneous orthotropic plate resting on Winkler type of elastic foundation with SSSS and CCCC boundary conditions ( =100,
=100,  =100, μ=0).
=100, μ=0).
4 Conclusions
1) It is observed that the present method yields accurate results with low number of terms of orthogonal polynomials for first modes of vibration. For higher modes of vibration, higher number of terms of orthogonal polynomials should be used.
2) To show the applicability of the present method, different combinations of boundary condition, elastic foundation parameters, density and non-homogeneity parameters are considered. It is concluded that natural frequency of plates is more sensitive to shearing layer coefficient rather than Winkler coefficient.
3) It is concluded that density parameter has weakening effect on natural frequency while by increasing the non-homogeneity parameter, natural frequency increases.
References
[1] LEISSA A W. Vibration of plates [R]. NASA SP 160. Washington DC: U.S. Government Printing Office, 1969.
[2] GUPTA A K, TRIPTI JOHRI, VATS R P. Study of thermal gradient effect on a non-homogeneous orthotropic rectangular plate having bi-direction linearly thickness variation [J]. Meccanica, 2010, 45(3): 393–400.
[3] LAL R, KUMAR Y, GUPTA U S. Transverse vibrations of non-homogeneous rectangular plates of uniform thickness using boundary characteristic orthogonal polynomials [J]. Int J Appl Math Mech, 2010, 6(14): 93–109.
[4] JAFARI A A, EFTEKHARI S A. An efficient mixed methodology for free vibration and buckling analysis of orthotropic rectangular plates [J]. Applied Mathematics and Computation, 2011, 218: 2670–2692.
[5] LUO Ying-qin, HONG Ming, LIU Yuan. Analytical solutions to the fundamental frequency of arbitrary laminated plates under various boundary conditions [J]. J Marine Sci Appl, 2015, 14: 46–52.
[6] HUANG M, MA X Q, SAKIYAMA T, MATUDA H, MORITA C. Free vibration analysis of orthotropic rectangular plates with variable thickness and general boundary conditions [J]. Journal of Sound and Vibration, 2005, 288: 931–955.
[7] XING Y F, LIU B. New exact solutions for free vibrations of thin orthotropic rectangular plates [J]. Composite Structures, 2009, 89: 567–574.
[8] FERREIRA J M, ROQUE C M C, NEVES A M A, JORGE R M N, SOARES C M M. Analysis of plates on Pasternak foundations by radial basis functions [J]. Computational Mechanics, 2010, 46: 791–803.
[9] KUMAR Y, LAL R. Vibrations of nonhomogeneous orthotropic rectangular plates with bilinear thickness variation resting on Winkler foundation [J]. Meccanica, 2012, 47: 893–915.
[10] SHARMA S, GUPTA U S, SINGHAL P. Vibration analysis of non-Homogeneous orthotropic rectangular plates of variable thickness resting on Winkler foundation [J]. Journal of Applied Science and Engineering, 2012, 15: 291–300.
[11] BAHMYARI E, RAHBAR-RANJI A. Free vibration analysis of orthotropic plates with variable thickness resting on non-uniform elastic foundation by element free Galerkin method [J]. Journal of Mechanical Science and Technology, 2012, 26: 2685–2694.
[12] HSU M H. Vibration analysis of orthotropic rectangular plates on elastic foundations [J]. Composite Structures, 2010, 92: 844–852.
[13] LIU M F, CHANG T P, WANG Y H. Free vibration analysis of orthotropic rectangular plates with tapered varying thickness and Winkler spring foundation [J]. Mechanics Based Design of Structures and Machines, 2011, 39: 320–333.
[14] YAN W, WANG Zhong-min, MIAO R. Element-free Galerkin method for free vibration analysis of rectangular plates with interior elastic point, supports and elastically restrained edges [J]. Journal of Shanghai University: English Edition, 2010, 14(3): 187–195.
[15] BHAT R B. Natural frequencies of rectangular plates using characteristic orthogonal polynomials in the Rayleigh–Ritz method [J]. Journal of Sound and Vibration, 1985, 102: 493–499.
(Edited by DENG Lü-xiang)
Received date: 2015-01-27; Accepted date: 2015-04-23
Corresponding author: Ahmad Rahbar-Ranji; Tel: +98–21–64543114, Fax: +98–21–66412495; E-mail: rahbar@aut.ac.ir