J. Cent. South Univ. Technol. (2011) 18: 1272-1277
DOI: 10.1007/s11771-011-0832-1
Variation characteristics of aquifer parameters induced by groundwater source heat pump operation under variable flow
WANG Song-qing(王松庆), ZHANG Xu(张旭)
College of Mechanical Engineering, Tongji University, Shanghai 200092, China
? Central South University Press and Springer-Verlag Berlin Heidelberg 2011
Abstract: The variation characteristics of aquifer parameters, induced by groundwater source heat pump (GWSHP) operation under variable flow, were theoretically analyzed through a case study, in which the characteristics of building air conditioning load were considered. The results, compared with the constant flow operation, indicate that the influence on the variations of porosity, hydraulic conductivity and confined water head is decreased by 48%, 51% and 71%, respectively, under variable flow operation. The security of variable flow operation is superior to that of constant flow. It is also concluded that the climate region and function of the buildings are primary factors which affect the suitability of variable flow operation in GWSHP.
Key words: groundwater source heat pump; variation characteristic; aquifer parameter; variable flow
1 Introduction
Ground water source heat pump (GWSHP), widely used in China, has some advantages such as energy efficiency, reliable stability and low initial cost. Many researchers have been devoted into the research on GWSHP. LI [1] acquired an analytical solution of the groundwater seepage flow for a confined aquifer using the integral transform method, which may provide a foundation for heat transfer analysis of the standing column well system. NAM and OOKA [2] conducted the numerical simulation of water and heat transfer for the GWHP system based on an air-conditioning experiment utilizing real-scale equipment. The mathematic model of single-well circulation system of GWSHP was built [3]. AL-SARKHI et al [4] discussed the performance evaluation of standing column well for potential application of ground source heat pump in Jordon. STEFANO and MASSIMO [5] studied the feasibility of GWSHP, which provided heating and cooling in a new large commercial building. LEE and HAHN [6] evaluated and characterized the groundwater temperature data obtained from the Korean National Groundwater Monitoring Station for GWSHP application. However, less effort was put into the study on GWSHP operation under variable flow at present. The main reason was that some research results showed, compared with the operation mode of variable chilled water flow in common air conditioning unit, the energy efficiency potential of the operation mode of variable cooling water flow was relatively low. The advantages and disadvantages of the operation mode of variable cooling water flow were mainly considered from the point of energy efficiency. As the water source of GWSHP is obtained from underground space, which is different from others, the choice of operation strategy should not only consider the energy efficiency potential, but also pay more attention to the problem of particles migration induced by GWSHP operation. The variation characteristics of aquifer parameters might cause the problem of borehole collapse, even the failure of water pumping system in GWSHP. It is extremely important to choose suitable operation strategy which could give a reference to effective application of GWSHP in China.
2 Calculation of maximum groundwater flow under GWSHP full-load operation
It is essential to ascertain the maximum groundwater flow under GWSHP full-load operation, which is prior to the study on the operation of variable flow in GWSHP. The maximum groundwater flow is relevant to some basic parameters such as the performance of GWSHP, the temperature of groundwater and the air conditioning load in building. The maximum groundwater flow can be calculated through Eq.(1) under refrigerating conditions in summer [7]:
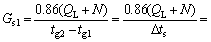
 (1)
(1)
where Gs1 is the maximum groundwater flow under refrigerating conditions in summer, QL is the maximum refrigeration capacity, tg1 is the temperature of groundwater inlet into GWSHP in summer, tg2 is the temperature of groundwater outlet from GWSHP in summer, ?ts is the temperature difference between inflow and outflow groundwater in GWSHP, and E is the energy efficiency of GWSHP.
The maximum groundwater flow can be calculated under heating conditions in winter:
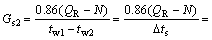
 (2)
(2)
where Gs2 is the maximum groundwater flow under heating conditions in winter, QR is the maximum heating capacity, tw1 is the temperature of groundwater inlet into GWSHP in winter, tw2 is the temperature of groundwater outlet from GWSHP in winter, and C is the coefficient of performance of GWSHP.
The design value of the groundwater flow in GWSHP is selected from the higher value between Gs1 and Gs2. The energy efficiency of GWSHP is the ratio of actual refrigeration capacity to actual consumed power in GWSHP operation. And the coefficient of performance of GWSHP is the ratio of actual heating capacity to actual consumed power in GWSHP operation. The values of E and C, with different refrigeration or heating requirements, should not be lower than specified values under nominal condition in GWSHP operation [7]. As it is seen from Eqs.(1) and (2), the necessary groundwater flow is inversely proportional to the temperature difference between inflow and outflow of groundwater in GWSHP. However, a small flow can lead to the operating efficiency of GWSHP decreasing considerably. Usually, the temperature difference between inflow and outflow of groundwater in GWSHP should be controlled within 15 °C. Some research results showed that the temperature difference between 4.5 °C and 6.5 °C was suitable [8].
3 Governing equations of particles migration
The interaction between groundwater and aquifer was proceeded with GWSHP operating. The solid particles would be separated from aquifer and the fluidized particles were transported with groundwater. It is a complex fluid-solid coupled problem in porous media. It must be carried out by quantification with relevant governing equations. The governing equations, based on the representative elementary volume method, are presented to study this problem.
3.1 Basic definitions
It is assumed that the aquifer is saturated and a volume element dV consists of the following three constituents: groundwater, fluidized particles and aquifer solid skeleton. The three constituents are illustrated in Fig.1. The masses and volumes of fluid, fluidized particles and solid skeleton are respectively denoted by mff, mfs, ms, dVff, dVfs and dVs. The basic parameters are defined as follows [9].
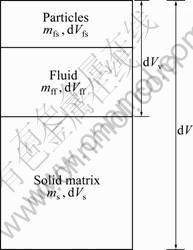
Fig.1 Schematic diagram of mixing element in representative elementary volume
1) Pore volume:
dVv=dVff+dVfs (3)
2) Porosity:
 (4)
(4)
3) Fluidized particles concentration:
 (5)
(5)
4) Average densities of fluid  fluidized particles
fluidized particles  and solid skeleton
and solid skeleton  in representative elementary volume:
in representative elementary volume:
 (6)
(6)
 (7)
(7)
 (8)
(8)
3.2 Governing equations
As for the fully penetrating well, the seepage in radial direction is dominant compared with axial direction when GWSHP operates, consequently the situation of GWSHP pumping could be regarded as a typical problem of 1-D seepage in which the axial direction is ignored. The schematic diagram is shown in Fig.2. The mathematical model presented is based on mass conservation and consideration of particle transport. It is assumed that the solid skeleton is rigid so that the elastic deformation of aquifer is not considered. The fluid is assumed to be incompressible. The governing equations of fluid, fluidized particles and solid skeleton are built based on continuity equations [9]:
 (9)
(9)
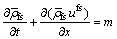 (10)
(10)
 (11)
(11)
where uff is the average actual velocity of fluid, ufs is the average velocity of fluidized particles, us is the average velocity of solid skeleton, and m is a mass-generation term, which corresponds to the rate of net mass eroded and fluidized at any time and point.
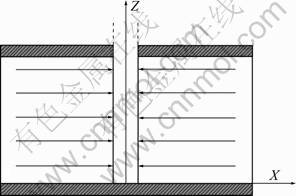
Fig.2 Schematic diagram of one dimensional flow of groundwater
It is important to simplify the mathematical model through establishing the relationship of velocities of three constituents. This issue is usually considered as follows [10]. The solid skeleton is assumed to be rigid and the velocity to be zero. It is assumed that the fluid and the fluidized particles share the same velocity at any instant. The following equations can be obtained through simplifying the previous equations and considering the basic definitions and the relationship between seepage velocity and average actual velocity:
 (12)
(12)
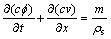 (13)
(13)
 (14)
(14)
where v is the seepage velocity, and m is the function of porosity, fluidized particles concentration and seepage velocity.
If the seepage velocity value does not exceed the critical value or the fluidized particles concentration is zero, the mass-generation term is nonexistence [11]:
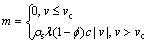 (15)
(15)
where λ is the erosion parameter and it can be seen as a constant for the same aquifer, and vc is the critical seepage velocity value, which corresponds to the initiation of particle migration.
3.3 Influence on aquifer caused by particles migration
As for the single well, the value of pumping discharge would be the maximum under full-load operation of GWSHP. It could be calculated from
Qmax=vmaxA (16)
where vmax is the maximum value of seepage velocity on borehole wall, Qmax is the maximum value of pumping discharge, and A is the pumping area.
Combined with Darcy’s law, the calculation formulas of pumping groundwater flow are [12]
Q=KJA (17)
v=KJ (18)
where K is the hydraulic conductivity of aquifer, and J is the hydraulic gradient.
These parameters are also expressed by basic equations as
 (19)
(19)
 (20)
(20)
 (21)
(21)
where k is the permeability, k0 is a constant relevant to permeability, μ is the kinetic viscosity, g is the gravitational acceleration, pB is the pore pressure on B section, pC is the pore pressure on C section, HB is the water head on B section, HC is the water head on C section, and L is the distance between B section and C section.
It can be seen that the particles migration causes the variation of porosity. However, the variation of porosity can also influence the variation characteristics of hydraulic conductivity and confined water head. They are the primary reasons that cause the failure of water pumping system in GWSHP. Consequently, the study on the influence of aquifer parameters induced by GWSHP operation under variable flow is extremely critical.
4 Influence on variation characteristics of aquifer parameters induced by GWSHP operation under variable flow
4.1 Control method of variable flow operation in GWSHP
The differential temperature method is widely used in variable cooling water flow control at present. The temperature sensors are installed on inlet tube and outlet tube of cooling water, respectively. The controllers can receive temperature signals and then compare it with designed temperature difference in order to regulate the speed of pump and flow. The speed of pump and flow could be fallen to keep the designed temperature difference under GWSHP part-load operation. Thus, the differential temperature method is considered as the main operational control method for GWSHP under variable flow. As seen from Eqs.(1) and (2), if the temperature difference between inflow and outflow groundwater in GWSHP is constant and E and C values remain approximately constant, the flow is proportional to air conditioning load. The relationship between groundwater flow and air conditioning load is built with differential temperature method. It is assumed that the temperature difference is 5 °C and E and C values are constant in this work. It is indicated that the pump and motor efficiency will decrease obviously in small flow range, and the analysis of energy efficiency has practical significance only when the flow is more than 50% of the total flow [13]. By considering the energy efficiency potential of GWSHP, the variation range of flow rate is from 50% to 100% of total groundwater flow and the accommodation amplitude is 10%. The requirement of groundwater flow is proportional to air conditioning load. If the part air conditioning load rate is lower than 50%, the value is chosen as 50%.
4.2 A case study
The annual dynamic air conditioning load is basic for the study on the operation mode of variable flow. A case study about typical office building in Shenyang was carried out. The calculation and analysis of annual dynamic air conditioning load were realized by DeST software in this case. And then, the influence on variation characteristics of aquifer parameters, induced by GWSHP operation under variable flow was discussed.
4.2.1 Calculation of air conditioning load and flow
In this case study, the GWSHP in the office building was assumed to operate from 9am to 5pm on each day, with 90 d in summer and 150 d in winter every year. The relevant calculation parameters of air conditioning load are listed in Table 1.
The maximum values of annual dynamic air conditioning load are 764.1 and 613.3 kW in summer and winter, respectively. The maximum groundwater flows can also be calculated through Eqs.(1) and (2) in summer and winter, respectively. The values of E and C are chosen from Ref.[7]. Thus, the maximum groundwater flow values are Gs1=160 m3/h, Gs2= 79.16 m3/h
As the value of Gs1 is larger than Gs2, the value of Gs1 is selected as the design reference. The pumping well adopts fully penetrating well and the amounts are three. The pumping groundwater flow and well diameter are selected as 53.33 m3/h and 700 mm, respectively, for single well. It is assumed that confined aquifer, which is composed by coarse sand, is homogeneous and isotropic and the thickness is 25 m.
Then, the distribution characteristic of annual dynamic air conditioning load in office building was analyzed. The results, as shown in Fig.3, indicate that the air conditioning system operates mostly under part load rate (PLR) of 30%-70% in summer. The time frequency of PLR, which is lower than 50%, is accounted for 61.35%. The PLR is below 50% during most time in winter. However, the time frequency of PLR, which is lower than 50%, is accounted for 90.79%. In summer, the minimum groundwater flow is 50% Gs1, which is more than Gs2. Then, the groundwater flow can be selected as 80 m3/h, which can also meet the heating requirement in winter.
4.2.2 Analysis
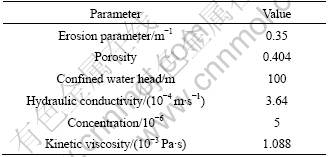
The governing equations of particles migration, which are functions of time and space, are discretized using a second-order implicit finite difference scheme. The specific parameters and initial and boundary conditions are listed in Table 2. It is assumed that the groundwater can be recharged completely and the far field water head is a constant which is 5 m away from the center of borehole, and the effects on particle migration problem caused by recharging can be neglected. The actual seepage velocity exceeds the critical value in all conditions.
Table 1 Relevant parameters of air conditioning load calculation
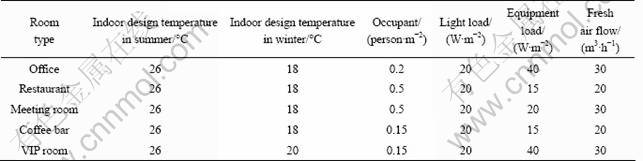
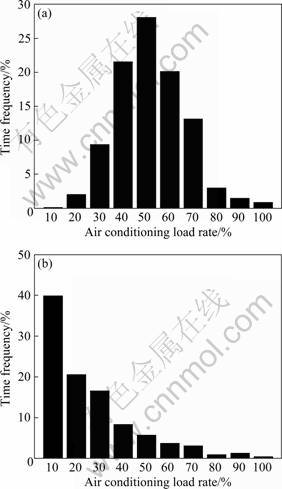
Fig.3 Time frequencies of air conditioning load rate in office building: (a) In summer; (b) In winter
Table 2 Aquifer correlation parameter
The study on different operation modes, variable flow and constant flow, was carried out for GWSHP. The flow remained a constant Gs1 in a year, which was the so-called constant flow operation mode. The influence on aquifer parameters was analyzed through comparative analyses under two conditions. The variation characteristics of porosity, hydraulic conductivity and confined water head distribution were analyzed in five-year operation of GWSHP with different operation modes. The calculated results of porosity variation indicate that the particles migration can be proceeded under GWSHP operation if the actual seepage velocity exceeds the critical value.
Firstly, the porosity, as shown in Fig.4, increases by 6.48% and 3.39% than initial one on borehole wall, respectively, after five-year operation of GWSHP with different operation modes. Compared with the constant flow mode, the effect on porosity variation is decreased by 48% under variable flow operation. Thus, the operation under variable flow in GWSHP, by considering the characteristics of air conditioning load in building, is superior to the constant flow. It is indicated that the reliability of the variable flow operation is better than constant flow through case study.
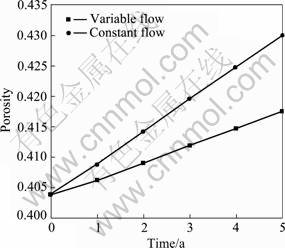
Fig.4 Porosity variations on borehole wall with different operation modes
Secondly, the variation characteristic of hydraulic conductivity, as shown in Fig.5, is obtained from Eqs.(19) and (20) combined with porosity variation. The results indicate that the hydraulic conductivity on borehole wall increases gradually with porosity variation annually and the variation tendency is more obvious as the operation time increases. For different operation modes, the hydraulic conductivity increases by 32.1% and 15.8% than the initial one on the borehole wall, respectively, after five years. It is indicated that the porosity influences the hydraulic conductivity distinctly even when the variation of porosity is small. The influence on the hydraulic conductivity variation, compared with the constant flow operation, is decreased by 51% under variable flow operation.
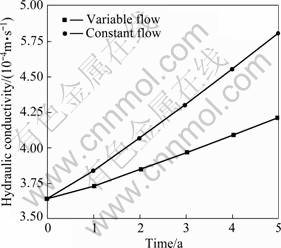
Fig.5 Hydraulic conductivity variations on borehole wall with different operation modes
Finally, the variation characteristics of confined water head are analyzed through the variation characteristics of hydraulic conductivity, which are induced by GWSHP operation under different modes. The variation characteristics of confined water head are obtained from Eqs.(17)-(21) and are shown in Fig.6. The results indicate that the water head on borehole wall increases gradually with hydraulic conductivity variation annually. The confined water head increases by 0.68% and 0.2% than initial one on borehole wall, respectively, after five years. Compared with the variation characteristics of porosity and hydraulic conductivity, the variation of confined water head is relatively little. However, the variable flow operation, which influences the variation characteristic of confined water head, is only 29% compared with constant flow. Consequently, the constant flow operation impacts the variation characteristics of confined water head obviously than variable flow.
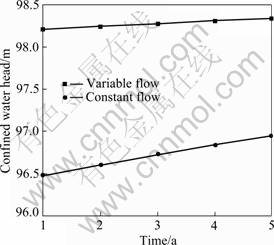
Fig.6 Confined water head variations on borehole wall with different operation modes
5 Conclusions
1) Compared with the constant flow operation, the influence on the variations of porosity, hydraulic conductivity and confined water head are decreased by 48%, 51% and 71%, respectively, under variable flow operation combined with the characteristics of air conditioning load in building. The reliability of the variable flow operation is superior to constant flow. The variable flow operation is a reasonable operation mode that can effectively prevent the failure problem of water pumping system in GWSHP.
2) The influence on the variation characteristics of aquifer parameters is associated with the characteristics of air conditioning load directly. The climate region and function of the buildings are primary factors which affect the suitability of variable flow operation in GWSHP.
References
[1] LI M. Analysis of seepage flow in a confined aquifer with a standing column well [J]. Journal of Hydrodynamics, 2007, 19(1): 84-91.
[2] NAM Y J, OOKA R. Numerical simulation of ground heat and water transfer for groundwater heat pump system based on real-scale experiment [J]. Energy and Buildings, 2010, 42(1): 69-75.
[3] REES S J, SPITLER J D, DENG Z, ORIO C D, JOHNSON C N. A study of geothermal heat pump and standing column well performance [J]. ASHRAE Transaction, 2004, 110(1): 3-13.
[4] AL-SARKHI A, ABU-NADA E, NIJMEH S, AKASH B. Performance evaluation of standing column well for potential application of ground source heat pump in Jordan [J]. Energy Conversion and Management, 2008, 49(4): 863-872.
[5] STEFANO L R, MASSIMO V C. Open-loop groundwater heat pumps development for large buildings: A case study [J]. Geothermics, 2009, 38(3): 335-345.
[6] LEE J Y, HAHN J S. Characterization of groundwater temperature obtained from the Korean national groundwater monitoring stations: Implication for heat pumps [J]. Journal of Hydrology, 2006, 329(3/4): 514-526.
[7] LU Yao-qing. Practical heating air conditioning design manual [M]. China Architecture & Building Press, 2008: 2362-2375. (in Chinese)
[8] ZHANG Mou-xiong. Performance of the chiller with variable chilled-water flow [J]. Journal of HV&AC, 2000, 30(6): 56-58. (in Chinese)
[9] STAVROPOULOU M, PAPANASTASIOU P, VARDOULAKIS I. Coupled wellbore erosion and stability analysis [J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1998, 22(9): 749-769.
[10] XUE S, YUAN Y. Sanding process and permeability change [J]. Journal of Canadian Petroleum Technology, 2007, 46(4): 33-39.
[11] WAN R G, WANG J. Modelling sand production and erosion growth under combined axial and radial flow [J]. Journal of Canadian Petroleum Technology, 2004, 43(12): 33-38.
[12] PAPAMICHOS E, VARDOULAKIS I. Sand erosion with a porosity diffusion law [J]. Computers and Geotechnics, 2005, 32(1): 47-58.
[13] LI Su-long, ZOU Na. Control methods for variable condenser water flow in air conditioning systems [J]. Journal of HV&AC, 2005, 35(12): 51-54. (in Chinese)
(Edited by YANG Bing)
Foundation item: Project(2006BAJ01B05) supported by the National Science and Technology Pillar Program during the 11th Five-Year Plane Period
Received date: 2010-09-15; Accepted date: 2010-12-25
Corresponding author: ZHANG Xu, Professor, PhD; Tel: +86-21-65984243; E-mail: zhangxu-hvac@tongji.edu.cn