Unified analytical solution for deep circular tunnel with consideration of seepage pressure, grouting and lining
来源期刊:中南大学学报(英文版)2017年第6期
论文作者:李学峰 杜守继 CHEN Bing-kui(陈兵奎)
文章页码:1483 - 1493
Key words:unified strength theory (UST); intermediate principal stress; seepage pressure; grouting; lining; analytical solution
Abstract: A new unified analytical solution is presented for predicting the range of plastic zone and stress distributions around a deep circular tunnel in a homogeneous isotropic continuous medium. The rock mass, grouting zone and lining are assumed as elastic-perfectly plastic and governed by the unified strength theory (UST). This new solution has made it possible to consider the interaction between seepage pressure, lining, grouting and rock mass, and the intermediate principal stress effect together. Moreover, parametric analysis is carried out to identify the influence of the related parameters on the plastic zone radius. Under the given conditions, the results show that the plastic zone radius decreases with an increasing cohesion, internal friction angle and hydraulic conductivity of lining and unified failure criterion parameter, respectively; whereas the plastic zone radius increases with the growth of elasticity modulus of lining. Comparison of results from the new solution and the other published one shows well agreement and provides confidence in the new solution proposed.
Cite this article as: LI Xue-feng, DU Shou-ji, CHEN Bing. Unified analytical solution for deep circular tunnel with consideration of seepage pressure, grouting and lining [J]. Journal of Central South University, 2017, 24(6): 1483-1493. DOI: 10.1007/s11771-017-3552-3.
J. Cent. South Univ. (2017) 24: 1483-1493
DOI: 10.1007/s11771-017-3552-3
LI Xue-feng(李学峰), DU Shou-ji(杜守继), CHEN Bing(陈兵)
Department of Civil Engineering, Shanghai Jiao Tong University, Shanghai 200240, China
 Central South University Press and Springer-Verlag Berlin Heidelberg 2017
Central South University Press and Springer-Verlag Berlin Heidelberg 2017
Abstract: A new unified analytical solution is presented for predicting the range of plastic zone and stress distributions around a deep circular tunnel in a homogeneous isotropic continuous medium. The rock mass, grouting zone and lining are assumed as elastic-perfectly plastic and governed by the unified strength theory (UST). This new solution has made it possible to consider the interaction between seepage pressure, lining, grouting and rock mass, and the intermediate principal stress effect together. Moreover, parametric analysis is carried out to identify the influence of the related parameters on the plastic zone radius. Under the given conditions, the results show that the plastic zone radius decreases with an increasing cohesion, internal friction angle and hydraulic conductivity of lining and unified failure criterion parameter, respectively; whereas the plastic zone radius increases with the growth of elasticity modulus of lining. Comparison of results from the new solution and the other published one shows well agreement and provides confidence in the new solution proposed.
Key words: unified strength theory (UST); intermediate principal stress; seepage pressure; grouting; lining; analytical solution
1 Introduction
For deep tunnel engineering, the accurate assessment of plastic zone radius and stress redistribution are crucial aspects of the planning, design and construction as well as operating periods. For this purpose, different closed-form solutions based on different failure criterions for axisymmetric circular tunnel in infinite medium with initial hydrostatic in-situ stress have been presented. All of these solutions include simplifying assumptions, and commonly assume the rock mass governed by either the linear Mohr-Coulomb (M-C) [1-3] or non-linear Hoek-Brown (H-B) [4-7] failure criterion. However, neither M-C nor H-B failure criterion considers the effect of intermediate principal stress on rock strength. In fact, the strength potential of rock mass has closely relationship with the intermediate principal stress effect [8]. Among different strength theories, unified strength theory (UST) has good performance in that it is capable of reflecting the intermediate principal stress effect for different materials under various stress states [9]. In recent years, several analytical solutions for deep tunnel based on the UST have been proposed in Ref. [10-14].
Considering the effect of seepage pressure, analytical solutions of ground stresses and displacements for deep circular tunnel in saturated ground are derived by LI et al [3], however their solutions neglected the influence of grouting and intermediate principal stress effect. Although several solutions presented by ZHANG et al [11-13] have considered the intermediate principal stress effect while grouting effect was not considered in their solutions. In addition, the solutions in ZENG et al [10, 14] highlighted the intermediate principal stress effect and the different yielding conditions of support, while the seepage pressure and grouting effect were neglected. Their studies were complemented by HU et al [2], and an analytical solution for deep circular tunnel is presented by considering the influence of seepage pressure and grouting, but the intermediate principal stress effect is not revealed
Note that all these solutions are insightful to identify the development of plastic zone and stress distribution surrounding a deep circular tunnel, but none of them have made it possible to allow the interaction between seepage pressure, lining and grouting as well as rock mass and the effect of intermediate principal stress to be evaluated comprehensively.
The key objective of this work is to propose a new unified analytical solution for deep circular tunnel subject to seepage pressure and in-situ stress under plane strain conditions. This new solution should allow the interaction between seepage, lining, grouting as well as rock mass to be evaluated and different effects of the intermediate principal stress under different stress states to be considered. It is assumed that lining, grouting and rock mass are elastic-plastic and governed by the UST. The other published solution is used to validate the new solution. In addition, parametric study is also carried out to investigate the influence of intermediate principal stress and the lining mechanical properties on the plastic zone radius. In this work, as a rule, tensile stress is taken as positive.
2 Unified strength theory (UST)
The UST, expanded by YU et al.[9], is known for it can take the effect of intermediate principal stress into account in the non-linear unified failure criterion, and can be expressed both in terms of principal stresses and shear strength parameters (cohesion c and internal friction angle φ). Its regular expression is as follows:
When  ,
,
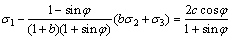 (1a)
(1a)
When 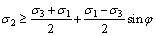 ,
,
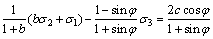 (1b)
(1b)
where σ1, σ2 and σ3 are major, intermediate and minor principal stresses, respectively. The unified failure criterion parameter b reflects the influence of intermediate principal stress, and different values of b relates different failure criterion, with 0≤b≤1. The value of b can be determined from material mechanical tests.
3 Description of problem
A sketch model of the proposed problem is shown in Fig. 1. In this model, R0 and R1 are the inner and outer radius of the lining. R2 is the outer radius of grouting circle, and R3 is the outer boundary of rock mass in the problem. Pw0 and Pw3 are the water pressure act on the inner face of lining (i.e. R=R0) and the far-field stable water pressure boundary (i.e. R=Rw3), respectively. The in-situ stress q acts at outer radius of rock mass R3. kl, kg and kr are the hydraulic conductivity of the lining, the grouting and the rock mass, respectively. For the lining, El and μl are the elasticity modulus and Poisson ratio as well as cl and φ1 are the cohesion and internal friction angle, respectively; for the grouting, correspondingly to Eg and μg as well as cg and φg; for the rock mass, correspondingly to Er and μr as well as cr and φr. Rlp, Rgp and Rrp are plastic zone radius in lining, grouting circle and rock mass, respectively. Plp, Pgp, and Prp are the boundary stresses at plastic zone radius Rlp, Rgp and Rrp, respectively. P1 and P2 are boundary stresses at R1 and R2. The excavation of deep circular tunnel is assumed in a homogeneous, isotropic, initially linear elastic rock mass. During tunnel excavation period, it is also assumed that the lining and grouting are constructed immediately after the excavation, so the stress relief can be neglected. Therefore, the lining will share the majority loads transferred from grouting and rock mass so that plastic zone occurs first in lining. And once the stresses exceed yield strength of grouting and rock mass, the plastic zone will then expands further to grouting circle and rock mass. In this work, three plastic states are considered, i.e. the elastic-plastic interface occurs in the lining correspondingly to Fig. 1(a), in the grouting correspondingly to Fig. 1(b) and in the rock mass correspondingly to Fig. 1(c), respectively.
In terms of the seepage field around tunnel, following assumptions are made:
1) The water is incompressible, and Darcy’s law is applicable.
2) The water flow surrounding the tunnel can be assumed as axial symmetry with respect to the tunnel center, and steady-state groundwater flow.
3) The flow is radial.
Based on the above simplified assumptions, the seepage pressure around a circular tunnel can be derived in polar coordinate as follows [15]:
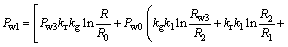
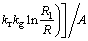 , R0
, R0
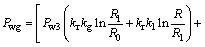
 , R1
, R1
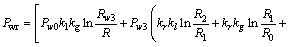
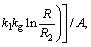 R2
R2
where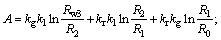 Pwl, Pwg and Pwr are the seepage pressure acting on the lining, grouting and rock mass,respectively.
Pwl, Pwg and Pwr are the seepage pressure acting on the lining, grouting and rock mass,respectively.
On the assumption of axial symmetric plane strain conditions, the relationship between the intermediate principal stress and the out-of-plane stress σz follows σ2=σz=m(σR+σθ)/2 [16], in which the m is in the range of 2μ≤m≤1 and μ is the Poisson ratio. For simplicity, it is assumed that m=2μ and m=1 in the elastic and plastic zone, respectively. As a result, σ2=(σ1+σ3)/2<(σ1+σ3)/ 2+sinφ(σ1-σ3)/2, thus Eq. (1a) should be adopted. With consideration of seepage pressure Pw, Eq. (1a) can be written as follows:
For σ1=σR and σ3=σθ,
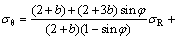
 (3a)
(3a)
For σ1=σθ and σ3=σR,
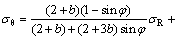
 (3b)
(3b)
where η is the equivalent surface porosity of the rock mass. Determined from field and laboratory studies, η is generally close to unity and always greater than 0.85 in Ref. [17], and thus in this work η=1 was used.
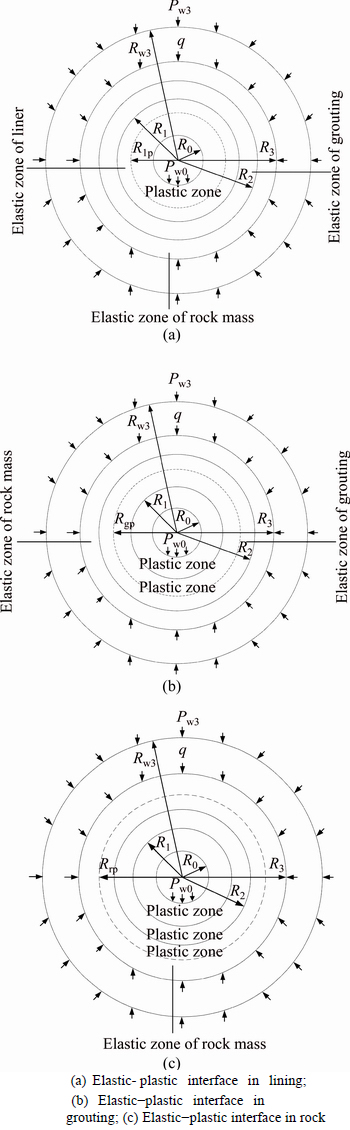
Fig. 1 Schematic illustration of analytical model:
4 New unified analytical solution based on UST
For case of σ1=σR and σ3=σθ, three plastic states in tunnel construction period will be explored in this study, namely, the elastic-plastic interface is in lining, in grouting circle and in rock mass, respectively.
4.1 Elastic-plastic interface in lining (R0≤Rlp≤R1)
In this situation, the lining is under the elastic- plastic state, namely, it has both plastic and elastic zones; the grouting circle and rock mass are still in elastic state, as shown in Fig. 1(a).
For axisymmetric problem, the equilibrium equation taking seepage pressure Pw into account in polar coordinates can be written as
 (4)
(4)
where the subscripts R and θ indicate the radial and tangential directions, respectively. By introducing Eq. (2a) and Eq. (3a) with c=cl and φ=φl for the lining into Eq. (4), and with the stress boundary condition σRpl=-Pw0 at R=R0, the stress in the plastic zone of lining can be expressed as follows:
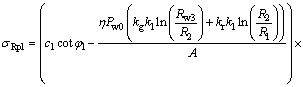
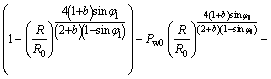
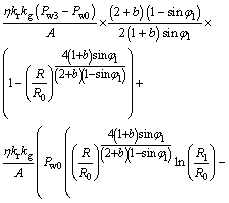
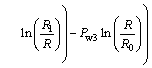 (5)
(5)
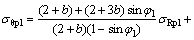
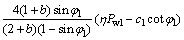 (6)
(6)
with following stress boundary conditions:
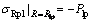
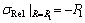
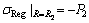
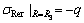 (7)
(7)
Based on elastic theory, the stress and displacement in the elastic zone of the lining can be derived as follows:
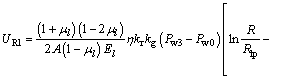
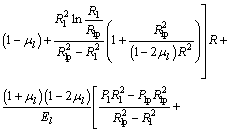
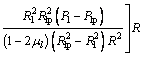 (8)
(8)
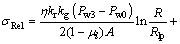
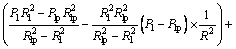
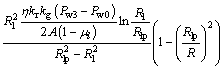 (9)
(9)
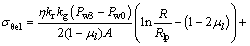
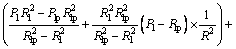
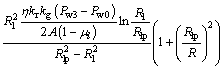 (10)
(10)
And the stress and displacement in the elastic zone of the grouting are as follows:


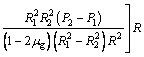 (11)
(11)
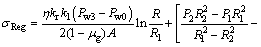
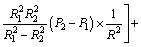
 (12)
(12)
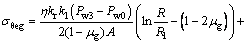
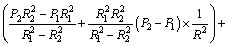
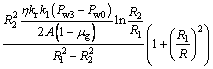 (13)
(13)
And the stress and displacement in the elastic zone of the rock mass are as follows:
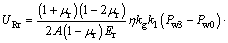
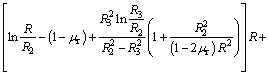
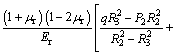
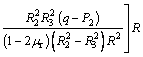 (14)
(14)
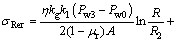
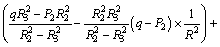
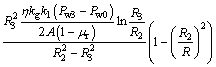 (15)
(15)
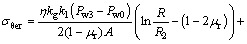
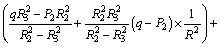
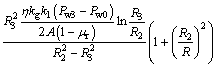 (16)
(16)
Based on the continuity of displacement fields at the interface between grouting and lining, i.e., at R=R1:
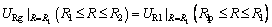 (17a)
(17a)
and at the interface between grouting and rock mass, i.e., at R=R2
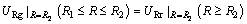 (17b)
(17b)
At the elastic-plastic interface of lining, i.e., at R=Rlp, the stress satisfies the following equation:
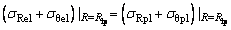 , i.e.,
, i.e.,
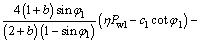
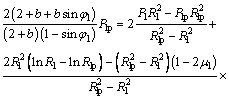
 (18)
(18)
With Eq. (17a) and Eq. (17b), the expressions of boundary stress P1 and P2 in terms of Rlp and Plp can be obtained, and with Eq. (18), a transcendental equation can be deduced to find the radius of plastic zone Rlp by iterative method. By introducing the value of R=Rlp into Eq. (5) the value of Plp can be obtained. Then (P1, P2), (σRer, σθer, URr), (σRel, σθel, URl) and (σReg, σθeg, URg) can be obtained, and so forth.
4.2 Elastic-plastic interface in grouting (R1≤Rgp≤R2)
In this situation, grouting circle is in elastic-plastic state, i.e., it has both plastic and elastic zones, correspondingly to Fig. 1(b). The rock mass is in elastic state, while lining is in fully plastic state. And at R=R1, plastic stress P1 can be obtained as follows:
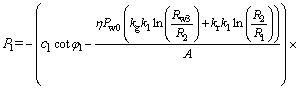

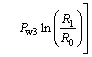 (19)
(19)
By introducing Eq. (2b) and Eq. (3a) with c=cg and φ=φg for the grouting circle into Eq. (4), and with the stress boundary condition σRpg=-P1 at R=R1, the stress in the plastic zone of grouting circle can be expressed as follows:
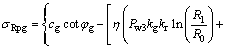



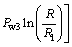 (20)
(20)
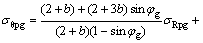
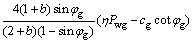 (21)
(21)
With following boundary conditions:
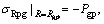
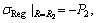
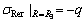 (22)
(22)
and based on elastic theory, the radial and tangential stresses and displacement in the elastic zone of the grouting circle can be derived as follows:
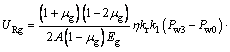
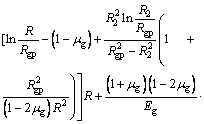
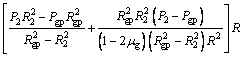 (23)
(23)
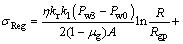
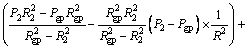
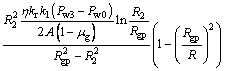 (24)
(24)
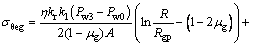
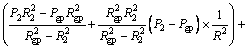
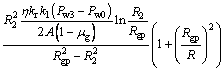 (25)
(25)
For the elastic zone of rock mass, the stress and displacement field are the same as Eqs. (14)-(16).
At R=R2, i.e., interface between grouting circle and rock mass, displacement continuity equation can be obtained as follows:
 (26)
(26)
At the elastic-plastic interface of grouting circle, i.e., at R=Rgp, the stress satisfies the following equation:
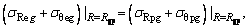 i.e.,
i.e.,
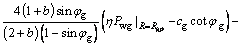
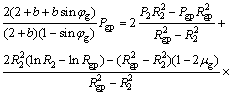
 (27)
(27)
With Eq. (26), the expressions of boundary stress P2 in terms of Rgp and Pgp can be obtained, and then with Eq. (27), a transcendental equation can be deduced to find the plastic zone radius Rgp by iterative method. And then the values of (Pgp, P2) can be obtained. Furthermore, the plastic and elastic stress fields for grouting circle can be readily obtained from Eqs.(20)- (25).
4.3 Elastic-plastic interface in rock mass (R2≤Rrp≤R3)
In this situation, rock mass is in elastic-plastic state, i.e., it has both plastic and elastic zone, correspondingly to Fig. 1(c), while lining and grouting are in entirely plastic state. And at R=R2, plastic stress P2 can be obtained as follows:
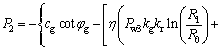
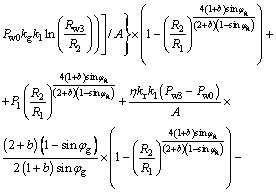
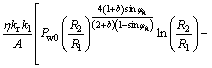
 (28)
(28)
By substituting Eq. (2c) and Eq. (3a) with c=cr and φ=φr for the rock mass into Eq. (4), and with the stress boundary condition σRpr=-P2 at R=R2, the stress in the plastic zone of rock mass can be expressed as follows:
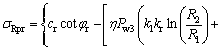

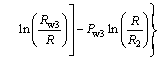 (29)
(29)
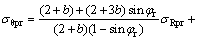
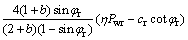 (30)
(30)
With following stress boundary condition:
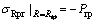 ,
,  (31)
(31)
the displacement and stress in the elastic zone of rock mass can be written as follows:
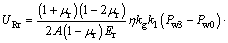
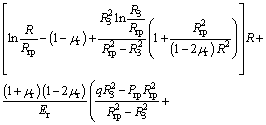
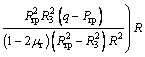 (32)
(32)
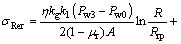
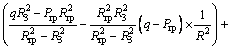
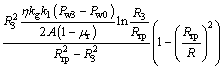 (33)
(33)
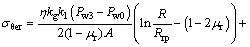
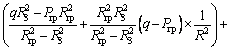
 (34)
(34)
At the elastic-plastic interface of rock mass, i.e., at R=Rrp, the stress satisfies the following equation:
 ,i.e.,
,i.e.,
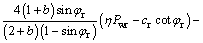
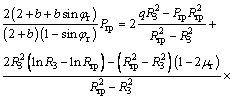
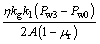 (35)
(35)
With Eqs. (19) and (28), the values of P1 and P2 can be obtained, and then, a transcendental equation can be deduced by Eq. (35) to find the plastic zone radius Rrp by iterative method. And then the value of Prp can be obtained. Furthermore, the plastic and elastic stress fields for rock mass can be readily obtained from Eqs. (29)- (34).
It should be noted that Eqs. (5)-(35) are derived for the case σ1=σR and σ3=σθ. While for the case σ1=σθ and σ3=σR, the yielding condition should be Eq. (3b), then the stress and radius of plastic zone need to be developed again by the similar procedure.
This new unified analytical solution can be used for elastic-plastic analysis of a deep circular tunnel by considering the effect of intermediate principal stress and interaction between seepage pressure, lining, grouting and rock mass, and it embraces some previous solutions for similar problem. For example, the solution presented in Ref. [2] can be generated from this new solution with b=0; while the solution developed in Ref. [10] can obtained using cg=cr, Eg=Er, μg=μr, φg=φr and η=0 from this new solution. Therefore, solutions in Refs. [2, 10] are special cases of the new proposed solution in this work. Another solution for a deep circular tunnel proposed in Ref. [3] can also be obtained from this new analytical solution with cg=cr, Eg=Er, μg=μr, φg=φr and b=0. In other words, the previous solutions based on the M-C failure criterion can be obtained from this new unified analytical solution with b=0.
By adopting different values of b within its limits, additional new results which consider different yield criterions can be obtained. Consequently, the new unified analytical solution proposed in this work is a series of results instead of one specific solution and it is suitable for a wide range of rock mass by considering different factors according to different engineering conditions.
5 Validation for new unified analytical solution
To validate the proposed solution, the same example presented by ZENG et al [10] was used. Figure 2 shows perfectly agreement between the plastic zone radius computed with the ZENG’s solution [10] and with the new unified analytical solution by setting η=0 and cg=cr, Eg=Er, μg=μr, φg=φr. This confirms the theoretical validity of the new solution in section 4. Moreover, Fig. 2 also indicates that the plastic zone radius by considering seepage pressure is larger than that of without consideration of seepage pressure. This comparison illustrates the necessity and importance of considering the seepage pressure in the elastic-plastic analysis of deep circular tunnel. Furthermore, Fig. 2 also reveals that plastic zone radius will be effectively reduced by grouting between lining and rock mass (the properties of grouting are shown in Table 1).
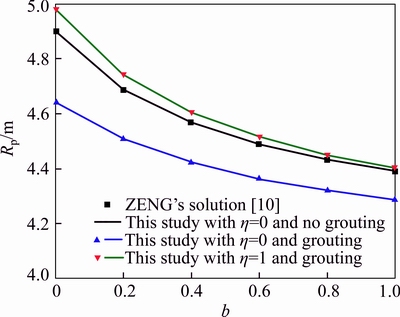
Fig. 2 Comparison of plastic zone radius between this work and ZENG’s solution [10]
Table 1 Material mechanical properties and geometry parameters (modified from Ref. [2])

6 Parameter analysis
To identify the effect of intermediate principal stress and properties of lining on the plastic zone radius, A MATLAB program is used to carry out the computations for the new analytical model. The parameters used in the computation are listed in Table 1.
In parametric analysis for investigating the effect of each parameter, the value of one parameter is varied in reasonable range, while the other parameters are kept constant as in Table 1. It should be pointed out that the parametric analysis mainly focuses on the case of elastic-plastic interface in lining. The parametric analysis for the case of elastic-plastic interface in grouting and rock mass is not illustrated herein. In addition, the conditions that ensure the elastic-plastic interface in lining, grouting and rock mass are not focus of this wrok.
6.1 Effect of unified failure criterion parameter
Figure 3 presents plastic zone radius for different unified failure criterion parameter b. It can be seen that the plastic zone radius is significantly affected by the value of parameter b. As expected, the plastic zone radius is reduced with an increase of b for all the cases analyzed (η=1 and η=0). The reason for this is that of no effect of intermediate principal stress is considered for b=0, while for b=1, the strength potentials of lining are achieved by taking the effect of intermediate principal stress into account. An increase in b value from 0 to 1 leads to a reduction of nearly 6.95% and 4.8% in plastic zone radius for η=1 and η=0, respectively. This comparison indicates that analytical solutions based on M-C (i.e., b=0) failure criterion are more conservative and give an overprediction for plastic zone radius. Furthermore, the radius of plastic zone for considering influence of seepage pressure (η=1) is larger than that of without considering of it (η=0). Such differences are important, in particular, for the tunnel preliminary design or excavation in the saturated rock or ground.
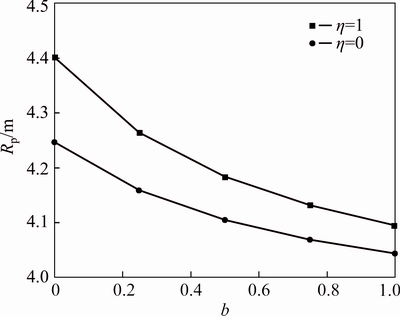
Fig. 3 Effect of b on radius of plastic zone
6.2 Effect of inner friction angle of lining
Figure 4 shows the effect of φl (inner friction angle of lining) on the plastic zone radius. It is found that plastic zone radius reduces with the increase of φl. Under given conditions, a growth in φl value from 40° to 50° leads to a reduction of nearly 10.4% and 5.04% in plastic zone radius for b=0 and b=1, respectively. When b increases from 0 to 1, the plastic zone radius decreased by 10.1% and 4.7% under φl=40° and φl=50°, respectively. Among the considered three levels of φl, the combination φl=50°, b=1 generates the minimum plastic zone radius, while the φl=40°, b=0 gives the maximum one. This can be attributed that an increase in φl value enhances the shear strength, thus it is more difficult to reach the plastic state. These trends indicate that both inner friction angle of lining and the intermediate principal stress affect the development plastic zone in the lining.
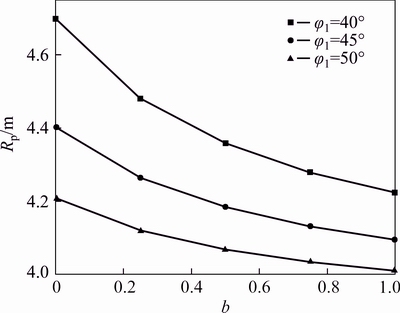
Fig. 4 Effect of b and inner friction angle of lining on radius of plastic zone
6.3 Effect of cohesion of lining
The effect of cl ( cohesion of lining) on the radius of plastic zone is shown in Fig. 5. Three levels of cl (cl=4 MPa, 5 MPa and 6 MPa) are considered. It is found that both cl and unified strength theory parameter b affect the plastic zone radius. Figure 5 shows that, with the growth of cl the reduction of plastic zone radius gradually gets small between b=0 and b=1. For example, when b=0 compared with b=1, plastic zone radius is decreased by 10.6 % and 6.6% for cl=4 MPa and cl=6 MPa, respectively. This may be attributed to the fact that different lining has different intermediate principal stress effect. The poor quality of lining (for example, cl=4 MPa in this work) has a pronounced effect of intermediate principal stress, whereas the intermediate principal stress plays a less significant role for good quality of lining (for example, cl=6 MPa in this work). Furthermore, when b=1, the plastic zone in lining almost vanish for cl=6 MPa; while for cl=4 MPa, the plastic zone of lining still exists. This might be due to an increase in cl value increases the shear strength of lining.
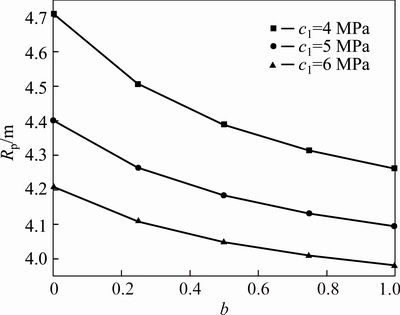
Fig. 5 Effect of b and cohesion of lining on radius of plastic zone
6.4 Effect of elasticity modulus of lining
As shown in Fig. 6, El (elasticity modulus of lining) also influences the development of plastic zone in lining, and the plastic zone radius increases with growth of El. This may be attributed to the fact that the increase in El may improve the constraint ability of the lining deformation and may lead a larger stress; thus it is easier to reach plastic state. For instance, an increase in El value from 25 to 35 GPa leads to a growth in plastic zone radius from 4.4008 to 4.61 m for b=0. Furthermore, when b increases from 0 to 1, there is 6.95%, 7.78% and 8.54% reduction of plastic zone radius for El=25, 30 and 35 GPa, respectively. The reason for this reduction is similar to the effect of intermediate principal stress.
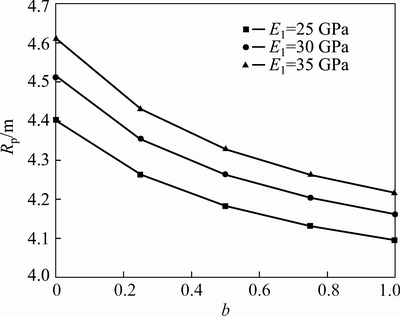
Fig. 6 Effect of b and elasticity modulus of lining on radius of plastic zone
6.5 Effect of hydraulic conductivity of lining
The influence kl (hydraulic conductivity of the lining) on the plastic zone radius is illustrated in Fig. 7. It is found that the plastic zone radius is reduced with an increasing of kl. This may be attributed to the fact that a growth in kl enhances the drainage of underwater, thus the seepage force decreases and reduces the range of plastic zone. For instance, a growth in kl value from 1×10-8 to 1×10-4m/s leads to a reduction of 2.4% in plastic zone radius for b=0; with parameter b increases from 0 to 1, plastic zone radius decreases by 7%, 5.62% and 5.1% for cases kl=1×10-8m/s, kl=1×10-6m/s and kl=1×10-4m/s, respectively. Among the studied cases,the case b=0, kl=1×10-8m/s gives the maximum radius of plastic zone, while the case b=1, kl=1×10-4m/s gives the minimum one.
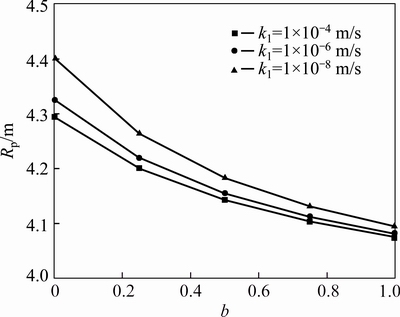
Fig. 7 Effect of b and hydraulic conductivity of lining on radius of plastic zone
From Figs. 3-7, there is a same tendency that plastic zone radius decreases with the increasing unified failure criterion parameter b for all cases studied no matter how different mechanical parameter of lining. This fact shows that intermediate principal stress should be properly considered in tunnel engineering both for safety and economical purpose.
7 Conclusions
1) The new unified solution considers the interaction between seepage pressure, lining, grouting and rock mass, and the effect of the intermediate principal stress. With different value of unified failure criterion parameter b, different analytical solutions can be obtained from this new solution, and previous analytical solutions that based on the Mohr-Coulomb (M-C) failure criterion are special cases of the new analytical solution. Therefore, more choices are available in the new solution to give more realistic analysis.
2) The plastic zone radius decreases with the unified failure criterion parameter b increases. It is concluded that strength potentials of lining can be better achieved by considering the effect of intermediate principal stress. In addition, the radius of plastic zone in the case of considering influence seepage pressure (η=1) is larger than that of without considering the influence of it (η=0).
3) The influence of the mechanical properties of lining on radius of plastic zone is also obvious and is necessary to consider in the deep tunnel elastic-plastic analysis. The plastic zone radius decreases with the increase of cl (cohesion of lining), φl (internal friction angle of the lining) and kl (hydraulic conductivity of the lining), respectively. However, the radius of plastic zone increases with the growth of El (elasticity modulus of the lining).
References
[1] MASSINAS S A , SAKELLARIOU M G. Closed-form solution for plastic zone formation around a circular tunnel in half-space obeying Mohr–Coulomb criterion [J]. Géotechnique, 2009, 59(8): 691-701.
[2] HU Li-sheng, WANG Jian-xiu, LU Yao-ru. Elastoplastic solution for deep tunnel considering influences of groundwater, grouting and lining [J]. Rock and Soil Mechanics, 2012, 33(3): 757-766. (in Chinese)
[3] LI Peng-fei, Qian Fang, ZHANG Ding-li. Analytical solutions of stresses and displacements for deep circular tunnels with linings in saturated ground [J]. Journal of Zhejiang University: Science A (Applied Physics & Engineering), 2014, 15(6): 395-404.
[4] SHARAN S K. Analytical solutions for stresses and displacements around a circular opening in a generalized Hoek-Brown rock [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(1): 78-85.
[5] HUANG Fu, ZHANG Dao-bing, SUN Zhi-bin, WU Ben. Influence of pore water pressure on upper bound analysis of collapse shape for square tunnel in Hoek-Brown media [J]. Journal of Central South University of Technology, 2011, 18(2): 530-535.
[6] ARRANZA-TORRES C. Elasto-plastic solution of tunnel problems using the generalized form of the Hoek-Brown failure criterion [J]. International Journal of Rock Mechanics and Mining Sciences, 2004, 41(3): 480-481.
[7] CHEN Ran, TONON F. Closed-form solutions for a circular tunnel in elastic-brittle-plastic ground with the original and generalized Hoek–Brown failure criteria [J]. Rock Mechanics and Rock Engineering, 2011, 44(2): 169-178.
[8] CAI M. Influence of intermediate principal stress on rock fracturing and strength near excavation boundaries-Insight from numerical modeling [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(5): 763-772.
[9] YU Mao-hong. Unified strength theory and its applications [M]. Berlin: Springer, 2004: 129-172.
[10] ZENG Kai-hua, XU Jia-xiong. Unified semi-analytical solution for elastic-plastic stress of deep circular hydraulic tunnel with support yielding [J]. Journal of Central South University, 2013, 20(6): 1742-1749.
[11] ZHANG Chang-guang, ZHAO Jun-hai, ZHANG Qing-he, HU Xiang-dong. A new closed-form solution for circular openings modeled by the unified strength theory and radius-dependent Young’s modulus [J]. Computers and Geotechnics, 2012, 42: 118-128.
[12] ZHANG Chang-guang, WANG Jiang-feng, ZHAO Jun-hai. Unified solutions for stresses and displacements around circular tunnels using the unified strength theory [J]. Science China: Technological Sciences, 2010, 53(6): 1694-1699. (in Chinese)
[13] ZHANG Chang-guang, ZHANG Qing-he, ZHAO Jun-hai, XU Fei, WU Chuang-zhou. Unified analytical solutions for a circular opening based on non-linear unified failure criterion [J]. Journal of Zhejiang University: Science A (Applied Physics & Engineering), 2010, 11(2): 71-79.
[14] ZENG Kai-hua, LI Jian-hua, XU Jia-xiong, YE Qing. Analytical solution for stresses of deep hydraulic tunnel considering linear yielding [J]. Journal of Central South University: Science and Technology, 2012, 43(3): 1131-1137. (in Chinese)
[15] CAI Mao-hong, CAI Yong-ping. Structural stress calculation for hydraulic pressure tunnel [M]. Beijing: ChinaWater & PowerPress, 2004: 19-20. (in Chinese)
[16] LI Jian-chun, MA Guo-wei, YU Mao-hong. Penetration analysis for geo-material based on unified strength criterion [J]. International Journal of Impact Engineering, 2008, 35(10): 1154-1163.
[17] TERZAGHI K. Simple tests determine hydrostatic uplift [J]. Engineering News Record. 1936, 116(25): 872-875.
(Edited by DENG Lü-xiang)
Cite this article as: LI Xue-feng, DU Shou-ji, CHEN Bing. Unified analytical solution for deep circular tunnel with consideration of seepage pressure, grouting and lining [J]. Journal of Central South University, 2017, 24(6): 1483-1493. DOI: 10.1007/s11771-017-3552-3.
Foundation item: Project(51378309) supported by National Natural Science Foundation of China
Received date: 2015-09-09; Accepted date: 2016-05-11
Corresponding author: LI Xue-feng, PhD; Tel: +86-15121036238; E-mail: sdwywd@163.com

