Label
随便看看

目录结构

详情信息展示

参考文献

稀有金属2009年第2期

钨合金丝材无模拉拔成形温度场及流动应力梯度

张士宏 齐广霞

沈阳理工大学材料科学与工程学院

中国科学院金属研究所

摘 要：

简述了目前钨合金丝材加工技术特点及变形过程存在的技术问题, 分析了采用无模拉拔成形技术加工钨合金丝材的可行性及变形机制。通过实验研究, 确定了钨合金丝材无模拉拔成形的温度场模型和流动应力模型, 确定了钨合金丝材无模拉拔成形变形区温度梯度及流动应力梯度。研究结果表明, 钨合金丝材无模拉拔成形时, 当Z≤ZM时变形区温度场沿轴向呈线性分布;当Z>ZM时变形区温度场沿轴向呈非线性分布, 温度梯度沿轴向呈线性分布;变形区流动应力场沿轴向接近线性分布, 流动应力梯度接近于常数。

关键词：

无模拉拔;钨合金丝材;温度场;流动应力梯度;

中图分类号： TG359

收稿日期：2007-09-04

基金：辽宁省教育厅科学研究计划资助项目 (2004D020);

Temperature Field and Yield Stress Grads during Wire Die-Less Drawing of Tungsten Alloy

Abstract：

The deformation characteristics, the technological problems, the deformation mechanism and feasibility of wire die-less drawing of tungsten alloy were analyzed.The model of temperature field and flow stress were determined.The grads model of temperature field and flow stress were studied by experiment and theory analysis.Results showed that the temperature field of deformation presented linear law along axes when Z≤ZM during wire die-less drawing of tungsten alloy, and the temperature field of deformation presented non-linear law along axes when Z>ZM, temperature grads and yield stress presented linear law along axes, and yield stress grads approached constant along axes.

Keyword：

die-less drawing;tungsten alloy wire;temperature field;yield stress grads;

Received： 2007-09-04

钨合金材料从大直径拉拔成直径为0.1 mm时, 需要经过近35道次拉拔; 在拉拔过程中, 拉丝模承受较大的摩擦力、 压力和拉力; 拉丝模一般采用硬质合金模或金刚石模, 成本较高; 由于摩擦的存在, 拉拔过程中加剧应力和变形不均匀分布, 使金属的变形抗力增加, 降低模具的使用寿命及产品的表面质量; 拉拔时提高温度可以使加工硬化过程减弱, 金属变形抗力降低, 拉拔力减小, 但由于温度的提高也可能降低润滑剂润滑性能, 摩擦力增加, 变形力增大, 因此拉拔时金属的最佳变形温度较难确定 [1,2] 。

Schade [3] 提出丝材拉拔成形的主要技术问题包括闷车、 断丝、 表面质量差等, 从而引起成品率低、 加工时间长等。 Zhang Xinquan [4] 采用有限元法分析了钨合金丝材拉拔时的工艺参数及合理模具结构, 并通过实验确定了钨合金材料的应力-应变关系, 给出了拉拔力、 变形均匀性、 应力分布等。 Weygand [5] 分析了钨合金丝材拉拔加工中出现的断丝现象及产生原因, 采用有限元法对拉拔变形进行了数值模拟, 给出合理的工艺参数范围。 Anon [6] 分析了钨合金丝材拉拔模具的磨损以及产生原因, 并提出了改进措施, 如采用合理润滑剂、 模具圆角半径、 模具材料选择等。 Doeland [7] 对钨合金丝材拉拔的技术问题进行了研究和讨论, 并分析了钨合金丝材拉拔成形的特点。 钨合金丝材具有很好的高温力学性能和导电导热性能, 广泛应用于灯丝等发光发热仪器, 同时钨合金丝材还广泛应用于热处理用加热设备的线圈等。 Mordyuk [8] 研究了超声波拉拔对单晶体钨合金线材的组织结构、 高温性能、 物理性能等的影响, 并分析了位错的分布等。

在钨合金丝材研究方面, Mordyuk B N等 [8] 采用超声处理技术对钨合金丝材拉拔工艺进行处理, 超声技术除了可以降低钨合金的变形抗力, 还可以改变组织结构, 提高耐高温持久性, 钨合金单晶体的蔓延规律, 确定了钨合金性能与位错分布之间的关系。 Browning P F等 [9] 分析了钨合金丝材在拉拔成形时断裂的原因是残余拉伸应力引起的, 并提出了避免断裂产生的具体措施, 如保持高的拉拔温度, 并实现了七道次的拉拔工序。 Briant C L等 [10] 研究了钨合金丝材在拉拔成形时出现的初次再结晶和二次再结晶, 如果采用低的加热速率, 二次再结晶的温度就相对较高。 Lee M等 [11] 研究了钨合金拉拔成形时润滑剂的选择与使用, 认为钨合金拉拔工艺必须具备的条件是完全液态润滑剂、 避免快速加热、 防止润滑剂氧化和反应等。 其他学者研究了在钨合金丝材表面涂覆钾化合物, 给出了一些关键技术参数 [12,13] 。

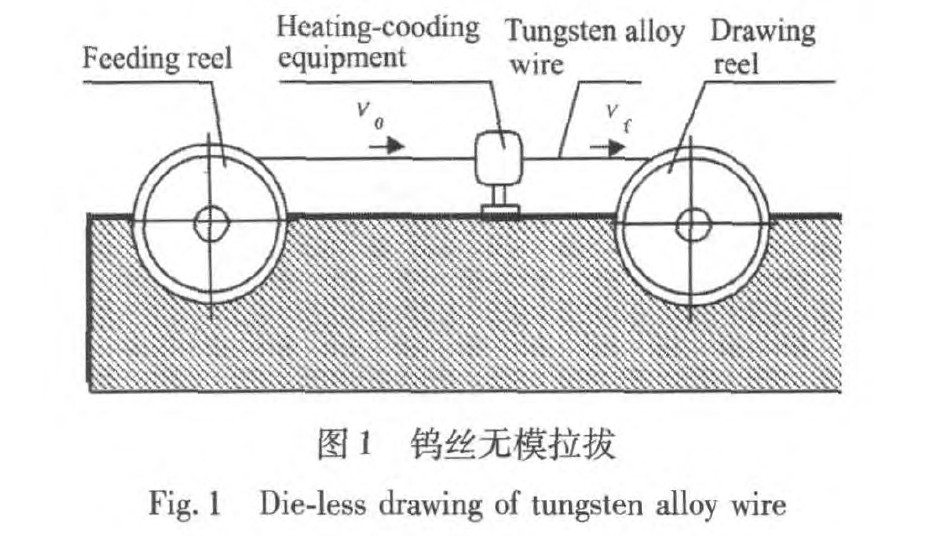
日本学者Sekiguchi H等采用一种无模成形技术成功加工出理想的碳钢和不锈钢材料变断面轴类件 [14] 。 本文作者将无模成形技术应用于钨合金丝材拉拔成形中, 定义为无模拉拔成形技术, 获得了满意的结果。 采用无模拉拔成形技术, 如图1所示, 不采用模具和润滑剂, 从而可以克服有模加工存在的缺点, 而且装置简单, 另外在拉伸过程中对钨丝进行某些热处理。 无模拉伸时采用电磁感应加热方法, 采用水冷却或空气冷却 [14] 。 Symmons G R [15] 采用液体流动学应用于拉拔工艺中获得了很好的润滑效果。 Yamada Y [16] 等应用梯度温度场实现了无模拉伸技术, 并加工出Ni-Ti合金线材。 Wengenroth [17] 对无模拉伸成形开展了理论和实验研究。 Wang [18] 提出了无模拉伸成形力能参数理论计算模型。 Carolan [19] 研究了无模拉伸成形在高碳钢中的应用。 Fortunier [20] 对无模拉伸成形的稳定性及条件进行了研究。 Weidig [21] 研究无模拉伸成形对材料组织性能的影响规律。 Wang [22] 研究了无模拉伸成形在不锈钢管材加工中的应用及其对尺寸精度的影响。 Wright [23] 介绍了有关无模拉伸成形的基础理论。 Gliga [24] 介绍了无模拉伸成形的基本理论及应用等关键技术问题。 Tiernan [25] 研究了无模拉伸成形方法在线材拉伸中的生产及应用。 前人的研究结果也提到温度场的分布规律, 但没有对温度梯度和流动应力梯度模型进行深入理论分析。

目前, 国内外对钨合金丝材的主要加工方法是拉丝工艺, 包括静液挤压拉伸、 流体静力润滑拉丝、 流体动力润滑拉丝等, 本文研究了采用无模拉拨成形技术加工钨合金丝材的可行性及变形机制。

1 钨合金无模拉拔成形变形特点

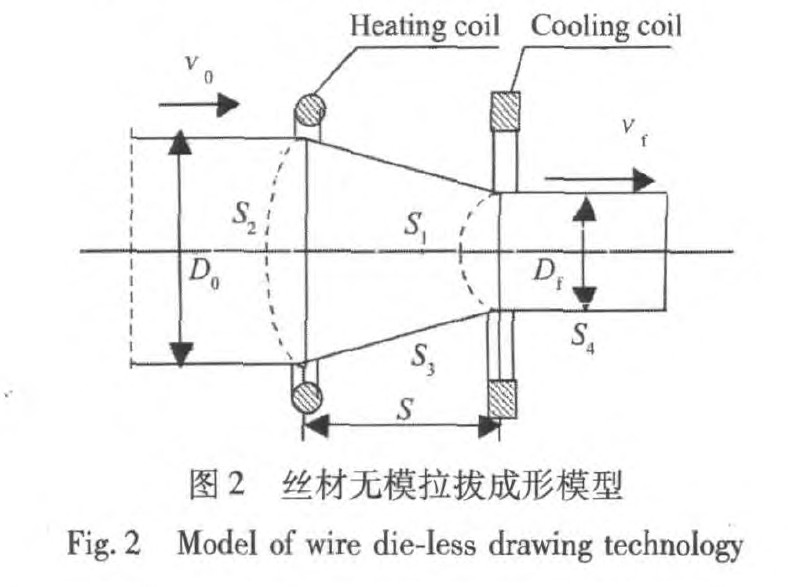
无模拉拔成形工艺是不使用模具仅靠金属变形抗力随温度变化的性质实现的柔性塑性加工技术, 是一种高精度、 高效率、 低能耗、 无污染、 少无切削柔性近终成形技术。 其基本原理是采用感应加热线圈对材料进行局部地加热到高温, 而感应加热线圈和冷却喷嘴 (简称冷热源, 可以采用水冷或者风冷) 则固定不动, 冷热源左侧线材以一定的进给速度向右移动, 另外以一定的速度拉伸线材的右端, 只要给定拉伸速度与进给速度的比值, 就可以获得所需的线材断面尺寸, 所获得的线材的断面减缩率由速度的比值确定, 见图1。 由于此方法无摩擦且属于金属热加工的一种形式, 故即使材料的可加工性低, 也可以获得较大的断面减缩率。 图2所示为钨合金丝材无模拉拔成形模型。

2 温度分布实验结果及分析

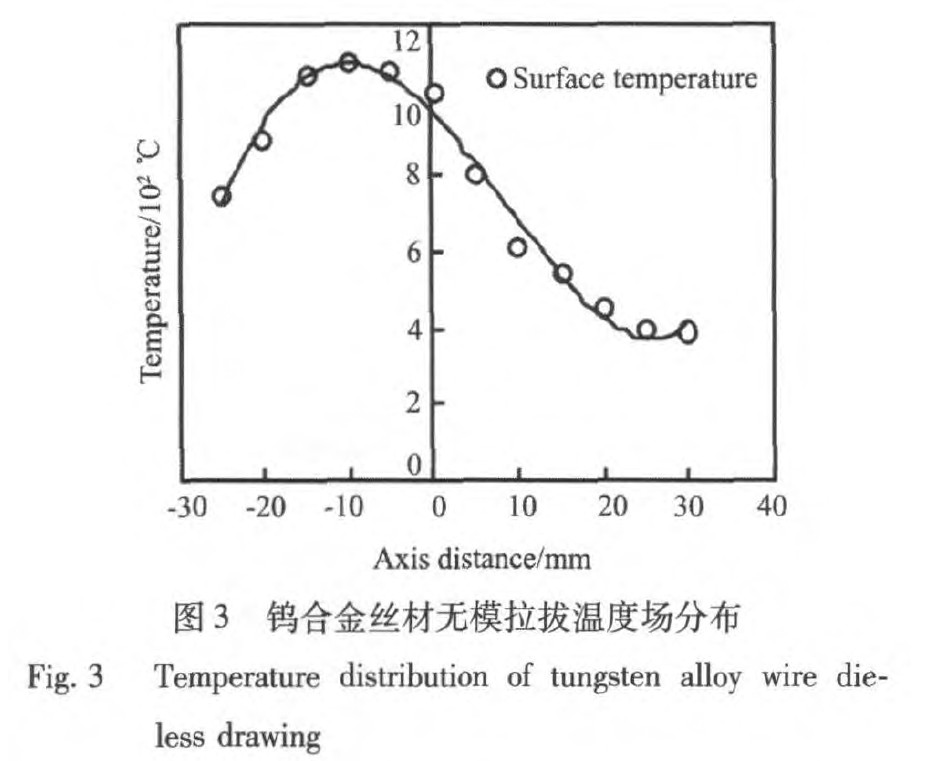
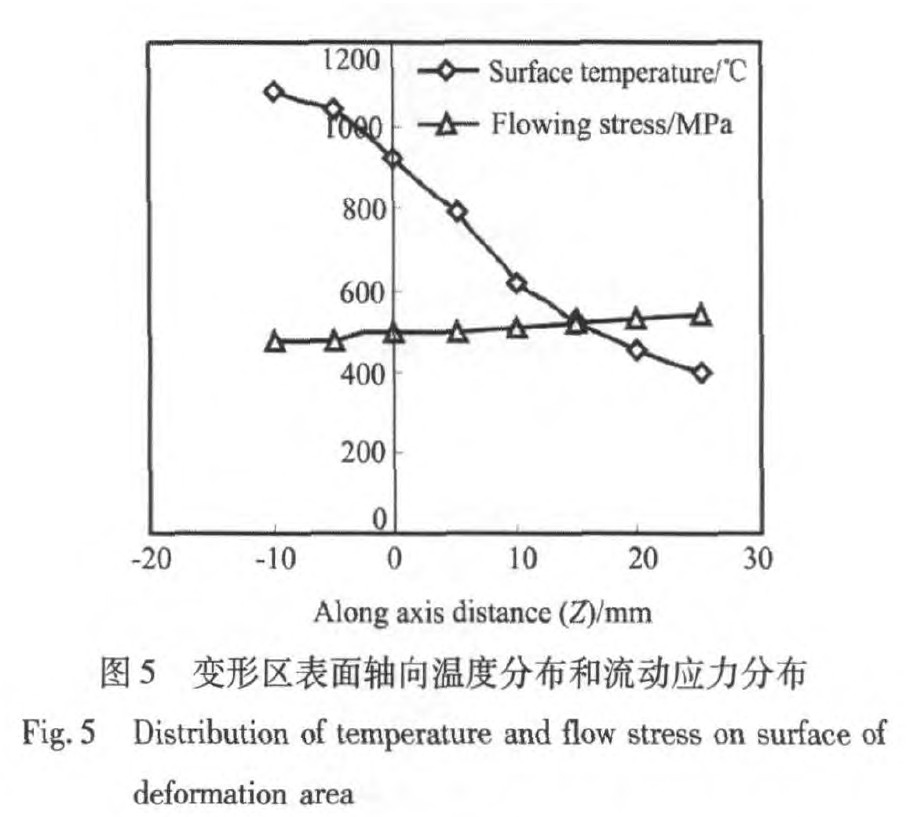
无模拉拔时, 由于变形区温度梯度而产生的流动应力梯度是无模拉拔成形过程稳定进行的前提条件, 因此对变形区温度分布进行分析有利于建立温度场数学模型, 为变形区温度场及变形过程数值模拟准备必要的知识。 实验材料为W1钨合金。 钨合金加热方法采用高频感应加热, 采用红外测温方法测试变形区表面温度。 无模拉拔时变形区表面温度分布的测试结果见图3。 对实验测试结果进行回归分析, 得到了温度场分布的数学模型:

Τ={24.4Ζ+1377??当-S≤Ζ≤ΖΜ0.2152Ζ2-25.27Ζ+907.55?当ΖΜ≤Ζ≤S???(1)

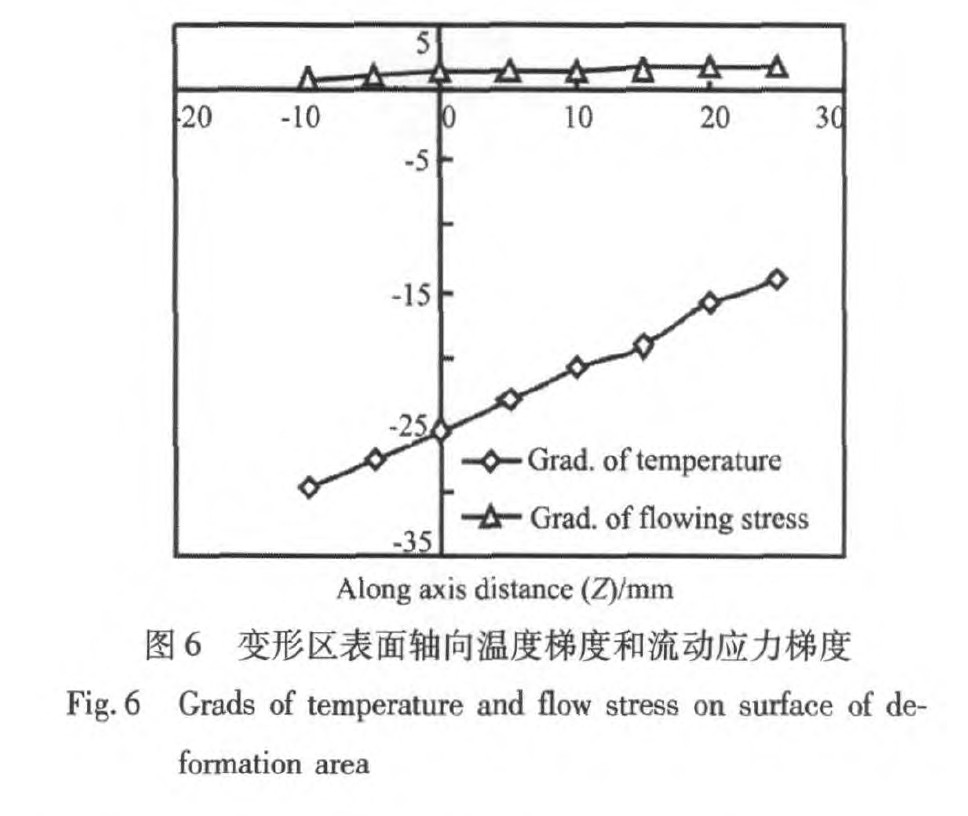
式中, T为温度, ℃; S为冷热源间距, mm; Z为轴向坐标, 原点在加热线圈的中心处 (图2) ; ZM=S/2。 则拉伸件表面轴向温度梯度:

dΤdΖ={24.4??当-S≤Ζ≤ΖΜ0.4304Ζ-25.27?当ΖΜ≤Ζ≤S???(2)

3 流动应力及流动应力梯度

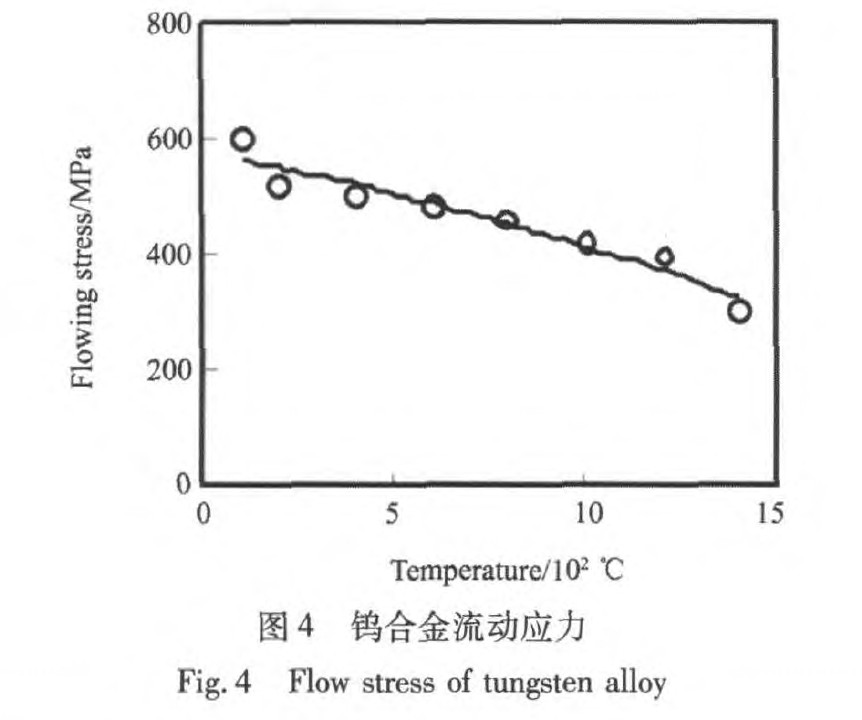
由于无模拉拔时变形区温度分布呈山峰形, 即变形区每一点温度值不相同, 所以无模拉拔变形区中流动应力分布也不同。 在理论计算时, 需要确定变形区的流动应力分布规律。 采用热模拟实验方法测得了W1钨合金材料的流动应力σs与温度的关系, 测试结果见图4。 对应于图4的曲线拟合函数见式 (3) :

σs=-4×10-5T2-0.1228T+575.78 (3)

将式 (1) 代入式 (3) 得到流动应力:

σs={0.0238Ζ2-0.3525Ζ+482.5?当-S≤Ζ≤ΖΜ0.01419Ζ2+1.272Ζ+497.2?当ΖΜ≤Ζ≤S???(4)

流动应力梯度:

dσsdΖ={0.0476Ζ-0.3525??当-S≤Ζ≤ΖΜ0.0284Ζ+1.272??当ΖΜ≤Ζ≤S???(5)

变形区温度和流动应力沿轴向分布如图5所示, 变形区温度梯度和流动应力梯度沿轴向分布如图6所示。 从图5和图6可以看出, 表面温度沿轴向呈非线性分布, 流动应力和温度梯度沿轴向都接近线性分布, 流动应力梯度接近于常数。

4 结 论

1. 钨合金丝材无模拉拔成形时, 当Z≤Zm时变形区温度场沿轴向呈线性分布; 当Z≥Zm时变形区温度场沿轴向呈非线性分布, 温度梯度沿轴向呈线性分布。

2. 钨合金丝材无模拉拔成形时, 变形区流动应力场沿轴向接近线性分布, 流动应力梯度接近于常数。

参考文献

[1] 郑良永.钨丝工艺学[M].上海:上海科学技术出版社, 1996

[2] 印协世.钨丝生产原理、工艺及其性能[M].北京:冶金工业出版社, 1998.

[3] Schade P.Wire drawing failures and tungsten fracture phenome-na[J].International Journal of Refractory Metals and Hard Ma-terials, 2006, 24 (4) :332.

[4] Zhang Xinquan, Jin Qijiang, Wang Zutang.Rigid plastic FEManalysis of the effect of pass reduction and die angle on the qualityof the tungsten drawing wire product[J].Rare Metals (inChin.) , 1987, 6 (1) :1.

[5] Weygand S M, Riedel H, Eberhard B.Numerical simulation ofthe drawing process of tungsten wires[J].International Journalof Refractory Metals and Hard Materials, 2006, 24 (4) :338.

[6] Anon P J.Tungsten carbide wire drawing dies Part II[J].Wire Industry, 1992, 59 (701) :391.

[7] Doeland O M, Maaren A C.Optimization of tungsten-wiredrawing process based on certain theories of wire drawing[J].Wire, 1976, 26 (5) :187.

[8] Mordyuk B N, Mordyuk V S.Ultrasonic drawing of tungstenwire for incandescent lamps production[J].Ultrasonics, 2004, 42 (1-9) :109.

[9] Browning P F, Briant C L, Rajan K.An analysis of splittingfailures during the drawing of tungsten wire[J].EngineeringFailure Analysis, 1995, 2 (2) :105.

[10] Briant C L, Horacsek O, Horacsek K.The effect of wire historyon the coarsened substructure and secondary recrystallization ofdoped tungsten[J].Metallurgical Transactions A-Physical Metal-lurgy and Materials Science, 1993, 24 (4) :843.

[11] Lee M, Flom D.Lubrication mechanisms in tungsten and mo-lybdenum wire drawing[J].Lubrication Engineering, 1991, 47 (2) :135.

[12] Harmat P, Bartha L, Grosz T.The rate of bubble deformationduring tungsten wire drawing[J].International Journal of Re-fractory Metals&Hard Materials, 2002, 20 (4) :295.

[13] Grosz T, Borbely S, Harmat P.Small-angle neutron scatteringstudy of potassium-doped tungsten wire samples[J].MaterialsScience Forum, 2001, 378 (3) :446.

[14] Sekiguchi H, Kobatake K.Development of dieless drawingprocess[J].Advanced Technology of Plasticity, Proc.2nd ICTP[C].1987.347.

[15] Symmons G R.Performance comparison of ploymer fluids indieless wire drawing[J].Journal of Materials Process Technolo-gy.1994, 43 (5) :13.

[16] Yamada Y.Applications of dieless drawing to Ni-Ti wire and ta-pered steel wire manufacture[J].Kobe Res.Dev. (in Jap.) , 1992, 42 (2) :93.

[17] Wengenroth W, Pawelski O.Theoretical and experimental in-vestigations into dieless drawing[J].Steel Research, 2001, 72 (10) :402.

[18] Wang Z T, Luan G F, Bai G R.Study of the deformation veloc-ity field and drawing force during the dieless drawing of tube[J].Journal of Materials Processing Technology, 1999, 94 (2) :73.

[19] Carolan R, Tiernan P, Commerford P.The dieless drawing ofhigh carbon steel[J].Materials Science Forum, 2004, 447-448:513.

[20] Fortunier R, Sassoulas H, Montheillet F.Thermo-mechanicalanalysis of stability in dieless wire drawing[J].InternationalJournal of Mechanical Sciences, 1997, 39 (5) :615.

[21] Weidig Ursula, Kaspar Radko, Pawelski.Multiphase micro-structure in steel bars produced by dieless drawing[J].Steel Re-search, 1999, 70 (4-5) :172.

[22] Wang Z T, Zhang S H, Luan G F.Experiment study on thevariation of wall thickness during dieless drawing of stainless steeltube[J].Journal of Materials Processing Technology, 2002, 120 (1-3) :90.

[23] Wright N, Wright E.Basic analysis of dieless drawing[J].Wire Journal International, 2000, 33 (5) :138.

[24] Gliga M, Canta T.Theory and application of dieless drawing[J].Wire Industry, 1999, 66 (785) :294.

[25] Tiernan P, Hillery M.An investigation of the dieless drawingmethod for the production of mild steel wire[J].Wire JournalInternational, 1999, 32 (12) :94.

[1] 郑良永.钨丝工艺学[M].上海:上海科学技术出版社, 1996

[2] 印协世.钨丝生产原理、工艺及其性能[M].北京:冶金工业出版社, 1998.

[3] Schade P.Wire drawing failures and tungsten fracture phenome-na[J].International Journal of Refractory Metals and Hard Ma-terials, 2006, 24 (4) :332.

[4] Zhang Xinquan, Jin Qijiang, Wang Zutang.Rigid plastic FEManalysis of the effect of pass reduction and die angle on the qualityof the tungsten drawing wire product[J].Rare Metals (inChin.) , 1987, 6 (1) :1.

[5] Weygand S M, Riedel H, Eberhard B.Numerical simulation ofthe drawing process of tungsten wires[J].International Journalof Refractory Metals and Hard Materials, 2006, 24 (4) :338.

[6] Anon P J.Tungsten carbide wire drawing dies Part II[J].Wire Industry, 1992, 59 (701) :391.

[7] Doeland O M, Maaren A C.Optimization of tungsten-wiredrawing process based on certain theories of wire drawing[J].Wire, 1976, 26 (5) :187.

[8] Mordyuk B N, Mordyuk V S.Ultrasonic drawing of tungstenwire for incandescent lamps production[J].Ultrasonics, 2004, 42 (1-9) :109.

[9] Browning P F, Briant C L, Rajan K.An analysis of splittingfailures during the drawing of tungsten wire[J].EngineeringFailure Analysis, 1995, 2 (2) :105.

[10] Briant C L, Horacsek O, Horacsek K.The effect of wire historyon the coarsened substructure and secondary recrystallization ofdoped tungsten[J].Metallurgical Transactions A-Physical Metal-lurgy and Materials Science, 1993, 24 (4) :843.

[11] Lee M, Flom D.Lubrication mechanisms in tungsten and mo-lybdenum wire drawing[J].Lubrication Engineering, 1991, 47 (2) :135.

[12] Harmat P, Bartha L, Grosz T.The rate of bubble deformationduring tungsten wire drawing[J].International Journal of Re-fractory Metals&Hard Materials, 2002, 20 (4) :295.

[13] Grosz T, Borbely S, Harmat P.Small-angle neutron scatteringstudy of potassium-doped tungsten wire samples[J].MaterialsScience Forum, 2001, 378 (3) :446.

[14] Sekiguchi H, Kobatake K.Development of dieless drawingprocess[J].Advanced Technology of Plasticity, Proc.2nd ICTP[C].1987.347.

[15] Symmons G R.Performance comparison of ploymer fluids indieless wire drawing[J].Journal of Materials Process Technolo-gy.1994, 43 (5) :13.

[16] Yamada Y.Applications of dieless drawing to Ni-Ti wire and ta-pered steel wire manufacture[J].Kobe Res.Dev. (in Jap.) , 1992, 42 (2) :93.

[17] Wengenroth W, Pawelski O.Theoretical and experimental in-vestigations into dieless drawing[J].Steel Research, 2001, 72 (10) :402.

[18] Wang Z T, Luan G F, Bai G R.Study of the deformation veloc-ity field and drawing force during the dieless drawing of tube[J].Journal of Materials Processing Technology, 1999, 94 (2) :73.

[19] Carolan R, Tiernan P, Commerford P.The dieless drawing ofhigh carbon steel[J].Materials Science Forum, 2004, 447-448:513.

[20] Fortunier R, Sassoulas H, Montheillet F.Thermo-mechanicalanalysis of stability in dieless wire drawing[J].InternationalJournal of Mechanical Sciences, 1997, 39 (5) :615.

[21] Weidig Ursula, Kaspar Radko, Pawelski.Multiphase micro-structure in steel bars produced by dieless drawing[J].Steel Re-search, 1999, 70 (4-5) :172.

[22] Wang Z T, Zhang S H, Luan G F.Experiment study on thevariation of wall thickness during dieless drawing of stainless steeltube[J].Journal of Materials Processing Technology, 2002, 120 (1-3) :90.

[23] Wright N, Wright E.Basic analysis of dieless drawing[J].Wire Journal International, 2000, 33 (5) :138.

[24] Gliga M, Canta T.Theory and application of dieless drawing[J].Wire Industry, 1999, 66 (785) :294.

[25] Tiernan P, Hillery M.An investigation of the dieless drawingmethod for the production of mild steel wire[J].Wire JournalInternational, 1999, 32 (12) :94.