Article ID: 1003-6326(2005)02-0395-05
Noise reduction of punch press mechanical clutch engagement
CHEN Wei(陈 威)1, 2, 3, SUN Chang-qing(孙长青)1, LI Yun-liang(李运良)1
(1. Department of Mechanical Engineering, Academy of Armored Forces Engineering, Beijing 100072, China;
2. Research and Development Center, Jiangsu Fasten Block Corporation,Jiangyin 214433, China;
3. Department of Materials Physics and Chemistry, Harbin Institute of Technology, Harbin 150001, China)
Abstract:
Among the noises of the punch press, blanking noise and engaging noise of the clutch should be paid most attention to. The latter is generated by the engagement impacts of the rotating key on the spline bush. In order to absorb the pressing energy and reduce the noise radiated, polyurethane cushions were added to the spline bush keyways and the clutch running noise reduction has reached 10.7dB(A). Considering such factors as the running characteristics of the punch press clutch, the demand for cushioning performance and the demand of the clutch temperature field for damping materials, the temperature field of the rigid clutch spline bush was simulated to find out whether the temperature of polyurethane go beyond its critical application temperature, using the finite element method. According to the characteristic that the deforming memory alloy can restore the remembered shape with the temperature rising, the actual temperature of the spline bush was measured. Consequently, the theoretical temperature turned out to be close to the measured temperature.
Key words:
punch press; clutch; noise reduction; polyurethane CLC number: TB53;
Document code: A
1 INTRODUCTION
Among the noises of the punch press, blanking noise and engaging noise of the clutch need to be paid most attention to[1]. The peak value of engaging noise of the clutch measured at an operators position is always more than 90dB(A)[ 2-5]. The engaging noise decides the duration of the operator exposed to the noise. The rigid clutch engagement noises are generated by a series of impacts, namely, the impact between the rotating key and the spline bush, that between the rotating key and the crank shaft and that between the crank shaft and the bearing liner[6]. The rigid rotating key clutch is widely applied on 20-1000kN punch press. In 1985, Koos et al[7]studied the rigid clutch noise of punch, who did experiments respectively under 170, 230 and 300kN punch press, using sandwich flexible rotating key made of steel-hard nylon which was wear-resisting, tear-resisting, oil resistant and adaptable instead of primary steel one. As a result, the punch used the new type of rotating key could work normally and 6-9dB(A) noise reduction was achieved on the operators position. During the punching process, the nylon cushion endures not only the impact load of the rotating key, but also the thermal load generated between the rotating key and the spline bush, so the demand to nylon material is relatively high. But Koos didnt strictly analyze whether nylon material could satisfy consecutive working of punch rotating key. Therefore, to further study the reason for the production of the punch clutch engaging noise and corresponding controlling measure is of great significance for the transformation of existing punch and the design of new punch.
2 ANALYSIS OF PUNCH PRESS CLUTCH ENGAGEMENT NOISE AND APPROACHES TO RELATED NOISE REDUCTION
There are in essence 3 or 4 keyways on the spline bush and the rotating key engages with one of them each time. The clutch engagement noises are generated by a series of impacts[8-10]. And in the engagement process, three kinds of notable impacts occur, namely, the impact between the rotating key and the keyway while the rotating key entering the keyway of the spline bush, the impact between the rotating key and the spline bush and the crank shaft under the inertial force of the flywheel joined with the spline bush (as shown in Fig.1), and the impact between the crank shaft and the bearing liner.
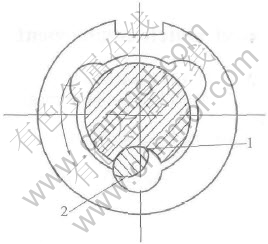
Fig.1 Zones of two major impacts in engagement process
Among the three important impacts of the rotating key clutch, the impact between the crank shaft and the bearing liner is the secondary, the impact between the rotating key and the crank shaft the close-clearance, and the impact between the rotating key and the spline bush contributes to the noise mostly. Therefore, some damping materials, such as polyurethane, can be added to the spline bush to absorb vibration energy and reduce the energy that would otherwise be converted to sound energy.
The structure of the spline bush has been developed, as shown in Fig.2. A 3mm thick polyurethane elastic cushion was inlaid into the keyway of the spline bush impacted by the rotating key. And to prevent the polyurethane elastic cushion from crosswise shifting under the impact of the rotating key, two assembling pins (locating plungers) were added, which were interferingly fitted with the pin holes. The polyurethane cushions and the spline bush were felted up by polyurethane adhesive.
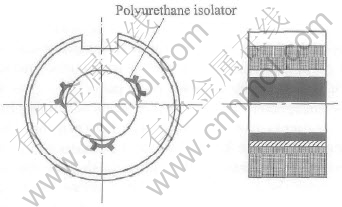
Fig.2 Structure of soft spline bush
During the racing of the press punch, the friction between the spline bush and the crank shaft generates heat, and the polyurethane elastic cushion is influenced by the impact of rotating key. So more attention has to be paid to the techniques of the adhesion of the polyurethane elastic cushion to the spline bush, and accordingly, the operation has to be done according to the process specifications constituted in Ref.[2].
3 ANALYSIS ON TEMPERATURE FIELD OF FRICTION HEAT GENERATED BY CRANKSHAFT AND SPLINE BUSH
As mentioned above, noise reduction can be achieved by the addition of polyurethane cushion, but considering that the performance of polyurethane deteriorates with the temperature rising and that the normal application temperature of polyurethane of various type is different, it is necessary to analyze the temperature field of the spline bush, in order to foretell its life-span.
1) Friction power
According to the frictional theory and the force analysis of the crank shaft[11], the friction power generated by the relative slide of the spline bush inner face on the crank shaft has been calculated as follows: P=67W[12].
2) Thermal current
Since the spline bush revolves at a very high speed, it would be reasonable to assume that the heat generated from the spline bush distribute axisymmetrically (especially after it has been working for a long time) and that the spline bush absorb half of the frictional heat as well as the shaft. The thermal current that flows into the inner face of the spline bush (q) can be obtained from Eqn.(1):
![]()
where S is a half of the surface area of the spline bush inner hole under the friction.
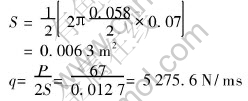
So the boundary conditions are as follows:
1) Along the interface between the boundary of the spline bush and the crankshaft, the thermal current flowing into the spline bush can be determined.
2) Along the rest interface, the heat transfer is by convection.
Because of the low temperature, convection heat transfer has been taken into consideration instead of radiation heat transfer. When the spline bush works steadily after successive running, the temperature of the spline bush reaches the peak. Therefore we can analyze the temperature field here only. The structures of the spline bush, the outer bush and the flywheel boss are all axisymmetrical. In the cylindrical coordinates system, the temperature has no relation to the annular coordinates and is but a function of coordinates r and z. Because of the symmetrical structure, we can merely analyze a half of any longitudinal section at random, namely, the temperature distillation function T(r, z) of the upper part from the section a—b can be analyzed as is shown in Fig.3.
Since there is no heat source in the solution domain established by the spline bush and the wheel boss (referred to as conduction heat tube), the temperature distillation function T(r, z) here must meet the differential equation:
![]()
Since the friction heat generated between the spline bush inner face and the crank shaft is the same as the free heat radiating problems of the flywheel boss inner face, the third boundary condition of the finite element temperature field analysis can be applied[13]. Namely,
![]()
This is equivalent to the variation problem:
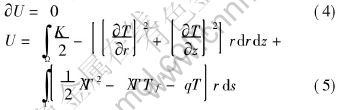
where Ω—a quarter of the longitudinal section of the conduction heat tube (combined by the spline bush and the wheel boss); Г—the given temperature boundary; Tf—the ambient temperature; q—the inflowing thermal current; λ—the heat dispersion factor.
The solution domain can be divided into triangular elements, and the temperature of each element can be computed from the temperatures of the three nodes {T}e=[Tl, Tm, Tn]T, using the interpolation method, namely
T(r, z)=[N]T{T}(6)
The temperature matrix equation is
[H]{T}={p}(7)
where
{p}=∑{p}e,
[H]=∑([H1]e+[H2]e).
Then the overall heat balance equation can be derived[14], from which the spline bush temperature distribution may be calculated. Fig.4 shows the FEM model of conduction heat tube, and Fig.5 shows the calculation results.
4 TEMPERATURE MEASUREMENT OF SPLINE BUSH
Reformed memory alloy has the property that when temperature reaches the given value, it begins to change its phase until it is restored to the heat setting shape (the lower the transformation temperature, the stronger the irreversibility of restoration). So we bent the memory alloy wire to a certain angle before the measurement and wrote it down, then put it into the temperature field, making it deform freely with the temperature rising, lastly we brought it out of the field and measured its final angle. Then we bent another same memory alloy wire to the original angle, and put it to an adjustable temperature field, such as the adjustable warm water, making it deforming until its angle was the same as the measured final angle in the conduction heat tube temperature field. The corresponding temperature here is the measured temperature of the conduction heat tube.
Actually, we used a NiTi memory alloy wire with 0.5mm in diameter and the measured temperature of the conduction heat tube was 60℃. There was a discrepancy of less than 2℃ between the measured temperature and the theoretic temperature derived from the above, which is allowable in engineering.
The polyurethane elastic cushion adopted in the experiments is made in Nanjing Rubber Plant, whose normal application temperature is 80℃[15]. The above experimental results show that it is feasible to add polyurethane elastic cushion to the spline bush keyways of the 100kN punch press ro tating key clutch to reduce noises, because the working temperature is within the normal application temperature. At present, the normal application temperature of the polyurethane elastic cushion made in Japan has reached 130℃[16]or more, so it is possible to reduce the rigid clutch noise of punch press of bigger tonnage by using this method.
Fig.3 Illustration of thermal current
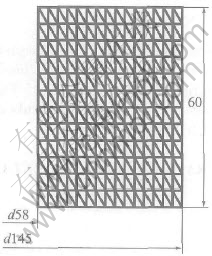
Fig.4 FEM model of conduction heat tube
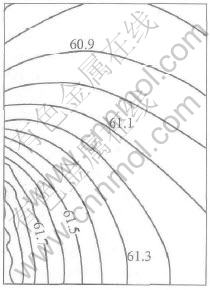
Fig.5 Temperature distribution of conduction heat tube
5 ANALYSIS ON EXPERIMENTAL RESULTS
In fact, there are many noise sources in the clutch system, but we have only compared the situations before and after the clutch spline bush was reformed, without reference to the impact between the end of the rotating key and the closer and so on. The measurement results of the ND-2 type sound grader adopted in the experiments are shown in Fig.6.
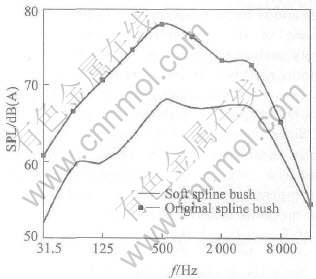
Fig.6 Noise frequency spectrum of clutch spline bush
Comparing the two curves in Fig.6, we can find that after polyurethane elastic cushion is added to the spline bush, the engagement noise of the rotating key reduce, sharply owing to its damping function, and noise reduction varies from 2dB(A) to 10.7dB(A) (in low frequency band, noise reduction is especially remarkable (about 10dB(A)), while in high frequency band it is from 2dB(A) to 3dB(A))
6 CONCLUSIONS
1) After polyurethane cushions were added to the spline bush keyways, the clutch running noise reduction has reached 10.7dB(A).
2) The working temperature of the spline bush keyways of the rigid clutch has been calculated by the finite element method and measured with memory alloy. And the theoretic temperature was close to the measured temperature, which was lower than the normal application temperature of the polyurethane elastic cushion[4] (80℃). So the damping material can meet the application demand.
REFERENCES
[1]Koss L L. Mean square pressure of a transmit oscillator and applications to punch press noise [J]. Journal of Sound and Vibration, 1985,102(4): 527-549.
[2]MA Chang-fu. 460 Questions in Operational Adhesive Technique [M]. Beijing: Jindun Press, 1992. 44-59.(in Chinese)
[3]CHEN Wei. Experimental Studies on Punch press Noise Reduction and Finite Element Analysis [D]. Harbin: Harbin Industrial University , 1997. 35-41.
[4]Shanxi Chemical Research Institute. Polyurethane Elastic Body [M]. Beijing: Chemical Industry Press, 1982. 419-451.(in Chinese)
[5]Koss L L, Moffatt J A. Vibration and acoustic data for punch press [J]. Journal of Sound & Vibration, 1982, 80(4): 543-554.
[6]Chee K K. Parameters affecting punch press noise radiation [J]. Sheet Metal Industries, 1982, 4: 364-371.
[7]Koss L L, Alfredson R J. Identification of transient sound sources on a punch press [J].Journal of Sound and Vibration, 1985, 34(1): 11-13.
[8]Dunn S E, Stevens K K, Uhlar D A. consideration in applying damping coating material properties determined from beam tests to the control of plate vibration problems [J]. Inter-Noise, 19, 83: 541-544.
[9]CHEN Wei-min, CAO Xi-bin. Analysis and control of noise produced by the presses during stamping [J]. Journal of Material Processing Technology, 1994, 42: 87-94.
[10]CHEN Wei-min, CAO Xi-bin. Study on the moving accuracy of the slide in the press [J]. Journal of Harbin Institute of Technology(English edition), 1994, E-1(2): 67-70.
[11]Koss L L, Moffatt J A. Vibration and acoustic data for a punch press [J]. Journal of Sound and Vibration, 1982, 80(4): 543-554.
[12]Koss L L. Mean square pressure of a transient oscillator and applications to punch press noise [J]. Journal of Sound and Vibration, 1985,102(4): 527-549.
[13]Luzzato E, Lecointer C. Some simple and effective methods for source identification with geometrical acoustic models [J]. Journal of Sound and Vibration, 1986, 105(3): 473-490.
[14]Richards E J, Carr I. On the prediction of impact noise, Part Ⅹ [J]. The Design and Testing of a Quietened Drop Hammer, 1986, 104(1):137-164.
[15]Cuschieri J M. On the prediction of Impact noise(part Ⅷ)—diesel engine noise [J]. Journal of Sound and Vibration, 1985, 102(1): 21-56
[16]LIU Gang, CHEN Wei-min, CHEN Wei. Construction and solution to a new dynamic model of forging hammers damping system [J]. Journal of Harbin Institute of Technology, 1996, E-3(2): 92-96.(in Chinese)
Received date: 2004-12-16; Accepted date: 2005-01-18
Correspondence: CHEN Wei, Professor, PhD; Tel: +86-10-66718148; E-mail: zgychenwei sina.com


