
Subsequent yield loci of 5754O aluminum alloy sheet
WANG Hai-bo(王海波), WAN Min(万 敏), WU Xiang-dong(吴向东), YAN Yu(阎 昱)
School of Mechanical Engineering and Automation, Beihang University, Beijing 100191, China
Received 17 June 2008; accepted 13 March 2009
Abstract: Complex loading paths were realized with cruciform specimens and biaxial loading testing machine. Experimental method for determining the subsequent yield locus of sheet metal was established. With this method, the subsequent yield loci of 5754O aluminum alloy sheet were obtained under complex loading paths. Theoretical subsequent yield loci based on Yld2000-2d yield criterion and three kinds of hardening modes were calculated and compared with the experimental results. The results show that the theoretical subsequent yield loci based on mixed hardening mode describe the experimental subsequent yield loci well, whereas isotropic hardening mode, which is widely used in sheet metal forming fields, predicts values larger than the experimental results. Kinematic hardening mode predicts values smaller than the experimental results and its errors are the largest.
Key words: aluminum alloy sheet; complex loading path; subsequent yield locus; hardening mode
1 Introduction
In sheet metal forming processes, accurate prediction of both the geometry and the mechanical properties of the final product depends on reasonable constitutive models. For this reason, the development of plasticity theory has been pursued for many years. Rigorous experimental investigation, however, is also crucial for ensuring that the constitutive model adequately describes the mechanical behavior of the sheet metal under a variety of complex loading conditions[1].
Biaxial tensile testing with different types of cruciform specimens has become the most popular method to realize various stress states during biaxial tension. To minimize calculation errors of stress, the shapes of cruciform specimens have been optimized using FEM and different optimization algorithms[1-2].
KUWABARA et al[3-4] studied the successively plastic work contours of cold-rolled steel sheet and aluminum alloy sheet under biaxial tension. They found that Gotoh yield criterion describes the yield behavior of steel sheet with good accuracy whereas the Taylor model fits the 6xxx aluminum alloy sheet very well. GREEN et al[5] used a type of cruciform specimen with a thinned center and slots in the arms to study the biaxial tensile behavior of 1145 aluminum alloy sheet. In addition, a finite element analysis was carried out using different phenomenological models of anisotropic plasticity.
In the above researches, the loading paths of these experiments are linear during the deforming processes and the plastic work contours present isotropic hardening trend. However, loading paths will change during most sheet metal forming processes, and some areas of the material may experience multiaxial and multi-path loading conditions. Generally speaking, a change in loading path has a significant influence on the plastic deformation of materials. Under complex loading deformation conditions, the material can exhibit qualitatively a more different hardening mode than in monotonic deformation[6-8].
Some experimental methods such as tension- compression tests, tension-shear tests and multistage tension tests have been used to investigate the hardening modes of sheet metals under complex loading conditions. However, only some specific subsequent yield points can be determined and the subsequent yield loci cannot be obtained[9]. So only the hardening modes of these specific points are investigated. Biaxial tension testing with a cruciform specimen has been used to determine the plastic work contours of sheet metals, which are obtained under linear loading paths and are commonly considered the subsequent yield loci for simplicity.However, it should be noted that the plastic work contours do not coincide with the subsequent yield loci [10].
Therefore, it is necessary to investigate the subsequent yield loci of sheet metal and determine the hardening mode. In this work, the subsequent yield loci of 5754O aluminum alloy sheet are obtained with cruciform specimens and biaxial loading testing machine. The theoretic subsequent yield loci are calculated based on different hardening modes and compared with the experimental results.
2 Experimental
2.1 Testing system and cruciform specimen
The testing system includes a loading testing machine, a control unit and an application software. The loading test machine consists of six independently controlled axes (two axes in each direction of x, y and z) and each axis is actuated by one hydraulic cylinder. A complete description of the test system was given by WU et al[9, 11]. Only controlled axes in x and y direction were used here. In order to minimize the calculation error of the stress in the central area, the shape and dimension of the cruciform specimen were optimized by means of combining FEM with orthogonal design, and the optimized specimen is illustrated in Fig.1. The cruciform specimens were machined using laser and the width of slots in the arms was 0.2 mm.
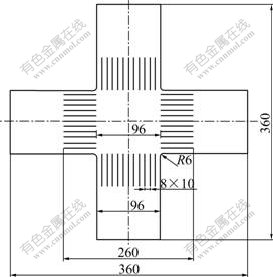
Fig.1 Schematic diagram of cruciform specimen (unit: mm)
2.2 Procedure of biaxial tensile test
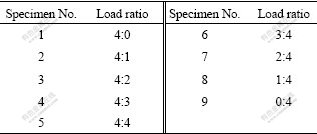
Linear loading paths with different load ratios were adopted for a group of specimens, and the experimental program is shown in Table 1. Here, “load ratio” is the ratio of the load along rolling direction to the load along transverse direction.
Table 1 Linear loading path
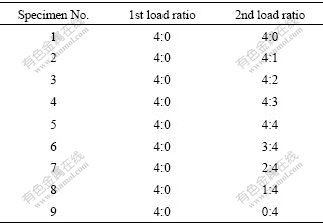
Complex loading path consists of two linear loading paths with different load ratios, which is shown in Table 2. In the first loading path, a certain load ratio is adopted for a group of specimens. After a certain pre-strain and unloading, in the second loading path, the second load ratios are then adopted for the same specimens.
Table 2 Complex loading path
Normal stress components σx and σy were determined by dividing the measured tensile loads Fx and Fy by the current cross-sectional area of gage section.
2.3 Determination of experimental plastic work contours and initial yield locus
Plastic work contours are used to investigate the suitability of a yield criterion and are commonly considered subsequent yield loci for simplicity[10]. According to the principle of constant amount of total plastic work per unit volume, the relationship between stress—plastic strain along two directions in biaxial tensile test and equivalent stress—equivalent plastic strain can be written as
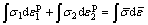 (1)
(1)
where σ1, 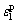 and σ2,
and σ2, 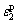 are the stress and plastic strain along rolling direction and transverse direction, respectively;
are the stress and plastic strain along rolling direction and transverse direction, respectively; 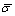 and
and 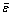 are the equivalent stress and equivalent plastic strain.
are the equivalent stress and equivalent plastic strain.
First, uniaxial tensile tests in rolling direction of the specimen were carried out and the stresses 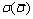 corresponding to some values of plastic strain
corresponding to some values of plastic strain 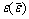 were determined. The corresponding plastic work W per unit volume was measured for each
were determined. The corresponding plastic work W per unit volume was measured for each 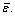 In the biaxial tensile tests, true stress components were determined at the same plastic W. Then plastic work contours were constructed in plane stress space.
In the biaxial tensile tests, true stress components were determined at the same plastic W. Then plastic work contours were constructed in plane stress space.
The experimental plastic work contour at 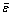 =0.2% was adopted as the experimental initial yield locus.
=0.2% was adopted as the experimental initial yield locus.
2.4 Determination of experimental subsequent yield loci
The complex loading path presented in Table 2 was used for investigating the subsequent yield locus. After the first loading and unloading, the specimens had some pre-strain and are called “new” specimens here. The specimens with no pre-strain (no deformation) were called “original” specimens. Therefore, the initial yield locus of the “new” specimens was the subsequent yield locus of the “original” specimens.
The initial yield locus of the “new” specimens was determined in the second loading path with the same method as that for determining the experimental initial yield locus. Then the subsequent yield locus of the “original” specimens was constructed in the plane stress space.
3 Theoretical subsequent yield loci
A more accurate constitutive model consisting of yield criterion and hardening mode is needed to analyze forming processes of sheet metal better. The common hardening modes consist of isotropic, kinematic and mixed hardening modes.
Isotropic hardening mode can be expressed by yield criterion and is most widely used. Many kinds of yield criterions were proposed by scholars all over the world. In recent years, BARLAT et al[12-16] proposed several yield criterions in order to describe the anisotropy of sheet metals more accurately. Yld2000-2d yield criterion was proposed by BARLAT et al[16] in 2003. The orthotropic yield function is reasonably suitable to describe the anisotropy of rolled sheets, especially that of aluminum alloy sheets[16-19]. Yld2000-2d is given by
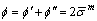 (2)
(2)
where exponent m is a material coefficient and
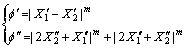 (3)
(3)
and 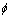 is the sum of two isotropic functions, which are symmetric with respect to
is the sum of two isotropic functions, which are symmetric with respect to 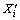 and
and 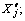
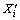 and
and 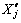 are the principal values of the matrices,
are the principal values of the matrices, 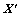 and
and 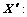
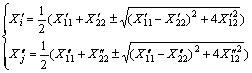 (4)
(4)
Components of 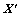 and
and 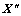 are obtained from the following linear transformation of the Cauchy stress:
are obtained from the following linear transformation of the Cauchy stress:
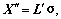
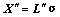 (5)
(5)
where
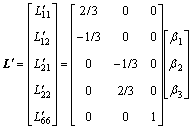 (6)
(6)
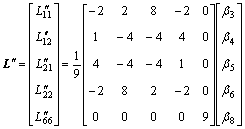 (7)
(7)
In Eqs.(5), (6) and (7), σ is Cauchy stress and β1-β8 are eight anisotropic coefficients. The procedure of solving β1-β8 numerically was developed according to Ref.[16].
L-C kinematic hardening model proposed by LEMAITRE and CHABOCHE[20] is mostly used for kinematic hardening mode, which can be expressed as
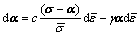 (8)
(8)
where c and γ are material constants; 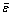 is equivalent plastic strain; σ and α are stress and back stress tensors.
is equivalent plastic strain; σ and α are stress and back stress tensors.
Mixed hardening mode is a combination of isotropic and kinematic hardening mode, for which the constitutive equation can be written as
 (9)
(9)
where 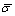 is equivalent stress. Eq.(9) means isotropic hardening when α=0 and kinematic hardening when
is equivalent stress. Eq.(9) means isotropic hardening when α=0 and kinematic hardening when 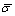 is a constant.
is a constant.
Yld2000-2d yield criterion and L-C kinematic hardening model are used in this work.
The theoretical subsequent yield loci are obtained with transformation (including expansion and moving) of the theoretical initial yield locus. The expansion and moving are solved with numerical method and the procedure for solving the transformation of different kinds of hardening modes is developed.
4 Results and discussion
4.1 Plastic work contours
Based on Eq.(1) and the experimental stress—plastic strain curves, the experimental plastic work contours for different equivalent plastic strains are determined. Also, the theoretical plastic work contours calculated based on Yld2000-2d for different equivalent plastic strains are compared with the experimental plastic work contours. As shown in Fig.2, Yld2000-2d yield criterion can well describe the trends of the plastic work contours, which proves the suitability of Yld2000-2d. In Fig.2, “Exp. 0.2%” denotes experimental initial yield locus mentioned above.
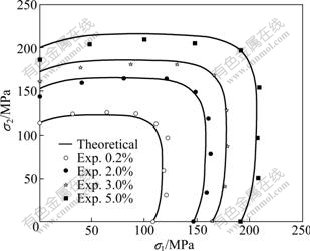
Fig.2 Comparison of Yld2000-2d yield criterion and experimental plastic work contours
4.2 Subsequent yield loci
The experimental subsequent yield loci are determined after pre-strains of 3.6% and 5.8%. In addition, the experimental subsequent yield loci are compared with the theoretical subsequent yield loci based on different kinds of hardening modes at the same pre-strains, as shown in Fig.3. I, K and M denote the theoretical subsequent yield loci based on isotropic, kinematic and mixed hardening modes, respectively, and the curve drawn with thick lines represents the theoretical initial yield locus. “Exp.0.2%” denotes the experimental initial yield locus.
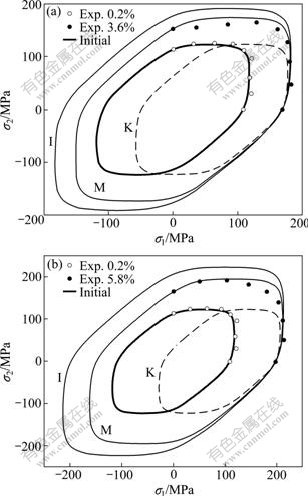
Fig.3 Comparison of theoretical and experimental subsequent yield loci (I—Isotropic hardening mode; K—Kinematic hardening mode; M—Mixed hardening mode)
As seen from Fig.3(b), over all, the theoretical yield loci based on mixed hardening mode describe the experimental yield loci well, whereas isotropic hardening mode predicts values larger than the experimental results. Kinematic hardening mode predicts values smaller than the experimental results and its errors are the largest.
As is well known, the isotropic hardening mode can describe the homogeneous expansion instead of the moving of the yield loci. And from Figs.3(a) and (b), it can be obviously found that the experimental subsequent yield loci present a trend of shift to right so that the isotropic hardening mode cannot describe the subsequent yield loci accurately. The kinematic hardening mode can describe the moving instead of the expansion of the yield loci. However, the expansion of the subsequent yield loci is clearly shown in Fig.3(a), which indicates that the kinematic hardening mode cannot describe the subsequent yield loci accurately either. The mixed hardening mode is a combination of the isotropic and the kinematic hardening modes so that it can describe the expansion and moving of the yield loci simultaneously. Therefore, the mixed hardening model can describe the true subsequent yield loci.
As indicated in Fig.3, when the 2nd load ratios are 4?0, 4?1, 4?2 and 4?3, the isotropic and the kinematic hardening modes can reasonably describe the subsequent yield behavior. From Fig.3(b), it is found that, when the 2nd load ratio is 4?4, the mixed hardening mode still has large error though it describes the subsequent yield behavior better than the other hardening modes.
It can be easily proved that the plastic work contours are coincident with the subsequent yield loci based on isotropic hardening mode for the same equivalent plastic strain. The latter does not coincide with the experimental subsequent yield loci. Therefore, the plastic work contours do not coincide with the experimental subsequent yield loci as mentioned by KUWABARA[10].
5 Conclusions
1) Complex loading paths are realized using cruciform specimens and biaxial loading testing machine. The subsequent yield loci of 5754O aluminum alloy sheet are obtained. The experimental plastic work contours are also obtained.
2) Theoretical plastic work contours are calculated based on Yld2000-2d yield criterion and they describe the experimental plastic work contours well.
3) The theoretical subsequent yield loci based on Yld2000-2d yield criterion and three kinds of hardening modes are calculated, and compared with the experimental results. Because of the moving and expansion of the experimental subsequent yield loci, the yield loci based on the mixed hardening mode describe the experimental results well; whereas those based on the other hardening modes do not. When the 2nd load ratio is 4?4, the mixed hardening mode still has large error.
4) The view that the subsequent yield loci do not coincide with the plastic work contours is verified.
References
[1] KUWABARA T, IKEDA S, KURODA K. Measurement and analysis of differential work hardening in cold-rolled steel sheet under biaxial tension [J]. J Mater Process Technol, 1998, 80/81(8): 517-523.
[2] YU Yong, WAN Min, WU Xiang-dong, ZHOU Xian-bin. Design of cruciform biaxial tensile specimen for a limit strain analysis by FEM [J]. J Mater Process Technol, 2002, 123(1): 67-70.
[3] KUWABARA T, IKEDA S, KURODA K. Measurement and analysis of yield locus of sheet aluminum alloy 6xxx [C]// Numisheet’99. Besancon, 1999: 85-90.
[4] IKEDA S, KUWABARA T. Measurement and analysis of work hardening of sheet metals under plane-strain tension [C]// Numisheet’2002. Jeju Island, 2002: 97-102.
[5] GREEN D E, NEALE K W, MACEWEN S R, MAKINDE A, PERRIN R. Experimental investigation of biaxial behavior of an aluminum sheet [J]. Int J Plasticity, 2004, 20: 1677-1706.
[6] BARLAT F, FERREIRA D J M, GRACIO J J, LOPES A B, RAUCH E F. Plastic flow for non-monotonic loading conditions of an aluminum alloy sheet sample [J]. Int J Plasticity, 2003, 19: 1215-1244.
[7] GARDEY B, BOUVIER S, RICHARD V, BACROIX B. Texture and dislocation structures observations in a dual-phase steel under strain-path changes at large deformation [J]. Mater Sci Eng A, 2005, 400/401: 136-141.
[8] WU P D, MACEWEN S R, LLOYD D J, JAIN M, TUGCU P, NEALE K W. On pre-straining and the evolution of material anisotropy in sheet metals [J]. Int J Plasticity, 2005, 21: 723-739.
[9] WU Xiang-dong, WAN Min, HAN Fei, WANG Hai-bo. Stress-strain curves of different loading paths and yield loci of aluminum alloy sheets [J]. Trans Nonferrous Met Soc China, 2006, 16: s1460-s1464.
[10] KUWABARA T. Advances in experiments on metal sheets and tubes in support of constitutive modelling and forming simulations [J]. Int J Plasticity, 2007, 23: 385-419.
[11] WU Xiang-dong, WAN Min, ZHOU Xian-bin. Biaxial tensile testing of cruciform specimen under complex loading [J]. J Mater Process Technol, 2005, 168(1): 181-183.
[12] BARLAT F, LIAN J. Plastic behaviour and stretchability of sheet metals (Part I): A yield function for orthotropic sheets under plane stress condition [J]. Int J Plasticity, 1989, 5: 51-66.
[13] BARLAT F, LEGE D J, BREM J C. A six-component yield function for anisotropic materials [J]. Int J Plasticity, 1991, 7: 693-712.
[14] BARLAT F, BECKER R C, HAYASHIDA Y, MAEDA Y, YANAGAWA M, CHUNG K, BREM J C, LEGE D J, MATSUI K, MURTHA S J, HATTORI S. Yielding description of solution strengthened aluminium alloys [J]. Int J Plasticity, 1997, 13: 385- 401.
[15] BARLAT F, MAEDA Y, CHUNG K, YANAGAWA M, BREM J C, HAYASHIDA Y, LEGE D J, MATDUI K, MURTHA S J, HATTORI S, BECKER R C, MAKOSEY S. Yield function development for aluminium alloy sheets [J]. J Mech Phys Solids, 1997, 45(11/12): 1727-1763.
[16] BARLAT F, BREM J C, YOON J W, CHUNG K, DICK R E, LEGE D J, POURBOGHRAT F, CHOI S H, CHU E. Plane stress yield function for aluminum alloy sheets (Part I): Theory [J]. Int J Plasticity, 2003, 19: 1297-1319.
[17] YOON J W, BARLAT F, DICK R E, CHUNG K, KANG T J. Plane stress yield function for aluminum alloy sheets (Part II): FE formulation and its implementation [J]. Int J Plasticity, 2004, 20: 495-522.
[18] LEE G M, KIM D, KIM C, WENNER M L, WAGONER R H, CHUNG K. Spring-back evaluation of automotive sheets based on isotropic-kinematic hardening laws and non-quadratic anisotropic yield functions (Part II): Characterization of material properties [J]. Int J Plasticity, 2005, 21: 883-914.
[19] LEE G M, KIM D, KIM C, WENNER M L, CHUNG K. Spring-back evaluation of automotive sheets based on isotropic-kinematic hardening laws and non-quadratic anisotropic yield functions (Part III): Applications [J]. Int J Plasticity, 2005, 21: 915-953.
[20] LEMAITRE J, CHABOCHE J L. Mechanics of solid materials [M]. Cambridge: Cambridge University Press, 1990: 161-241.
Foundation item: Project(50475004) supported by the National Natural Science Foundation of China
Corresponding author: WANG Hai-bo; Tel: +86-10-82338613; E-mail: rock_haibo@me.buaa.edu.cn
DOI: 10.1016/S1003-6326(08)60409-4
(Edited by YANG Bing)