Potential mechanisms of pore-fluid movement from continental lithospheric mantle into upper continental crust
来源期刊:中南大学学报(英文版)2008年第1期
论文作者:赵崇斌 彭省临 刘亮明 B. E. HOBBS A. ORD
文章页码:81 - 81
Key words:underground pore-fluid; porosity wave; continental crust; heat and mass transport
Abstract: Through integrating the state of the art scientific knowledge in different research fields, some potential mechanisms of large-scale movements of underground pore-fluids such as H2O and CO2 in the continental lithosphere were presented and discussed. The results show that the generation and propagation of porosity waves are important mechanisms to transport mass and heat fluxes from the continental lithospheric mantle into the lower continental crust; the generation and propagation of porosity waves, pore-fluid flow focusing through lower and middle crustal faults, advection of pore-fluids through the lower and middle crust, and whole-crust convection in some particular cases are important mechanisms to transport mass and heat fluxes from the lower into the upper continental crust; heat and mass transport through convective pore-fluid flow is the most effective mechanism of ore body formation and mineralization in hydrothermal systems; due to heat and mass exchange at the interface between the earth surface, hydrosphere and atmosphere, it is very important to consider the hydro-geological effect of the deep earth pore-fluids such as H2O and CO2 on the global warming and climate change in future investigations.
基金信息:the National Natural Science Foundation of China
J. Cent. South Univ. Technol. (2008) 15: 81-88
DOI: 10.1007/s11771-008-0017-8
![]()
ZHAO Chong-bin(赵崇斌)1, 2, PENG Sheng-lin(彭省临)1, LIU Liang-ming(刘亮明)1, B. E. HOBBS2, A. ORD2
(1. Computational Geosciences Research Centre, Central South University, Changsha 410083, China;
2. CSIRO Division of Exploration and Mining, P. O. Box 1130, Bentley, WA 6102, Australia)
Abstract:Through integrating the state of the art scientific knowledge in different research fields, some potential mechanisms of large-scale movements of underground pore-fluids such as H2O and CO2 in the continental lithosphere were presented and discussed. The results show that the generation and propagation of porosity waves are important mechanisms to transport mass and heat fluxes from the continental lithospheric mantle into the lower continental crust; the generation and propagation of porosity waves, pore-fluid flow focusing through lower and middle crustal faults, advection of pore-fluids through the lower and middle crust, and whole-crust convection in some particular cases are important mechanisms to transport mass and heat fluxes from the lower into the upper continental crust; heat and mass transport through convective pore-fluid flow is the most effective mechanism of ore body formation and mineralization in hydrothermal systems; due to heat and mass exchange at the interface between the earth surface, hydrosphere and atmosphere, it is very important to consider the hydro-geological effect of the deep earth pore-fluids such as H2O and CO2 on the global warming and climate change in future investigations.
Key words: underground pore-fluid; porosity wave; continental crust; heat and mass transport
1 Introduction
Large-scale movements of underground pore-fluids such as H2O and CO2 in the continental lithosphere are not only fundamental to understand the generation and formation of giant hydrothermal ore deposits within the upper continental crust, but also important to understand the release of heat and CO2 from the deep earth into the hydrosphere and atmosphere. A natural extreme example of such a release is the magma and volatile eruption during a volcano event, which can make considerable negative contributions to the greenhouse effect. Compared with a relatively quick volcano process that usually occurs between tectonic plates, the release of heat and CO2 from the continental intra-plate into the hydrosphere and atmosphere is a very slow process, so its hydro-geological effects on the global warming and climate change need to be considered on large-length and time scales. For this reason, understanding the large-scale movements of underground pore-fluids such as H2O and CO2 in the continental lithosphere has become an important multidisciplinary research topic in recent years.
Because the continental lithosphere can be viewed as a porous material, the pores of this material may be filled by different kinds of underground pore-fluids such as magmas in the continental lithospheric mantle, volatile gases and liquids in the continental crust. Generally, the origin of most precious metals may be considered to come from the inner core[1]. Such metals can be carried, by large-scale mantle convection[2-5], from the lower part into the upper part of the mantle (i.e. the lithospheric mantle). As a direct consequence, a material variation zone from the viscous magma of the mantle into the solid porous rock of the crust must exist, because the upper aethenosphere is in a partial melting state. In this regard, precious metals, H2O and CO2 must be transported, in some way through the partially molten magma, from the continental lithospheric mantle into the lower continental crust. As a result, the precious metals can be transported from the lower continental crust into the upper continental crust by volatile gases and/or compressed liquids, which are originated from H2O and CO2 being contained in the magma. If pressure, temperature and chemical conditions are favourable, the precious metals may be precipitated so that ore deposits can be generated in the upper continental crust. Although the general picture about the origin of most precious metals is clear, the required mechanism behind heat and mass transport, especially the mass transport of precious metals from the continental lithospheric mantle into the upper continental crust, seems to remain unclear until recently. There is no doubt that the understanding of such a mechanism is the key to the understanding of large-scale metal-bearing fluid movements in the deep continental lithosphere, which has bothered exploration geologists for many years.
Although a large amount of geochemical research has demonstrated that mantle materials can be transported from the continental lithospheric mantle into the upper continental crust in some ways[6-9], research on the detailed physical process related to this transport is still very limited. If the detailed mechanism of transporting heat and mantle materials from the continental lithospheric mantle into the upper continental crust is known, then it is possible to simulate the transport process of mantle materials as well as the ore forming process using contemporary computational algorithms and supercomputers. Therefore, the main purpose of this paper is to explore potential answers to the following fundamental question closely associated with the deep earth hydrogeology and its effects on hydrothermal ore body formation and mineralization: How does the underground pore-fluid move from the continental lithospheric mantle into the upper continental crust? Through reviewing and integrating the state of the art scientific knowledge and outcomes in different research fields and disciplines, it is possible to answer the above-mentioned fundamental question.
2 Potential mechanisms of heat and mass transport from continental lithospheric mantle into lower continental crust
A central issue related to the large-scale underground pore-fluid movement from the continental lithospheric mantle into the upper crust of the earth is to find the origin of pore-fluids in the deep earth. Extensive scientific research has demonstrated that H2O, CO2 and other fluids can be dissolved in the silicate melts by chemical reactions. Although the released volatile fluids during the magma solidification are comprised of H2O, CO2, H2S, HCl, HF, SO2 and other substances[10], H2O is the most abundant magmatic volatile and CO2 is the second most abundant magmatic volatile in the intruded magma. For this reason, the solubility of H2O in igneous- rock melts has been investigated for many years. Extensive studies[10] have demonstrated that if the constraints of the solution model for the NaAlSi3O8-H2O system are imposed, H2O solubilities in the igneous-rock melts are essentially identical to those in NaAlSi3O8 melts. Therefore, the solubility of H2O in the NaAlSi3O8 melt can be used to approximately determine the mass source of the released volatile fluids during the magma solidification. Fig.1 shows the projection of relative atomic positions in the albite (NaAlSi3O8) melts, where 262 g NaAlSi3O8 is capable of dissolving 18 g water. This implies that the movement of the deep earth pore-fluids is closely associated with that of the silicate melts. Thus, investigating the mechanism of magma movement from the continental lithospheric mantle into the lower crust of the earth is the key to understand the origin and movement of underground pore-fluids in the deep earth.
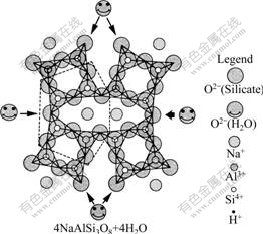
Fig.1 Projection of relative atomic positions in albite melt with possible dissolved water
2.1 Brief introduction of porosity wave transporting heat and mass model
Due to the special location within the earth, continental lithospheric mantle materials are usually subject to high temperature and pressure relative to the upper continental crust. Because the continental lithospheric mantle is a bridge to link the upper aethenosphere with the lower continental crust, there is a transition zone between the viscous fluid material (i.e. magma) and porous solid material (i.e. rock). This implies that the pores of the continental lithospheric mantle material can be filled with the magma coming from the mantle, although the porosity of the continental lithospheric mantle is very small (down to 0.03 or even less). Given the above constraints, understanding how magma transports from the continental lithospheric mantle into the lower continental crust and how the released volatile gases or liquids continue to transport in the lower continental crust is the key to the understanding of the mechanism of heat and mass transport from the continental lithospheric mantle into the lower continental crust. Since the deep mantle material can be treated as a viscous magma and the crust material can be treated as a porous medium, there is a mechanism question on the material exchange between the mantle and the continental crust. To answer this question, a new conceptual model for material exchange between the mantle and the continental crust was proposed[11]. The basic idea behind the proposed conceptual model is that the porosity wave, which was originally proposed for dealing with both magma ascending in the continental lithospheric mantle and upward underground pore-fluid flow in sedimentary basins[12-13], can also transport heat and mass from the mantel into the continental crust through two key processes: magma solidification and porous material consolidation. The proposed conceptual model of porosity wave transporting heat and mass[11] is used to demonstrate how heat and mantle materials are transported into the continental crust through the generation and propagation of porosity waves in the lithosphere.
2.2 Heat and mass transport processes through generation and propagation of porosity waves
As shown in Fig.2, the continental lithosphere is composed of porous materials, although the porosity of the porous material may be very small. In this figure, g is the acceleration due to gravity. From the structural geology point of view, the whole continental lithosphere can be divided into the continental crust and continental lithospheric mantle by the Moho surface. It is noted that although both the Moho surface and the interface between the lithospheric mantle and the asthenosphere are expressed by straight lines in Fig.2, the following discussions are also valid if these straight lines are replaced by curved lines. Since the upper aesthenosphere is in a partial melting state, it is possible to transport magma within the continental lithospheric mantle through the generation and propagation of the porosity wave[12]. BARNES[10] has also demonstrated that the solidification temperature of the ascending magma can be significantly reduced if water exists in the continental lithospheric mantle. For instance, the solidification temperature of the magma containing water may be reduced to about 1 100 K in the continental lithosphere. In this case, the ascending magma may be transported to the Moho surface through the generation and propagation of the porosity wave in the continental lithospheric mantle. As a result, the porous material of the continental lithospheric mantle (i.e. between the Moho surface and the top surface of the upper aesthenosphere) may be filled with the ascending magma, which originally comes from the aesthenosphere. On the other hand, the porous material of the continental crust (i.e. between the Moho surface and the surface of the earth) is filled with underground pore-fluids such as H2O and CO2, which may be either gas or liquid, depending on the local temperature and pressure conditions. The Moho surface is assumed to be a relatively impermeable thin layer due to the solidification of the ascending magma. This means that the Moho surface can be considered as a solidification surface of the ascending magma. If the initial thickness of the continental crust is expressed by HC0, the initial thickness of the continental lithosphere is HC0+HL-C0, where HL-C0 is the initial thickness of the continental lithospheric mantle. Owing to gravity, the porosity of the porous material decreases with depth. Since the strength of the porous material is temperature dependent, it also decreases with depth due to the existence of the downward positive temperature gradient in the continental lithosphere.
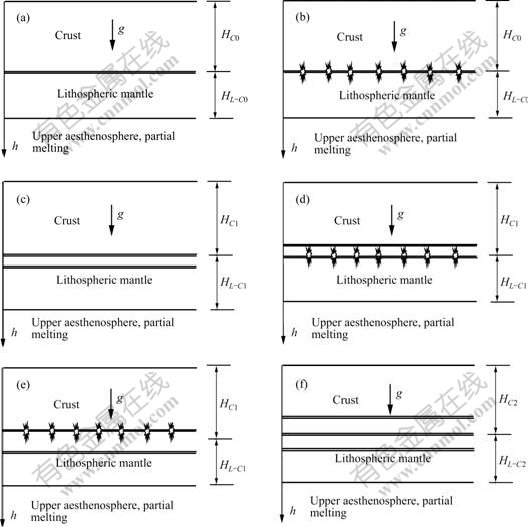
Fig.2 Sketch of transport mechanism of mantle materials through porosity waves (Vertical scale is exaggerated to allow mechanism to be explained): (a) t=t0, Moho of a thin impermeable layer leads to the pressure increase underneath the layer; (b) t=t1, thin impermeable layer collapses due to underneath pressure increase; (c) t=t2, formation of a new upward thin layer leads to the rise of Moho, while formation of a new downward thin layer results in downward movement of crustal material; (d) t=t3, new downward thin impermeable layer collapses due to underneath pressure increase; (e) t=t4, collapse of the new upward thin layer leads to magma ascending, while formation of the second new downward thin layer results in further downward movement of crustal material; (f) t=t5, formation of the second new upward thin layer leads to the further rise of Moho, while formation of the third new downward thin layer results in downward movement of more crustal material
At the initial stage (t=t0), any perturbation of either temperature or pressure at the bottom of the continental lithosphere due to mantle convection or mantle crust interaction can result in magma ascending from the upper aesthenosphere into the continental lithospheric mantle. The ascending magma moves towards the Moho surface through the generation and propagation of the porosity wave[12]. Because the Moho surface is a relatively impermeable thin layer, the ascending magma accumulates just under it until the increased magma pressure due to the magma accumulation exceeds the material strength of the layer. Generally, the increased magma pressure due to the magma accumulation may play two important roles in the generation of the porosity wave near the Moho surface. First, the increased magma pressure can cause an expansion of pores (or create pores if they do not exist) so that the porosity just underneath the Moho surface is increased. Second, the increased magma pressure can cause the collapse of the impermeable thin layer since the material strength of this layer is relatively low due to its location in a rather high temperature region. Once the increased magma pressure exceeds the material strength of the impermeable thin layer, the accumulated magma outbursts and penetrates the thin layer. This is the first stage of the porosity wave generation near the Moho surface (t=t1). The outbursted magma can travel upward some distance due to the local extra pressure gradient created by the magma accumulation at the initial stage until it becomes solidified due to heat loss to surrounding rocks. The solidification of the ascending outbursted magma can generate a new upward impermeable thin layer above the initial Moho surface. This consequence is equivalent to the upward movement of the initial Moho surface. Also, the released volatiles such as H2O and CO2 during magma solidification can travel upward in the continental crust in exactly the same form of the porosity wave as underground pore-fluid travels upward in sedimentary basins[13]. This indicates that heat and mantle volatile materials such as H2O and CO2 can also be transported into the upper continental crust by the porosity wave, which will be discussed in the next section. At the same time, the expanded pores of the underlying material of the initial impermeable layer may become consolidated and closed due to the release of the local pressure. As a result, a new downward impermeable layer is generated under the initial Moho surface. This consequence is equivalent to the downward movement of the initial crust material. The generation of the new upward and downward impermeable layer indicates the propagation of the generated porosity wave. This may be considered as the second stage of the generation and propagation of the porosity wave near the Moho surface (t=t2). As these processes repeat and continuously progress (see t=t3, t4 and t5 in Fig.2), the formation of the second new upward thin impermeable layer leads to the further rise of the Moho surface, while the formation of the third new downward thin impermeable layer results in the downward movement of more continental crustal materials. This indicates that as the porosity wave travels upwards, some mantle materials in the form of either magma or volatile pore-fluid move upwards in the continental lithosphere, while some continental crust materials move downwards in the continental lithosphere. This implies that it is possible to transport mass and heat from the continental lithospheric mantle to the upper continental crust through the generation and propagation of the porosity wave in the continental lithosphere.
2.3 Geological implication of generation and propag- ation of porosity waves
As the downwardly moved continental crust material replaces the initial continental lithospheric mantle material, the replaced continental lithospheric mantle material may cause the rest of the lithospheric mantle material to move downwardly so that some continental lithospheric mantle materials at the bottom of the continental lithosphere may be melted due to the increase of temperature and the whole thickness of the continental lithosphere is gradually reduced. This phenomenon can be also described as the thermal erosion that takes place just at the bottom of the lithospheric mantle. On the other hand, the upward movement of the Moho surface can lead to the thinning of the continental crust. Clearly, the adjustment of the thermal structure in the continental lithosphere due to the upward mass and heat fluxes carried by the porosity wave may be an important mechanism to reduce the thickness of the continental lithosphere.
It needs to be pointed out that if the temperature of magma solidification is higher than that of the Moho surface, then the first generated solidification surface of the ascending magma can be defined as the reference solidification surface. With the increase of time, this reference solidification surface can be in coincidence with the Moho surface due to the generation and propagation of the porosity wave, indicating that all the above-mentioned discussions are also valid for other cases, in which the temperature of magma solidification is higher than that of the Moho surface.
3 Potential mechanisms of heat and mass transport from lower into upper continental crust
Although magma can be directly transported from the continental lithospheric mantle into the upper continental crust through lower and middle crustal cracks, the quantity of the magma transported in such a way may be limited due to the constraints of both the magma viscosity and the heat losses to the surrounding rocks. This means that both the timing and quantity of the transported pore-fluids such as H2O and CO2 are not enough to generate giant hydrothermal ore deposits in the upper continental crust. For this reason, we will concentrate on the potential mechanism of transporting volatile gases and liquids from the lower into the upper continental crust.
Clearly, the first potential mechanism to transport volatile gases and liquids such as H2O and CO2 is the generation and propagation of the porosity wave. In this case, pore-fluids such as H2O and CO2 are transported from the lower into the middle continental crust of low porosity, just in the same way as underground pore-fluid is squeezed out of the sedimentary basin[13]. Since the porosity of the continental crustal material decreases with the increase of the depth from the earth surface, the second potential mechanism to transport volatile gases and liquids such as H2O and CO2 is the advective flow of pore-fluid. In this situation, the pore-fluid pressure is lithostatic in the lower continental crust, but between the lithostatic and hydrostatic in the middle and upper continental crust, depending on the porosity distribution within the continental crust[14]. The third potential mechanism to transport volatile gases and liquids such as H2O and CO2 from the lower into the upper continental crust is the flow focusing of the underground pore-fluid in the lower and middle continental crust[15]. If the continental crust is relatively thick and homogeneous, it is possible to transport volatile gases and liquids such as H2O and CO2 through underground convective flow within the whole continental crust[16]. Although it has been long debated about the possibility of the whole continental crust convection because of the lithostatic pressure gradient within the continental crust, it is indeed impossible for the whole continental crust convection to occur under the constant-temperature bottom condition. However, the recent theoretical analysis has demonstrated that if the constant-temperature bottom condition is replaced by the constant-conductive-heat- flux bottom condition, the whole continental crust convection of underground pore-fluid such as H2O and CO2 is possible, even though the pore-fluid pressure gradient is lithostatic within the continental crust[16].
Fig.3 shows that under the constant-conductive-heat- flux bottom condition, the convective flow is destabilized by the increase of the upward throughflow within the continental crust. In this figure, ![]() is the critical Rayleigh number and
is the critical Rayleigh number and ![]() , where v is
, where v is
the velocity of the upward throughflow, α is the thermal diffusivity of the crustal material and H is the thickness of the continental crust. The mechanism of whole-crust convection has been used to explain the gold mineralization in the Yilgarn Craton, Western Australia[17].
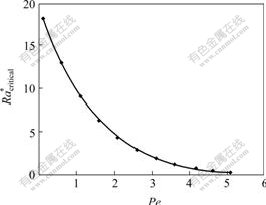
Fig.3 Variation of critical Rayleigh number with Peclet number of upward throughflow in continental crust
4 Potential mechanisms of heat and mass transport within upper continental crust
Heat and mass transport in the upper continental crust can be carried out in various ways, namely underground pore-fluid advection, convection, diffusion/ dispersion and a combination of them[18-19]. However, heat and mass transport through underground convective pore-fluid flow is one of the most effective mechanisms to ore body formation and mineralization in hydrothermal systems within the upper continental crust from the following three points of view: 1) since the underground pore-fluid moves circularly within hydrothermal systems, consumption of the pore-fluid is the minimum within the system. This enables the underground convective flow to last quite a long period of time, provided that the high temperature at the bottom of the system can last during the same period of time; 2) since the underground convective pore-fluid flow is of circular flow regime, it is an effective and efficient tool to mix different chemical species within the hydrothermal system; and 3) the underground convective pore-fluid flow may result in the highly-localized distribution of temperature in a hydrothermal system. This provides a favorable condition under which highly- localized, high-grade, giant ore deposits may be formed. For these reasons, the study of the underground convective pore-fluid flow instability in the upper continental crust has attracted ever-increasing attentions for many years[20].
4.1 Finite element simulation of convective pore-fluid flow
To demonstrate this potential mechanism, the finite element method is used to simulate the underground convective pore-fluid flow in the upper continental crust. For this purpose, it is necessary to consider the following two coupled processes: underground pore-fluid flow and heat transport. For each process, the related scientific laws can be used to derive the governing equations for describing the process itself and the contributions of another process to this process. In this regard, Darcy’s law is used to describe pore-fluid flow in porous rocks, while Fourier’s law is used to describe heat transport in porous rocks. In addition, the Boussinesq approximation, which reveals that the density of underground pore-fluid is directly proportional to the temperature difference of the underground pore-fluid, is employed to describe a change in the density of the underground pore-fluid due to a change in the temperature of the underground pore-fluid. Using the above-mentioned scientific laws, the corresponding governing equations for the coupled problem between steady-state (i.e. long-term) under- ground pore-fluid flow and heat transport in two- dimensional porous rocks can be expressed as
ui,i=0 (1)
![]() (2)
(2)
![]() (3)
(3)
![]() (4)
(4)
![]() (5)
(5)
where ui is the velocity component in the xi direction; p and T are pressure and temperature; ρ0 and T0 are the reference density of underground pore-fluid and the reference temperature of the medium; μ and cp are the dynamic viscosity and specific heat capacity of the underground pore-fluid;![]() and
and![]() are the second-order thermal conductivity tensors for the underground pore-fluid and solid matrix in the porous rock, respectively;
are the second-order thermal conductivity tensors for the underground pore-fluid and solid matrix in the porous rock, respectively; ![]() and βT are the porosity of the medium and the thermal volume expansion coefficient of the underground pore-fluid; kij is the second-order intrinsic permeability tensor of the medium; gi is the gravity acceleration component in the
and βT are the porosity of the medium and the thermal volume expansion coefficient of the underground pore-fluid; kij is the second-order intrinsic permeability tensor of the medium; gi is the gravity acceleration component in the ![]() direction.
direction.
Eqns.(1)-(5) present a set of governing equations for the fully coupled problem between the underground pore-fluid flow and heat transport in porous rocks. Due to the high non-linearity involved in the governing partial differential equations, the geometrical irregularity and material heterogeneity, this coupled problem needs to be solved using numerical methods. For this reason, the finite element method was used to discretize Eqns.(1) to (5) in Refs.[20-21]. In order to verify the derived finite element formulations and related computer program, several benchmark problems, for which the geometry and boundary conditions can be exactly simulated using the finite element method, were proposed to derive analytical solutions. By comparing the related numerical solutions of such benchmark problems with the analytical ones, the derived finite element formulations and related computer program have been verified before they are used to solve more complicated and complex problems in geological systems.
4.2 Parameters used in finite element model
The computer model considered in this investigation is a hydrothermal system of 110 km in length and 13 km in depth. The computational domain is filled with pore-fluid saturated homogeneous porous rocks, which is a part of the upper continental crust. This kind of system is called the long system, since multiple convection cells will take place. From the numerical modelling point of view, the simulation of a long system is often a challenge problem in the sense that multiple convective cells are not easy to simulate. However, the understanding of underground convective flow in long systems can help us better understand some fundamental issues behind ore body formation and mineralization in the upper continental crust.
In order to simulate geothermal conditions, the bottom is assumed to be hotter than the top of the computational domain. This means that the pore-fluid saturated rocks are uniformly heated from below. The computational domain is modeled by 3 956 quadrilateral elements. The boundary conditions of this problem are as follows: 1) temperature at the top and bottom of the computational domain is 20 ℃ and 150 ℃ respect- ively; 2) all the lateral vertical boundaries are insulated and impermeable in the horizontal direction; 3) both the top and the bottom boundaries are impermeable in the vertical direction. The following parameters are used in the computations. For the underground pore-fluid, dynamic viscosity is 1×10-3 Pa?s; reference density is 1 000 kg/m3; volumetric thermal expansion coefficient is 2.07×10-4 Pa?s; specific heat is 4 185 J/(kg?℃); thermal conductivity coefficients in both the horizontal and the vertical directions are 0.6 W/(m?℃). For the porous rock matrix, porosity is 0.1; specific heat is 815 J/(kg?℃); thermal conductivity coefficients in both the horizontal and vertical directions are 3.35 W/(m?℃); the intrinsic permeability of the whole porous rock in the computational domain is 10-14 m2.
4.3 Results from finite element simulation
Fig.4 shows the distributions of the underground pore-fluid velocity, streamline and temperature in the upper continental crust considered. In the presentation of the streamline, each clockwise convection cell is separated by an anti-clockwise convection cell. The related numerical simulation results from this example have demonstrated that: 1) multiple convection cells of the underground pore-fluid flow indeed take place in the given upper continental crust; 2) the distribution of temperature is highly localized, which is a direct result of the coupling between the temperature and pore-fluid velocity due to the occurrence of the underground convective pore-fluid flow; 3) heat and deep earth pore-fluids such as H2O and CO2 (in the case of the top boundary being permeable) can be exchanged at the interface between the earth surface, hydrosphere and atmosphere. This interaction is very important for understanding the hydro-geological effect of the deep earth pore-fluids such as H2O and CO2 on the global warming and climate change, so further research is needed to consider such an interaction on large-length and time scales.

Fig.4 Distributions of underground pore-fluid flow, streamline and temperature in the upper continental crust: (a) Pore-fluid velocity; (b) Streamline; (c) Temperature 5 Conclusions
1) The generation and propagation of porosity waves in the continental lithosphere are important mechanism to transport mass and heat fluxes from the continental lithospheric mantle into the lower continental crust. In contrast, the generation and propagation of porosity waves, underground fluid focusing through lower and middle crustal faults, advection of underground pore-fluids such as H2O and CO2 through the lower and middle crust, and whole-crust convection in particular cases, are important mechanisms to transport mass and heat fluxes from the lower into the upper continental crust.
2) Although mass and heat in the upper continental crust can be transported by underground pore-fluid advection, convection, diffusion/dispersion and a combination of them, mass and heat transport through underground convective pore-fluid flow is the most effective mechanism of ore body formation and mineralization in hydrothermal systems.
References
[1] TURCOTTE D L, SCHUBERT G. Geodynamics: Applications of continuum physics to geological problems[M]. New York: John Wiley and Sons, 1982: 450.
[2] RICHTER F M. Convection and large-scale circulation of the mantle[J]. Journal of Geophysical Research, 1973, 78: 8735-8745.
[3] BUCK W R, PARMENTIER E M. Convection between young oceanic lithosphere: Implication for thermal structure and gravity[J]. Journal of Geophysical Research, 1986, 91: 1961-1974.
[4] DOIN M P, FLEITOUT L, CHRISTENSEN U. Mantle convection and stability of depleted and undepleted continental lithosphere[J]. Journal of Geophysical Research B, 1997, 102(2): 2771-2787.
[5] NEIL E A, HOUSMAN G A. Rayleigh-Taylor instability of the upper mantle and its role in intraplate orogeny[J]. Geophysical Journal International, 1999, 138(1): 89-107.
[6] ZHANG R, HU S. A case study of the influx of upper mantle fluids into the crust[J]. Journal of Volcanology and Geothermal Research, 2002, 118(3/4): 319-338.
[7] DURRHEIM R J, MOONEY W D. Evolution of the precambrian lithosphere: Seismological and geochemical constraints[J]. Journal of Geophysical Research B, 1994, 99(8): 15359-15374.
[8] OWENS T J, ZANDT G. Implication of crustal property variations for models of Tibetan plateau evolution[J]. Nature, 1997, 387(6628): 37-43.
[9] HERMANN J, MUNTENER O, GUNTHER D. Differentiation of Mafic magma in a continental crust-to-mantle transition zone[J]. Journal of Petrology, 2001, 42(1): 189-206.
[10] BARNES H L. Geochemistry of hydrothermal ore deposits[M]. New York: John Wiley and Sons, 1997: 972.
[11] ZHAO C, HOBBS B E, ORD A, LIN G, M?HLHAUS H B. Theoretical and numerical analysis of large-scale heat transfer problems with temperature-dependent pore-fluid densities[J]. Engineering Computations, 2005, 22(2): 232-252.
[12] MCKENZIE D. The generation and compaction of partially molten rock[J]. Journal of Petrology, 1984, 25(3): 713-765.
[13] CONNOLLY J A D, PODLADCHIKOV Y Y. Temperature- dependent viscoelastic compaction and compartmentalization in sedimentary basins[J]. Tectonophysics, 2000, 324(3): 137-168.
[14] ZHAO C, HOBBS B E, M?HLHAUS H B. Analysis of pore-fluid pressure gradient and effective vertical-stress gradient distribution in layered hydrodynamic systems[J]. Geophysical Journal International, 1998, 134(2): 519-526.
[15] ZHAO C, HOBBS B E, M?HLHAUS H B, ORD A, LIN G. Analysis of steady-state heat transfer through mid-crustal vertical cracks with upward throughflow in hydrothermal systems[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2002, 26(14): 1477-1491.
[16] ZHAO C, HOBBS B E, M?HLHAUS H B. Theoretical and numerical analyses of convective instability in porous media with upward throughflow[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1999, 23(7): 629-646.
[17] SORJONEN-WARD P, ZHANG Y, ZHAO C. Numerical modelling of orogenic processes and mineralization in the south eastern part of the Yilgarn Craton, Western Australia[J]. Australian Journal of Earth Sciences, 2002, 49(6): 935-964.
[18] ZHAO C, HOBBS B E, HORNBY P, ORD A, PENG S. Numerical modelling of fluids mixing, heat transfer and non-equilibrium redox chemical reactions in fluid-saturated porous rocks[J]. International Journal for Numerical Methods in Engineering, 2006, 66(7): 1061-1078.
[19] ZHAO C, HOBBS B E, ORD A, HORNBY P, PENG S, LIU L. Mineral precipitation associated with vertical fault zones: the interaction of solute advection, diffusion and chemical kinetics[J]. Geofluids, 2007, 7(1): 3-18.
[20] ZHAO C, M?HLHAUS H B, HOBBS B E. Finite element analysis of steady-state natural convection problems in fluid-saturated porous media heated from below[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1997, 21(12): 863-881.
[21] ZHAO C, HOBBS B E, M?HLHAUS H B. Finite element modelling of temperature gradient driven rock alteration and mineralization in porous rock masses[J]. Computer Methods in Applied Mechanics and Engineering, 1998, 165(1/4): 175-187.
Foundation item: Project(10672190) supported by the National Natural Science Foundation of China
Received date: 2007-06-15; Accepted date: 2007-08-28
Corresponding author: ZHAO Chong-bin, Professor; Tel: +86-731-8830841; E-mail: chongbin.zhao@iinet.net.au

