
J. Cent. South Univ. (2018) 25: 1766-1773
DOI: https://doi.org/10.1007/s11771-018-3867-8
Stress distribution and its influencing factors of bottom-hole rock in underbalanced drilling
ZHANG Ran(张然)1, 2, LI Gen-sheng(李根生)2, TIAN Shou-ceng(田守嶒)2
1. Key Laboratory of Fluid and Power Machinery of Ministry of Education, Xihua University,Chengdu 611743, China;
2. State Key Laboratory of Petroleum Resource and Prospecting, China University of Petroleum,Beijing 102249, China
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2018
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2018
Abstract: The underbalanced drilling has been widely used due to its advantages of high drilling efficiency and low cost etc., especially for hard formation drilling. These advantages, however, are closely related to the stress state of the bottom-hole rock; therefore, it is significant to research the stress distribution of bottom-hole rock for the correct understanding of the mechanism of rock fragmentation and high penetration rate. The stress condition of bottom-hole rock is very complicated while under the co-action of overburden pressure, horizontal in-situ stresses, drilling mud pressure, pore pressure and temperature etc. In this paper, the fully coupled simulation model is established and the effects of overburden pressure, horizontal in-situ stresses, drilling mud pressure, pore pressure and temperature on stress distribution of bottom-hole rock are studied. The research shows that: in air drilling, as the well depth increases, the more easily the bottom-hole rock is broken; the mud pressure has a great effect on the bottom hole rock. The bigger the mud pressure is, the more difficult to break the bottom-hole rock; the max principle stress of the bottom-hole increased with the increasing of mud pressure, well depth and temperature difference. The bottom-hole rock can be divided into 3 regions respectively according to the stress state, 3 direction stretch zone, 2 direction compression area and 3 direction compression zone; the corresponding fragmentation degree of difficulty is easily, normally and hardly.
Key words: thermo-poroelastoplasticity; bottom-hole rock stress; fully coupled; numerical solution; fragmentation mechanism
Cite this article as: ZHANG Ran, LI Gen-sheng, TIAN Shou-ceng. Stress distribution and its influencing factors of bottom-hole rock in underbalanced drilling [J]. Journal of Central South University, 2018, 25(7): 1766–1773. DOI: https://doi.org/10.1007/s11771-018-3867-8.
1 Introduction
With the petroleum exploration and development towards deep formation continuously, the rock drill-ability becoming worse and worse, mechanical drilling rate also fell sharply. The underbalanced drilling has been widely used due to its advantages of high drilling efficiency and low cost etc., especially for hard formation drilling. These advantages, however, are closely related to the stress state of the bottom-hole rock; therefore, it is important to research the stress distribution of bottom-hole rock for the correct understanding of the mechanism of rock fragmentation and high penetration rate.
In recent years, the research on the related problems in the process of underbalanced drilling is mainly focused on the stability of the borehole, and the research on the distribution of the bottom-hole stress distribution in underbalanced drilling is relatively small. MCLELLAN et al [1] have used the software package named STABViewTM to forecast the optimum bottom-hole pressure for underbalanced drilling; SALEHI et al [2, 3] used the elastic-plastic model and finite- explicit and finite-element method to analyse the wellbore instability problems in underbalanced drilling in depleted Iranian fields; AZEEMUDDIN et al [4] established a geomechanical model to predict the optimal mud weights under various degrees of underbalanced drilling; ROSHAN et al [5] have established the three dimensional wellbore model to determine the optimal mud composition and a range of bottom hole pressure for underbalanced drilling. QIU et al [6] presented a wellbore stability study to evaluate the potential wellbore instability risk with UBD; AMINUL et al [7, 8] analyzed the reasons which cause wellbore instability during underbalanced drilling in shale. HE et al [9] derived a new wellbore collapse pressure model based on seepage mechanics and linear elastic theory and a new analytical model that takes fluid seepage into consideration is introduced to examine wellbore circumferential stresses. The high drilling efficiency and low cost of underbalanced drilling has been widely recognized by engineers; some study on stress distribution of bottom-hole rock in underbalanced drilling has been done. RUMZAN et al [10] established the three dimensional finite element model of bottom-hole rock and researched the stress state under various mud pressure; ZHANG et al [11] have analyzed the stress state of bottom-hole rock under different mud pressure and temperature and the rock failure mechanism also has been elaborated; PENG et al [12] have studied the stress state of bottom-hole rock before drilling, drilling and after drilling based on the excavation method; LI et al [13] have used the finite element method to explore the mechanism of influence exerted by pore pressure and temperature of strata on the stress state of bottom hole rock; CHANG et al [14–17] established the fluid-solid coupling model with the bottom-hole differential pressure and the numerical simulation calculation of the bottom-hole stress field under differential pressures is calculated with the finite element method. Many important conclusions have been drawn from the above research, but few studies of the fully coupled thermo-poroelastoplasticity analysis of bottom-hole rock have been found.
The main objective of this paper is to investigate the stress state of bottom-hole rock based on the fully coupled thermo- poroelastoplasticity model and the effects of overburden pressure, horizontal in-situ stresses, drilling mud pressure, pore pressure and temperature on stress distribution of bottom-hole rock are studied.
2 Thermo-poroelastic theory
It is well known that the formation temperature increases with the increase of formation depth. At the bottom-hole, the drilling mud has a cooling effect on the bottom-hole rock because its temperature is lower than formation. The bottom- hole rock matrix and the pore medium will shrink. The mutual seepage will occur between the drilling mud and pore fluid because of the pressure difference.
The constitutive equation of rock matrix is as follows:
 (1)
(1)
The coupled energy and fluid mass balance equations can be written as:
 (2)
(2)
The relevant parameters in Eq. (2) can be calculated with the following equations:
 (3)
(3)
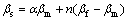 (4)
(4)
 (5)
(5)
 (6)
(6)
where the p and T represent the pore pressure and temperature respectively. The constant α represents Biot coefficient. Km is the drained bulk modulus of poroelastic matrix. Kf is the bulk modulus pore fluid. ks is the means rock thermal conductivity coefficient. km is the thermal conductivity coefficient of rock skeleton. kf is the thermal conductivity coefficient of pore fluid. βm is the thermal expansivity of rock skeleton. βf is the thermal expansivity of pore fluid. β is the Biot’s modulus. βs is the undrained thermal expansivity of saturated rock. k is the rock intrinsic permeability, k=K·μ/(γw·μ) is the fluid viscosity. cf and cm are the fluid and rock specific heat capacities. ρm and ρf mean rock skeleton and pore fluid densities. f is the body forces, and the indices i,j stand for x and y coordinate axes. The coefficients λ and G are elastic moduli, known as Lame’s parameters, v is Darcy velocity, n is porosity, t represents time.
3 Plastic yield criterion and mechanical parameters of rock
The Drucker-Prager failure criterion has been used to determine whether shear failure occurs [18–21].
 (7)
(7)
where I1 and J2 represent the first stress invariant and the second deviatoric stress invariant, respectively.
 (8)
(8)
 (9)
(9)
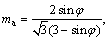
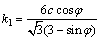 (10)
(10)
where φ is the internal frictional angle, c is the cohesion stress. σ1, σ2 and σ3 are the principle stress in the cylindrical coordinate system.
Taking the Batan-2# well in Tarim Basin as an example, the drill core is shown in Figure 1. The physical parameters of core, such as elastic modulus, Poisson ratio, permeability coefficient, porosity, cohesion stress, friction angle, are obtained by using the corresponding equipment in State Key Laboratory of oil and gas reservoir geology and Development Engineering. The measured physical parameters are shown in Table 1, the rock samples are shown in Figure 2 and Figure 3 shows the stress–strain curves in different confining pressures.

Figure 1 Drill core in Bantan-2#
Table 1 Physical parameters of rock
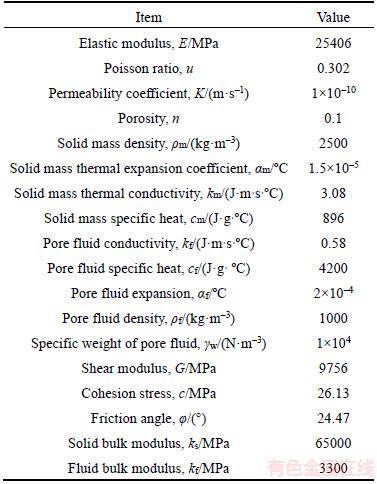

Figure 2 Rock samples (a), rock specimen after triaxial compression experiment (b) and after uniaxial compression experiment (c)
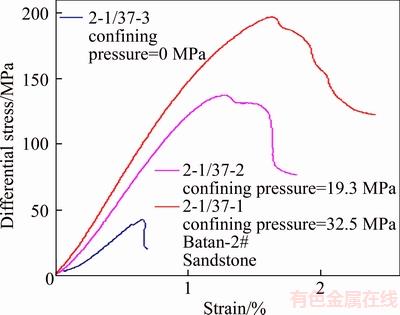
Figure 3 Stress–strain curves in different confining pressures
4 Establishment and verification of finite element model
4.1 Establishment of finite element model
The three dimensional simulation model of bottom-hole rock is established based on the fully coupled thermo-poroelastoplasticity theory. The model is squared with the length of 2000 mm; the wellbore radius is 108 mm and the depth is 1000 mm. The meshes near the wellbore wall and bottom-hole are refined; the finite element model is shown in Figure 4. The bottom-hole rock suffers overburden pressure σz, in-situ stress σH and σh, mud pressure Pw, pore pressure Pf. The temperature difference between the drilling mud and formation is also considered. The wellbore wall is a seepage boundary, the drilling fluid and the formation pore fluid can be freely circulated, that is to say, the pore pressure of wellbore wall is equal to the pressure of the drilling mud. As the process of forming a new bottom hole is soon as bit broken rock, the seepage time is assumed as 5 s. In order to facilitate the analysis, two paths X (radial) and Z (axial) are marked, as shown in Figure 4(b).
4.2 Verification of numerical simulation model
The stress distribution around the wellbore for an elastic and homogeneous formation is as follow [22]:
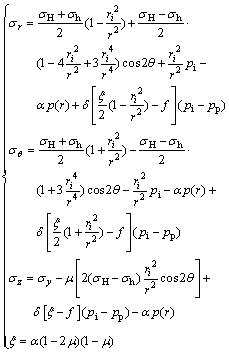 (11)
(11)
where σr, σθ, σz are the radial stress, tangential stress and z-axis stress respectively; α is Biot coefficient; δ is a coefficient related to the permeable, it is equal to 1, when the wellbore wall is permeable, or equal to 0; p(r) is the pore pressure at the r; pi is drilling mud pressure; pp is initial pore pressure; f is porosity; μ is Poisson ratio; σH and σh are the maximum and minimum horizontal principal stresses, respectively.
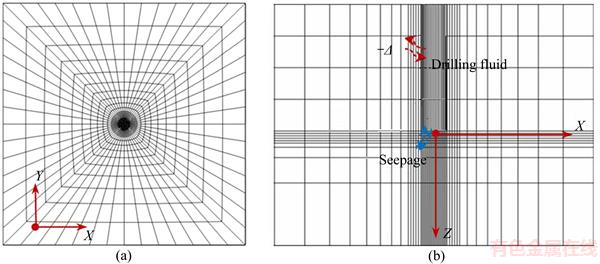
Figure 4 Finite element model of bottom-hole
In order to verify the numerical simulation results, the numerical results of wellbore wall which away from the bottom 0.8 m were compared to the analytical solutions of the pore-elastic model, the results are shown in Figure 5. Both the numerical simulation and analytical solutions are without considering the effect of temperature and the fully coupling of thermo-poroelastoplasticity. The comparisons of the two type solutions indicate the validity of numerical simulation model. The effect of temperature and rock plastic yield will be considered in the following sections.
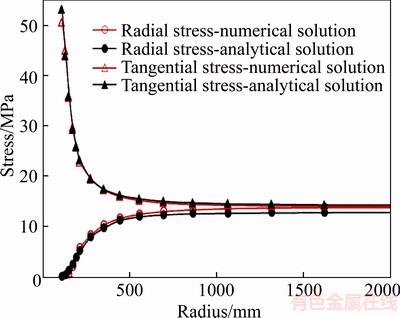
Figure 5 Comparison with numerical solution and analytical solution
5 Stress distribution of bottom-hole rock in underbalanced drilling
5.1 Effect of drilling mud pressure
The stress condition of bottom-hole rock is very complicated while under the co-action of overburden pressure, horizontal in-situ stresses, drilling mud pressure, pore pressure and temperature etc. The initial formation temperature is 90 °C, drilling mud temperature is 60 °C and well depth is 3000 m in this section. The stresses distribution along the two paths under different mud pressures is shown in Figures 6–8. As Figure 6 shows, the maximum principle stress of the bottom- hole rock increases with the increasing of drilling mud pressure and with the distance away from the axis of the borehole, the stress decreases; the minimum value appeared at the junction of the bottom hole and wall, and it presents the compressive stress. The minimum principal stress distribution is shown in Figure 7, in air drilling, the stress of the central part of the bottom-hole rock presents tensile, the other presents compressive. With the increase of the mud pressure, the tensile stress area becomes smaller, the compressive stress region becomes larger, and the minimum principal stress at the bottom-hole presents a compressive stress at last. It indicates that the bottom-hole rock can be divided into 3 regions respectively according to the stress state, 3 direction stretch zone (or unidirectional compression zone, the central part of bottom-hole), 2 direction compression area (the outside of the central part) and 3 compression zone (the junction of the bottom hole and wellbore wall); the corresponding fragmentation degree of difficulty is easily, normally and hardly.
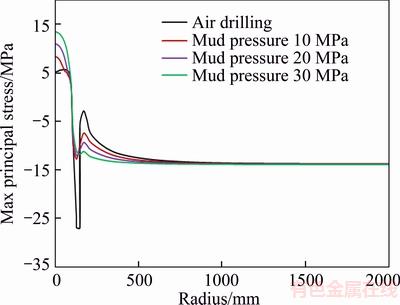
Figure 6 Effect of mud pressure on max principal stress (X-direction)
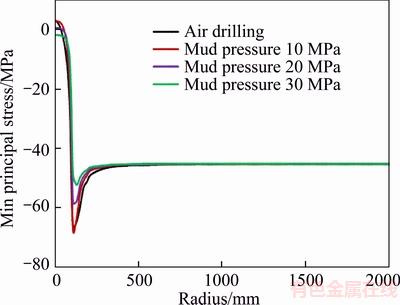
Figure 7 Effect of mud pressure on min principal stress (X-direction)
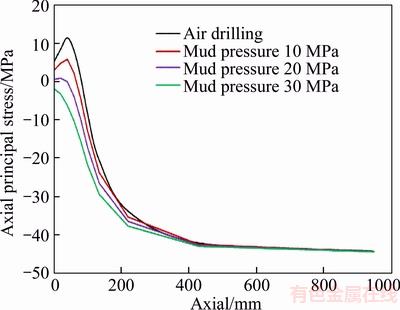
Figure 8 Effect of mud pressure on axial principal stress (Z-direction)
Figure 8 shows the stress distribution of bottom-hole rock in axial direction. As it shows that the stress increases first and then decreases in air drilling, the maximum principle stress at the bottom-hole surface is 5.1 MPa and it is 11.4 MPa away from the bottom surface 40 mm. In the underbalanced drilling, the stress decreases when the mud pressure increases, which has a change trend from the tensile stress to compressive stress. In balanced drilling, the stress of the bottom hole is completely in the state of compressive, it confirms the hold effect of mud on bottom-hole rock.
5.2 Effect of well depth
The distribution of maximum principle stress, minimum principal stress and axial stress are shown in Figures 9–11 respectively (the differential pressure is 10 MPa, temperature difference is 30 °C). With the increase of well depth, the maximum principal stress of bottom-hole surface increases. This is mainly because the increase of well depth causing the mud pressure on bottom- hole increases, the pore pressure increases, the tensile stress rock matrix suffered also increases. The minimum principal stress of central part shows little change, but it decreases rapidly near the wellbore wall and the deeper the well depth is, the smaller the stress is. Figure 11 shows the axial stress under different well depths. The axial stress decreases with the increase of well depth, which is shown as compressive stress and finally stabilized at a certain value.
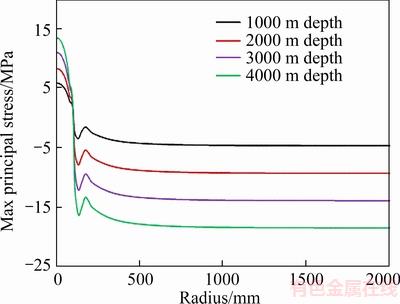
Figure 9 Effect of well depth on max principal stress (X-direction)
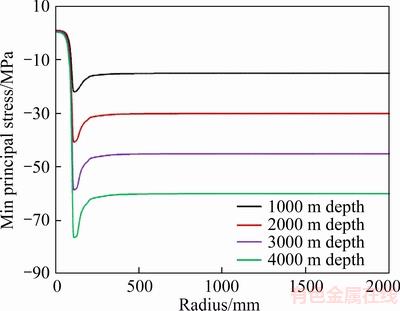
Figure 10 Effect of well depth on min principal stress (X-direction)
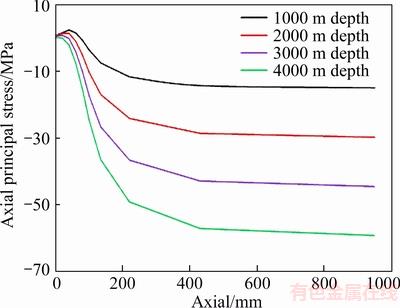
Figure 11 Effect of well depth on axial principal stress (Z-direction)
For the air drilling, the stress distribution of the bottom-hole rock along the axis of the borehole under different well depths is shown in Figure 12 (temperature difference is 30 °C). From the figure, with the increase of depth, the axial stress first increases then decreases and keep a stable state; with the increase of depth the maximum principal stress of the bottom-hole surface changes little, the stress at a certain distance below the bottom-hole increases with the increase of the well depth, which indicates that in gas drilling, the deeper the well is, the easier the rock is broken.
5.3 Effect of temperature difference
The distribution of the maximum principal stress under different temperature as shown in Figure 13, it can be seen from the figure, the bigger the temperature difference the larger the maximum principal stress, it is beneficial to rock fragmentation. As shown in Figure 14, the influence of temperature on bottom-hole rock stress only beneath the surface within the scope of a certain distance from the bottom-hole surface, which is related to the heat transfer coefficient of rock matrix and pore fluid, heat conduction time and other factors.
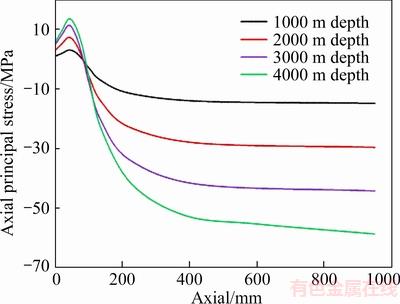
Figure 12 Effect of well depth on axial principal stress in air drilling (Z-direction)
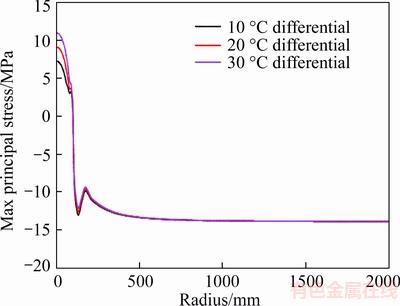
Figure 13 Effect of temperature difference on max principal stress (X-direction)
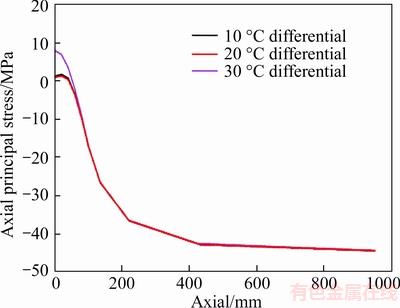
Figure 14 Effect of temperature difference on axial principal stress (Z-direction)
6 Conclusions
In this work, the fully coupled thermo-poroelastoplasticity model of bottom-hole rock is established, and then the solutions are obtained through the finite element method. The following conclusions are drawn.
1) In air drillings, the axial stress increases first and then decrease, the rock is more easily broken in the deeper well. In the underbalanced drilling, the stress decreases when the mud pressure increases, which has a change trend from the tensile stress to compressive stress. In balanced drilling, the stress of the bottom hole is completely in the state of compressive, it confirms the hold effect of mud on bottom-hole rock.
2) The bigger the temperature difference, the larger the maximum principal stress, it is beneficial to rock fragmentation. The effect of temperature difference on stress distribution only beneath the surface within the scope of a certain distance from the bottom-hole surface, which is related to the heat transfer coefficient of rock matrix and pore fluid, heat conduction time and other factors.
3) The bottom-hole rock can be divided into 3 regions respectively according to the stress state, 3 direction tensile zone (or unidirectional compression zone at the central part of bottom- hole), 2 direction compression area (the outside of the central part) and 3 direction compression zone (the junction of the bottom hole and wellbore wall); the corresponding fragmentation degree of difficulty is easily, normally and hardly.
References
[1] MCLELLAN P, HAWKES C. Borehole stability analysis for underbalanced drilling [J]. Journal of Canadian Petroleum Technology, 2001, 40(5): 31–38. DOI: 10.2118/01-05-01.
[2] SALEHI S, HARELAND G, NYGAARD R. Numerical simulations of wellbore stability in under-balanced-drilling wells [J]. Journal of Petroleum Science & Engineering, 2010, 72(3, 4): 229–235. DOI: 10.1016/j.petrol.2010.03.022.
[3] SALEHI S, HARELAND G, DEHKORDI K K. Wellbore Stability analysis in UBD Wells of Iranian fields [C]// 1st EAGE International Petroleum Conference and Exhibition. Manama, Bahrain: Society of Petroleum Engineers, 2007: SPE-105155-MS. DOI: 10.2118/105155-MS.
[4] AZEEMUDDIN M, ONG S, MAYA D A, GUZMAN E. Underbalanced drilling borehole stability evaluation and implementation in depleted reservoirs San Joaquin field, Eastern Venezuela [C]// IADC/SPE Drilling Conference. Miami, USA: Society of Petroleum Engineers, 2006: SPE-99165-MS. DOI: 10.2118/99165-MS.
[5] ROSHAN H, RAHMAN S. 3D borehole model for evaluation of wellbore instabilities in underbalanced drilling, [C]// SPE EUROPEC/EAGE Annual Conference and Exhibition, Vienna, Austria: Society of Petroleum Engineers, 2011: SPE-143320-MS. DOI: 10.2118/143320-MS.
[6] QIU K, GERRYO Y, TAN C P, MARSDEN J R. Underbalanced drilling of a horizontal well in depleted reservoir: A wellbore stability perspective [J]. SPE Drilling & Completion, 2008, 23(2): 159–167. DOI: 10.2118/105215-MS.
[7] ISLAM M A, SKALLE P, FARUK O. Analytical and numerical study of consolidation effect on time delayed borehole stability during underbalanced drilling in shale [C]// KIPCE 09/SPE Drilling Conference, Kuwait, 2009: SPE 127554. DOI: 10.3997/2214-4609.20145406.
[8] AMINUL ISLAM A, SKALLE P, TANTSEREV E. Underbalanced drilling in shale- perspective of factors influences mechanical borehole instability [C]// IPTC 09/SPE Drilling Conference, Doha, Qatar, 2009: IPTC 13826.
[9] HE S M, WANG W, TANG M, HU B, XUE W C. Effects of fluid seepage on wellbore stability of horizontal wells drilled underbalanced [J]. Journal of Natural Gas Science & Engineering, 2014, 21(11): 338–347. DOI: 10.1016/j.jngse. 2014.08.016.
[10] RUMZAN I, SCHMITT D R. The Influence of well bore fluid pressure on drilling penetration rates and stress dependent strength [M]// Rock Mechanics in the National Interest. Lisse, Netherlands: A.A. Balkema, 2001.
[11] ZHANG H, ZHANG H, GUO B, GANG M. Analytical and numerical modeling reveals the mechanism of rock failure in gas UBD [J]. Journal of Natural Gas Science & Engineering, 2012, 4(3): 29–34. DOI: 10.1016/j.jngse.2011.09. 002.
[12] PENG Y. Finite element model for analyzing stress field of bottom hole based on excavating method [J]. Acta Petrolei Sinica, 2006, 27(6): 133–136, 140. (in Chinese)
[13] LI S. Stress state of bottom-hole rocks in underbalanced drilling [J]. Acta Petrolei Sinica, 2011, 32(2): 329–334. DOI: 10.7623/syxb200606035 (in Chinese)
[14] CHANG D, LI G, SHEN Z, TIAN S C, SHI H Z, SONG X Z. A study on the effect of bottom-hole differential pressure on the rock stress field [J]. Energy Sources Part A: Recovery Utilization & Environmental Effects, 2014, 36(3): 275–283. DOI: 10.1080/15567036.2010.538808.
[15] CHANG D Y, LI G S, HUANG Z W, SHEN Z H, TIAN S C, SHI H Z. Study of effect of bottom-hole differential pressure on vertical well bottom-hole stress field [J]. Yantu Lixue/Rock & Soil Mechanics, 2011, 32(2): 356–362. (in Chinese)
[16] CHANG D Y, LI G S, SHEN Z H, HUANG Z W, TIAN S C, SHI H Z, SONG X Z. Study of bottom-hole stress field with differential pressure of 3D in-situ stress under different drilling conditions [J]. Yantu Lixue/Rock & Soil Mechanics, 2011, 32(5): 1546–1552. DOI: 10.3969/j.issn.1000- 7598.2011. 05.040. (in Chinese)
[17] CHANG D, LI G, SHEN Z, HUANG Z W, TIAN S C, SHI H Z, SONG X Z. The stress field of bottom hole in deep and ultra-deep wells [J]. Acta Petrolei Sinica, 2011, 32(4): 697–703. DOI: 10.7623/syxb201104021. (in Chinese)
[18] ZHU X H, LING Y M, HUA T. Numerical simulation of DTH bit’s gas-solid two-phase flow in air drilling [J]. Journal of Central South University: Science and Technology, 2011, 42(10): 3040–3047. (in Chinese)
[19] ZHU X, LIU W. The effects of drill string impacts on wellbore stability [J]. Journal of Petroleum Science & Engineering, 2013, 109: 217–229. DOI: 10.1016/ j.petrol.2013.08.004.
[20] ZHU Y W, CAI Y Q, XU H. ABAQUS and analyses of rock engineering [M]. Hong Kong: The Chinese Book Publishing House, 2005. (in Chinese)
[21] ZHU X, LIU W, LIU Q. The mechanism and law of wellbore instability due to drill string impact in air drilling [J]. International Journal of Oil Gas & Coal Technology, 2014, 8(2): 153–181. DOI: 10.1504/IJOGCT.2014.064843.
[22] BRADLEY W B. Failure of inclined boreholes [J]. Journal of Energy Resources Technology, 1979, 101(4): 232. DOI: 10.1115/1.3446925.
(Edited by HE Yun-bin)
中文导读
欠平衡钻井井底岩石的应力分布及其影响因素
摘要:欠平衡钻井具有钻井效率高和成本低等优势,尤其在硬地层钻井中效果显著。但是由于受上覆岩层压力、水平地应力、钻井泥浆压力、孔隙压力和井底温度等因素影响,导致井底岩石的应力状态复杂。因此,研究井底岩石的应力分布对于正确认识欠平衡钻井中的岩石破碎机理具有重要意义。本文建立了全耦合仿真模型,研究了上覆岩层压力、水平地应力、钻井泥浆压力、孔隙压力和温度对井底岩石应力分布的影响。研究表明:在空气钻井中,井深越深则井底岩石的破坏程度越高;泥浆压力对井底岩石破碎影响显著,泥浆压力越大,越难以破坏井底岩石;井底最大主应力随着泥浆压力、井深和温差的增大而增大;根据应力状态分别将井底岩体划分为3个区域:3向拉伸区、2向受压区和3向受压区。其中3向拉伸区岩石最易破坏,而3向受压区岩石则难以破碎。
关键词:热孔弹塑性耦合;井底岩石应力;完全耦合;数值解;破碎机理
Foundation item: Projects(U1562212, 51525404) supported by the National Natural Science Foundation of China; Project(JYBFX-YQ-1) supported by the Research Project of Key Laboratory Machinery and Power Machinery (Xihua University), Ministry of Education, China
Received date: 2017-03-01; Accepted date: 2017-05-16
Corresponding author: ZHANG Ran, PhD, Lecturer; Tel: +86-18160014622; E-mail: zhangran87@foxmail.com; ORCID: 0000-0002- 7671-5622