J. Cent. South Univ. (2012) 19: 2037-2046 

DOI: 10.1007/s11771-012-1242-8

Complete load transfer behavior of base-grouted bored piles

ZHANG Qian-qing(张乾青)^{1, 2, 3}, ZHANG Zhong-miao(张忠苗)^{2, 3}

1. Geotechnical and Structural Engineering Research Center, Shandong University, Ji’nan 250061, China;

2. Key Laboratory of Soft Soils and Geoenvironmental Engineering of Ministry of Education

(Zhejiang University), Hangzhou 310058, China;

3. Institute of Geotechnical Engineering, Zhejiang University, Hangzhou 310058, China;

? Central South University Press and Springer-Verlag Berlin Heidelberg 2012

Abstract: A field study on the behavior of three destructive piles in soft soils subjected to axial load was presented. All the three piles with different diameters were base-grouted and installed with strain gauges along the piles. The complete load transfer behavior of the base-grouted pile was analyzed using measured results. Moreover, the thresholds of the relative pile-soil displacement for fully mobilizing skin frictions in different soils were investigated, and pile tip displacements needed to fully mobilize tip resistances were analyzed. The results of the full-scale loading tests show that the skin frictions are close to the ultimate values when the pile-soil relative displacements are 1%-3% of pile diameter, and the pile tip displacements needed to fully mobilize the tip resistances are about 1.3%-2.0% of pile diameter. The load transmission curve of the soils around the pile tip corresponds to a softening model when the pile is loaded to failure.

Key words: destructive full-scale test; post-grouting technique; load-displacement response; axial force; tip resistance; skin friction

1 Introduction

Cast-in-situ bored piles have been widely used as the foundations of heavy-load structures because of their high bearing capacity, relatively low cost and easy length adjustments during the installation. A majority of previous studies [1-3] have been conducted to estimate the behavior of axially loaded pile. However, due to the presence of uncertainties, errors inevitably arise with the estimations. Two primary sources of error can be distinguished: those arising from the evaluation of input design parameters, such as soil properties, and those arising from geotechnical calculation models [4]. The sources of uncertainty from the soil properties have been studied by PHOON and KULHAWY [5], ZHANG et al [6], and FENTON et al [7]. ZHANG et al [4] proposed that even if input design parameters had been estimated correctly, the prediction from a geotechnical calculation model was still expected to deviate from reality due to the systematic errors associated with the model itself, and the model errors may be from the constitutive behavior of materials, simplification of boundary conditions, use of empirical assumptions, and numerical errors.

To capture a “true” behavior of pile, field tests for ultimate and serviceability limit states need to be conducted. In China, most of the field tests are carried out using working piles for verification purpose, and the loading tests are terminated before the failure state is reached. Therefore, very limited field data are available for the field test of the pile loaded to failure, and there is a need to analyze the behavior of the pile in the destructive loading test.

In this work, full-scale destructive field tests on three piles with 0.7, 0.8 and 1.0 m in diameter were carried out to investigate their axial compressive performance. Detailed measured data of the piles were adopted 1) to understand the load-displacement response of the destructive pile under compression, 2) to clarify the load transfer mechanism of the axially loaded pile, 3) to compare the measured unit skin friction with the recommended value in the Chinese Technical Code for Building Pile Foundations [8] and the cone penetration tests (CPTs), and 4) to explore the thresholds of the displacement for fully mobilizing skin frictions in different soils.

2 Site conditions and pile conditions

The field tests were conducted in Hangzhou, China. The detailed soil profiles and properties are given in Table 1, in which E_s is the compression modulus of each soil layer; γ_sat is the saturated unit weight; c and φ are the cohesion and the internal friction angle of each soil layer, respectively, which are derived from consolidated undrained (CU) triaxial tests or so-called quick shear test commonly used in China; q_su and q_bu are the recommended values of the ultimate unit skin friction and the tip resistance, respectively, estimated from the CPTs.

Table 1 Soil profiles and properties

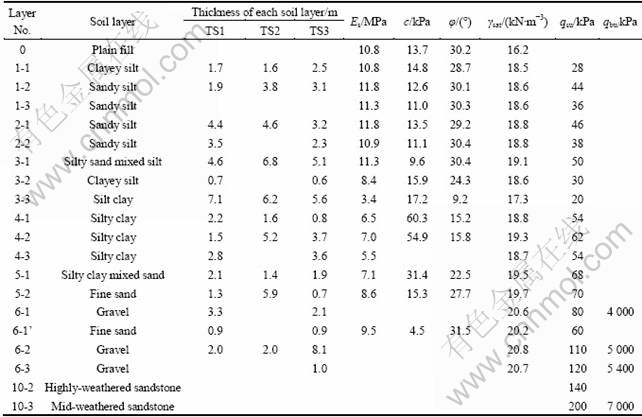
The cast-in-situ bored piles TS1 and TS2 with 0.7 m and 0.8 m in diameter had lengths of 39.1 m and 40.0 m, respectively, and were drilled into 6-2 gravel layer, whereas the 47 m-long bored pile TS3 of 1.0 m in diameter was embedded into 6-3 gravel layer. The elastic modulus of the concrete for the three piles E_c was assumed to be 33.5 GPa. All the information about the piles are summarized in Table 2, in which L is the pile length, D is the pile diameter, and α_f is the ratio of actual to theoretical concrete volume.

Table 2 Parameters of test piles

3 Base-grouting technique

After the piles were formed, base-grouting was conducted to improve pile bearing capacity through increasing both skin friction near the pile toe and pile end-bearing capacity as well as reduce settlement. Details about post-grouting technique were discussed in Ref. [9]. Grouting amount to inject around the piles  TS1, TS2 and TS3 were 2 500, 3 000 and 4 000 kg, respectively.

4 Test methods

To investigate the mechanism of load transfer, 24 vibrating wire rebar strain gauges were attached to the steel rebar cage at eight locations of each pile. Based on the locations of the strain gauges, each pile was divided into eight parts from pile top to end, as shown in Fig. 1.

A slow maintained-load method was adopted for the tests. The load was applied in increments by the reaction of jacks at the pile top, and was allowed to move under each maintained-load increment until a certain rate of displacement was achieved (e.g., the settlement was not greater than 0.1 mm within every 1 h, and occurred twice continuously). The piles were loaded to failure. The unloading tests were performed by reducing the load in decrements that were twice the loading increments.

The settlement at the pile head was derived from the dial gauges located at the pile top. The test method for the pile tip settlement was summarized as follows: 1) A steel pipe of 66 mm in diameter was attached to the steel reinforcement cage; 2) A steel pipe of 33 mm in diameter was placed inside the 66 mm-diameter steel pipe before the loading test was conducted; 3) The settlement at the pile tip was measured with the dial gauges located on the  33 mm-diameter steel pipe during the loading test.

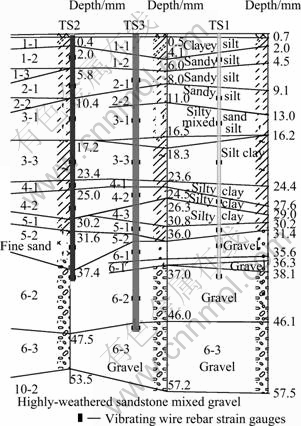
Fig. 1 Distributions of ground soil and locations of strain gauges

5 Analysis on load-test results

5.1 Load-displacement responses of pile

The pile compression is estimated by subtracting the tip settlement from the measured total head displacement. The load-settlement responses of the piles are shown in Fig. 2.

Figure 2 shows that the load-displacement curves at the pile head and the pile tip have distinct plunge points, whereas the pile compression is normal. It can also be shown that both the residual settlements at the pile head and the pile tip are very large, which indicates that the rebound displacements after unloading are nearly zero. Punching failure is therefore very likely to occur at the pile tip. The failure criteria for loading tests of the three piles are summarized as follows: 1) When the applied load at the pile TS1 head is loaded to 9 360 kN, the settlement at the pile head and the pile tip displacement sharply increases, reaching 53.2 mm and 30.3 mm, respectively. Punching failure is thereby very likely to occur at the pile tip; 2) When the pile TS2 head load increases to 11 000 kN, there is rapid increase in the settlements at the pile head and pile tip, approaching to 60.6 mm and 40.3 mm, respectively. Punching failure is thereby very likely to occur at the pile tip; 3) When the applied load at the pile TS3 head is loaded to 16 200 kN, the settlements at the pile head and the pile tip sharply increase, getting 65.5 mm and 39.0 mm, respectively. Punching failure is therefore very likely to occur at the pile tip.

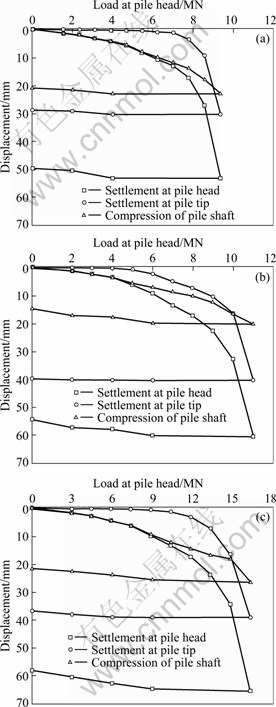
Fig. 2 Load-displacement curves of piles under compression: (a) TS1; (b) TS2; (c) TS3

The load prior to failure is determined to be the ultimate load capacity. The maximum pile head loads (or the so-called destructive loads), the ultimate bearing capacities of the three piles and their corresponding displacements are summarized in Table 3, in which P_max is the maximum applied load at the pile head; S_maxt, S_maxb and S_maxp are the measured pile head settlement, pile end displacement and pile compression under the load of P_max, respectively; P_u is the ultimate bearing capacity of the pile; S_ut, S_ub and S_up are the measured pile head settlement, pile end displacement and pile compression under the load of P_u, respectively.

Table 3 Maximum applied load, ultimate bearing capacity of piles and their corresponding displacements

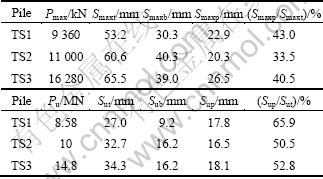
Table 3 shows that the pile compression corresponding to P_u is 51%-66% of the pile head settlement. Therefore, for the long post-grouted pile, the pile shaft compression should be taken into account, and enhancement of the shaft strength can reduce the pile settlement.

5.2 Axial force and mobilized base load

Using the measured vibration frequency of the vibrating wire strain gauge at a certain depth, the average axial force at that depth can be calculated following the method in Ref. [10]. The distribution of the axial force along pile is shown in Fig. 3.

The distribution shows that the axial force decreases with depth because of the skin friction but increases with the applied load at the pile head. The dashed curve plotted in Fig. 3 shows the distribution of the axial force under the destructive load (9 360, 12 000 and 16 200 kN for the piles TS1, TS2 and TS3, respectively). Under the destructive load, the applied load cannot keep stable, and decreases due to the degradation of the skin frictions as discussed later, e.g., the applied loads remain at 6 500,  8 000 and 11 000 kN for the piles TS1, TS2 and TS3, respectively.

According to the Chinese Technical Code for Building Pile Foundations [8], the ultimate bearing capacity of a bored pile, P_u, is determined by

              (1)

where P_sc and P_tc are the maximum computed values of the total skin friction and the tip load, respectively; q_su,I and q_bu are suggested values of the limiting unit skin friction of soil layer i and the ultimate unit base resistance, respectively; A_pb is the pile area at the pile base; A_p,i is the pile area interfacing with soil layer i; n is the number of soil layers.

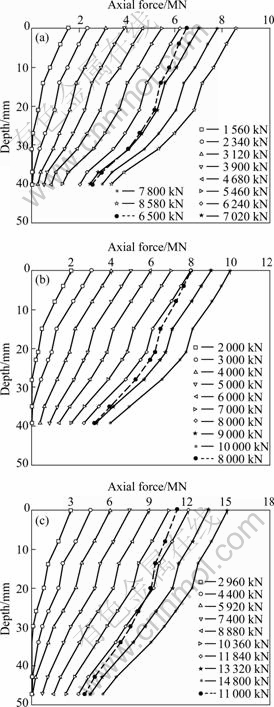
Fig. 3 Axial forces of test piles under different load levels:   (a) TS1; (b) TS2; (c) TS3

The ultimate bearing capacity, the maximum total value of the skin friction and the tip load can be estimated from the soil parameters (see Table 1) and the empirical formula of ultimate bearing capacity suggested by the Chinese Technical Code for Building Pile Foundations. These above-mentioned values are summarized in Table 4, in which P_sm and P_tm are the maximum measured values of the total skin friction and the tip load, respectively; P_uc and P_um are the maximum calculated and measured values of the bearing capacity of the pile, respectively.

Table 4 Calculated and measured values of skin friction and tip load

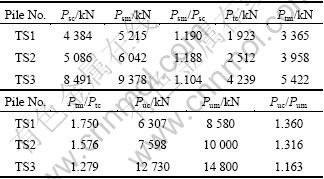
Table 4 shows that for the base-grouted piles embedded into gravel layer, the measured values of the total skin friction and the tip resistance are about 1.1-1.2 times and 1.3-1.8 times the calculated values estimated from the CPTs, respectively, whereas the measured values of the ultimate bearing capacity are about 1.2-1.4 times the calculated values.

The ratios of the mobilized base load to the total load for the three piles under different loads, expressed in percentages, are shown in Fig. 4.

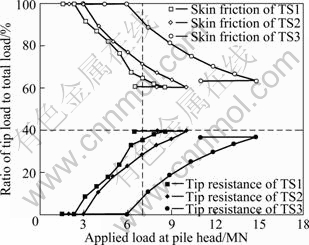
Fig. 4 Ratios of tip load to total load under different load levels

Figure 4 shows that under low load level, the applied load is all supported by the skin friction before the base load is fully mobilized. The tip load is gradually mobilized with increasing applied load. It is likely that higher level of applied load is required to mobilize tip load for the piles with larger diameter. It can also be known that under the same applied load, the ratio of the tip load to the total load decreases with increasing the pile diameter. When the applied load is close to the capacity load, the tip load of the three piles is approximately 40% of the applied load. Under the destructive load, the tip load decreases to a residual value because of the punching failure at the pile end. However, the ratio of the tip load to the pile head load is identical to that under the capacity load level.

5.3 Skin friction of each pile section

Skin friction along each pile can be calculated by dividing the difference of two consecutive axial forces by the pile area between two strain gauges. The skin friction is, therefore, an average value corresponding to the distance between the locations of two strain gauges. The distributions of the skin friction along the piles TS1, TS2 and TS3 are shown in Fig. 5.

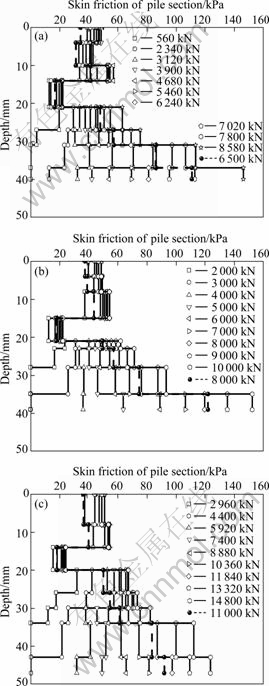
Fig. 5 Skin friction distributions along test piles: (a) TS1;    (b) TS2; (c) TS3

Figure 5 shows that the skin friction is gradually mobilized from the pile head to the pile tip and increases with increasing the applied load before the skin friction is fully mobilized. However, when the skin friction is fully developed, a reduction in the skin friction occurs as the displacement further increases. Under the destructive load, the skin friction along the pile depth decreases, as shown with the dashed line in Fig. 5.

The measured average skin frictions under the capacity load are compared with the values estimated from the CPTs and the values suggested by the Chinese Technical Code for Building Pile Foundations in Table 5, in which q_bu is the ultimate tip resistance.

The comparisons show that the measured skin frictions are approximately 1.03-1.33 times the values estimated from the CPTs. A greater factor is found for the skin frictions mobilized in the soils near the pile end. This is because the mudcake near the pile base is solidified by grouting, and larger skin frictions are mobilized. It can also be known that the ultimate tip resistances derived from the loading tests are about 1.28-1.66 times the values derived from the CPTs, indicating that the base-grouting technique has a significant enhancement effect on the tip resistance. It is noted that the value of the ultimate skin friction suggested by the Chinese Technical Code for Building Pile Foundations is determined by the soil parameters. Apparently, these values cannot reflect the influence of the soil stress state (soil depth) on the skin friction. The measured results from these loading tests show that the ultimate skin friction is different even if in the same  soil type due to the different soil stress states (soil depths). Therefore, the calculated ultimate bearing capacity of the pile will be more accurate by considering the influence of the soil stress state on the skin friction value.

5.4 Relationship between skin friction and pile-soil relative displacement

The soil displacement is assumed to have no influence on the pile displacement. Therefore, the relative displacement between soil and pile can be predicted using measured strain of the reinforcing steel bar, which can be referred to Ref. [10].

Figure 6 shows the relationship between skin friction and pile-soil relative displacement at different depths.

Figure 6 shows that the skin friction has a good correlation with the pile-soil relative displacement and decreases from a peak value with increasing load. The skin friction degradation investigated in the non- destructive test [11] only exists at shallow depth, and the shaft resistance at deeper soils is not fully developed due to less relative displacement between soils and pile. Unlike the results of the non-destructive tests, the softening is accompanied with a reduction in the skin friction and observed along the pile depth. This is to say, the skin friction along the pile depth is close to the limiting state and decreases from a peak value with increasing load due to the failure of the soil structures. This causes a decrease in the total applied load.

The thresholds of the displacement for fully mobilizing the shaft resistances in different soils, δ_u, can be derived from Fig. 6 and summarized in Table 6.

Table 5 Skin friction of soil layers under pile capacity load

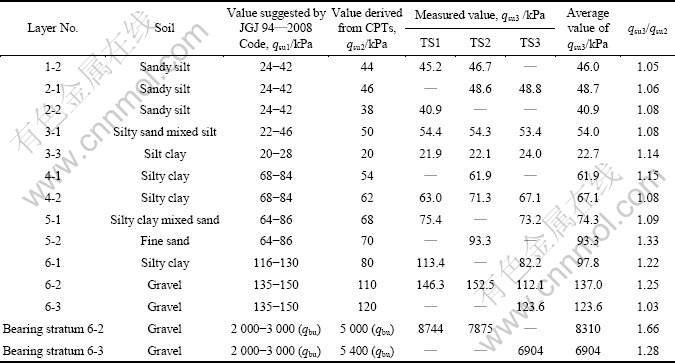
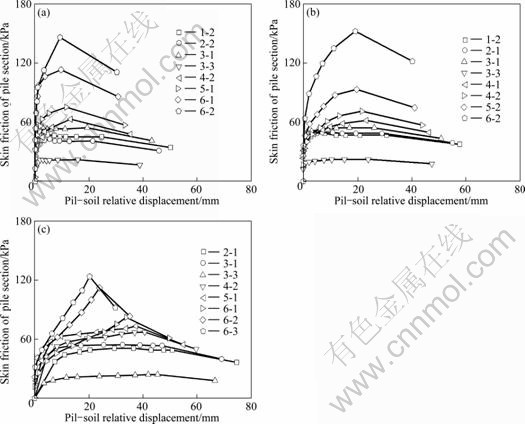
Fig. 6 Skin friction versus pile-soil relative displacement: (a) TS1; (b) TS2; (c) TS3

Table 6 Thresholds of relative pile-soil displacement for fully mobilizing skin frictions in different soils

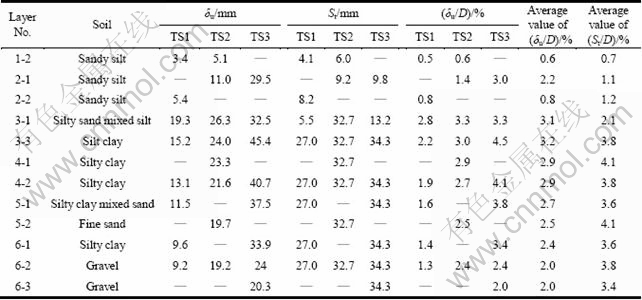
Table 6 shows that the threshold of the relative pile-soil displacement for fully mobilizing skin friction is different even if in the same soil type, due to the different soil stress states. The load tests conducted by TOUMA and REESE [12] showed that the threshold of the relative pile-soil displacement for fully mobilizing skin frictions in sand was about 13 mm, and this value was not related to pile diameter. However, the results from these full-scale loading tests show that the thresholds of the relative pile-soil displacement for fully mobilizing skin frictions in different soils generally increase with increasing the pile diameter. Generally speaking, the skin frictions are close to the limited values when the pile-soil relative displacements are 1%-3% of pile diameter (D), e.g., the thresholds of relative pile-soil displacement for fully mobilizing skin frictions in the sandy silt, silty sand mixed silt, silty clay and gravel are about 3-30 mm (0.6%-2.2%D), 20-30 mm (3.0%D), 13-23 mm (2.9%D), and 10-34 mm (2.0%-2.4%D), respectively.

It can also be concluded from Table 6 that the pile head displacement needed to mobilize ultimate skin friction ranges from 4 mm to 41 mm (about 1%-4% of pile diameter) in different soils, which is in agreement with the values suggested by XIN [13]. On the other hand, the ultimate skin frictions at shallow soils are found to be at the pile head displacement of 1% of pile diameter, which is smaller than the displacement needed for the skin frictions at deeper soils.

5.5 Tip displacement-tip resistance curves

The pile end settlement can be estimated in the following equation, as suggested by RANDOLPH and WROTH [14]:

                              (2)

where S_b is the settlement at the pile tip; P_b is the mobilized base load; G_b and υ_b represent the shear modulus and Poisson ratio of the soil beneath the pile tip (0.3 for gravel in this work), respectively; r_b is the radius of the pile tip (0.35, 0.4 and 0.5 m used in this calculation for the test piles TS1, TS2 and TS3, respectively).

As shown in Fig.7, the relationship between tip load and displacement can be approximately modeled by using a secant shear modulus of the tip soil, as expressed in the following form [14]:

                          (3)

where G_t is the secant shear modulus of the soil beneath the pile tip; G_bi is the initial shear modulus of the tip soil; R_f is the failure ratio defined as the ratio of the residual tip load to the maximum tip load (measured values of R_f, 0.85 and 0.90 used in this work, respectively); P_bmax is the maximum tip load (3 365, 3 956 and 5 422 kN for the piles TS1, TS2 and TS3, respectively).

The ultimate tip load, P_bmax, can be calculated using the following equation [15]:

                              (4)

where N_q can be determined by its relationship      with internal friction angle as shown in Ref. [16]. In this work, N_q is assumed to be 16.0; σ_v0 is the effective stress at the pile tip, which can be calculated using soil properties given in Table 1; A_b is the pile area at the pile base (0.385, 0.502 and 0.785 m² used in this calculation for the piles TS1, TS2 and TS3, respectively).

The calculated and measured values of P_bmax are summarized in Table 7.

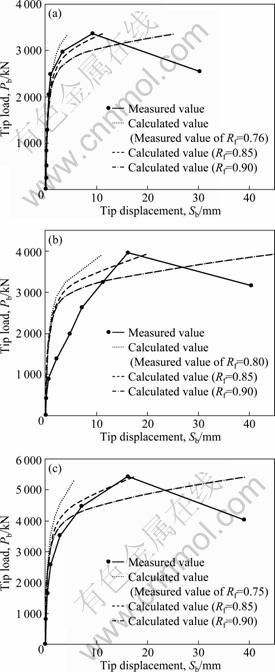
Fig. 7 Pile tip displacement versus tip load: (a) TS1; (b) TS2; (c) TS3

Table 7 Calculated and measured values of P_bmax

The results in Table 7 show that the ratio of the maximum measured tip load estimated from the field test to the maximum calculated tip load is 0.81-0.90, whereas the ratio of the maximum measured tip load derived from the CPT to the maximum calculated tip load ranges from 1.0 to 1.5. It is noted that the value of N_q significantly influences the accuracy of the calculated ultimate tip load. However, the change of the internal friction angle related to the value of N_q is not considered because of the effects of grouting on the soils beneath the pile toe. Actually, the internal friction angle of the soils around the pile base may increase, resulting in an increase in the ultimate tip load estimated from Eq. (4). Therefore, the ultimate tip loads calculated by Eq. (4) will be larger than the values given in Table 7 if these changes are taken into account, and may be consistent with the field tests results.

The theoretical tip displacement versus tip resistance curves of the piles TS1, TS2 and TS3 are shown in Fig. 7. It is noted that the tip load can be estimated from the strain measured by the strain gauge near the tip of each pile.

Figure 7 shows that the pile tip displacements needed to fully mobilize the tip resistances are 9.2, 16.2 and 16.2 mm for the piles TS1, TS2 and TS3, corresponding to 1.3%, 2.0%, 1.6% of the pile diameter, respectively. This is not consistent with the observation of O’NEILL [17] that the maximum value of tip resistance was reached at a tip settlement of perhaps 5% of pile diameter at the pile tip. The negative shaft friction will develop along the pile before the loading test when a pile is base-grouted, and the soils underneath the pile base have been pre-loaded, the pile-settlement response will therefore be stiffer than the normal piles. If the pile base soils have been pre-sheared, the additional pile base settlement to reach failure at pile tip during the loading test will perhaps be smaller than that without being base-grouted. This may explain why the settlement to reach failure of the pile tip observed is smaller than that reported by O’NEILL.

In the non-destructive loading test [11, 13], the load versus displacement relationship developed at the pile base follows a hardening model. Different from the result of the non-destructive piles, the load transmission curves of the soils around pile base correspond to softening models in the destructive loading tests, as shown in   Fig. 7. Actually, in the non-destructive loading test, the tip resistance is not fully mobilized under the maximum load. This is the reason why the load-displacement relationship developed at the pile base follows a hardening model. Actually, the hardening model of the load-displacement relationship developed at the pile base does not exist in the destructive loading tests due to the punching failure of the soils around pile base.

Figure 7 also shows that the value of the failure ratio has a significant influence on the shape of tip displacement-load curve. In this work, the measured value of the failure ratio is approximately 0.80. Before the base load is fully mobilized, the theoretical curves derived from the measured failure ratios are not generally in good agreement with the measured curves. However, the theoretical curves estimated from the assumed failure ratio of 0.85 agree well with the measured curves. The assumed value of failure ratio is therefore a key factor in simulating the hardening model of the load-displacement curve developed at the pile base in the non-destructive loading tests. These findings are useful for revising the evaluation of the pile bearing capacity and establishing a reasonable load- displacement relationship at the pile tip.

6 Conclusions

1) For the base-posted piles embedded into gravel layer, the measured total skin friction and the tip load are about 1.1-1.2 times and 1.3-1.8 times the calculated values, whereas the measured ultimate bearing capacities are about 1.2-1.4 times the calculated values. The ultimate tip resistances derived from the loading test are about 1.28-1.66 times the values derived from the CPTs, indicating that the post-grouting technique has a significant enhancement effect on the tip resistance.

2) In the destructive tests, the skin friction along the pile is close to the limiting state and decreases from a peak value with increasing the load. The results from the full-scale loading tests also show that the skin frictions are close to the ultimate values when the pile-soil relative displacements are 1%-3% of pile diameter.

3) In the destructive loading test, the load- displacement relationship developed at the pile base follows a softening model. The pile tip displacements needed to fully mobilize the tip resistances are about 1.3%-2.0% of pile diameter.

References

[1] ZHANG Qian-qing, ZHANG Zhong-miao, HE Jing-yu. A simplified approach for settlement analysis of single pile and pile groups considering interaction between identical piles in multilayered soils [J]. Computer and Geotechnics, 2010, 37(7/8): 969-976.

[2] ZHANG Min, WANG Xing-hua, WANG You. Ultimate end bearing capacity of rock-socketed pile based on generalized nonlinear unified strength criterion [J]. Journal of Central South University of Technology, 2011, 18(1): 208-215.

[3] XU Y, ZHANG L M. Settlement ratio of pile groups in sandy soils from field load tests [J]. Journal of Geotechnical and Geoenvironmental Engineering, 2007, 133(8): 1048-1054.

[4] ZHANG L M, XU Y, TANG W H. Calibration of models for pile settlement analysis using 64 field load tests [J]. Canadian Geotechnical Journal, 2008, 45(1): 59-73.

[5] PHOON K K, KULHAWY F H. Characterization of geotechnical variability [J]. Canadian Geotechnical Journal, 1999, 36(4): 612-624.

[6] ZHANG L M, TANG W H, ZHANG L L, ZHENG J G. Reducing uncertainty of prediction from empirical correlations [J]. Journal of Geotechnical and Geoenvironmental Engineering, 2004, 130(5): 526-534.

[7] FENTON G A, GRIFFITHS D V, CAVERS W. Resistance factors for settlement design [J]. Canadian Geotechnical Journal, 2005, 42(5): 1422-1436.

[8] Technical Code for Building Pile Foundations (JGJ 94—2008) [S]. China Architecture and Building Press, Beijing, 2008. (in Chinese)

[9] ZHANG Zhong-miao, YU Jun, ZHANG Guang-xing, ZHOU Xin-min. Test study on the characteristics of mudcakes and in situ soils around bored piles [J]. Canadian Geotechnical Journal, 2009, 46(3): 241-255.

[10] ZHANG Zhong-miao, ZHANG Qian-qing, YU Feng. A destructive field study on the behavior of piles under tension and compression [J]. Journal of Zhejiang University: Science A, 2011, 12(4): 291-300.

[11] ZHANG Qian-qing, ZHANG Zhong-miao, YU Feng, LIU Jun-wei. Field performance of long bored piles within piled rafts [J]. Proceedings of the Institution of Civil Engineers: Geotechnical Engineering, 2010, 163(6): 293-305.

[12] TOUMA F T, REESE L C. Behavior of bored piles in sand [J]. Journal of the Geotechnical Engineering Division, 1974, 100(GT7): 749-761.

[13] XIN Gong-feng. Test and theory study on shaft resistance softening of large diameter and supper-long piles [D]. Hangzhou: Zhejiang University, 2006. (in Chinese)

[14] RANDOLPH M F, WROTH C P. Analysis of deformation of vertically loaded pile [J]. Journal of the Geotechnical Engineering Division, 1978, 104 (12): 1465-1488.

[15] CHOW Y K. Analysis of vertically loaded pile groups [J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1986, 10(1): 59-72.

[16] BEREZANTZEV V G, KHRISTOFOROV V, GOLUBKOV V. Load bearing capacity and deformation of piled foundations [C]// Proceedings of the 5th International Conference on Soil Mechanics and Foundation Engineering. Paris, 1961, 2: 11-15.

[17] O’NEILL M W. Side resistance in piles and drilled shafts [J]. Journal of Geotechnical and Geoenvironmental Engineering, 2001, 127(1): 1-16.

(Edited by YANG Bing)

Foundation item: Project(51078330) supported by the National Natural Science Foundation of China

Received date: 2011-04-19; Accepted date: 2011-07-07

Corresponding author: ZHANG Qian-qing, PhD; Tel: +86-15868452035; E-mail: zqq5820948@126.com