
Fractal analysis of fracture surfaces in aluminum borate whisker-reinforced aluminum alloy 6061 composite
WANG Liang, WANG Li-dong, FEI Wei-dong
School of Material Science and Engineering, Harbin Institute of Technology, Harbin 150001, China
Received 17 June 2010; accepted 15 August 2010
Abstract: The effect of interface reaction on the fractal dimensions of fracture surface in aluminum borate whisker-reinforced aluminum alloy 6061 composite was investigated. The composite was fabricated by squeeze-casting technique. The fracture surfaces created in tensile test were measured by vertical sectioning method. Fractal phenomena were found in two plots, in which the measuring units were from 3 to 15 μm and 1 to 5 μm, respectively. The relation was established between the tensile properties and fractal dimension with measuring units of 3-15 μm. The results show that the ultimate tensile strength increases while the fracture surface roughness increases with fractal dimension increasing due to the change in fracture mode depending on the degree of interface reaction status induced by heat treatment. But when the measuring units are 1-5 μm, fractal dimension does not change with heat treatment.
Key words: aluminum matrix composite; aluminum borate whisker; fractal; fracture
1 Introduction
The mechanical properties of materials are related to the fracture mechanism directly, especially during microscopic fracture events and crack extending process. Therefore, quantitative fractography plays an important role in material research by using both optical microscope and scanning electron microscope. In 1984, MANDELBROT et al[1] first introduced the concept of fractal dimension to materials science in order to discover the affiliation between mechanical properties and the tortuosity of fracture surfaces, consequently, fractal was used to describe such self-affine nature of the body with irregular geometrical shape. Many methods were invented to measure the fractal dimension of fracture surface, among which slit island method[2] and vertical sectioning method[3] were the most effective ones. Although some contradiction was found between those two methods, most researchers chose the vertical sectioning method since it related better with the properties[4]. In recent studies, this analysis method has achieved great results in theoretic simulation[5-6] as well as in solving practical problems[7-9].
Metal alloy matrix composites (MMCs) reinforced by inorganic whiskers have been widely studied as important structural materials in the 21th century, due to their excellent properties and designable performance. Among such MMCS, aluminum matrix composite reinforced by aluminium borate whiskers (Al18B4O33w, denoted by ABOw) has great civil prospects because of its good performance and low cost. Recent research[10] indicated that the interface reaction between ABO whisker and aluminum alloy matrix is the key influencing factor to their mechanical properties, since such reaction produces spinel phases nearby whiskers, and the degree of interfacial reaction could be dominated by heat treatment directly, especially when the matrix alloy contains magnesium. In previous study[11], a relationship between the characteristic of subcracks on fracture surfaces and the mechanical performance of the composite was obtained.
Since fractal method is barely used in MMCs research, and there is bifurcation in even scanty results[4, 7], it is reasonable to pay great attention to investigating their fracture fractal behavior. In the present study, the relationship between the mechanical properties of ABOw/6061 composite and its fractal pattern in fracture surface is analysed and the fractal mechanism of the composites is studied.
2 Experimental
2.1 Specimen preparation
Aluminum borate whiskers reinforced aluminum alloy 6061 was used as experimental material. The whisker has a diameter of 0.5-2 μm and a length of 10-30 μm, and has a volume fraction of 25% in the composite. The composite was fabricated by squeeze- casting technique under a pressure of 200 MPa at 800 °C and its die temperature was about 500 °C. The size of tensile specimens cut from the composite ingot is shown in Fig.1. Those specimens were solution treated (T4 treatment) in a NaCl salt-bath furnace at 510 °C for 20, 50, 80, 110 and 150 min, respectively, and then quenched into water at room temperature. Those specimens were polished and then tested on an INSTRON 5569 tensile machine. Fractographs were examined by a Hitachi S3700 SEM and a Philips CM-12 TEM.

Fig.1 Dimensions of tensile specimen (Unit: mm)
2.2 Fundamental of vertical sectioning method
Considering the fracture crack path is a self-affine Koch-Kurve, such irregular object can be described by its fractal dimension. MANDELBROT[12] firstly pointed out that measuring the length of an irregular curve was related with the scale used to measure it. While the magnification increased or the size of the measuring unit decreased, the length increased without a limit. In that case, the relationship between the measured length (L) and the measuring unit (η) can be obtained:
 (1)
(1)
where L0 is a constant and D represents the fractal dimension of the curve. Therefore, by linearizing Eq.(1), the linear relationship between transformed measured length and measuring unit is obtained:
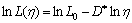 (2)
(2)
where D*=D-1.
Then the fractional part of the fractal parameter of the self-similarity curve can be calculated, which educes the fractal dimension D.
3 Results and discussion
3.1 Microstructure and interface reaction
From the TEM photographs of as-cast composite (Fig.2(a)) and solution-treated composite (Fig.2(b)), it can be seen that the interface becomes rougher after solution treatment because interfacial reaction produces more reaction product MgAl2O4 particles, as shown in reactions (3). In other words, the degree of interfacial reaction increases with increasing solution treatment time, which is in agreement with the previous research[13].
 (3)
(3)
In recent study[11], it was declared that suitable interfacial reaction does not reduce the composite mechanical properties but improves the stress transfer ability of the interface between whiskers and matrix. A proper amount of spinel product could increase the roughness of interface, which also conduces to a better stress transmission from the softer matrix to the harder whiskers, leading to a better performance. If the degree of interface reaction is too low, the interface will be flat and easy to debond; while if it is too high, large brickle reaction will be produced, which leads the interface easy

Fig.2 TEM micrographs of ABOw/6061 composites: (a) As-cast; (b) 510 °C solution treated for 120 min
to laminate. Since the mechanical properties depend on the strength of interface, the composites do not have good performance in both conditions above.
3.2 Self-affine and fractal dimensions of fracture surface
Figure 3 shows the side view of a representative composite fracture surface in different magnifications. It can be seen that the fracture curve of composite is self-affine, all the fractographs have a similar impression and cannot be distinguished if the scales are not given. It suggests that the fracture surface of ABOw/6061 composite is a typical Koch-Kurve, which could be investigated by fractal analysis method. Figure 4 shows the comparison of fracture side view of the composite and 6061 aluminum alloy.
Different from the composite micrographs, lots of tear ridges can be found on the fracture surface of 6061 alloy, which indicates that these two specimens rupture with different mechanisms. The aluminum alloy has typical microvoid accumulation fracture and produces a

Fig.3 SEM micrographs of tensile fracture side view of ABOw/6061 composite in different magnifications
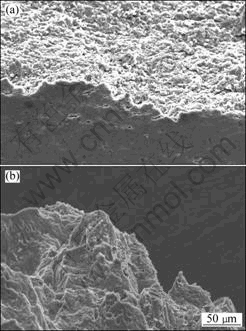
Fig.4 SEM micrographs of tensile fracture side view of specimens: (a) ABOw/6061 composite; (b) 6061 Al alloy
different crack curve. Comparing with the composite, the aluminum alloy 6061 has a much more uniform microstructure which determines its fracture behavior, leading to a quite various crack surface. However, the crack surface of 6061 alloy is also self-affine and has its own fractal property.
Vertical sectioning method was carried on hundreds of SEM micrographs, the measuring units varied from 1 to 20 μm and the measured lengths were about 2 000 m. The representative fractal dimensions of fracture curves are obtained, as shown in Fig.5. These specimens are aluminum borate whisker-reinforced aluminum alloy 6061 composites with different T4 heat treatment time. Both hollow circles and solid squares in Fig.5 are data points needed in Eq.(2), but only those solid square ones are analyzed, since the hollow circles are not in accordance with Eq.(2). In other words, whether those measuring units are too long or too short, the fracture curve does not have obvious fractal characters. That is to say, the major fractal plot is between such measuring units, and it is about 3 to 15 μm in this situation. The fractal dimensions of these specimens are educed out of such data. The little error of linear analysis indicates that the fractal phenomenon in composite fracture curve is remarkable, and the difference among fractal dimensions of those specimens is obviously related to their fracture mechanism, which will be discussed later.
It is easy to understand when the measuring unit is too long, fractal behavior is hard to find[6, 12]. But when the measuring unit decreases, the fractal will always exist theoretically. Also, plenty of researches indicated that

Fig.5 Fracture curves of different composites: (a) As-cast; (b) 510 °C solution treated for 20 min; (c) 510 °C solution treated for 50 min; (d) 510 °C solution treated for 80 min
mixed fractals could take place in the same specimen[14], and they may have superposed plots and may have different qualities. The fractal dimensions of composites were examined when the measuring units η ranged from 1 to 5 μm, and fractal phenomena are shown in Fig.6, which is the high magnification micrographs of the same fracture curves studied in Fig.5. Since the truth of fractal existing was discussed clearly, the number of data points was decreased and a reasonable result was also obtained. Regardless of measurement error and the mathematical error, it is found that this group of fractal dimensions hardly changes with heat treatment, which suggests that the microstructure of all composite fracture cracks has not changed evidently due to the T4 heat treatment when the measuring unit is less than 5 μm.
Consulting the interface reaction product MgAl2O4 particles mentioned in Eq.(3) are in the size of the length of ABOw and they always emerge along their long-axis since they are spinel[15], it is clear that such large objects do not affect the fracture fractal dimension with small measuring unit efficaciously. But when the measuring units become larger than the diameter of a whisker, fractal phenomena will be affected by the interface reaction products. Because the fractal dimension is related to the roughness of fracture surface, if any large planes emerge on the fracture surface, the fractal dimension will be reduced by them, whether these planes are large spinel product or whiskers. This theory explains the effect of T4 treatment on the fractal dimension of composites fracture surface.
3.3 Tensile properties and fractal dimensions
All fracture curves of composites heat treated for different time were analyzed. The major fractal dimensions result and the ultimate tensile strength (UTS) of specimens for correlation are shown in Fig.7. Since most researchers pointed out that fractal dimension increases with increasing toughness in material[8, 14], the composite used in this study is also ensured to have such positive correlation. Although the untreated composite has a discrete fractal dimension, it is reasonable to believe that the composite has a different fracture mode since there is almost no interface reaction product containing in this composite. Another proof is that the aluminum alloy 6061 itself has a fractal dimension of 1.062, which obviously cannot be discussed
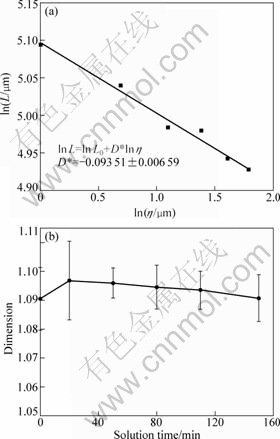
Fig.6 Fracture curve (a) and fractal dimensions (b) of composites with small measuring unit
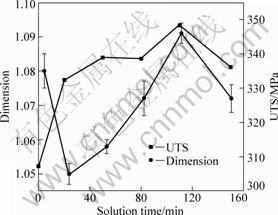
Fig.7 Fractal dimensions and UTS of ABOw/6061Al composites
together with the composites since they have quite different fracture mechanism.
It is believed that the change of both fractal dimensions and UTS properties are determined by interface reaction. Too little product would lead to an interface debonding, while too much interface reaction product would lead to an interfacial fracture. Both mechanisms bring about low UTS since the stress is not transmitted to whiskers, and engenders a lower surface roughness at meantime, which suggests a low fractal dimension. In other words, at the very beginning, a quantity of interface reaction product particles create different kinds of fracture surface by presenting themselves in different fracture mechanisms, which affects the fractal dimension. The fracture modes of “interface debonding”, “whisker twisting off” or “product brittle fracture” decided by the increase of interface reaction make the interface have a peak of toughness which affects the UTS, and also make the fracture surface have a peak of roughness which affects the fractal dimension at the same time.
4 Conclusions
1) The degree of interfacial reaction increases with increasing solution treatment time. Suitable interfacial reaction can improve the UTS of aluminum borate whisker-reinforced aluminum alloy 6061 composites, up to maximum of 348 MPa.
2) The fracture curves of composite are self-affine, which are fractal surfaces in a plot from 3 to 15 μm and another plot from 1 to 5 μm. Using vertical sectioning method, such curves are measured and their fractal dimensions are calculated.
3) The fractal dimension has a positive correlation with the composite mechanical properties when the measuring units are much larger than the diameter of whisker, from 3 to 15 μm, and it is related to the interface reaction between whiskers and matrix alloy. While the measuring units are close to the diameter of whiskers, 1 to 5 μm, the fractal dimension barely changes with heat treatment.
References
[1] MANDELBROT B B, PASSOJA D E, PAULLAY A J. Fractal character of fracture surfaces of metals [J]. Nature, 1984, 308(4): 721-722.
[2] LIN G M, LAI J K L. Fractal characterization of fracture surfaces in a resin-based composite [J]. Journal of Materials Science Letters, 1993, 12(7): 470-472.
[3] LUNG C W, MU Z Q. Relationship of initiator length with fractal dimension of fractured surfaces of high strength steels [J]. Journal of Materials Science and Technology, 1996, 12(6): 479-480.
[4] LUNG C W. Fractal description of fractured surfaces in materials [J]. Key Engineering Materials, 1998(145-149): 145-150.
[5] MECHOLSKY J J. Estimating theoretical strength of brittle materials using fractal geometry [J]. Materials Letters, 2006, 60(20): 2485-2488.
[6] ANNAMARIA C, ANTONELLA T, Leonardo Esposito. Fractal analysis of cracks in alumina-zirconia composites [J]. Journal of the European Ceramic Society, 2003, 23(3): 469-479.
[7] SPAGNOLI A. Fractality in the threshold condition of fatigue crack growth: an interpretation of the Kitagawa diagram [J]. Chaos, Solitons and Fractals, 2004, 22(3): 589-598.
[8] VENKATESH B, CHEN D L, BHOLE S D. Three-dimensional fractal analysis of fracture surfaces in a titanium alloy for biomedical applications [J]. Scripta Materialia, 2008, 59(4): 391-394.
[9] MAHAMUD M. Textural changes during CO2 activation of chars: A fractal approach [J]. Applied Surface Science, 2007, 253(14): 6019-6031.
[10] FEI Wei-dong, JIANG Xiang-dong, LI Chao, YAO Zhong-kai. Effect of interfacial reaction on fracture behaviour of aluminium borate whisker reinforced aluminium composite [J]. Material Science and Technology, 1997, 13: 918-922.
[11] WANG Liang, HU Jin, LI Zhi-jun, FEI Wei-dong. Fracture behavior of aluminum borate whisker-reinforced aluminum alloy 6061 composite [J]. Materials Science and Engineering: A, 2008, 497(1-2): 358-362.
[12] MANDELBROT B B. The fractal geometry of nature [M]. San Francisco: WH Freeman, 1982: 223-225.
[13] HU Jin, FEI Wei-dong, YAO Zhong-kai. Microstructure evolution of alumina borate whisker reinforced AA2024 composite after T6 treatment [J]. Materials Science and Engineering A, 2002, 333: 170-175.
[14] DAGUIER P, NGHIEM B, BOUCHAUD E, CREUZET F. Pinning and depinning of crack fronts in heterogeneous materials [J]. Physical Review Letters, 1997, 78(6): 1062-1065.
[15] HU Jin, CHU Wu-yang, FEI Wei-dong, ZHAO Lian-cheng. Effect of interfacial reaction on corrosion behavior of alumina borate whisker reinforced 6061Al composite [J]. Materials Science and Engineering A, 2004, 374: 153-159.
硼酸铝晶须增强6061铝合金复合材料的断口分形分析
王 靓,王黎东,费维栋
哈尔滨工业大学 材料科学与工程学院,哈尔滨 150001
摘 要:硼酸铝晶须增强6061铝合金基复合材料中的界面反应影响其断口分形维数。该复合材料由压力铸造法制备,采用垂直剖面法分析拉伸断裂断口。当量尺度分别取3-15 μm以及1-5 μm时,可以在断口上发现分形现象。当量尺度在3-15 μm的分形域,其分形维数与材料抗拉强度显著正相关。实验结果表明,随热处理时间的延长,界面反应产物的分布与状态可以改变材料的微观断裂模式,进而影响断口的粗糙度和分形维数,同时决定材料的抗拉强度。而当量尺度为3-15 μm的分形域,其分形维数受热处理程度的影响不很显著。
关键词:铝基复合材料;硼酸铝晶须;分形;断裂
(Edited by FANG Jing-hua)
Foundation item: Project (20070213042) supported by Specialized Research Fund for the Doctoral Program of Higher Education, China
Corresponding author: FEI Wei-dong; Tel: +86-451-86418647; E-mail: wdfei@hit.edu.cn
DOI: 10.1016/S1003-6326(11)60737-1