Structural stability of intermetallic compounds of Mg-Al-Ca alloy

ZHOU Dian-wu(周惦武)^{1, 2}, LIU Jin-shui(刘金水)³, ZHANG Jian(张 健)³, PENG Ping(彭 平)³

1. State Key Laboratory of Advanced Design and Manufacturing for Vehicle Body, Hunan University,

Changsha 410082, China;

2. School of Mechanical and Automobile Engineering, Hunan University, Changsha 410082, China;

3. School of Materials Science and Engineering, Hunan University, Changsha 410082, China

Received 13 October 2006; accepted 22 December 2006

Abstract: A first-principles plane-wave pseudopotential method based on the density functional theory was used to investigate the energetic and electronic structures of intermetallic compounds of Mg-Al-Ca alloy, such as Al₂Ca, Al₄Ca and Mg₂Ca. The negative formation heat, the cohesive energies and Gibbs energies of these compounds were estimated from the electronic structure calculations, and their structural stability was also analyzed. The results show that Al₂Ca phase has the strongest alloying ability as well as the highest structural stability, next Al₄Ca, finally Mg₂Ca. After comparing the density of states of Al₂Ca, Al₄Ca and Mg₂Ca phases, it is found that the highest structural stability of Al₂Ca is attributed to an increase in the bonding electron numbers in lower energy range below Fermi level, which mainly originates from the contribution of valence electron numbers of Ca(s) and Ca(p) orbits, while the lowest structural stability of Mg₂Ca is resulted from the least bonding electron numbers near Fermi level.

Key words: plane-wave pseudopotential theory; structural stability; electronic structure; Al₂Ca; Al₄Ca; Mg₂Ca

Magnesium alloys, especially approximately 90% Mg-Al-based alloys, have been used in automobile industry as cast products[1-2]. For the Mg-Al-based alloys, β-Mg₁₇Al₁₂ is an essential phase that plays an important role in strengthening grain boundary and suppressing high-temperature grain-boundary sliding, whereas the softening of the phase at the elevated temperature is detrimental to the creep property of the alloys. Therefore, the use of these magnesium alloys is limited. Due to the low cost, calcium has been used for improving the poor heat resistance property of Mg-Al- based alloys since 1980’s, and there have been increasing attentions to the development of the Mg-Al-Ca based alloys in automobile industry as cast products.

The recent experiment investigations show that the addition of calcium to the Mg-Al-based alloys has the effect on enhancing the thermal stability of the Mg₁₇Al₁₂ precipitates at elevated temperatures by forming the structure of (Mg,Ca)₁₇Al₁₂ solid solution or has the effect on greatly improving the heat resistance by forming magnesium-calcium and aluminum-calcium intermetallic compounds[3-6]. MIN et al[7] investigated the valance electron structure(VES) of Al₂Ca，Al₄Ca and Mg₂Ca intermetallic compounds by method of the empirical electron theory(EET). They thought that the bond network of Al₂Ca has high bond energy and the difference of the bond strength between different crystalline directions is not remarkable, so its stability is the highest among the three structures studied. Both Al₄Ca and Mg₂Ca have weak bonds or weak bond zones that lead to breakage easily, which accounts for the low stability of the structure. But the alloying ability and the structural stability of these intermetallic compounds in Mg-Al-based alloys containing calcium have not been well studied yet from the alloy energy view. ZHOU et al[8] investigated the structure stability of calcium alloying Mg₁₇Al₁₂ phase. In this study, a first-principles plane-wave psendopotentials method based on density functional theory was used to investigate the energetic and electronic structure of Al₂Ca, Al₄Ca and Mg₂Ca phases. Moreover, the structural stability and electronic mechanism of these phases were studied.

2 Method and models of computation

2.1 Method of computation

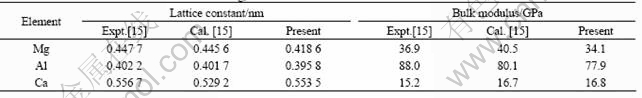
Cambridge Serial Total Energy Package (CASTEP) [9], a first-principles plane-wave psendopotential method based on density functional theory, was used in this work. CASTEP used a plane-wave basis set for the expansion of the single-particle Kohn-Sham wave-functions, and psendopotential to describe the computationally expensive electron-ion interaction, in which the exchange-correlation energy by the generalized gradient approximation(GGA) of Perdew was adopted for all elements in the models by adopting Perdew-Burke- Ernzerhof parameters[10]. Ultrasoft psendopotential represented in reciprocal space was used[11]. A finite basis set correction and the Pulay scheme of density mixing were applied for the evaluation of energy and stress[12-14]. The atomic orbits used in the present calculations are: Mg 2p⁶3s², Al 3s²3p¹, Ca 3s²3p⁶4s². To access the accuracy of computation method, a series of calculations were performed on the bulk properties of Mg, Al and Ca. The results are listed in Table 1. All atomic positions in the model were relaxed according to the total energy and force using the Broyden-Flecher- Goldfarb-Shanno(BFGS) scheme, based on the cell optimization criterion (RMs force of 5.0×10^-5 eV/nm, stress of 0.01 GPa, and displacement of 5.0×10^-5 nm). The calculation of total energy and electronic structure was followed by cell optimization with self-consistent- field(SCF) tolerance of 5.0×10^-7 eV. In the present calculation, the cutoff energy of atomic wave functions (PWs), E_cut, was set at 330 eV. Sampling of the irreducible wedge of the Brillouin zone was performed with a regular Monkhorst-Pack grid of special k-points, which is 6×6×6.

Table 1 Lattice constants and bulk modulus for Mg, Al and Ca

2.2 Models of computation

The structure of Al₄Ca is tetragonal DI₃ type as shown in Fig.1(a). Its unit cell has the highest symmetry 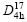,space group I₄/mmm, 10 atoms with lattice constants of a=b=0.435 nm and c=1.107 nm. The atomic coordinates in the unit cell are

+2Ca: (0, 0, 0);

+4Al(Ⅰ): (0, 0, z), (0, 0, -z), z=0.380;

+4Al(Ⅱ): (0, 1/2, 1/4), (1/2, 0, 1/4).

The primitive cell of Al₄Ca was used in the calculations as shown in Fig.1(b). It has 5 atoms, i.e. 1 calcium atom and 4 aluminum atoms. 4 aluminum atoms are 2 Al(Ⅰ) and 2 Al(Ⅱ) atoms.Al₂Ca has an ordered cubic C15 structure with lattice constants a=b=c=0.802 nm. As shown in Fig.1(c), its unit cell has the highest symmetry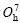 space group Fd3m. In a unit cell of the Al₂Ca phase there are 24 atoms and their atomic coordinates are as follows:

+8Ca: (0, 0, 0), (1/4, 1/4, 1/4);

+16Al: (5/8, 5/8, 5/8), (7/8, 7/8, 5/8), (5/8, 7/8, 7/8), (7/8, 5/8, 7/8).

The primitive cell of Al₂Ca was used in the calculations as shown in Fig.1(d). It has 6 atoms, i.e. 2 calcium atoms and 4 aluminum atoms.

Mg₂Ca has a hexagonal C14 type structure as shown in Fig.1(e) with the lattice constants of a=b=0.622 nm and c=1.000 nm, its unit cell has the highest symmetry 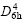 space group P6₃/mmc. There are 12 atoms in a unit cell and their atomic coordinates are as follows:

+4Ca: (1/3, 2/3, z), (-1/3, -2/3, -z), (1/3, 2/3, 1/2-z), (-1/3, -2/3, z-1/2), z=0.062;

+2Mg(Ⅰ): (0, 0, 0), (0, 0, 1/2);

+6Mg(Ⅱ): (x, x, 1/4), (-2x, -x, 1/4), (x, -x, 1/4), (-x, -x, -1/4), (2x, x, -1/4), (-x, x, -1/4), x=-0.170.

The unit cell of Mg₂Ca was used in the calculations.

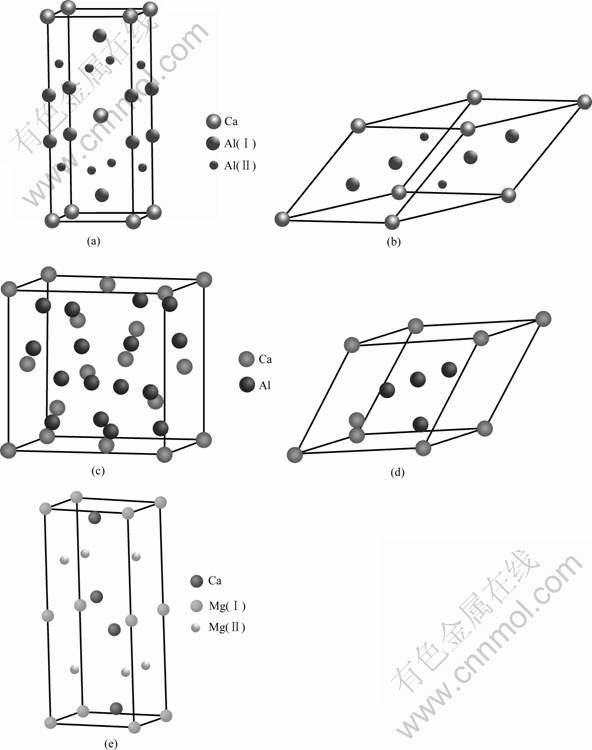
Fig.1 Models of crystal cell (a) and primitive cell (b) of Al₄Ca, crystal cell (c) and primitive cell (d) of Al₂Ca, crystal cell of Mg₂Ca (e)

3 Results and discussion

3.1 Equilibrium lattice constants

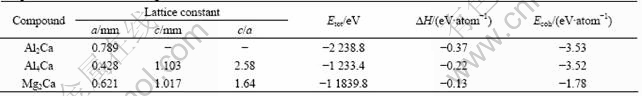
The lattice constants of Al₂Ca, Al₄Ca and Mg₂Ca structures were estimated from the minimized total energy. The results are listed in Table 2. It is found that the present lattice constant a of Al₂Ca is 0.789 nm (the value is obtained by conversion of the lattice constant  0.557 6 nm of the primitive cell of Al₂Ca), which is close to the experimental values[7] of a=0.804 nm and the error of lattice constant calculated here relative to the experiment result is about 1.87%. The present lattice constants a, c and c/a ratio of Al₄Ca are 0.428 nm, 1.103 nm (the value is obtained by conversion of the lattice constant 0.629 4 nm of the primitive cell of Al₄Ca), 2.58, respectively, which are close to the experimental values[16] of a=0.436 nm, c=1.109 nm and c/a=2.54, and the error of c/a ratio calculated here relative to the experiment result is about 1.55%. The present lattice constants a, c and c Table 1/a ratio of Mg₂Ca are 0.621 nm, 1.017 nm, 1.64, respectively, which are also close to the experimental values[7] of a=0.624 nm, c=1.015 nm and c/a=1.67. Hence, the computation parameters selected in this paper are suitable.

3.2 Formation heat

Formation heat (?H) of Al₂Ca, Al₄Ca and Mg₂Ca crystal or primitive cell per atom was calculated by using the following expression[17-18]:

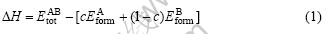
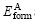where   refers to the total energy per atom of intermetallic compound at the equilibrium lattice constant, and are the single atomic energies of pure constituents A and B in the solid states, respectively, c refers to the fractional concentration of the constituent A. The total energies of Al₂Ca, Al₄Ca primitive cell and Mg₂Ca crystal cell are also listed in Table 2. The energy of the single atom was also calculated by using the same code as crystal or primitive model. The calculated energies of Mg, Al and Ca atoms are -977.9, -57.2 and -1 003.8 eV, respectively. The calculated results of formation heat of Al₂Ca, Al₄Ca and Mg₂Ca are also listed in Table 2. It is found that formation heat of Al₂Ca and Mg₂Ca is -0.37, -0.13 eV/atom, respectively, which is close to the corresponding result of -39.1 kJ/mol (about -0.405 24 eV/atom), -4.5 kJ/mol (about -0.046 64 eV/atom), from thermodynamic data in Ref.[19]. Further analysis shows that formation heat of Al₂Ca, Al₄Ca and Mg₂Ca is all negative, which means that the structure of these phases can exist and be stable[20]. Because the negative formation heat of Al₂Ca, Al₄Ca and Mg₂Ca is increased gradually, it can be inferred that Al₂Ca phase has the strongest alloying ability, next Al₄Ca, finally Mg₂Ca.

Table 2 Equilibrium lattice constants (a, c), total energy (E_tot), formation heat (?H) and cohesive energy (E_coh) of intermetallic compounds Al₂Ca, Al₄Ca and Mg₂Ca

3.3 Cohesive energy

The cohesive intensity and structural stability of crystal is correlation to its cohesive energy[20], and the cohesive energy of crystal is defined as either the energy that is needed when the crystal is formed by combining with the freedom atom, or the work that is needed when the crystal decomposes into the single atom. Hence, the bigger the cohesive energy, the more stable the crystal structure[20]. In this work, the cohesive energies (E_coh) of Al₂Ca, Al₄Ca and Mg₂Ca crystal or primitive cell per atom were calculated by[18]

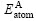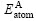where  and are the total energies of atoms A and B in the freedom states. The energies of Mg, Al, Ca free atom are -976.4, -53.5 and -1 001.8 eV, respec- tively. The energies of the free atoms were also calculated by using the same code as crystal or primitive model. The cohesive energy of the single atom of all crystal or primitive cell was calculated by Eqn.(2). The results are also listed in Table 2. It is found that the cohesive energy of the single atom of Al₂Ca is E_coh=-3.53 eV/atom, that of Mg₂Ca is E_coh=-1.78 eV/atom. Compared with the experimental results[19], the total energy of Al₂Ca is -316.9 kJ/mol (about -3.28 eV/atom), that of Mg₂Ca is -160.4 kJ/mol (about -1.66 eV/atom), the calculated results in this study are closer to the experimental values. Fig.2 shows the cohesive energies of Al₂Ca, Al₄Ca and Mg₂Ca, indicating that the cohesive energy of Al₂Ca is higher than that of Al₄Ca, and that of Mg₂Ca is only half of Al₂Ca or Al₄Ca. Hence, among the three phases, Al₂Ca phase has the highest structural stability, next Al₄Ca, finally Mg₂Ca.

Fig.2 Cohesive energies (E_coh) of Al₂Ca, Al₄Ca and Mg₂Ca phases

3.4 Gibbs energy

Grounded on the above calculated results, it is found that the cohesive energy of Al₂Ca is close to that of Al₄Ca. In order to compare with the structural stability of Al₂Ca and Al₄Ca, the Gibbs energies of Al₂Ca and Al₄Ca was calculated based on some hypothetical ideas and experimental data.

Based on experiment, the relationship between Gibbs energies and temperature of Al₂Ca and Al₄Ca in the temperature range of 673-903 K can be expressed as [21]

?G(Al_0.67Ca_0.33)=-(10.43±0.15)+(1.89±0.31)×10^-3T

 (3)

?G(Al_0.8Ca_0.2)=-(4.04±0.08)+(0.86±0.16)×10^-3T

 (4)

The results calculated by Eqns.(3) and (4) are shown in Fig.3(a). It is obvious that the Gibbs energies of Al₂Ca are lower than those of Al₄Ca in the temperature range of 673-903 K. The lower the Gibbs energies are, the more stable the structure is. Hence, the structure of Al₂Ca is more stable than that of Al₄Ca. However, the actual work temperature of intermetallic compounds of Mg-Al-Ca alloy is usually below 423 K. It is necessary to discuss the structural stability of Al₂Ca and Al₄Ca in the temperature range of 0-673 K, the results are shown in Fig.3(b).

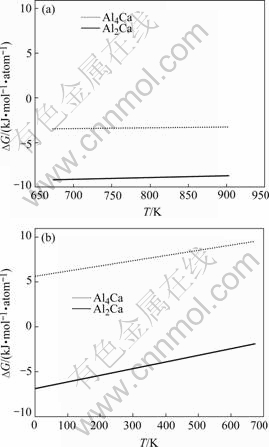
Fig.3 Relationship between Gibbs energies and temperature for Al₂Ca and Al₄Ca at different temperature ranges: (a) 673-903 K; (b) 0-673 K

Thermodynamic data from experiment measure- ment were summarized by OZTURK K et al[21], and the general relationship between the Gibbs energies of Al₂Ca, Al₄Ca and temperature was given by the following expression:

where 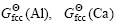are the Gibbs energies per mole of fcc-Al, fcc-Ca, respectively.

In this work, the structural stability of Al₂Ca and Al₄Ca in the temperature range of 0-673 K was investigated by employing Eqns.(5) and (6). Here, it is necessary to replace Eqns.(5) and (6) by Eqns.(7) and (8), which can be expressed as

where  ?G=?H-T?S (?H and ?S mean formation heat and entropy, respectively). In this work, it is supposed that there is no work exchange with environment in the systems of Al₂Ca and Al₄Ca. Moreover, there is also no change for formation heat of Al₂Ca and Al₄Ca. Hence, the following expression can be obtained based on Eqns.(7) and (8):

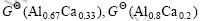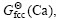
where are the Gibbs energies per mole of Al₂Ca, Al₄Ca, respectively.  and 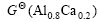denote the total energies of fcc-Al, fcc-Ca, Al_0.67Ca_0.33, Al_0.8Ca_0.2, and their calculated values are 5.519, 96.852, -36.002, -23.801 MJ/(mol? atom), respectively. Based on these calculated data from Eqns.(9) and (10), the relationship between Gibbs energies of Al₂Ca and Al₄Ca and temperature in the range of 0-673 K can be expressed by

?G(Al_0.67Ca_0.33)= -6.87+7.44×10^-3T            (11)

?G(Al_0.8Ca_0.2)=5.63+5.82×10^-3T               (12)

According to Eqns.(11) and (12), it is obvious that the Gibbs energies of Al₂Ca are lower than those of Al₄Ca (see Fig.3(b)), which indicates that the structure of Al₂Ca is more stable than that of Al₄Ca.

3.5 Density of states

Further analysis on the total and partial density of states(DOS) of Mg₂Ca, Al₄Ca and Al₂Ca was performed to study the electronic structure mechanism on improving structural stability. The total and partial DOSs of Al₂Ca, Al₄Ca primitive and Mg₂Ca crystal cell per atom are shown in Figs.4(a), (b) and (c), respectively. It is found that the main bonding peaks between -10.0 and 10.0 eV mainly originate from the contribution of valence electron numbers of Al(s) and Al(p) orbits, while the main bonding peaks between -25 and -20 eV, -45 and -40 eV are the results of the bonding between Ca(p) and Ca(s). The energy range and the contribution of valence electron of Al₄Ca (see Fig.4(b)) are the same as those of Al₂Ca. The main bonding peaks of Mg₂Ca (see Fig.4(c)) are also located at three energy ranges, but the contribution of valence electron is different from that of Al₂Ca and Al₄Ca. The main bonding peaks between -10.0 and 10.0 eV are dominated by the valence electron numbers of Ca(p), Mg(s) and Mg(p) orbits, while the peaks between -25 and -20 eV are caused by the bonding of Ca(p), and there is a contribution of the Ca(s) and Mg(p) to two peaks between -45 and -40 eV, respectively.

Fig.4 DOSs of Al₂Ca primitive cell (a) Al₄Ca primitive cell (b) and Mg₂Ca crystal cell (c) per atom; DOSs of Al₂Ca, Al₄Ca and Mg₂Ca (d) per atom; DOS of Al₂Ca and Al₄Ca (e) per atom

The total density of states (DOS) (see Fig.4(d)) of Al₂Ca, Al₄Ca and Mg₂Ca per atom near the Fermi level were analyzed. It is found that the bonding electron numbers of Mg₂Ca are 1.981 5 between the Fermi level and -10 eV, which is smaller than 2.678 2 of Al₄Ca and 2.798 7 of Al₂Ca, respectively. The smaller the bonding electron numbers are, the weaker the charge interactions are in Refs.[22-23]. Hence, Mg₂Ca phase has the lowest structural stability. Further analysis on the density of states(DOS) (see Fig.3(e)) of primitive cell of Al₄Ca and Al₂Ca per atom was performed in low energy range far below the Fermi level. It is found that the altitude of the main bonding peaks between -25 and -20 eV and between -45 and -40 eV is significantly different. As far as Al₄Ca is concerned, the altitude of the main bonding peaks between -25 and -20 eV is 2.241 2 electronic state/eV, the altitude between -45 and -40 eV is 0.741 2 electronic state/eV, while that of the Al₂Ca is 3.462 0 electronic state/eV, 1.240 8 electronic state/eV, respec- tively. This indicates that the bonding electron numbers of Al₂Ca are more than those of Al₄Ca in these two energy ranges. The more the bonding electron numbers are, the stronger the charge interactions are, and further electron numbers in low energy range far below the Fermi level will lead to more stable structure[22-23]. Hence, the structure of Al₂Ca is more stable than that of Al₄Ca.

4 Conclusions

1) The energetic and electronic structures of intermetallic compounds(Al₂Ca, Al₄Ca and Mg₂Ca) of Mg-Al-Ca alloy were investigated by using a first- principles plane-wave pseudopotential method based on the density functional theory.

2) Al₂Ca phase has the strongest alloying ability and the highest structural stability, next Al₄Ca, finally Mg₂Ca.

3) The increase of the structural stability of Al₂Ca is attributed to an increase in the bonding electron numbers at lower energy level below Fermi level, which mainly originates from the contribution of valence electron numbers of Ca(s) and Ca(p) orbits, while the lowest structural stability of Mg₂Ca is caused by the least bonding electron numbers near Fermi level.

References

[1] MORDIKE B L, EBERT T. Magnesium properties-application- potential [J]. Mater Sci Eng A, 2001, A302(1): 37-45.

[2] LUO A, PEKGULERYUZ M O. Review cast magnesium alloys for elevated temperature application [J]. J Mater Sci, 1994, 29(20): 5259-5271.

[3] MIN X G, DU W W, XIE F, SUN Y S. The melting point of Mg₁₇Al₁₂ phase improved by alloyed with Ca and analysis based on the empirical electron theory(EET) [J]. Chinese Sci Bull, 2002, 47(2): 109-112.

[4] SHAW C, JONES H. Structure and mechanical properties of two Mg-Al-Ca alloys consolidated from atomized power [J]. Mater Sci Tech, 1999, 15(1): 78-83.

[5] SONG H N, YUAN G Y, WANG Q D, ZHU Y P, DING W J. Microstructure and mechanical properties of heat resistant Mg-Zn-Si-Ca alloy [J]. The Chinese Journal of Nonferrous Metal, 2002, 12(5): 956-960.(in Chinese)

[6] NIE J F, MUDDLE B C. Precipitation hardening of Mg-Ca(-Zn) alloys [J]. Scripta Mater, 1997, 37(10): 1475-1481.

[7] MIN X G, SUN Y S, XUE F, DU W W, WU D Y. Analysis of valence electron structures (VES) of intermetallic compounds containing calcium in Mg-Al-based alloys [J]. Mater Chem Phys, 2002, 78(1): 88-93.

[8] ZHOU D W, PENG P, ZHUANG H L, HU Y J, LIU J S. First-principle study on structural stability of Ca alloy Mg₁₇Al₁₂ phase [J]. The Chinese Journal of Nonferrous Metal, 2005, 15(4): 546-551. (in Chinese)

[9] LINDAN P J D, SEGALL M D, PROBERT M J, PICKARD C J, HASNIP P J, CLARK S J, PAYNE M C. First-principles simulation: Ideas, illustrations and the CASTEP code [J]. J Phys: Condens Matter, 2002, 14(11): 2717-2744.

[10] MARLO M, MILMAN V. Density-functional study of bulk and surface properties of titanium nitride using different exchange-correlation functionals [J]. Phys Rev B, 2000, 62(4): 2899-2907.

[11] VANDERBILT D. Soft self-consistent pseudopotentitals in a generalized eigenvalue formalism [J]. Phys Rev B, 1990, 41(11): 7892-7895.

[12] HAMMER B, HANSEN L B, NORKOV J K. Improved adsorption energetics with density-functional theory using revised Perdew- Burke-Ernzerh of functionals [J]. Phys Rev B, 1999, 59(11): 7413-7421.

[13] FRANSCIS G P, PAYNE M C. Finite basis set corrections to total energy pseudopotential calcaulations [J]. J Phys: Condens Matter, 1990, 2(19): 4395-4404.

[14] MONKORST H J, PACK J D. Special points for Brillouin-zone integrations [J]. Phys Rev B, 1976, 13(12): 5188-5192.

[15] HUANG K. Solid Physics [M]. Beijing: Higher Education Press, 1985: 68.

[16] HONG S Y, FU C L. Phase stability and elastic moduli of Cr₂Nb by first-principles calculation [J]. Intermetallics, 1999, 7(1): 5-9.

[17] SAHU B R. Electronic structure and bonding of ultralight LiMg [J]. Mater Sci Eng B, 1997, B49(1): 74-78.

[18] SONG Y, GUO Z X, YANG R, LI D. First principles study of site substitution of ternary elements in NiAl [J]. Acta Mater, 2001, 49(9): 1647-1654.

[19] LI C H, HOE J L, WU P. Empirical correlation between melting temperature and cohesive energy of binary Laves phases [J]. J Phys Chem Solids, 2003, 64(2): 201-212.

[20] ZUBOV V I, TRETIAKOV N P, TEIXEIRA R J N, SANCHEZ O J F. Calculations of the thermal expansion, cohesive energy and thermodynamic stability of a van der Waals crystal-fullerene C₆₀ [J]. Phys Lett A, 1995, 198(1): 223-227.

[21] OZTURK K, CHEN L Q, LIU Z K. Thermodynamic assessment of the Al-Ca binary system using random solution and associate models [J]. J Alloys Comp, 2003, 340(1/2): 199-206.

[22] FU C L, WANG X D, YE Y Y, HO K M. Phase stability, bonding mechanism, and elastic constants of Mo₅Si₃ by first-principles calculation [J]. Intermetallics, 1999, 7(2): 179-184.

[23] NYLEN J, GARCIA F J, MOSEL B D, POTTGEN R, HAUSSEVMANN U. Structural relationships, phase stability and bonding of compounds PdSn_n (n=2,3,4) [J]. Solid State Sci, 2004, 6(1): 147-155.

Foundation item: Project(20020530012) supported by the Doctoral Program Foundation of Ministry of Education of China

Corresponding author: ZHOU Dian-wu; Tel: +86-13017297124; E-mail: ZDWe_mail@yahoo.com.cn

(Edited by CHEN Wei-ping)