J. Cent. South Univ. (2020) 27: 2899-2913

DOI: https://doi.org/10.1007/s11771-020-4517-5

Brazilian disc test study on tensile strength-weakening effect of high pre-loaded red sandstone under dynamic disturbance

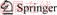
GONG Feng-qiang(宫凤强)^{1, 2, 3}, WU Wu-xing(伍武星)³, ZHANG Le(张乐)³

1. Engineering Research Center of Safety and Protection of Explosion & Impact of Ministry of

Education (ERCSPEIME), Southeast University, Nanjing 211189, China;

2. School of Civil Engineering, Southeast University, Nanjing 211189, China;

3. School of Resources and Safety Engineering, Central South University, Changsha 410083, China

Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020

Abstract: Tensile failure (spalling or slabbing) often occurs on the sidewall of deep tunnel, which is closely related to the coupled stress state of deep rock mass under high pre-static load and dynamic disturbance. To reveal the mechanism of rock tensile failure caused by this coupled stress mode, the Brazilian disc tests were carried on red sandstone under high pre-static load induced by dynamic disturbance. Based on the pure static tensile fracture load of red sandstone specimen, two static load levels (80% and 90% of the pure static tensile fracture load) were selected as the initial high pre-static loading state, and then the dynamic disturbance load was applied until the rock specimen was destroyed. The dynamic disturbance loading mode adopted a sinusoidal wave (sine-wave) load, and the loading wave amplitude was 20% and 10% of the pure static tensile fracture load, respectively. The dynamic disturbance frequencies were set to 1, 10, 20, 30, 40, and 50 Hz. The results show that the tensile failure strength and peak displacement of red sandstone specimens under coupled load actions are lower than those under pure static tensile load, and both parameters decrease significantly with the increase of dynamic disturbance frequency. With the increase of dynamic disturbance frequency, the decrease range of tensile strength of red sandstone increased from 3.3% to 9.4% when the pre-static load level is 80%. While when the pre-static load level is 90%, the decrease range will increase from 7.4% to 11.6%. This weakening effect of tensile strength shows that the deep surrounding rock is more likely to fail under the coupled load actions of pre-static load and dynamic disturbance. In this tensile failure mechanism of the deep surrounding rock, the stress environment of deep sidewall rock determines that the failure mode of rock is a tensile failure, the pre-static load level dominates the tensile failure strength of surrounding rock, and dynamic disturbance promotes the strength-weakening effect and affects the weakening range.

Key words: spalling; deep surrounding rock; strength-weakening effect; pre-static load; dynamic disturbance; tensile failure; Brazilian disc test

Cite this article as: GONG Feng-qiang, WU Wu-xing, ZHANG Le. Brazilian disc test study on tensile strength-weakening effect of high pre-loaded red sandstone under dynamic disturbance [J]. Journal of Central South University, 2020, 27(10): 2899-2913. DOI: https://doi.org/10.1007/s11771-020-4517-5.

1 Introduction

During the excavation of many deep hard-rock tunnels, obvious spalling (or slabbing) failure is often observed at the tunnel sidewall (Figure 1) [1-5]. The spalling is essentially a typical tensile failure phenomenon in deep engineering or laboratory tests [1, 2, 6-15]. For example, HE et al [11] had performed a true triaxial test using cubic sandstone specimens with a circular hole and observed the tension failure of the rock plate on the sidewall. Furthermore, GONG et al [10, 12, 14], LUO et al [6, 8] and WU et al [9] carried out a series of indoor true triaxial tests on circular, rectangular or D-shaped tunnels and observed obvious tensile failure of sidewalls, which is consistent with the tensile failure mode of sidewalls of the deep underground cavern (Figure 1). ZHOU et al [13] conducted a study on the influence of curvature radius of tunnels excavation section on slabbing of the deep tunnel. To study the stress state during the tensile failure of the hard-brittle tunnel sidewalls, researchers have carried out a lot of in-situ tests or laboratory physical tests [16-20]. However, it is difficult to reasonably explain the tensile failure phenomena of tunnel sidewalls based on classical rock statics or dynamics theory developed by shallow mining or excavation. In view of this situation, LI et al [21-23] conducted an exploration of coupled dynamic and static loading mechanics. For example, LI et al [21] proposed that the deep rock was affected by high pre-static stress (gravity or tectonic stress) coupled with dynamic loads produced arising from blasting and impact (Figure 2). GONG et al [22, 23] carried out research on one-dimensional and three-dimensional coupled dynamic and static loading tests.

Figure 1 Tensile fracture failure on deep tunnel sidewall:

The deep tunnel is subjected to static stress before excavation, and is further subjected to dynamic disturbance during the opening process [24-26], and then the tunnel will be under the coupled loading state of “pre-static stress+dynamic disturbance”. Additionally, tensile fracture failure under this stress coupled loading state is one of the main modes of surrounding rock failure in deep underground rock engineering.

It is important to understand the mechanism of rock failure under the coupled static and dynamic loads when dealing with various deep rock engineering problems [21-23, 27]. At present, the determination of tensile failure characteristics is mainly focused on the Brazilian disc test [28-30]. Therefore, the Brazilian disc test was used to design the test plan under dynamic and static coupling loading (Figure 2(b)) in this study.

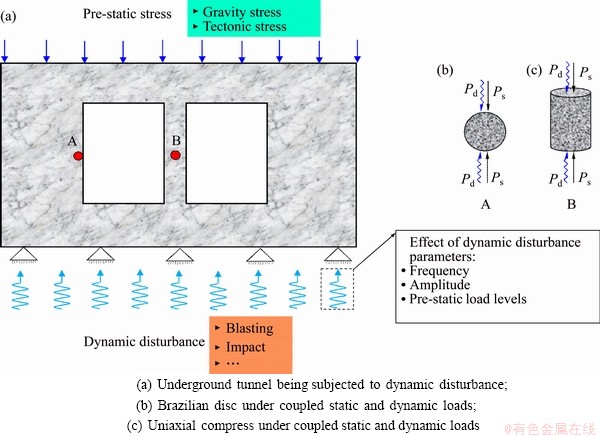
Figure 2 Schematic diagrams of rock stress models at deep level (revised after [21]):(P_s and P_d represent pre-static stress and dynamic disturbance, respectively)

The Brazilian disc test is one of the most effective methods to measure the indirect tensile fracture strength of rock. In existing studies, Brazilian disc tests were mainly based on theoretical, experimental, and numerical studies of specific rock types under static or quasistatic loads [31-36]. As research progressed, the Brazilian disc test was extended to the dynamic tensile fracture strength test using the Split Hopkinson Pressure Bar (SHPB) device [30, 37-39]. In these tests, rock specimens were stress-free before being subjected to static or dynamic loads and they cannot be applied to the coupled static and dynamic loading state. In this paper, the red sandstone specimens were used in Brazilian disc test at different pre-static load levels under dynamic disturbance on an MTS landmark electrohydraulic servo test machine. The variation in the tensile fracture strength of red sandstone subjected to dynamic disturbance under different pre-static load levels was analyzed through experiments. The conclusion of strength-weakening effect was obtained, and related mechanism and characteristics were discussed and analyzed, combined with the tensile fracture failure mode of the sidewall of the deep tunnel on-site to verify the validity of the conclusion.

2 Specimen preparation and test plan

2.1 Specimen preparation

The red sandstone was selected from the same fine sandstone plate with good lithology in Junan County, Linyi City, Shandong Province, China. Red sandstone was machined to the cylinder specimen with diameter of 50 mm (d=50 mm) and width of 25 mm (W=25 mm). The average natural air-dry density of the specimen was 2426.04 kg/m³ (coefficient of variation 0.014), and the average longitudinal wave velocity was 3227.04 m/s (coefficient of variation 0.022), which indicates the red sandstone rock material with good homogeneity. Moreover, the uniaxial compression of the specimen was measured and the average uniaxial compressive strength of the specimen was 100 MPa, which is a typical hard rock. To ensure the accuracy of the test, the surface of the specimens are carefully polished so that the errors of parallelism and perpendicularity at the both ends of the specimens are less than 0.02 mm, which meets the requirements of the ISRM (International Rock Mechanics Society) [31].

2.2 Test equipment

All Brazilian disc tests under the pure static tensile fracture load or “high pre-static load + dynamic disturbance” in this study were performed on the MTS Landmark electrohydraulic servo tester with spacer loading. The MTS Landmark electrohydraulic servo tester is shown in Figures 3(a) and (b), and the loading diagram for the red sandstone is shown in Figure 3(c). The maximum load of the test machine is ±100 kN, the maximum displacement range is ±100 mm, and the dynamic disturbance frequency loading range is 0.01-100 Hz, which meets all accuracy and range requirements of this test.

2.3 Test plan

The Brazilian disc test under “high pre-static load+dynamic disturbance” was divided into two parts. (I) The pure static load Brazilian disc test performed under the continuously increasing load over the entire loading duration. The loading rate during this part of the experiment was 10 kN/min, the purpose of which was to determine the pure static load of the specimen. (II) The pre-static load levels of the two groups were set at 80% and 90% of their pure static load value. Moreover, the dynamic disturbance was sinusoidal wave loading with the amplitude of 10% and 20% of the pure static load. Under the conditions of the corresponding constant dynamic disturbance amplitudes above, the tensile fracture tests were performed on specimens with different dynamic disturbance frequencies (1, 10, 20, 30, 40 and 50 Hz). The purpose of setting this series of disturbance frequency is to better explore the change law of tensile fracture strength of specimen under different disturbance frequencies.

It is noteworthy that in the “high pre-static load+dynamic disturbance” test, the loading rate of the pre-static segment was 10 kN/min, which was consistent with the pure static loading condition. When the set corresponding pre-static level was reached, the sine-wave loading was applied, and then the sine-wave loading was performed according to the test requirements. Figure 4 shows the loading path for the same pre-static load with different dynamic disturbance frequencies (Figure 4(a)) and different pre-static loads at the same dynamic disturbance frequency (Figure 4(b)), where ΔF=2×dynamic disturbance amplitude, and T=1/F (F is the dynamic disturbance frequency). For better controlling the load application process, all dynamic disturbance tests adopted segment loading, i.e., the pre-static load stage was loaded at a rate was 10 kN/min (load-displacement control method) and the dynamic disturbance stage was the displacement control mode with the loading rate of 1 mm/min (displacement-load control method).

3 Test results and analysis

3.1 Test results for pure static load

Before the test, the basic parameters of the red sandstone specimens were measured using an acoustic wave tester, weighing instrument, and vernier caliper, and the specimens with larger differences were removed. After the test, the load-displacement curves of the three specimens under pure static load were drawn, as shown in Figure 5.

Figure 3 Loading device diagram:

Figure 4 Schematic diagrams of test loading path and loading model:

In Figure 5, the laws of the three curves are roughly the same, and the curve is roughly divided into two parts. Similar to the uniaxial compression test, the AB segment is the deformation concave segment, and the BC segment is the elastic deformation segment. In the AB segment, the specimens enter the nonlinear deformation section. In the BC segment, the specimens enter the elastic deformation stage and exhibit approximate elastic deformation. Up to the failure point C, the crack penetrates along the center at this time, the entire specimen is unstable and destroyed, and the load level suddenly drops, showing obvious brittleness.

Figure 5 Brazilian disc splitting load-displacement curve under pure static load

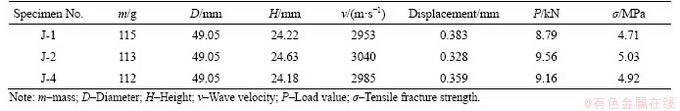
The specimen measurement data and pure static load test results are listed in Table 1. It can be seen that the maximum load values under pure static load test of specimens J-1, J-2, and J-4 were 8.79, 9.56 and 9.16 kN. Therefore, the average value of the pure static load of the specimen was 9.17 kN. The rock tensile strength was calculated using Eq. (1).

σ_bt=2P_b/(πDH)                           (1)

where σ_bt is the indirect tensile strength of rock, MPa; P_b is the maximum load of indirect tensile, N; D is the diameter of the test piece, mm; and H is the height of the specimen, mm.

Substituting the maximum load into Eq. (1), the pure static tensile fracture strength values of the three specimens were 4.71, 5.03 and 4.92 MPa, respectively, and the coefficient of variation was 0.027. The above results indicated that the peak load or tensile fracture strength of the three specimens are relatively close, indicating that the homogeneity of the specimens is good. Therefore, the average value of pure static tensile fracture strength of the specimen was 4.87 MPa and the average value of peak displacement of the specimen was 0.357 mm.

Table 1 Results of pure-static-load Brazilian disc test

3.2 Test results for “high pre-static load+ dynamic disturbance”

The specimen parameters and test results under different “high pre-static load+dynamic disturbance” loading conditions are listed in Table 2. Figure 6 shows the load-displacement curve of the Brazilian disc test under different dynamic disturbance loading conditions (Point D was the pre-static load level, and the DE segment was the dynamic disturbance process).

As shown, the curve before point D is consistent with the pure static load curves, which can be roughly divided into two segments. After the pre-static load level, the curve between D and E is subjected to repeated loading and unloading by high-frequency dynamic disturbance, the load- displacement curve becomes particularly dense, and the final specimen is destroyed by the dynamic disturbance. Then, the tensile fracture strength under the condition of “high pre-static load+ dynamic disturbance” is calculated by using the load value at failure point E. To accurately determine the location of E at the failure point, the last two disturbance processes are magnified, as shown in Figure 6.

3.3 Failure pattern of tension fracture of rock specimens

The failure mode of specimens can be used as an important index to reveal the failure mechanism of specimens, so it is necessary to analyze the failure mode. After the test, all specimens were fractured into two halves along the centerline, and the typical cases of “high pre-static load + dynamic disturbance” test failure were selected, as shown in Figure 7. As shown, V-shaped grooved failure zone occurs at both ends of the specimen owing to the extrusion of the gasket and the specimen basically fracture along the centerline. This failure mode is consistent with the conventional the splitting failure mode, which indicates that the failure mode of the specimen is a typical tensile fracture failure mode. From the perspective of the specimen tensile fracture mode, the results also illustrate the feasibility of “high pre-static load + dynamic disturbance” for determining the indirect tensile fracture strength of rock under dynamic and static coupled loading conditions.

3.4 Influence of hysteretic loops between DE

To investigate the effect of pre-static load levels and dynamic disturbance frequencies on hysteresis loops between DE, the slope between the upper and lower vertices of the hysteresis loop between DE is defined as a parameter reflecting the mechanical response of the rock specimen under high-frequency disturbance, the calculation model diagram is shown in Figure 8. Because the specimens were multiple loading and unloading during the dynamic disturbance process (Figure 6), the DE segment becomes dense; and the slope value of the single hysteresis loop is difficult to be convincing, but it is too cumbersome to calculate the slopes of all hysteresis loops. For carrying out statistical analysis of the slope of the DE segment more accurately and effectively, the slope values of the previous three cycles, the middle three cycles, and the latter three cycles were taken. Taking specimen 26 as an example (Table 2), the total number of cycles was 83. Table 3 lists the hysteresis loop slope values of specimen 26 in the early, middle, and late stages. To analyze the variation law between the slope values of the hysteresis loops in different periods, the slope values of the hysteresis loops of the three periods are shown in Figure 9.

Table 2 Test results for “high pre-static load + dynamic disturbance”

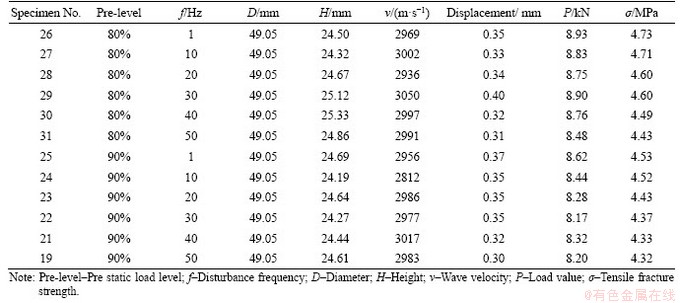
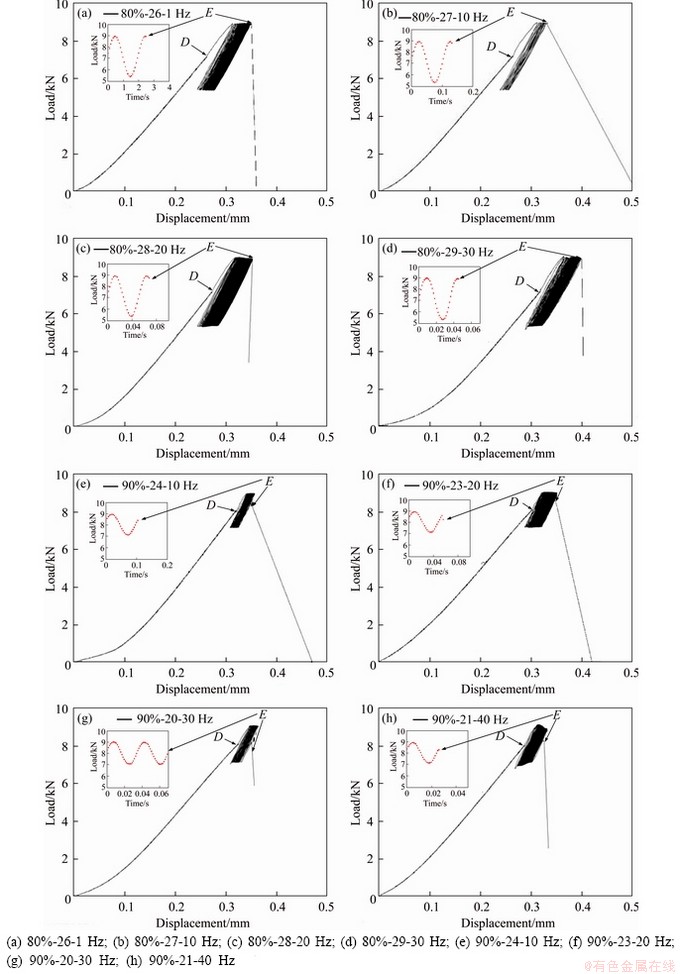
Figure 6 Load-displacement curve of Brazilian disc test with different pre-loads under dynamic disturbance:(80%-26-1 Hz means: 80%-80% of the pure static tensile fracture load, 26-specimen number, 1 Hz-dynamic disturbance frequency)

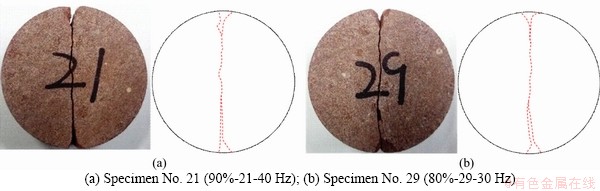
Figure 7 Splitting failure mode of specimen under “high pre-static load + dynamic disturbance” loading condition:

Figure 8 Hysteresis loop loading slope calculation model diagram

As shown in Figure 9, although the slope value of the hysteresis loop fluctuates up and down, the slope value of the hysteresis loop generally increases as the number of disturbance cycles increases. To find the slope value which can reflect the hysteresis loop in the DE interval of the specimen load-displacement curve, the slope values of the nine hysteresis loops are taken as the mechanical properties of the specimen under dynamic disturbance.

Table 3 Slope distribution of hysteresis loops in early, middle, and late stages of specimen 26

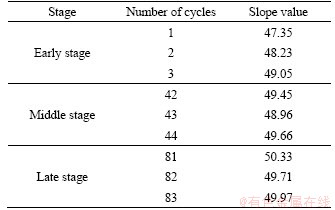
Figure 9 Change in slope values of the hysteresis loop in the early, middle, and late periods of specimen No. 26 (80%-26-1 Hz)

According to the prevision method, the slope of the hysteresis loop between DE of all specimens under different loading conditions was counted, and the average value of nine hysteresis loops of all specimens is shown in Figure 10. As shown, when different dynamic disturbance frequencies were applied at the same pre-static load level, the slope value of the specimen in the dynamic- disturbance- section DE did not change much, i.e., there is no correlation between the dynamic disturbance frequency and the hysteretic loop slope value. Therefore, the effect of increasing the slope value of the DE segment by changing the dynamic disturbance frequency under the same pre-static load is not obvious. However, by increasing the pre-static load levels, the slope value of the DE segment can be significantly improved. For example, the slope value at 90% pre-static strength was higher than the slope value at 80% pure-static load under the same conditions (except at 40 Hz, this may be because at a higher pre-static level, the disturbance amplitude is small, resulting in secondary crack development not very sufficient, and the displacement in one hysteresis loop is small, resulting in a large hysteresis loop slope value).

Figure 10 Variation in slope value of hysteresis loop with disturbance frequency

3.5 Tensile fracture strength and peak displacement of rock specimen

Figure 11 shows the variation in the tensile fracture strength with the dynamic disturbance frequency at the pre-static load levels, and these change amplitudes of tensile fracture strength are shown in Table 4. In Figure 11, the tensile strength of the specimens at the pre-static load level of 80% or 90% was lower than that under pure static load, and the tensile strength of the specimens at the pre-static load level of 90% is lower than that at the pre-static load level of 80%. The higher the pre-static load level is, the lower the tensile strength of the specimen is, and the more obvious the strength-weakening effect, indicating that the pre-static load level is the decisive factor in determining the tensile strength of the specimen. It also can be seen that the tensile fracture strength under the condition of “high pre-static load + dynamic disturbance” gradually decreases with an increase in the dynamic disturbance frequency, also showing an obvious strength-weakening effect. When the pre-static load level is 80%, with the increase of the dynamic disturbance frequency, the decrease range of tensile fracture strength of specimen increased from 3.3% to 9.4%. While when the pre-static load level is 90%, the decrease range will increase from 7.4% to 11.6%. Moreover, the tensile fracture strength and the disturbance frequency under the pre static load level of 80% and 90% follow the linear function of one variable relationship, and the coefficient of determination R² values were 0.9506 and 0.9198 (Figure 11). The fracture tensile strength decreases with the increase of disturbance frequency and is always smaller than the pure static tensile fracture strength. These results indicate that if the frequency of dynamic disturbances is the same, the higher the pre-static load level, the more significant the weakening effect of tensile fracture strength and the reduction of tensile fracture strength is the greatest under high-frequency disturbance.

Figure 11 Variation in tensile fracture strength with disturbance frequency under 80% and 90% pre-static loading conditions

Table 4 Change range of tensile fracture strength

Moreover, the relationship between the peak displacement of specimen and disturbance frequency is sorted, as shown in Figure 12. As Although the peak displacement of specimen is discrete, the overall law tends to decrease with the increase of disturbance frequency compared with the pure static load peak displacement. Compared with the pure static load peak displacement, the magnitude of the reduction in the peak displacement at the lower dynamic disturbance frequency is not significant.

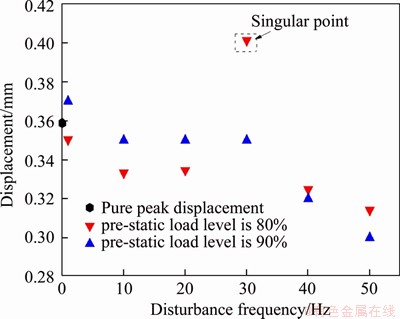
Figure 12 Variation in peak displacement of red sandstone specimens with disturbance frequency under pre-static load conditions

In summary, the variation of rock tensile fracture strength and peak displacement of specimen with the dynamic disturbance frequency under the pre-static load level were analyzed. The test results show that under the condition of “high pre-static load + dynamic disturbance”, the obvious weakening effect of both the tensile fracture strength and peak displacement of specimen indicates that the rock is more prone to failure, which can provide a certain theoretical basis for underground engineering such as deep tunnel excavation and rock-breaking.

4 Discussion

4.1 Strength-weakening effect analysis

In this experiment, the weakening effect of the tensile strength under the disturbance of deep rock excavation was confirmed, and the related mechanism and characteristics were discussed in detail as follows.

The tensile fracture strength-weakening effect of rock shows that the deep rock is more likely to fail under the coupled action of pre-static load and dynamic disturbance. Especially when the pre-static load level and the frequency of dynamic disturbances were high, the peak load reduction of the specimen was larger, showing a significant strength-weakening effect. In the tensile failure mechanism, the deep stress environment of the tunnel sidewall controls the surrounding rock failure mode as a tensile failure, the pre-static load level prevails the tensile failure strength of surrounding rock, and dynamic disturbance encourages the strength-weakening effect and influences the weakening range. The failure mechanism of this strength weakening-effect can be explained to a certain extent, why the tensile strength of deep rocks in actual engineering is less than the tensile strength obtained in laboratory tests. In fact, the strength-weakening effect of deep rock under high pre-static load is not only induced by dynamic disturbance, but also influenced by unloading. Our previous studies have shown that there is a significant strength-weakening effect after the unloading of three-dimensional high-stress rock. And the higher the unloading rate is, the more obvious the strength-weakening effect is [20]. The study also found that when the confining pressure is high, unloading will induce tensile cracks parallel to the loading direction on the free surface of the specimen [20]. Therefore, due to the effect of excavation unloading, tensile cracks parallel to the loading direction appear in the surrounding rocks of the deep tunnel, and then the surrounding rocks may be damaged by dynamic disturbance. It is also worth studying whether the strength of surrounding rock is weakened.

4.2 Comparison of fracture strength law with SCB test

To investigate the fracture characteristics of hard brittle surrounding rock of the tunnel sidewall with pre-crack under the coupled loading state of “pre-static stress + dynamic disturbance”, a series of semi-circular bending (SCB) tests were carried out on red sandstone (Figures 13 and 14) [40, 41].

In the SCB test, the loading mode and the test equipment were consistent with the Brazilian disc test in this paper. A lot of fracture tests have been carried out on SCB red sandstone specimens [40]. Four pre-static load levels (60%, 70%, 80% and 90% of the pure static fracture load) were selected and the dynamic disturbance loading were designed as sine-wave form. The disturbance amplitudes were 40%, 30%, 20% and 10% of the pure static fracture load correspondingly, and the dynamic disturbance frequencies were 1, 10, 20, 30, 40 and 50 Hz, respectively. The SCB test of red sandstone under the condition of “pre-static load + dynamic disturbance” is shown in Figure 13. The loading diagram and the red sandstone specimens are shown in Figures 13(a) and (b), and the stress loading mode was consistent with the Brazilian disc test in this paper. After the test, Figures 13(c) shows one of typical fracture load-displacement curves (90%-40 Hz), and Figure 13(d) shows the variation between the fracture toughness value and the disturbance frequency at different pre-static load levels. The test results indicated that the fracture toughness of red sandstone specimen decreases with the increase of disturbance frequency under different pre-static load levels, and shows an obvious fracture toughness weakening effect. For example, at the 90% pre-static level, the fracture toughness decreased from 5.919 MPa·m^1/2 under pure static load to 5.672 MPa·m^1/2 at 1 Hz, for a decrease of 4.17%. When the disturbance frequency increased to 50 Hz, the fracture toughness decreased to 5.234 MPa·m^1/2, for a decrease of 11.57%. These results indicate that under the action of “pre-static load + dynamic disturbance”, there will be the obvious weakening effect of fracture toughness for rock materials.

Figure 13 Red sandstone SCB test under “pre-static load+dynamic disturbance” [40]:

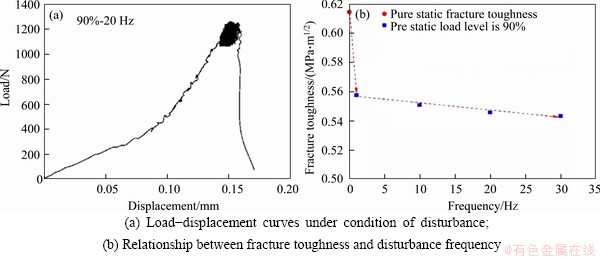
Figure 14 SCB marble specimens test results [41]:

Moreover, the same SCB tests were carried out on marble materials by GONG et al [41]. When the pre-static load level was set to 90% of the corresponding the pure static fracture load, the pure static fracture load value of the disturbance amplitude was 10% and the dynamic disturbance frequency was 1, 10, 20 and 30 Hz, respectively. The relationship between the fracture toughness and the disturbance frequency of the test specimen is shown in Figure 14.

The average fracture toughness of marble specimens under pure static load test is 6.147 MPa·m^1/2, and the average fracture toughness at disturbance frequency of 1 Hz is 5.573 MPa·m^1/2, which decreases by 9.3%. With the increase in the disturbance frequency, the fracture toughness of marble specimens decreased gradually, and finally decreased by 11.7% at 30 Hz. The overall fracture toughness was significantly reduced after “pre-static load + dynamic disturbance”.

The above test results show that, under the coupled action of pre-static load and dynamic disturbance, the tensile fracture strength will decrease with the increase of pre-static load and disturbance frequency, regardless of whether pre-cracks in the hard rock are parallel to the loading direction. That is, in other words, under the action of high static load, dynamic disturbance induces the weakening effect of rock tensile strength. Moreover, this effectively explains the cause of the tensile fracture failure at the sidewall of the deep tunnel. After the deep tunnel is excavated, the surrounding rock is in the state of high static stress compression at first, and then may be disturbed by dynamic disturbance (such as blasting, drilling, etc.). This coupled stress state will weaken the tensile fracture strength of the sidewall rock and further causes the sidewall to be easily damaged.

5 Conclusions

In this paper, the strength-weakening effect of tensile failure of high pre-static loaded red sandstone under dynamic disturbance is studied experimentally. The main conclusions are as follows:

1) When red sandstone specimen is under the coupled action of pre-static load and dynamic disturbance, the higher the pre-static load level is, the lower the tensile strength of the specimen is, and the more obvious the strength-weakening effect.

2) Compared with the pure static load test, the tensile fracture strength of red sandstone specimen under the condition of “high pre-static load + dynamic disturbance” was significantly reduced and showed a strength-weakening trend with an increase in the dynamic disturbance frequency.

3) The peak displacement of red sandstone specimen under the condition of “pre-static load + dynamic disturbance” was relatively small than that of pure static loading, and the overall appearance tended to decrease with an increase in the dynamic disturbance frequency.

(4) The mechanism of tensile strength- weakening effect of deep surrounding rock is summarized. The stress environment of deep surrounding rock determines the failure mode of surrounding rock is the tensile failure, and the pre-static load plays a leading role in the weakening of the tensile failure strength, and the dynamic disturbance induces further weakening of the strength and affects its strength weakening degree.

Contributors

GONG Feng-qiang provided the idea of the study, developed the overarching research goal, and led the research activity planning and execution. GONG Feng-qiang also made great contribution to the improvement of manuscript after the initial draft finished. WU Wu-xing conducted the experiments, analyzed the test data, and wrote the initial draft of the manuscript. ZHANG Le offered some valuable suggestions for the contents of the manuscript and analyzed the test data. All authors replied to reviewers’ comments and revised the final version.

Conflict of interest

GONG Feng-qiang, WU Wu-xing and ZHANG Le declare that they have no conflict of interest.

References

[1] JIANG Quan, FENG Xia-ting, FAN Yi-lin, FAN Qi-xiang, LIU Guo-feng, PEI Shu-feng. In situ experimental investigation of basalt spalling in a large underground powerhouse cavern [J]. Tunnelling and Underground Space Technology, 2017, 68(10): 82-94. DOI: 10.1016/j.tust.2017. 05.020.

[2] FENG Xia-ting, XU Hong, QIU Shi-li, LI, Shao-jun, YANG Cheng-xiang, GUO Hao-sen, CHENG Yuan, GAO Yao-hui. In situ observation of rock spalling in the deep tunnels of the China Jinping underground laboratory (2400 m depth) [J]. Rock Mechanics and Rock Engineering, 2018, 51: 1193-1213. DOI: 10.1007/s00603-017-1387-8.

[3] HOU Zhe-sheng, GONG Qiu-ming, SUN Zhou-Heng. Primary failure types and their failure mechanisms of deep buried and intact marble at Jinping II Hydropower station [J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 30(4): 727-732. DOI: 10.1631/jzus.B1000185.

[4] DOWDING C H, ANDERSSON C A. Potential for rock bursting and slabbing in deep caverns [J]. Engineering Geology, 1986, 22(3): 265-279. DOI: 10.1016/0013- 7952(86)90028-1.

[5] DIEDERICHS M S. The 2003 Canadian geotechnical colloquium: Mechanistic interpretation and practical application of damage and spalling prediction criteria for deep tunnelling [J]. Canadian Geotechnical Journal, 2007, 44(9): 1082-1116. DOI: 10.1139/T07-033.

[6] LUO Yong, GONG Feng-qiang, LIU Dong-qiao, WANG Shan-yong, SI Xue-feng. Experimental simulation analysis of the process and failure characteristics of spalling in D-shaped tunnels under true-triaxial loading conditions [J]. Tunnelling and Underground Space Technology, 2019, 90: 42-61. DOI: 10.1016/j.tust.2019.04.020.

[7] CAI Ming, KAISER P K, Rockburst support reference book-Volume I: Rockburst phenomenon and support characteristics [M]. Ontario: Ming Innovation, Laurentian University Sudbury, 2018: 70.

[8] LUO Yong, GONG Feng-qiang, LI Xi-bing, WANG Shan-yong. Experimental simulation investigation of the influence of depth on spalling characteristics in circular hard rock tunnel [J]. Jouranl Central South University, 2020, 27(3): 891-910. DOI: 10.1007/s11771-020-4339-5.

[9] WU Wu-xing, GONG Feng-qiang, YANG, Wei-min, Experimental simulation study of spalling in deep rectangular tunnel with plastic fine grain marble [J]. Tunnelling and Underground Space Technology, 2020, 98, 103319. DOI: 10.1016/j.tust.2020.103319.

[10] GONG Feng-qiang, LUO Yong, LI Xi-bing, SI Xue-feng, TAO Ming. Experimental simulation investigation on rockburst induced by spalling failure in deep circular tunnels [J]. Tunnelling and Underground Space Technology, 2018, 81: 413-427. DOI: 10.1016/j.tust.2018.07.035.

[11] HE Man-chao, LIU Dong-qiao, GONG Wei-li, WANG Cheng-chao, KONG Jie, DU Shuai, ZHANG Shen. Development of a testing system for impact rockbursts [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(9): 1729-1739. DOI: 10.13722/j.cnki.jrme.2014.09.001.

[12] GONG Feng-qiang, WU Wu-xing, LI Tian-bin, SI Xue-feng. Experimental simulation and investigation of spalling failure of rectangular tunnel under different three-dimensional stress states [J]. International Journal of Rock Mechanics and Mining Sciences, 2019, 122: 104081. DOI: 10.1016/ j.ijrmms.2019.104081.

[13] ZHOU Hui, LU Jing-jing, HU Shan-chao, ZHANG Chuan-qing, XU Rong-chao, MENG Fan-zhen. Influence of curvature radius of tunnels excavation section on slabbing of hard brittle rockmass under high stress [J]. Rock and Soil Mechanics, 2016, 31(1): 140-146. DOI: 10.16285/j.rsm. 2016.01.017.

[14] GONG Feng-qiang, WU Wu-xing, LI Tian-bin. Simulation test of spalling failure of surrounding rock in rectangular tunnels with different height-to-width ratios [J]. Bulletin of Engineering Geology and the Environment, 2020, 79: 3207-3219. DOI: /10.1007/s10064-020-01734-w.

[15] MARTIN C D, CHRISTIANSSON R. Estimating the potential for spalling around a deep nuclear waste repository in crystalline rock [J]. International Journal of Rock Mechanics and Mining Sciences, 2009, 46(2): 219-228. DOI: 10.1016/j.ijrmms.2008.03.001.

[16] LI Xi-bing. Rock dynamics fundamentals and applications [M]. Beijing: Science Press, 2014. (in Chinese)

[17] ANDERSSON J C, MARTIN C D, STILLE H. The Aspo pillar stability experiment: Part II-rock mass response to coupled excavation-induced and thermal-induced stresses [J]. International Journal of Rock Mechanics and Mining Sciences, 2009, 46(5): 879-895. DOI: 10.1016/j.ijrmms. 2009.03.002.

[18] CAI Ming, KAISER P K. In-situ rock spalling strength near excavation boundaries [J]. Rock Mechanics and Rock Engineering, 2014, 47: 659-675. DOI: 10.1007/s00603- 013-0437-0.

[19] LI Xi-bing, GONG Feng-qiang, TAO Ming, DONG Long-jun, DU Kun, MA Chun-de, ZHOU Zi-long, YIN Tu-bing. Failure mechanism and coupled static-dynamic loading theory in deep hard rock mining: A review [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2017, 9(4): 767-782. DOI: 10.1016/j.jrmge.2017.04.004.

[20] SI Xue-feng, GONG Feng-qiang. Strength-weakening effect and shear-tension failure mode transformation mechanism of rockburst for fine-grained granite under triaxial unloading compression conditions [J]. International Journal of Rock Mechanics and Mining Sciences, 2020, 131: 104347. DOI: 10.1016/j.ijrmms.2020.104347.

[21] LI Xi-bing, ZHOU Zi-long, LOK T S, HONG Liang, YIN Tu-bing. Innovative testing technique of rock subjected to coupled static and dynamic loads [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(5): 739-748. DOI: 10.1016/j.ijrmms.2007.08.013.

[22] GONG Feng-qiang, LI Xi-bing, LIU Xi-ling, ZHAO J. Experimental study of dynamic characteristics of sandstone under one-dimensional coupled static and dynamic loads [J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29(10): 2076-2085.

[23] GONG Feng-qiang, LI Xi-bing, LIU Xi-ling. Preliminary experimental study of characteristics of rock subjected to 3D coupled static and dynamic loads [J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 30(6): 1179-1190.

[24] ORTLEPP W D. The behaviour of tunnels at great depth under large static and dynamic pressures [J]. Tunnelling and Underground Space Technology, 2001, 16(1): 41-48. DOI: 10.1016/S0886-7798(01)00029-3.

[25] ZHANG Q B, ZHAO J. A review of dynamic experimental techniques and mechanical behaviour of rock materials [J]. Rock Mechanics and Rock Engineering, 2014, 47(4): 1411-1478. DOI: 10.1007/s00603-013-0463-y.

[26] MITELMAN A, ELMO D. Analysis of tunnel support design to withstand spalling induced by blasting [J]. Tunnelling and Underground Space Technology, 2016, 51(6): 354-361. DOI: 10.1016/j.tust.2015.10.006

[27] LI Xing, LI Xiao-feng, ZHANG Qian-bing, ZHAO Jian. A numerical study of spalling and related rockburst under dynamic disturbance using a particle-based numerical manifold method (PNMM) [J]. Tunnelling and Underground Space Technology, 2018, 81: 438-449. DOI: 10.1016/ j.tust.2018.08.026.

[28] GONG Feng-qiang, ZHAO Gao-feng. Dynamic indirect tensile strength of sandstone under different loading rates [J]. Rock Mechanics and Rock Engineering, 2014, 47(6): 2271-2278. DOI: 10.1007/s00603-013-0503-7.

[29] LI Di-yuan, WONG L N Y. The Brazilian disc test for rock mechanics applications: Review and new insights [J]. Rock Mechanics and Rock Engineering, 2013, 46(2): 269-287. DOI: 10.1007/s00603-012-0257-7

[30] GONG Feng-qiang, HU Jian. Energy dissipation characteristic of red sandstone in the dynamic Brazilian disc test with SHPB setup [J]. Advances in Civil Engineering, 2020(5): 1-10. DOI: 10.1155/2020/7160937.

[31] ISRM. Suggested methods for determining tensile strength of rock materials [J]. International Journal of Rock Mechanics and Mining Sciences, 1978, 15(3): 99-103. DOI: 10.1016/0148-9062(78)90003-7.

[32] D2936–08. Standard test method for direct tensile strength of intact rock core specimens [S]. Annual Book of ASTM Standards. ASTM International, West Conshohocken.

[33] D3967–08. 2008b. Standard test method for splitting tensile strength of intact rock core specimens [S]. Annual Book of ASTM Standards. ASTM International, West Conshohocken.

[34] CHEN Chao-shi, PAN E, AMADEI B. Determination of deformability and tensile strength of anisotropic rock using Brazilian tests [J]. International Journal of Rock Mechanics and Mining Sciences, 1998, 35(1): 43-61. DOI: 10.1016/　S0148-9062(97)00329-X.

[35] ERARSLAN N, WILLIAMS D J. Experimental, numerical and analytical studies on tensile strength of rocks　[J]. International Journal of Rock Mechanics and Mining Sciences, 2012, 49(1): 21-30. DOI: 10.1016/j.ijrmms. 2011.11.007.

[36] MARKIDES C F, KOURKOULIS S K. Naturally accepted boundary conditions for the Brazilian disc test and the corresponding stress field [J]. Rock Mechanics and Rock Engineering, 2013, 46(5): 959-980. DOI: 10.1007/s00603- 012-0351-x.

[37] ZHU W C, BAI Y, LI X B, NIU L L, Numerical simulation on rock failure under combined static and dynamic loading during SHPB tests [J]. International Journal of Impact Engineering, 2012, 49: 142-157. DOI: 10.1016/j.ijimpeng. 2012.04.002.

[38] GONG Feng-qiang, ZHANG Le, WANG Shan-yong. Loading rate effect of rock material with the direct tensile and three Brazilian disc tests [J]. Advances in Civil Engineering, 2019(2): 1-8. DOI: 10.1155/2019/6260351.

[39] GONG Feng-qiang, LI Xi-bing, DONG Long-jun. Algorithm to estimate tensile modulus of rock in disk impact splitting test [J]. Chinese Journal of Rock Mechanics and Engineering, 2013, 32(4): 705-713. DOI: 10.3969/j.issn.1000- 6915.2013.04.008.

[40] GONG Feng-qiang, ZHANG Le, LI Xi-bing, LUO Yong. Experimental study on fracture behaviors of hard rock under dynamic disturbance with different pre-static loads [J]. Chinese Journal of Rock Mechanics and Engineering, 2017, 36(8): 1841-1854. DOI: 10.13722/j.cnki.jrme.2016.1439.

[41] GONG Feng-qiang, LU Dao-hui, LI Xi-bing, RAO Qiu-hua, FU Zhen-tao. Toughness increasing or decreasing effect of hard rock fracture with pre-static loading under dynamic disturbance [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(9): 1905-1915. DOI: 10.13722/j.cnki. jrme.2014.09.022.

(Edited by HE Yun-bin)

中文导读

动力扰动下高预静载红砂岩抗拉强度弱化效应的巴西劈裂试验研究

摘要：深埋隧道侧壁围岩经常出现张拉破坏(板裂或层裂)，这与深部岩体在高预静载和动力扰动下的组合受力状态密切相关。为了揭示这种组合应力模式引发岩石张拉破坏的作用机理，对高预静载红砂岩试样进行了动力扰动作用下的巴西圆盘试验。以红砂岩试样纯静态拉伸断裂载荷为基础，选取两个预静载水平(80%和90%的纯静态张拉断裂载荷)作为初始高预静载水平，之后施加动态扰动载荷直至试样发生破坏。动态扰动方式采用正弦波载荷加载，加载波幅值分别为纯静态张拉断裂载荷的20%和10%。动态扰动频率设置为1，10，20，30，40和50 Hz。结果表明，红砂岩试样在组合作用下的抗拉破坏强度和峰值位移均低于纯静态张拉载荷下的抗拉破坏强度和峰值位移，而且这两个参数随着扰动频率的增加而显著降低。当预静载水平为80%时，红砂岩的抗拉强度的降低幅度随着动态扰动频率的增加而从3.3%增加到9.4%。当预静载水平为90%时，降低幅度由7.4%增加到11.6%。这种抗拉强度的弱化效应，表明深部围岩在预静载和动力扰动组合作用下更容易发生破坏。在破坏机理方面，深部侧壁围岩的受力环境决定了岩石破坏模式为拉伸破坏，预静载水平主导着围岩的拉伸破坏强度，动力扰动则对强度弱化有促进作用并且影响强度弱化的幅度。

关键词：板裂；深部围岩；强度弱化效应；预静载；动态扰动；张拉破坏；巴西圆盘试验

Foundation item: Projects(42077244, 41877272, 41472269) supported by the National Natural Science Foundation of China; Project(2242020R10023) supported by the Fundamental Research Funds for the Central Universities of Southeast University, China

Received date: 2020-06-12; Accepted date: 2020-09-06

Corresponding author: GONG Feng-qiang, PhD, Professor; Tel: +86-18175973819; E-mail: fengqiangg@126.com; ORCID: https://orcid. org/0000-0002-2040-4294