Trans. Nonferrous Met. Soc. China 23(2013) 749-755
Evaluation of varying ductile fracture criterion for 7075 aluminum alloy
Guo-zheng QUAN, Feng-biao WANG, Ying-ying LIU, Yu SHI, Jie ZHOU
School of Materials Science and Engineering, Chongqing University, Chongqing 400044, China
Received 21 May 2012; accepted 1 November 2012
Abstract: As one of the principal failures, ductile fracturing restricts metal forming process. Cockcroft-Latham type fracture criterion is suited for ductile fracture in bulk metal-forming simulation. Finding a way to evaluate the ductile fracture criterion (DFC) and identify the relationship between DFC and deformation conditions for a strain-softening material, 7075 aluminum alloy; however, it is a non-trivial issue that still needs to be addressed in a greater depth. An innovative approach is brought forth that the compression tests and numerical simulations provide mutual support to evaluate the ductile damage cumulating process and determine the DFC diagram. One of the results shows that for a fixed temperature, the maximum cumulated damage decreases regularly with increasing strain rate. The most important result shows that DFC of 7075 aluminum alloy at temperatures of 573-723 K and strain rates of 0.01-10 s-1 is not a constant but a change in a range of 0.255-0.453, thus it has been defined with varying ductile fracture criterion (VDFC) and characterized by a function of strain rate and temperature. According to VDFC diagram, the exact fracture moment and position during various forming processes will be predicted conveniently, in addition to which, the deformation domains with lower fracture risk corresponding to higher VDFC can be identified.
Key words: aluminum alloy; compression; damage; ductile fracture criterion
1 Introduction
Aluminum alloy has some advantageous properties that make them an excellent choice for a number of applications. Severely deformable aluminum alloy mostly exhibit narrow deformation windows in terms of temperature and strain rate, which generally can be defined by processing maps [1]. An important concern in aluminum alloy forming processes is whether the desired deformation can be performed without any fracture in workpiece. In industrial practice, however, the empirical know-how of the designer is decisive for the fracture-free quality of the products, but often requiring very costly trial-and-error [2]. Thus, there is a critical need for predicting and preventing fracture, which is a major feature of the forming process and the quality of the products. Most bulk metal-forming processes may be limited by ductile fracture, e.g., the occurrence of internal or surface fracture in the workpiece. If it is possible to predict the conditions within the deforming workpiece which lead to fracture, then it may be feasible to choose appropriate process conditions and to modify the forming processes in order to produce sound products [3].
The material damage state in industrial processes can be simulated by implementing damage model into finite element software. The occurrence of ductile fracture is often a limiting factor in metal forming processes. Therefore the damage degree, that is, the fracture tendency can be characterized as the ration of damage value and ductile fracture criterion (DFC). Historically, DFC is based on the experimental work that utilizes a deformation process that is related to actual industrial applications [4]. So far, the fracture criterion such as Cockcroft-Latham type [5] is suited for ductile fracture in bulk metal-forming simulation. Usually, DFC is considered a constant of material, like yield stress, stress limit. Cockcroft and Latham have not expounded whether DFC depends on the temperature and strain rate [1-7].
The main research objective is to analyze and uncover the response of DFC to deformation conditions for 7075 aluminum alloy. An innovative concept of damage sensitive rate is brought forth as the essential intermediate quantity to determine DFC. As for the innovative approach, the compression tests whether there are cracks, numerical simulations provide mutual support to determine the varying ductile fracture criterion (VDFC). Without a doubt, VDFC of 7075 aluminum alloy is convenient to predict the exact fracture moment and position during various forming processes.
2 Experimental
The chemical compositions (mass fraction, %) of 7075 aluminum alloy used in this study were Zn 5.5, Mg 2.2, Cr 2.2, Cu 1.7, Si 0.4, Fe 0.3, Mn 0.1, Al (balance). Before the experiment, the homogenized ingot was scalped to diameter of 10 mm and height of 12 mm with grooves on both sides filled with machine oil mingled with graphite powder as lubrication. On a computer- controlled, servo-hydraulic Gleeble 1500 machine, the specimens were resistance heated at a heating rate of 1 K/s and held at a certain temperature for 180 s to ensure a uniform starting temperature and decrease the material anisotropy. All the sixteen specimens were compressed to a true strain of 0.9163 (height reduction of 60%) at the temperatures of 573, 623, 673 and 723 K, and the strain rates of 0.01, 0.1, 1 and 10 s-1. During the compressing processes the variations of stress and strain were monitored continuously by a personal computer equipped with an automatic data acquisition system. The true compressive stress—strain curves of 7075 aluminum alloy deformed at four temperatures and four strain rates are shown in Fig. 1.
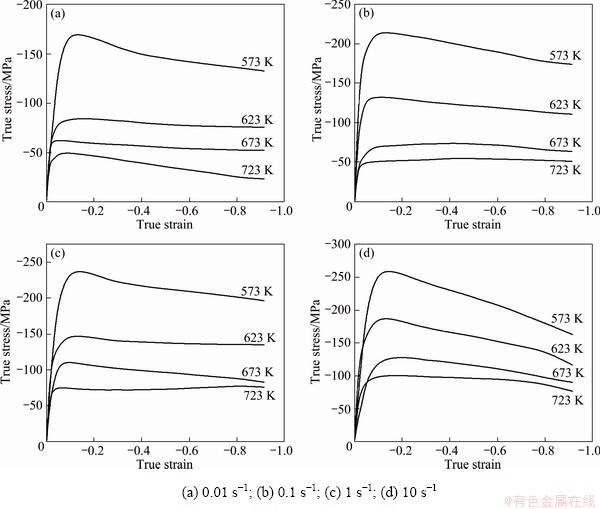
Fig. 1 True stress—strain curves of 7075 aluminum alloy at different strain rates and temperatures
3 Conditions for damage computation
3.1 Cockcroft-Latham’s DFC
In order to design an optimum plastic forming process without failure, a ductile fracture criterion is highly important and necessary. Numerous criteria have been developed for ductile fracture prediction in metal plastic deformation. Cockcroft and Latham [7-11], based on cumulative damage theory, developed a damage computation module based on a critical value of the tensile strain energy per unit volume, which has been applied successfully to a variety loading situations. It has been used to assess the degree of working imposed on aluminum alloy in such process as extrusion, rolling and upsetting by tools. Cockcroft-Latham’s damage [7-9] can be expressed as an amount of work that the ratio (D) of maximum tensile stress σT to effective stress  carries out through the applied equivalent strain
carries out through the applied equivalent strain  in a metal-working process, i.e.,
in a metal-working process, i.e.,
 (1)
(1)
where  is the total equivalent strain corresponding to fracture failure, and the maximum damage value, Dmax, is named ductile fracture criterion. The DFC value, Dmax, is dependent on the material metallurgical properties such as grain size, grain form and non-metallic inclusion content. By comparing D with Dmax, the risk of material failure during processing is assessed.
is the total equivalent strain corresponding to fracture failure, and the maximum damage value, Dmax, is named ductile fracture criterion. The DFC value, Dmax, is dependent on the material metallurgical properties such as grain size, grain form and non-metallic inclusion content. By comparing D with Dmax, the risk of material failure during processing is assessed.
In order to calculate D by FE simulation, Eq. (1) has to be converted to an appropriate discrete expression which is convenient for FE code, i.e.,
 (2)
(2)
where  is the equivalent strain rate, tf is the total time corresponding to fracture failure, and △t is the variable time increment used in the FE analysis.
is the equivalent strain rate, tf is the total time corresponding to fracture failure, and △t is the variable time increment used in the FE analysis.
As several series of billet samples had been compressed at different deformation temperatures and strain rates, the true stress-strain data collected resulted from the performance of simulations through an integration method using the subroutine in the DEFORM platform.
3.2 Way to determine Cockcroft-Latham type DFC
From Fig. 1, it can be seen that 7075 aluminum alloy is a typical strain-softening alloy. As is well known, for strain-hardening alloy the fracture criterion can be determined by directly comparing the simulation with the destructive experiments in terms of the critical deformation level. However, for strain-softening alloy the ductile fracture criterion cannot be determined directly because it is difficult to find visible cracks on the surface of deforming billet and the stress—strain curves do not present fracture points. Thus, finding an indirect way to evaluate the ductile damage criterion and identify its applicability and reliability, however, is a non-trivial issue that still needs to be addressed in greater depth.
According to the cumulative damage theory, the damage value a moment ago is less than that a moment later during a compressing process. Therefore, the maximum value seems to appear at the last simulation step. As the cumulation characters maybe contribute to finding the critical damage value. It is necessary to analyze the damage cumulating process. Thus, an innovative concept about the sensitive rate of Cockcroft-Latham damage (as Eq. (3)) in plastic deformation (Rstep) is brought out and defined as the ratio of the damage increment at one step (△D) to the accumulated value (Dacc). It is supposed that if the maximum damage value will keep increasing in a very small growth rate near to zero, it means that fractures have appeared [7]. Thus, the fracture time, that is, fracture strain or fracture height reduction will be identified and determined.
 (3)
(3)
The emergence of the assumptions above responds to Kachanov’s explaination of damage [12-14]. Kachanov explained the one-dimensional surface damage variable by considering a damaged body and a representative volume element (RVE) at a point  oriented by a plane defined by its normal
oriented by a plane defined by its normal  and its abscissa x along the direction
and its abscissa x along the direction  (as shown in Fig. 2) [12]. The value of the damage
(as shown in Fig. 2) [12]. The value of the damage 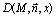 attached to the point M in the direction
attached to the point M in the direction  and the abscissa x is
and the abscissa x is
 (4)
(4)
where 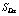 is the area of intersection of all the flaws with the plane defined by the normal
is the area of intersection of all the flaws with the plane defined by the normal  and abscissa x; S is the total area at the intersection plane.
and abscissa x; S is the total area at the intersection plane.
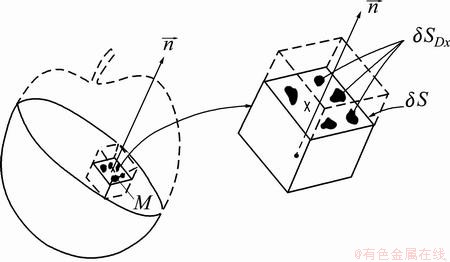
Fig. 2 Damaged RVE in damaged body
Damage D is bounded as 0≤D≤Dc, where Dc is a critical damage corresponding to the decohesion of atoms. D=0 represents the undamaged RVE material, and D=Dc represents the rupture failure in the remaining resisting area. As rupture failure occurs, damage D will keep increasing in a growth rate near to zero or equal to zero with increasing deformation strain. This means that the damage will not be sensitive to deformation.
4 Computation results
4.1 Cumulating process of ductile damage
FE analyses of upsetting deformation and damage value under the same process condition with the tests were carried out. Figure 3 shows the damage distribution at the last step (height reduction of 60%) at temperature of 573 K and strain rate of 0.01 s-1. From the simulation results of all the isothermal hot compression tests at the temperatures of 573, 623, 673 and 723 K, and the strain rates of 0.01, 0.1, 1 and 10 s-1, it can be seen clearly that the maximum damage value always appears in the region of upsetting drum corresponding to the higher strain, stress and strain rate, while the minimal value appears in the middle region. From outside to inside in diameter, the damage extent decreases sharply.
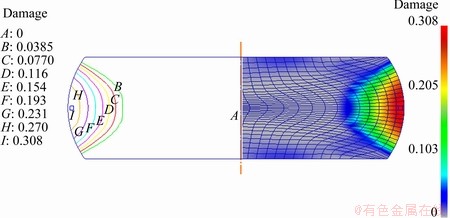
Fig. 3 Damage distribution at last step (height reduction of 60%) at temperature of 573 K and strain rate of 0.01 s-1
Based on the simulation results, the incremental ratios of Cockcroft-Latham type damage during the whole upsetting process at different temperatures and strain rates were evaluated and shown in Fig. 4. It can be seen that the damage increases non-linearly as the compressive true strain decreases from 0 to -0.3, then it increases nearly linearly. Comparing these curves with one another, it is found that, with the strain rates of 1, 10 s-1, for a fixed true strain, the maximum cumulated damage decreases with increasing temperature, while with the strain rate of 0.01 s-1 or 0.1 s-1, it is not apparently regular. In contrast, for a fixed temperature, the maximum cumulated damage decreases regularly with increasing strain rate (see Fig. 5). In further, it can be summarized that the changes of strain rates have a more significant effect on the cumulated damage, that is to say, the damage cumulating process is more sensitive to strain rate.
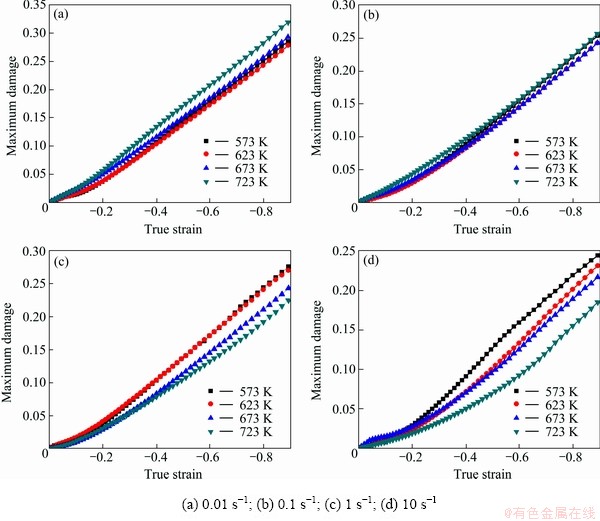
Fig. 4 Maximum damage varying during compressing process at different temperatures and strain rate
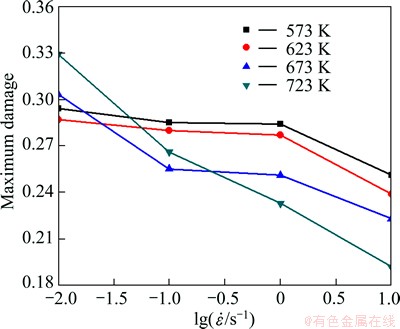
Fig. 5 Relationship between maximum damage and strain rate at end of compressing process
4.2 Evaluation of varying ductile fracture criterion (VDFC)
In order to evaluate the ductile fracture criteria, the fracture occurrence and the damage value at fracture time are necessary to be made certain. Based on the damage cumulating processes shown in Fig. 4, the sensitive rates of Cockcroft-Latham damage (as Eq. (3)) in plastic deformation (Rstep) at different temperatures and strain rates were calculated and shown in Fig. 6. It can be seen that, for all the deformation conditions, Rstep decreases to the trough point rapidly before true strain of 0.05-0.08, then it has a slight increase in true strain of 0.08-0.1, after which it decreases to zero by slow degree in true strain range of 0.1-0.92. The zero point has been added a tolerance 0-0.03 in this study, and it is assumed as the fracture time in tolerance is limited.
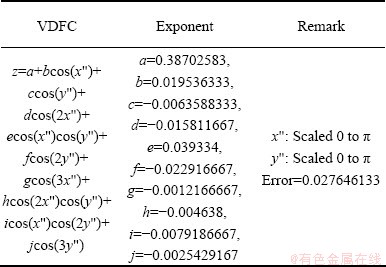
As the fracture time has been determined, the fracture analysis step and the correspondent DFC can be evaluated. The contour plot and response surface of DFC in Fig. 7 show the effects of temperature and strain rate on the ductile fracture criterion of 7075 aluminum alloy. As can be seen in Fig. 7, the ductile fracture criterion is not a constant but a change of 0.255-0.453, thus it can be defined a function of strain rate and temperature (see Table 1) with varying ductile fracture criteria. There is a ridgeline between the point at 573 K and 0.01 s-1 and point at 723 K and 10 s-1 in the scatter diagram, on the both sides of which, DFC value decreases significantly. In addition, there are two peak values on the ridgeline under the deformation conditions of 595-645 K and 0.1-0.01 s-1, 660-690 K and 0.333-0.355 s-1. It is worth explaining that the forming process will be safer under the conditions corresponding to higher DFC. Therefore, it can be educed that cracks will appear most easily under the deformation conditions of 573 K and 10 s-1 corresponding to the lowest DFC.
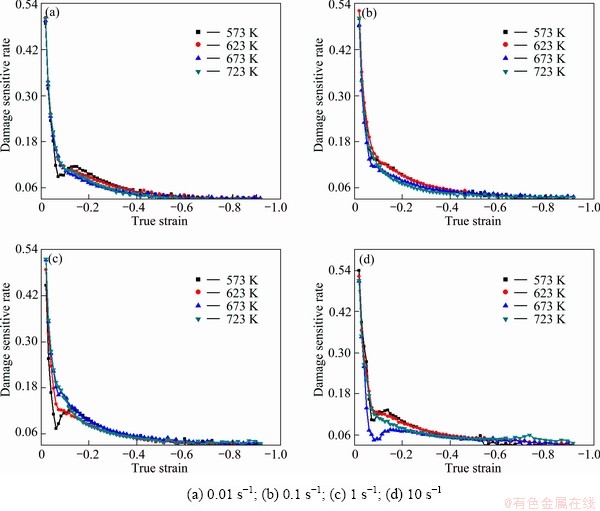
Fig. 6 Variation of damage sensitive rate under different temperatures and strain rates
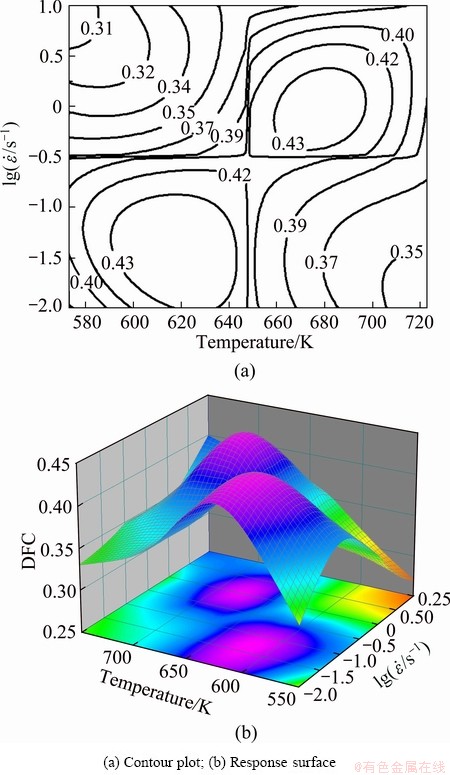
Fig. 7 Temperature and strain rate effect on ductile fracture criteria of 7075 aluminum alloy
Table 1 Approximate analytical formula for VDFC of 7075 aluminum alloy
5 Conclusions
1) By the approach of compression tests and numerical simulations, the ductile damage cumulating process and DFC diagram were evaluated. One of the results shows that for a fixed temperature, the maximum cumulated damage decreases regularly with increasing strain rate. The DFC of 7075 aluminum alloy at temperatures of 573-723 K and strain rates of 0.01-10 s-1 is not a constant but a change in a range of 0.255-0.453.
2) The varying ductile fracture criterion (VDFC) was characterized by bivariate cosine series function of strain rate and temperature, the corresponding contour plot and response surface. According to the VDFC diagram, the exact fracture moment and position during various forming processes will be predicted conveniently, in addition to which, two deformation domains with lower fracture risk are identified as 595-645 K and 0.1-0.01 s-1, and 660-690 K and 0.333-0.355 s-1.
References
[1] SOMMITSCH C, POELT P, RUEF G, MITSCHE S. On the modelling of the interaction of materials softening and ductile damage during hot working of alloy 80A [J]. Journal of Materials Processing Technology, 2006, 177(1-3): 282-286.
[2] KIM D J, KIM B M. Prediction of deformed configuration and ductile fracture for simple upsetting using an artificial neural network [J]. The International Journal of Advanced Manufacturing Technology, 2002, 19(5): 336-342.
[3] KO D C, KIM B M. The prediction of central burst defects in extrusion and wire drawing [J]. Journal of Materials Processing Technology, 2000, 102 (1-3): 19-24.
[4] ARABSHAHI H. Dynamic and static softening behaviors of AA2024 aluminum alloy under hot deformation applications [J]. International Journal of Basic & Applied Sciences, 2009, 9(9): 21-23.
[5] ZHANG X Q, PENG Y H, RUAN X Y. Simulation and fracture prediction for sintered materials in upsetting by FEM [J]. Journal of Materials Processing Technology, 2000, 105(3): 253-257.
[6] ALMOUSAWI M M, DARAGHEH A M, GHOSH S K, HARRISON D K. Some physical defects in metal forming processes and creation of a data base [J]. Journal of Materials Processing Technology, 1992, 32(1-2): 461-470.
[7] XIA Yu-feng, QUAN Guo-zheng, ZHOU Jie. Effects of temperature and strain rate on critical damage value of AZ80 magnesium alloy [J]. Transactions of Nonferrous Metals Society of China, 2010, 20(s2): s580-s583.
[8] DUMONTAB D, DESCHAMPSA A, BRECHETA Y. A model for predicting fracture mode and toughness in 7000 series aluminium alloys [J]. Acta Materialia, 2004, 52(9): 2529-2540.
[9] COCKCROFT M G, LATHAM D J. Ductility and workability of materials [J]. J Inst Met, 1968, 96: 33-39.
[10] WARD M J, MILLER B C, DAVEY K. Simulation of a multi-stage railway wheel and tyre forming process [J]. Journal of Materials Processing Technology, 1998, 80-81(3): 206-212.
[11] FAN W F, LI J H. An investigation on the damage of AISI-1045 and AISI-1025 steels in fine-blanking with negative clearance [J]. Materials Science and Engineering A, 2009, 499(1-2): 248-251.
[12] KRAJCINOVIC D. Damage mechanics [J]. Mechanics of Materials, 1989, 8(2-3): 117-197.
[13] BONORA N, GENTILE D, PIRONDI A. Identification of the parameters of a non-linear continuum damage mechanics model for ductile failure in metals [J]. The Journal of Strain Analysis for Engineering Design, 2004, 39(6): 639-651.
[14] BONORAA N, GENTILEA D, PIRONDIB A, NEWAZ G. Ductile damage evolution under triaxial state of stress: Theory and experiments [J]. International Journal of Plasticity, 2005, 21(5): 981-1007.
7075铝合金的变化型临界损伤因子
权国政,王凤彪,刘莹莹,石 彧,周 杰
重庆大学 材料科学与工程学院,重庆 400044
摘 要:韧性断裂作为一种主要的失效形式制约了成形工艺开发。Cockcroft-Latham损伤准则适用于多数合金韧性断裂失效的数值计算。对于应变软化型7075铝合金,确立临界损伤因子并揭示其与变形条件间的内在联系具有重要意义。通过压缩试验与数值模拟相互佐证的途径获得了7075铝合金临界损伤因子及其分布规律。结果表明:温度一定时,最大累积损伤值随着应变速率的增大而单调减小;7075铝合金的临界损伤因子不是常数,而是为在0.255~0.453范围内变化的变量;可由应变速率和温度为自变量表征临界损伤因子的变化规律。根据临界损伤因子规律分布图,可以精确地预测材料加工中发生断裂的时刻和位置,此外,还可识别出稳健的加工工艺参数区间。
关键词:铝合金;压缩;损伤;韧性断裂准则
(Edited by Xiang-qun LI)
Foundation item: Project (2012ZX04010081) supported by the National Key Technologies R & D Program of China; Project (cstc2009aa3012-1) supported by the Science and Technology Committee of Chongqing, China; Project (CDJZR11130009) supported by the Fundamental Research Funds for the Central Universities, China
Corresponding author: Guo-zheng QUAN; Tel: +86-23-65103065(O), +86-15922900904 (M); E-mail: quangz_2006@yahoo.com.cn
DOI: 10.1016/S1003-6326(13)62525-X