J. Cent. South Univ. Technol. (2011) 18: 2056-2060

DOI: 10.1007/s11771-011-0942-9

Guaranteed cost sampled-data control for

uncertain nonlinear time-varying delay system

FAN Li-ying(樊丽颖)^{1, 2}, WU Jun-feng(武俊峰)¹

1. School of Automation, Harbin University of Science and Technology, Harbin 150080, China;

2. Department of Mathematics, Harbin University of Science and Technology, Harbin 150080, China

? Central South University Press and Springer-Verlag Berlin Heidelberg 2011

Abstract: The robust guaranteed cost sampled-data control was studied for a class of uncertain nonlinear systems with time-varying delay. The parameter uncertainties are time-varying norm-bounded and appear in both the state and the input control matrices. By applying an input delay approach, the system was transformed into a continuous time-delay system. Attention was focused on the design of a robust guaranteed cost sampled-data control law which guarantees that the closed-loop system is asymptotically stable and the quadratic performance index is less than a certain bound for all admissible uncertainties. By applying Lyapunov stability theory, the theorems were derived to provide sufficient conditions for the existence of robust guaranteed cost sampled-data control law in the form of linear matrix inequalities (LMIs), especially an optimal state-feedback guaranteed cost sampled-data control law which ensures the minimization of the guaranteed cost was given. The effectiveness of the proposed method was illustrated by a simulation example with the asymptotically stable curves of system state under the initial condition of x(0)=[0.679 6  0].

Key words: nonlinear system; guaranteed cost sampled-data control; input delay; linear matrix inequalities

1 Introduction

In recent years, sampled-data control theory has attracted much attention, because modern control systems usually employ digital technology for controller implementation [1-2]. Two main approaches have been used for the sampled-data control of linear uncertain systems leading to conditions in terms of linear matrix inequalities (LMIs) [3]. The first one is the input delay approach firstly proposed by FRIDMAN et al, where the sampled-data system was modeled as a continuous-time system with an input delay [4-5]. The second approach is based on the representation of the sampled-data system in the form of impulsive model. The impulsive model approach was applied to sampled-data stabilization of linear uncertain systems in the case of constant sampling [6].

During the last decade, the robust stability and stabilization problem for guaranteed cost control of uncertain linear systems have drawn considerable attention in control system literatures [7-11]. It is well known that time-delay as well as parameter uncertainties occurs in many dynamic systems and frequently causes instability and performance degradation. It is also desirable to design a control system which not only is stable but also guarantees an adequate level of performance. One design approach to this problem is the so-called guaranteed cost control approach first introduced by CHANG and PENG [12]. This approach has the advantage of providing an upper bound on a given cost function and thus the system performance degradation incurred by the uncertainties is guaranteed to be less than this bound. Based on this idea, some significant results have been reported for the continuous-time case [13] and the discrete-time case [14]. Recently, these results have been extended to uncertain nonlinear systems. Although these designs have been proved to be efficient in solving various engineering problems, the guaranteed cost sampled-data control problem for uncertain nonlinear systems has not been fully investigated yet.

In this work, the problem tread is the design of a robust guaranteed cost sampled-data control law for a class of uncertain nonlinear system with time-varying delay. By using input delay approach, sufficient conditions are derived in terms of LMIs, which guarantee the asymptotical stability and performance index less than a certain bound for all admissible uncertainties. In the meantime, the design of optimal guaranteed cost control law was proposed. A numerical example was given to show the feasibility and effectiveness of the proposed technique.

2 Problem formulations

Consider the following uncertain nonlinear system:

           (1)

where x(t)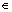Rⁿ, is the state vector; u(t)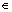R^m is the control input and f:R⁺×Rⁿ→Rⁿ is the uncertain nonlinear term; A, B and A_d are known real constant matrices of appropriate dimensions; ?A, ?B and ?A_d are real-value unknown matrices representing parameter uncertainties. In the present work, the admissible parameter uncertainties are assumed to be of the following form:

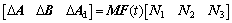                 (2)

where M, N₁, N₂ and N₃ are real constant matrices, and F(t)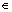R^i×j, is an unknown time-varying matrix function satisfying

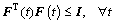                                                     (3)

It is assumed that all the elements of F(t) are Lebesgue measurable.

Assumption 1: The uncertain nonlinear term f satisfies:

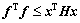                                                              (4)

where H is known positive definite matrix.

The continuous control signal u(t) is realized through the zero-order hold function, that is:

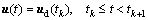                                           (5)

where 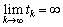 and u_d is a discrete-time control signal.

Assumption 2: .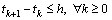

Define the continuous quadratic cost function as follows:

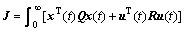                               (6)

where Q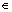R^n×n and R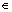R^m×m, are known symmetric and positive definite matrices.

Consider a state-feedback control law of the form:

                                            (7)

The digital control law as a delayed control is represented as follows:

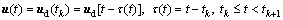  (8)

Under control law in Eq.(8), the closed-loop system is given by:

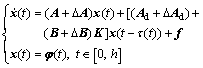                          (9)

Under Assumption 2, τ(t)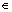(0, h] and  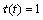for t≠t_k.

Definition 1: Consider the uncertain system (1), J^* is called guaranteed cost and u^* is called robust guaranteed cost sampled-data control law for the uncertain system in Eq.(1). If there exist a state-feedback control law u^* and a scalar J^*>0, the close-loop system in Eq.(9) is asymptotically stable, and for all admissible uncertainties (3) and (4), the cost function satisfies J≤J^*.

The guaranteed cost sampled-data control problem is to design a state-feedback control law such that not only the stability of closed-loop system but also an adequate level of performance is guaranteed under the parameter uncertainties by using input delay approach.

3 Results

An input delay approach will be developed to solve the problem formulated in Section 2. The following lemma is used in the proof of the main results.

Lemma 1 [15]: Let Y, M and N be the given matrices of compatible dimension, Y is a symmetric matrix, then for any F(t) satisfying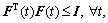

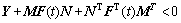
if and only if there exists a constant ε>0, such that

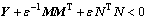
Lemma 2 [16]: For any real vector X and Y with appropriate dimension and any positive scalar α, we have

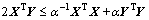
The following theorem gives sufficient condition for the existence of robust guaranteed cost sampled-data control law.

Theorem 1: If there exist symmetric and positive definite matrices P, S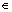R^n×n, for all admissible uncertainties in inequality (4) and Eq.(5), the following matrix inequality holds:

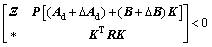                     (10)

where * is the symmetric elements of the symmetric matrix;

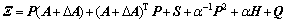
Then, system in Eq.(9) is asymptotically stable;

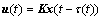                                                      (11)

is the guaranteed cost sampled-data control law, and the cost function is

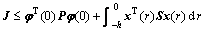                       (12)

Proof: Consider the following Lyapunov function:

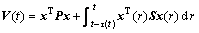                         (13)

where P>0, S>0, calculating the derivative along the trajectory of the system in Eq.(9) yields:

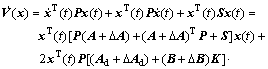
                                      (14)

By applying Assumption 1 and Lemma 2, it follows that:

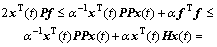
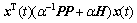                                     (15)

Substituting Eq.(15) into Eq.(14), it obtains that

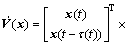
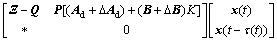
Inequality (10) implies

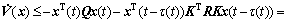
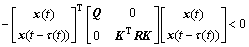
Therefore, system in Eq.(9) is asymptotically stable. Furthermore, by integrating both sides of the above inequality from 0 to ∞ and using the initial condition, as the closed-loop system Eq.(9) is asymptotically stable, it follows immediately that:

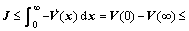
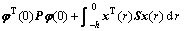
Thus inequality (12) holds. This proves the theorem.

Consequently, the sufficient condition for the existence of robust guaranteed cost sampled-data control law will be established in terms of matrix inequality.

Theorem 2: If there exist constants ε>0, α>0, W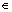R^m×n, and symmetric and positive definite matrices X, S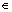R^n×n, the following matrix inequality holds:

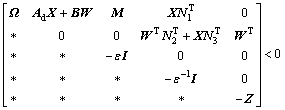(16)

Then

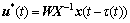                                              (17)

is a robust guaranteed cost sampled-data control law of the system in Eq.(1);

The cost function of system in Eq.(9) is as follows:

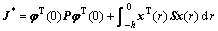                   (18)

Proof: Let

Y=

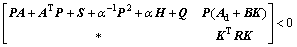
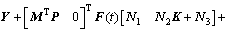
Then, inequality (10) is equivalent to inequality 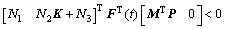(19).

           (19)

By applying Lemma 1, the above matrix inequality holds if and only if there exists a constant ε>0 such that

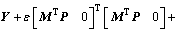
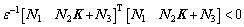
From Schur complement, it follows that:

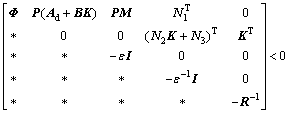(20)

where .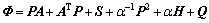

Pre- and post-multiplying (20) by diag(P^-1, P^-1, I, I, I), the following inequality is true:

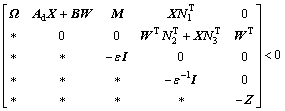
where 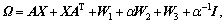 W₁= P^-1SP^-1, W₂=P^-1HP^-1, W₃=P^-1QP^-1, W=KP^-1, X=P^-1, Z=R^-1.

With arguments of the Theorem 1, this finishes the proof.

The following theorem develops this optimization problem.

Theorem 3: Consider the uncertain system (1) under sampled measurements with cost function in Eq.(6), if the following optimization problem holds:

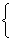
                                               (21)

s.t. inequality (16)

then

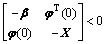                                                     (22)

and

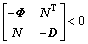                                                           (23)

where tr(Φ) represents the trace of Φ; D=S^-1 and N satisfies

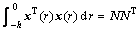
have a feasible solution ε, β, X, W, D, Φ, then the control law u^* is an optimal state-feedback guaranteed cost sampled-data control law which ensures the minimization of the guaranteed cost in Eq.(18).

Proof: By Theorem 2, if the inequality (16) has feasible solution ε, β, X, W, D, then the control law u^* is a guaranteed cost sampled-data control law of system (1), and the cost value satisfies Eq.(18). Inequalities (22) and (23) are equivalent to the following inequalities:

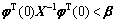 and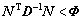

On the other hand,

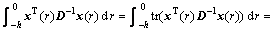
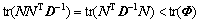
Therefore, the cost function in Eq.(18) satisfies

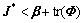
This completes the proof.

4 Numerical example

In this section, an example was chosen to illustrate the effectiveness of the designed guaranteed cost sampled-data control law with input delay.

Consider the uncertain nonlinear system (1), the parameters are given by (the parameter partly from Ref.[16])

, ,

, ,

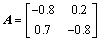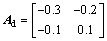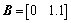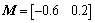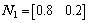, N₂=0.11, ,

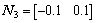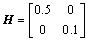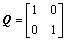, , R=1, ,

, , .

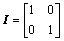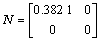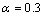By using mincx solver of Matlab LMI Control Toolbox to solve the LMIs of inequalities (16), (22) and (23), a set of feasible solutions can be obtained as follows:

, ,

, ,

.

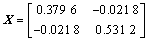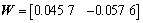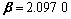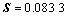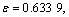
Therefore, the optimal guaranteed cost sampled-data control law and optimal guaranteed cost of the uncertain close-loop system (9) can be obtained as follows:

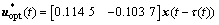
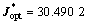
By using feasp solver of Matlab LMI Control Toolbox to solve the LMIs of inequality (16), the guaranteed cost sampled-data control law and guaranteed cost of the uncertain close-loop system in Eq.(9) can be obtained as follows (other associated matrices are omitted here):

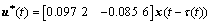
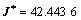
The state responses are shown in Fig.1. It can be seen that both state components converge to a constant when time increases, revealing the stability property of the system.

From the above simulation results, it is concluded that the performance index of the optimal guaranteed cost sampled-data control law is obviously smaller than that of general guaranteed cost sampled-data control law. Under the action of the optimal control law, the system in Eq.(9) is asymptotically stable, as shown in Fig.1.

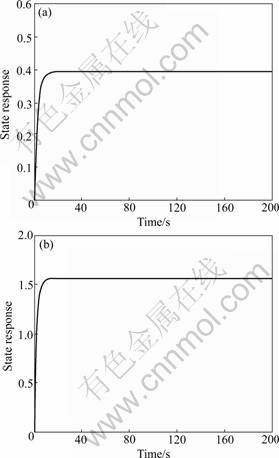
Fig.1 State response curves: (a) Variable X₁; (b) Variable X₂

5 Conclusions

1) Based on the Lyapunov stability theory, an input delay approach has been developed to design a robust guaranteed cost sampled-data control law, which guarantees not only the asymptotical stability but also a bound of quadratic performance level for the closed-loop system for all admissible parametric uncertainties. Sufficient conditions are formulated in the form of LMIs.

2) A convex optimization problem is introduced to select the optimal guaranteed cost sampled-data control law. The illustrative example demonstrates the effectiveness of the proposed method.

References

[1] GAO Hui-jin, WU Jun-li, SHI Peng. Robust sampled-data H_∞ control stochastic sampling [J]. Automatica, 2009, 7(45): 1729-1736.

[2] HU Bo, MICHEL A N. Robustness analysis of digital feedback control systems with time-varying sampling periods [J]. Journal of the Franklin Institute, 2000, 337(2): 117-130.

[3] BOYD S, GHAOUI E L, FERON E, BALAKRISHNAN V. Linear matrix inequalities in systems and control theory [M]. Philadelphia: SIAM frontier series, 1994: 3-4.

[4] FRIDMAN E. A refined input delay approach to sampled-data control [J]. Automatica, 2010, 46(2): 421-427.

[5] FRIDMAN E, SEURET A, RICHARD J. Robust sampled-data stabilization of linear systems: An input delay approach [J]. Automatica, 2004, 40(8): 1441-1446.

[6] HU L, LAM J, CAO Y, SHAO H. A LMI approach to robust H₂ sampled-data control for linear uncertain systems [J]. IEEE Transactions on Systems, Man and Cybernetics, Part B, 2003, 33(1): 149-155.

[7] LIU Fu-chun, YAO Yu, HE Feng-hua, JI Deng-gao. Robust guaranteed cost control for sampled-data systems with parametric uncertainties [C]// Proceedings of the 27th Chinese Control Conference. Kunming: Piscataway, Computer Society, 2008: 683- 687.

[8] FRIDMAN E. A new Lyapunov technique for robust control of systems with uncertain non-small delays [J]. IMA Journal of Mathematical Control and Information, 2006, 23(2): 165-179.

[9] PAPACHRISTODOULOU A, PEET M, NICULESCU S. Stability analysis of linear systems with time-varying delays: Delay uncertainty and quenching [C]// Proceedings of the 46th IEEE Conference on Decision and Control. New Orleans: Institute of Electrical and Electronics Engineers Inc., 2007: 2117-2122.

[10] LAI Xu-zhi, WEN Jing, WU Min. Design for robust stabilization of nonlinear systems with uncertain parameters [J]. Journal of Central South University of Technology, 2004, 11(1): 102-104.

[11] XIE Yong-fang, GUI Wei-hua, JIANG Zhao-hui, YANG Chun-hua. Design of decentralized robust H_∞ state feedback controller [J]. Journal of Central South University of Technology, 2006, 13(5): 559-562.

[12] CHANG S S L, PENG T K C. Adaptive guaranteed cost control of systems with uncertain parameters [J]. IEEE Transactions on Automatic Control, 1972, 17(4): 474-483.

[13] YU Li, CHU Jian. LMI approach to guaranteed cost control of linear uncertain time-delay systems [J]. Automatica, 1999, 35(6): 1155-1159.

[14] YU Li, GAO U-Rong. Optimal guaranteed cost control of discrete-time uncertain systems with both state and input delays [J]. Journal of the Franklin Institute, 2001, 338(1): 101-110.

[15] XIE Li-hua. Output feedback H_∞ control of systems with parameter uncertainty [J]. International Journal of Control, 1996, 63(4): 741-750.

[16] QIU Ji-qing, WANG Yan-jun, DU Xiao-ming, ZHAO Yan-chun, ZHU Xiao-dan. Robust guaranteed cost control of nonlinear uncertain time-varying delay systems [C]// Proceedings of the ninth International Conference on Machine Learning and Cybernetics. Qingdao: Piscataway, IEEE Computer Society, 2010: 969-974.

(Edited by HE Yun-bin)

Foundation item: Project(12511109) supported by the Science and Technology Studies Foundation of Heilongjiang Educational Committee of 2011, China

Received date: 2011-06-21; Accepted date: 2011-08-31

Corresponding author: FAN Li-ying, PhD; Tel: +86-451-86390686; E-mail: fan_liying@163.com