Trans. Nonferrous Met. Soc. China 22(2012) 925-934

Kinetics of thermal decomposition of lanthanum oxalate hydrate

ZHAN Guang¹, YU Jun-xia¹, XU Zhi-gao¹, ZHOU Fang², CHI Ru-an¹

1. Hubei Novel Reactor and Green Chemical Technology Key Laboratory, Key Laboratory for

Green Chemical Process of Ministry of Education, School of Chemical Engineering and Pharmacy,

Wuhan Institute of Technology, Wuhan 430074, China;

2. School of Minerals Processing and Bioengineering, Central South University, Changsha 410083, China

Received 6 April 2011; accepted 2 June 2011

Abstract: Lanthanum oxalate hydrate La₂(C₂O₄)₃·10H₂O, the precursor of La₂O₃ ultrafine powders, was prepared by impinging stream reactor method with PEG 20000 as surfactant. Thermal decomposition of La₂(C₂O₄)₃·10H₂O from room temperature to 900 ℃ was investigated and intermediates and final solid products were characterized by FTIR and DSC-TG. Results show that the thermal decomposition process consists of five consecutive stage reactions. Flynn-Wall-Ozawa (FWO) and Kissinger-Akahira- Sunose (KAS) methods were implemented for the calculation of energy of activation (E), and the results show that E depends on α, demonstrating that the decomposition reaction process of the lanthanum oxalate is of a complex kinetic mechanism. The most probable mechanistic function, G(α)=[1-(1+α)^1/3]², and the kinetic parameters were obtained by multivariate non-linear regression analysis method. The average E-value that is compatible with the kinetic model is close to value which was obtained by FWO and KAS methods. The fitting curve matches the original TG curve very well.

Key words: lanthanum oxalate decahydrate; TG-DSC; thermal decomposition; multivariate non-linear regression analysis

1 Introduction

Sustainable technological development is strongly dependent on new materials with particular mechanical, chemical, electrical, magnetic, or optical properties. In order to address this challenge, interdisciplinary research technologies, to develop new materials, especially inorganic materials, to impart new functional properties and to provide new processing methods for the formation of useful objects are under intense focus [1,2].

Within the class of inorganic materials, oxides perform various functions [3]. The oxide ceramics are well known materials for technical applications, particularly in electronic and structural areas. The purity of these materials is extremely important. In the oxide class, lanthanum oxide (La₂O₃) is one of the most widely studied oxide over the years. Lanthanum oxide has been widely applied to many industrial applications. For example, it is an important component of automobile exhaust-gas conversion [4], as a catalyst support in the formation of gas conversion catalyst [5] and as a catalyst of oxidative coupling of methane [6]. It is also used as a refractory oxide for calcium lights, optical glass [7] and in the formation of ceramics as a core for carbon arc electrodes [8]. However, as the raw material in these fields, synthesis of lanthanum oxide with good quality is very important [9]. The rare earth oxide can be prepared by oxalate thermal decomposition, but its characteristics on a great degree depend upon the calcining conditions. So monitoring of the process of oxalate thermal decomposition is an important issue [9].

BALBOUL et al [5] had reported that the characteristic of thermal decomposition of La₂(C₂O₄)·10H₂O to the onset of La₂O₃ could be measured by thermogravimetry (TG) and different thermal analysis (DTA). The characteristics of the lanthanum oxalate decahydrate and the activation energy of the observed thermal processes were obtained by means of IR-spectroscopy, X-ray diffractometry and N₂-adsorption isotherm, but kinetic of thermal analysis of various compounds is of major importance because of their frequent applications in calcination metallurgy and in the production of sorbents and catalysts with large-surface materials [10]. Unfortunately, most literatures rested on the understanding of the activation energy of the thermal processes and few people paid attention to the kinetics and the most probable model of thermal decomposition.

Compared with other methods, thermal decomposition process has many advantages, such as more effective control of size and shape of the particle, shorter preparation time and fewer impurities in the final product [11,12]. Thermal decomposition methods were preferably used to prepare the nanostructure ceramic materials [13,14]. In the future, the exploitation of such lanthanide oxide architectures by thermal decomposition may provide an opportunity of producing innovative ceramic materials with novel and tunable magnetic, electronic, or catalytic properties [15].

At present, the ultra-fine La₂O₃ powder can be prepared by solid phase, hydrothermal synthesis, precipitation, sol-gel and micro-emulsion methods, respectively. Among these methods, precipitation method is used widely for its simplicity. High and uniform super-saturation is the key factor to affect the size of the “ultra fine” solid particles in precipitation. In order to obtain the high and uniform super-saturation, a kind of new reactor, submerged circulative impinging stream reactor (SCISR), was developed [16]. SCISR can do homogeneous micro-mixing. The impingement zone can create high and uniform super-saturation environment for precipitation to yield nuclei in a huge amount, while the non-mixing regions with much lower super-saturation favor helping deactivation of micro-crystal surfaces [16].

In this work, lanthanum oxalate hydrate (precursor) was prepared by SCISR method with PEG 20000 as surfactant. The thermal decomposition of La₂(C₂O₄)₃·10H₂O from room temperature to 900 ℃ in nitrogen gas was investigated by DSC-TG. Intermediates and final solid products were characterized by FTIR. The kinetics of decomposition was studied by implementing Flynn-Wall-Ozawa (FWO) and Kissinger-Akahira- Sunose (KAS) methods and the most possible conversion function was simulated by the multiple non-linear regression method.

2 Experimental

2.1 Materials

Lanthanum nitrate hexahydrate (La(NO₃)₃·6H₂O, 99.9%, Aladdin, China), ammonium oxalate monohydrate((NH₄)₂C₂O₄·H₂O, analytical reagent, Sinopharm Chemical Reagent Co., Ltd, China), and PEG 20000(analytical reagent, Sinopharm Chemical Reagent Co., Ltd, China) were used for the preparation of oxalate.

2.2 Preparation of precursor

The precursor, lanthanum oxalate decahydrate, was prepared in SCISR. The sketch of the submerged circulative impinging stream reactor (SCISR) is shown in Fig. 1.

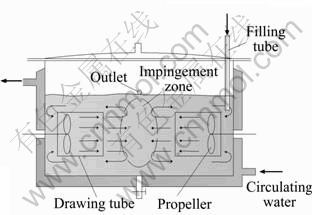
Fig. 1 Sketch of SCISR

When the temperature was 40℃ and the propellers rotated at 600 r/min, 625 mL of 0.1 mol/ L (NH₄)₂C₂O₄·H₂O solution, , and 0.2165 g of PEG 20000 were mixed together and added into the reactor firstly, then 125 mL of 0.1 mol/L La(NO₃)₃·6H₂O solution, was added in drop. After 30 min, white precipitate of precursor was formed, filtrated and then washed with distilled water and absolute alcohol, respectively. After being dried in a vacuum oven at 70 ℃ and grinded in agate mortar, lanthanum oxalate decahydrate powder was obtained. The TEM (JEM-100CXⅡ, Japan Electronics Co., Ltd., Japan) micrograph of La₂(C₂O₄)₃·10H₂O is shown in Fig. 2.

Fig. 2 TEM image of La₂(C₂O₄)₃·10H₂O

Figure 2 illustrates that many needle-shaped particles are in the size range of 5-100 nm. The obtained precursor was then heated at different temperatures ranging from room temperature to 900 ℃. Intermediates and final solid products were characterized by FTIR (Thermo Nicolet 380, Thermo Scientific, America).

2.3 Thermal analysis

DSC-TG analyses (NETZSCH STA409PC, NETZSCH, Germany) of lanthanum oxalate were carried out at heating rates of 10, 15, 20, and 30 ℃/min, from room temperature to 900 ℃ in a dynamic atmosphere of nitrogen (60 mL/min). The sample mass was about 5.5 mg. All records were corrected by the baseline subtraction (a run without pans under the same conditions).

2.4 FTIR analysis

FTIR spectra were obtained at a resolution of     4 cm^-1 over the range of 4000-400 cm^-1. The spectra of intermediates and final products were obtained from thin (>20 mg/m²), lightly loaded (<1%) KBr-supported (KBr, Jasco, spectroscopy grade) discs.

2.5 XRD analysis

The products obtained at different temperatures were determined at room temperature using a powder X-ray diffractometer (XD-5A, Shimazu, Japan) with a scanning step of 0.04° in the 2θ range of 20°-70° (Cu K_α radiation).

2.6 Thermal and kinetic analyses

The influence of different temperature regions upon the thermal behavior of chemical compounds can provide kinetic parameters indicating change in the reaction pathway. The complexity of a stage can be expressed from the activation energy (E) dependent on the extent of conversion (α). The activation energy can be obtained by isoconversional method. If E does not depend on α, the investigated process is a simple one and should be described by a unique kinetic triplet. If not, the process is complex. In this work integral isoconversional methods were used to analyze the non-isothermal kinetics of the lanthanum oxalate.

The extent of conversion, α, is defined by the following equation:

α=(m₀-m)/(m₀-m_f)                            (1)

where m is the mass of the sample at a given time t; m₀ and m_f refer to masses at the beginning and the end.

The rate of solid-state non-isothermal decomposition reactions is expressed as [17-23]:

dα/dT=(A/β)exp[-E/(RT)]f(α)                   (2)

where T is the temperature; β is the linear heating rate; f(α) is the reaction model depending on the reaction mechanism; A is the preexponential factor; R is the gas constant.

Rearranging Eq. (2) and integrating both sides of the equation lead to the following expression [24]:

    (3)

Here, P(u) =and u=E/(RT).

Flynn-Wall-Ozawa method [25-30] was derived from the integral method. The technique assumes that A, f(α) and E are independent of T while A and E are independent of α, and then Eq. (3) is integrated to give the following equation:

lg g(α)=lg(AE/R)–lg β+lg P[E/(RT)]              (4)

Using Doyle’s approximation [31-34] for the integral which allows for E/RT>20, Eq. (3) can be simplified as:

lg β=lg[AE/(Rg(α))]-2.315-0.4567E/(RT)        (5)

where g(α) is considered a constant value. Thereupon,  lg β has a linear relationship with 1/T and the activation energy can be obtained from the slope.

Kissinger-Aksahira-Sunose method [35-37] is an integral isoconversional method similar to the FWO method.

ln(β/T²)=ln[AE/(Rg(α))]-E/(RT)                 (6)

The dependence of ln(β/T²) on 1/T, calculated for the same α values at the different heating rates β, can be used to calculate the activation energy. TG data were selected under the different β and the same α, lg(β/T²)—1/T curve was plotted, line slope was calculated by least square method and activation energy E was obtained from the slope.

3 Results and discussion

3.1 Thermal decomposition process

DSC-TG curves corresponding to the thermal mass losses of the precursor at different heating rates, are shown in Fig. 3. All curves are approximately in the same shape, indicating that the mass loss is independent of the heating rate. It could be seen that there are five mass loss stages in the decomposition process.

At the first stage, a weak decomposition occurs in the temperature range of 25-226.2 ℃ (t_max=139.6 ℃). The experimental mass loss (14.5%) of the first stage is very close to the theoretical mass loss (14.9%), which could be attributed to the evolution of six water molecules. The second stage beginning at about 226.2 ℃ and ending at 295.2 ℃ with a mass loss about 4.9% can be assigned to the loss of two water molecules with the increased  temperature. The third stage occurs between 295.2 ℃ and 372.4 ℃ (t_max=329.2 ℃) with a mass loss about 5.03%, indicating that the last two water molecules of lanthanum oxalate decahydrate are lost. The previous three stages of thermal decomposition process are expressed as follows:

La₂(C₂O₄)₃·10H₂O→La₂(C₂O₄)₃·4H₂O+6H₂O      (7)

La₂(C₂O₄)₃·4H₂O→La₂(C₂O₄)₃·2H₂O+2H₂O       (8)

La₂(C₂O₄)₃·2H₂O→La₂(C₂O₄)₃+2H₂O            (9)
 

FTIR spectra of La₂(C₂O₄)₃·10H₂O and the intermediates heated at 140 and 250 ℃ are shown in  Fig. 4. It can be seen that these three spectra are very similar, demonstrating that only water molecules are lost at the previous three mass loss stages [5].

The fourth stage locates approximately between 372.4 ℃ and 600 ℃ (t_max=410.2 ℃) with a mass loss about 27.2%, which could be assigned to the decomposition of La₂(C₂O₄)₃ into La₂O₂CO₃. The decomposition process is as follows:
 

La₂(C₂O₄)₃→La₂O₂CO₃+3CO↑+2CO₂↑          (10)

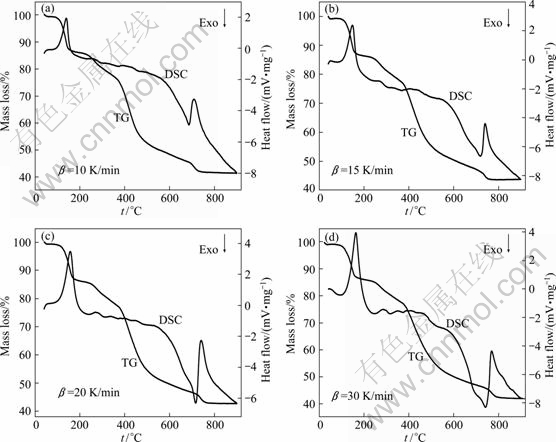
Fig. 3 TG-DSC plots for precursor at different heating rates: (a) 10 K/min; (b) 15 K/min; (c) 20 K/min; (d) 30 K/min

Fig. 4 IR spectra of precursor and powders heated at different temperatures: (a) Precursor and intermediate; (b) Intermediate
 

FTIR spectra of solid phases heated at 500 and 600 ℃ are shown in Fig. 4.

The broad absorption band peaks at 1501, 1450, 1357 and 851 cm^-1 are the indication of characteristic absorption peaks of the monoclinic IA system La₂O₂CO₃ [10].

The fifth stage locates at approximately 600 and 745.6 ℃ (t_max= 708.3 ℃) with a mass loss about 6.5%, which is account for the conversion of La₂O₂CO₃ to La₂O₃. The decomposition reaction is as follows:

La₂O₂CO₃→La₂O₃+CO₂↑                     (11)

It can be seen from the FTIR spectra of 700 and 800 ℃ that the characteristic absorbance bands at about 649 cm^-1 is the La—O stretching vibration. The broad absorption band at about 3604 cm^-1 corresponding to hydroxyl stretching vibration is present, which may be due to the surface adsorbed water by nascent state La₂O₃.

Figure 5 indicates that the powders of La₂(C₂O₄)₃·10H₂O decomposed into different products after calcining at different temperatures for 3 h. Products calcined at 390 and 500 ℃ are La₂O₂CO₃ powders and at 800, 900 ℃ are La₂O₃ powders, according to JCPDS cards 54—0212 and 05—0602; while at 600, 700 ℃ there is no obvious diffraction peak of La₂O₂CO₃ or La₂O₃. It is possible that at 500-800 ℃ most La₂O₂CO₃ decomposed into La₂O₃ but crystal of La₂O₃ had not grown well.

Fig. 5 XRD patterns of powders heated at different temperatures

Based on the results above, thermal decomposition data of La₂(C₂O₄)₃·10H₂O are listed in Table 1. It can be seen that the experimental values are consistent with the theoretical values for all stages.

3.2 Thermal decomposition kinetics

3.2.1 Activation energy

Applying Eqs. (5) and (6) on the TG data,     plots of lg β vs 1/T (FWO) and ln(β/T²) vs 1/T (KAS) corresponding to different α can be obtained by a linear regress of least–square method, respectively. The FWO and KAS analysis results taken from the four TG measurements of the first endothermic peak are shown in Figs. 6 and 7, respectively. By the same way, the plots of the other peaks can be obtained. The activation energy E can be calculated from the slopes of the straight lines. If E value is independent of α, the decomposition may be a simple reaction, otherwise, it is of multi-step reaction mechanism [38]. The average activation energies are calculated at those heating rates via the FWO and KAS methods in the α range of 0.1-0.9, and tabulated and drawn in Table 2, Table 3 and Fig. 8, respectively.

Table 1 Thermal decomposition data of La₂(C₂O₄)₃·10H₂O

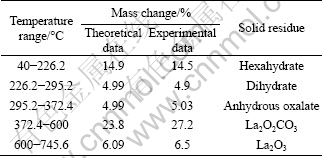
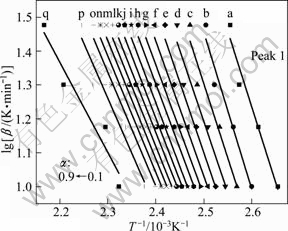
Fig. 6 lg β—1/T plots for E of the first endothermic peak by FWO method

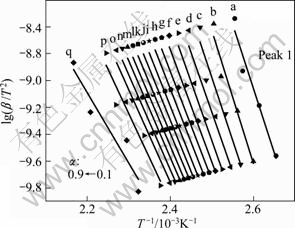
Fig. 7 ln(β/T²)—1/T plots for E of the first endothermic peaks KAS method

Table 2 Activation energies (E) based on FWO method with various extent of conversion (α)

Table 3 Activation energies (E) based on KAS method with various extent of conversion (α)

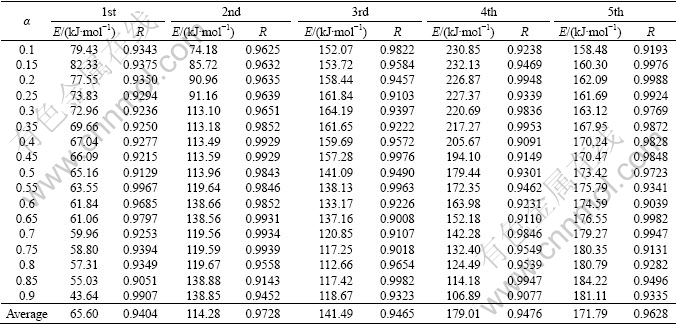
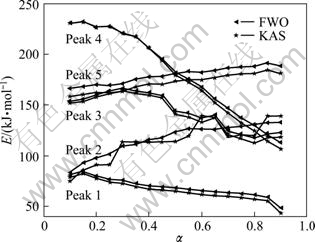
Fig. 8 Dependence of apparent value E on α

The activation energy values calculated by FWO method are close to the ones obtained by KAS method. And it also could be seen that E depends on α, demonstrating that the decomposition reaction process of the lanthanum oxalate is of a complex kinetic   mechanism [38]. For this multi-step reaction, FWO and KAS methods cannot obtain the accurate mathematical model and kinetic parameters of those thermal decomposition processes. So the most probable model and the kinetic parameters should be obtained by multivariate non-linear regression method [38]. The difference between the values of the activation energy obtained by FWO and KAS methods may be attributed to the different approximations of the temperature integral  they use [39].

3.2.2 Determination of probable mechanism

For a one-step reaction, the most probable model can be obtained by multivariate linear regression method but for a multi-step reaction, the most probable model should be obtained by multivariate non-linear regression method [38]. Multivariate non-linear regression is based on multiple heating rates by making the assumption that the parameters of the model are identical for measurements at all heating rates [37]. Compared with the single curve analysis, the quality of fit diminishes considerably for the non-applicable reaction types [37]. So the distinguishing ability between the individual reaction types improves drastically. In addition, there are no limitations with respect to the complexity of the model, and consequently it can be applied to multi-step reactions. Nonlinear regression allows a direct fit of the model to the experimental data without a transformation, which would distort the error structure.

The basic hypothesis of this method is that the kinetic parameters, E and lg A, do not change with reaction rate α, therefore, we can use the hypothetical model to obtain the parameter values (E and lg A) from fitting the original data by multivariate non-linear regression method. Finally, the model, in which the correlation coefficient R is approximate to 1 and E values are comparable to those by FWO and KAS methods, is the most probable mechanism. The entire process, multivariate non-linear regression method, which is one of the multiple scanning methods, is discussed in this paper to obtain the most probable model one by one. As it is very hard to separate the thermal decomposition process of lanthanum oxalate decahydrate that has no obvious mass loss platform in five one-stage processes, the consecutive reaction model (A→B→C→D) by multivariate non-linear regression method was used for the determination of the most probable model to calculate the kinetic model of Table 4 [40,41]. The calculated values of E, lg A and kinetic model fitted for every stages are presented in Table 5. It can be seen that the thermal decomposition process of lanthanum oxalate  decahydrate is a kind of five-stage consecutive   reaction, which has the same reaction model and the dominant function of the possible mechanism is g(α)=[1-(1+α)^1/3]² which indicates that the thermolysis mechanism is three-dimensional spherical symmetry diffusion. The kinetic parameters of the first stage are E=65.47 kJ/mol, lg(A/s^-1)=-1.6; the second stage E= 106.9 kJ/mol, lg(A/s^-1)=-0.1; the third stage E=120.9 kJ/mol, lg(A/s^-1)=-0.183; the fourth stage E=177.68 kJ/mol, lg(A/s^-1)=2.27; the fifth stage E=156.4 kJ/mol,  lg(A/s^-1)=-2.54. The plots fitted with the best model are presented in Fig. 9. The average E-values matching  with the kinetic model are close to values obtained by FWO and KAS methods. This fitting accuracy is acceptable.

Table 4 Kinetic function of g(α) used for present analysis

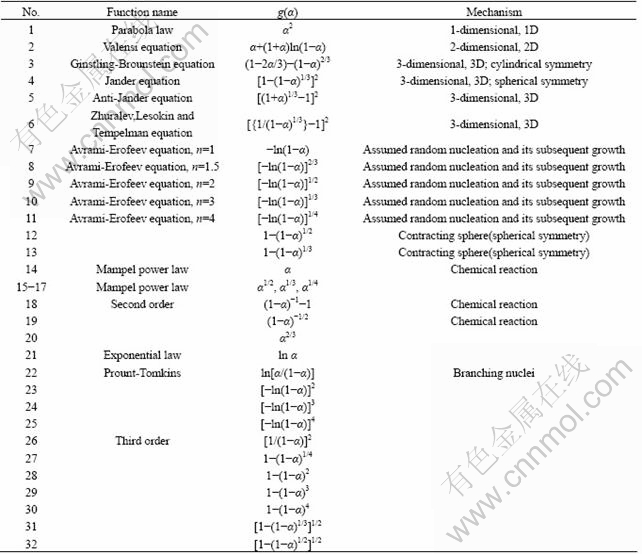
Table 5 Kinetic parameters by different mechanism

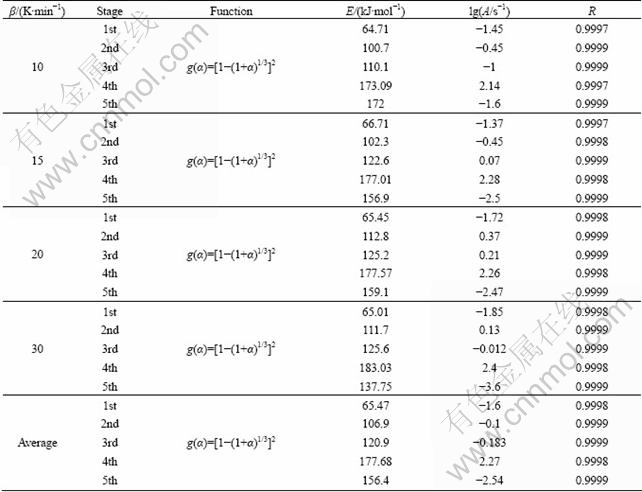
Fig. 9 Multiple non-linear regression results of TG curve: (a) Original TG curve; (b) Fitting TG curve

4 Conclusions

1) The thermal decomposition process of lanthanum oxalate decahydrate is a kind of five-stage consecutive reaction.

2) The activation energy values calculated by FWO method are close to the ones obtained by KAS method. E is depends on α, demonstrating that the decomposition reaction process of the lanthanum oxalate is of a complex kinetic mechanism.

3) For a multi-step reaction, the most probable model and the kinetic parameter should be obtained by multivariate non-linear regression method. The most probable mechanism function is g(α)=[1-(1+α)^1/3]². The kinetic parameters of the first stage are E=65.47 kJ/mol, lg(A/s^-1)=-1.6; the second stage E=106.9 kJ/mol, lg(A/s^-1)=-0.1; the third stage E=120.9 kJ/mol, lg(A/s^-1)=-0.183; the fourth stage E=177.68 kJ/mol, lg(A/s^-1)=2.27; the fifth stage E=156.4 kJ/mol, lg(A/s^-1)=-2.54. The fitting curve matches with the original TG curve very well.

References

[1] HU L, CHEN M. Preparation of ultrafine powder: The frontiers of chemical engineering [J]. Mater Chem Phys, 1996, 43(3): 212-219.

[2] WU X M, WANG L, TAN Z C, LI G H, QU S S. Preparation, characterization, and low-temperature heat capacities of nanocrystalline TiO₂ ultrafine powder [J]. J Solid State Chem, 2001, 156(1): 220-224.

[3] YAN D S. Synthesis and fabrication of nanostructured materials [J]. J Inorg Mater, 1995, 10(1): 1-6.

[4] MURUGAN V A, NAVALE S C, RAVI V. Synthesis of nanocrystalline La₂O₃ powder at 100 ℃ [J]. Mater Lett, 2006, 60(6): 848-849.

[5] BALBOUL B A A, EI-ROUDI A M, SAMIR E, OTHMAN A G. Non-isothermal studies of the decomposition course of lanthanum oxalate decahydrate [J]. Thermochim Acta, 2002, 387(2): 109-114.

[6] PETRYK J, KOLAKOWSKA E. Cobalt oxide catalysts for ammonia oxidation activated with cerium and lanthanum [J]. Appl Catal B, 2000, 24(2): 121-128.

[7] SCHWEIZER T, SAMSON B N, HECTOR J R, BROCKLESBY W S, HEWAK D W, PAYNE D N. Infrared emission from holmium doped gallium lanthanum sulphide glass [J]. Infrared Phys Techn, 1999, 40: 329-335.

[8] ESCUDERO M J, NOVOA X R, RODRIGO T, DAZA L. Influence of lanthanum oxide as quality promoter on cathodes for MCFC [J]. J Power Sources, 2002, 106(1-2): 196-205.

[9] CHEN Zhan-heng. Rare earth new materials and their application in the field of high technology [J]. Chinese Rare Earths, 2001, 21(1): 53-57. (in Chinese)

[10] ZHANG Ke-li, CHEN Xiong-bin, XI Mei-yun, SONG Li, ZHANG Xiao-feng, SUN Ju-tang. Thermal decomposition of lanthanum oxalate decahydrate [J]. J Wuhan Univ, 1996, 42(2): 163-166. (in Chinese)

[11] YANG Q, TANG K B, WANG C R, QIAN Y, ZHANG S. PVA-Assisted synthesis and characterization of CdSe and CdTe nanowires [J]. J Phys Chem B, 2002, 106(38): 9227-9230.

[12] BAKIZ B, GUINNETION F, ARAB M, BENLHACHEMI A, GAVARRIA J R. Elaboration, characterization of LaOHCO₃, La₂O₂CO₃ and La₂O₃ phases and their gas solid interactions with CH₄ and CO gases [J]. M J Condensed Matter, 2010, 12(1): 60-67.

[13] TANG J L, PAN T J, CHEN M. Synthesis of CdS nanoplates using poly (acrylic acid) as precursor by hydrothermal method [J]. Acta Chem Sinica, 2010, 68(4): 325-328.

[14] ZHANG H, YANG D R, LI S Z, JI Y J, MA X Y, QUE D L. Hydrothermal synthesis of flower-like Bi₂S₃ with nanorods in the diameter region of 30 nm [J]. Nanotechnology, 2003, 15(9): 1122-1125.

[15] REDDY B M, REDDY G K, KHAN A, GANESH I. Synthesis of monophasic Ce_0.5Zr_0.5O₂ solid solution by microwave-induced combustion method [J]. J Mater Sci, 2007, 42(10): 3557-3563.

[16] CHI Ru-an, XU Zhi-gao, WU Yuan-xin, WANG Cun-wen. Optimal conditions for preparing ultra-fine CeO₂ powders in a submerged circulative impinging stream reactor [J]. J Rare Earth, 2007, 25(4): 422-427.

[17] TANG W J, LIU Y W, YANG X, WANG C X. Kinetic studies of the calcination of ammonium metavanadate by thermal methods [J]. Ind Eng Chem Res, 2004, 43(9): 2054-2059.

[18] DOGANA F, AKATA H, KAYAB I. Synthesis, characterization and thermal degradation kinetics of poly (imino isophthaloyl imino (2,4,8,10-tetraoksoaspiro [5,5] undekan-3,9-dipropylene) [J] Chinese J Polym Sci, 2008, 26(1): 47-53.

[19] BROWN M E, MACIEJEWSKI M, VYAZOVKIN S, NOMEN R, SEMPERE J, BURNHAM A, OPFERMANN J, STREY R, ANDERSON H L, KEMMLER A, KEULEERS R, JANSSENS J, DESSEYN H O, LI C R., TANG T B, RODUIT B, MALEK J, MITSUHASHI T. Computational aspects of kinetics analysis, Part A: The ICTAC kinetics project-data, method and results [J]. Thermochim Acta, 2000, 355(1-2): 125-143.

[20] MACIEJEWSKI M. Computational aspects of kinetic analysis, Part B: The ICTAC kinetics project—The decomposition kinetics of calcium carbonate revisited, or some tips on survival in the kinetic minefield [J]. Thermochim Acta, 2000, 355(1-2): 145-154.

[21] VYAZOVKIN S. Computational aspects of kinetic analysis, Part C: The ICTAC kinetics project—The light at the end of the tunnel [J]. Thermochim Acta, 2000, 355(1-2): 155-163.

[22] BURNHAM A K. Computational aspects of kinetic analysis. Part D: The ICTAC kinetics project—Multi-thermal-history model-fitting methods and their relation to isoconversional methods [J]. Thermochim Acta, 2000, 355(1-2): 165-170.

[23] RODUIT B. Computational aspects of kinetic analysis. Part E: The ICTAC kinetics project—Numerical techniques and kinetics of solid state processes [J]. Thermochim Acta, 2000, 355(1-2): 171-180.

[24] ZHAN D, CONG C J, DIAKITE K, TAO Y, ZHANG K L. Kinetics of thermal decomposition of nickel oxalate dehydrate [J]. Thermochim Acta, 2005, 430(1-2): 101-105.

[25] HONG J H, YI T, MIN J, CONG C J, ZHANG K L. Preparation, thermal decomposition and lifetime of Eu(III)-phenanthroline complex doped xeroge [J]. Thermochim Acta, 2006, 440(1): 31-35.

[26] OPFERMANN J R, KAISERSBERGER E, FLAMMERSHEIM H J. Model free analysis of thermoanalytical data-advantages and limitations [J]. Thermochim Acta, 2002, 391(1-2): 119-127.

[27] JABER J O, PROBERT S D. Non-isothermal thermogravimetry and decomposition kinetics of two Jordanian oil shales under different processing conditions [J]. Fuel Process Technol, 2000, 63(1): 57-70.

[28] KHRASIHA Y H, SHABIB I M. Thermal analysis of shale oil using thermogravimetry and differential scanning calorimetry [J]. Energy Convers Manage, 2002, 43(2): 229-239.

[29] VYAZOVKIN S, WIGHT C A. Model-free and model-fitting approaches to kinetic analysis of isothermal and nonisothermal data [J]. Thermochim Acta, 1999, 340-341: 53-68.

[30] GAO Z, NAKADA M, AMASAKI I. A consideration of errors and accuracy in the isoconversional methods [J]. Thermochim Acta, 2001, 369(1-2): 137-142.

[31] TANG W J, LU Y W, ZHANG H, WANG C X. New approximate formula for Arrhenius temperature integral [J]. Thermochim Acta, 2003, 408(1-2): 39-43.

[32] GALWEY A K, BIOWN M E. Thermal decomposition of ionic solids [M]. Amsterdam: Elsevier, 1999.

[33] DIAKITE K, HONG J H, ZHAN D, ZHANG K L. Kinetics of the thermal decomposition of magnesium salicylate powder in air [J]. Chem Res Chinese U, 2006, 22(5): 617-620.

[34] GABAL M A. Kinetics of the thermal decomposition of CuC₂O₄-ZnC₂O₄ mixture in air [J]. Thermochim Acta, 2003, 199-208.

[35] CHEN Xiao-hu, WANG Hua, LIU Yi-min, FANG Min. Thermodynamic analysis of production of high purity titanium by thermal decomposition of titanium iodide [J]. Transactions of Nonferrous Metals Society of China, 2009, 19(5): 1348-1352.

[36] HU Rong-zu, SHI Qi-zhen. Thermal analysis kinetics [M]. Beijing: Science Press, 2001. (in Chinese)

[37] OPFERMANN J. Kinetic analysis using multivariate non-linear regression. I. Basic concepts [J]. J Therm Anal Calorim, 2000, 60(2): 641-658.

[38] OBAID A Y, ALYOUBI A O, SAMARKANDY A A, AL-THABAITI S A, AL-JUAID S S, EI-BELLIHI A A, EI-DEIFALLAH H M. Kinetics of thermal decomposition of copper(II) acetate monohydrate [J]. Therm Anal Calorim, 2000, 61(3): 985-994.

[39] BOONCHOM B, MAENSIRI S. Non-isothermal decomposition kinetics of NiFe₂O₄ nanoparticles synthesized using egg white solution route [J]. J Therm Anal Calorim, 2009, 97(3): 879-884.

[40] ZHANG K L, HONG J H, CAO G H, ZHAN D, TAO Y T, CONG C J. The kinetics of thermal dehydration of copper(II) acetate monohydrate in air [J]. Thermochim Acta, 2005, 437(1-2): 145-149.

[41] ZHANG J J, WANG R F, ZHAI X L, ZHAO J L, YANG H F, MO L P. Determination of mechanism function and kinetic parameters of thermal decomposition of the 2,2-dipyridine-tris(p-methoxybenzoate) europium(III) with non-isothermal TG and DTG curves [J]. Chinese J Inorg Chem, 2000, 16(1): 103-110.

水合草酸镧的热分解动力学

詹  光¹，余军霞¹，徐志高¹，周  芳²，池汝安¹

1. 武汉工程大学 化工与制药学院 绿色化工过程省部共建教育部重点实验室，

湖北省新型反应器与绿色化学工艺重点实验室，武汉 430074；

2. 中南大学 资源加工与生物工程学院，长沙 410083

摘  要：以PEG 20000为表面活性剂在撞击流反应器中制备La₂O₃超细粉体的前驱体十水草酸镧(La₂(C₂O₄)₃·10H₂O)。在室温至900 ℃下研究La₂(C₂O₄)₃·10H₂O的热分解过程，通过FTIR和DSC-TG对其反应中间物及最终固体产物进行分析。结果表明，该热分解过程由5个连续的反应阶段组成。采用Flynn-Wall-Ozawa (FWO)和Kissinger- Akahira-Sunose (KAS)法对活化能E进行求取，结果显示E值随着α的变化而变化，说明草酸镧的分解为复杂的热分解过程。采用多元非线性回归分析法对动力学方程和相关动力学参数进行拟合，得到动力学模型为G(α)=[1-(1+α)^1/3]²。采用该动力学模型求得的活化能平均值与采用FWO法和KAS法计算而得的活化能平均值十分接近，其拟合曲线与样品的热重分析曲线吻合。

关键词：十水草酸镧；TG-DSC；热分解；多元非线性拟合

 (Edited by LI Xiang-qun)

Foundation item: Project (IRT0974) supported by Program for Changjiang Scholars and Innovative Research Team in University, China; Project (50974098) supported by the National Natural Science Foundation of China

Corresponding author: CHI Ru-an; Tel: +86-27-87194980; Fax: +86-27-587195671; E-mail: rac@mail.wit.edu.cn

DOI: 10.1016/S1003-6326(11)61266-1