Dynamics of phase transformation of Cu-Ni-Si alloy with super-high strength and high conductivity during aging
LEI Qian(雷 前)1, LI Zhou(李 周)1, 2, PAN Zhi-yong(潘志勇)1,
WANG Ming-pu(汪明朴)1, 2, XIAO Zhu(肖 柱)1, CHEN Chang(陈 畅)1
1. School of Materials Science and Engineering, Central South University, Changsha 410083, China;
2. Key Laboratory of Nonferrous Metal Materials Science and Engineering of Ministry of Education,
Changsha 410083, China
Received 29 June 2009; accepted 25 August 2009
Abstract: The precipitation behaviors of the Cu-Ni-Si alloys during aging were studied by analyzing the variations of electric conductivity. The Avrami-equation of phase transformation kinetics and the Avrami-equation of electric conductivity during aging were established for Cu-Ni-Si alloys, on the basis of linear relationship between the electric conductivity and the volume fraction of precipitates, and the calculation results coincide well with the experiment ones. The transformation kinetics curves were established to characterize the aging process. The characteristics of precipitates in the supersaturated solid solution alloy aged at 723 K were established, and the results show that the precipitates are β-Ni3Si and δ-Ni2Si phases.
Key words: Cu; Cu-Ni-Si alloy; dynamics; phase transformation; precipitation; electrical conductivity
1 Introduction
Cu-Ni-Si alloys are used for electrical parts such as electrical connectors and lead-frames because of their high electrical conductivity and high strength[1]. The high strength is caused by nanometer-particle precipitates formed in the alloy during aging[2-3]. The electrical conductivity of the alloy in the aged state mainly depends on precipitating process[4-5]. There are many precipitates in form of δ-Ni2Si phase in the alloy during aging[6]. ZHAO et al[7] found out that when Cu-3.2Ni-0.75Si alloy was aged at 723 K after solution heat treatment, the modulated structure with Si-rich and Si-poor regions formed firstly, then the ordering (Cu, Ni)3Si nucleated from the modulated structure, and δ-Ni2Si precipitates formed at last with the increase of aging time. MONZEN et al[8] studied the microstructure and mechanical properties of Cu-Ni-Si alloys. And LOCKYER and NOBLE[9] claimed that the precipitate formed in Cu-2Ni-1Si (molar fraction, %) alloy had a structure corresponding to δ-Ni2Si, which was orthorhombic, and the precipitates formed in the early stages of aging had this structure (450 ℃, 1 h), as the precipitates did in the long-term aged alloys (1 000 h). GRYLLS and TUCK[10] believed that the δ-Ni2Si had a simple orthorhombic structure. Cu-Ni-Si alloy with super-high strength and high conductivity has a good prospect for replacing Cu-Be alloys in many applications [11-12]. The microstructure characteristics of Cu-Ni-Si alloys in the aging were experimentally studied by some researchers; however, there are few references to analyze the dynamics of phase transformation of Cu-Ni-Si alloy during aging.
Study on the transformation kinetics has an important effect for selection of the heat treatment process for the alloy to gain good properties. For this purpose, the kinetics equations of phase transformation and electrical conductivity equations of Cu-Ni-Si alloy were studied in this work. In addition, the microstructure of precipitates in aged Cu-Ni-Si alloys was investigated by transmission electron microscope.
2 Experimental
Three kinds of Cu-Ni-Si alloy ingots (Cu-5.2%Ni-1.2%Si, Cu-7.5%Ni-1.4%Si and Cu-8.0%Ni-1.8%Si, mass fraction) were prepared with medium-frequency induction furnace. Pre-specified amounts of Cu and Ni blocks were firstly melted in the furnace. Intermetallic Cu-Si master alloys of the required amounts were then added to the molten bath. The melting and casting operations were carried out in a N2 atmosphere to prevent the alloy from oxidizing. After surface defects were removed, the ingots were homogenized at 1 203 K for 24 h and subsequently rolled at 1 173 K, reducing the ingots thickness from 20 mm to 10 mm. The resultant strip was solution-treated at 1 243 K for 4 h in a N2+H2 atmosphere followed by water quenching. It was subsequently cold rolled with a 60% reduction in thickness (from 10 mm to 4 mm), and then aged at temperatures of 723 K and 773 K, respectively, immediately in a salt bath for various periods and water-cooled to room temperature. A series of samples (15 mm×15 mm×4 mm) were taken from the aged sheet for electrical conductivity analysis. The electrical conductivity of the samples aged under different conditions was measured by a digital eddy current tester. The microstructure of the samples taken from the aged sheet was observed with a Tecnai G220 transmission electron microscope under an operation voltage of 200 kV, and foil samples were reduced by an ion beam thinner.
3 Results and discussion
3.1 Calculation of volume fraction of precipitates of alloy
The electrical conductivity of the alloy in the aging state mainly depends on the purity of the copper matrix, the size and amount of the precipitates[13-14]. The precipitation of solute elements from the copper matrix through the formation of precipitates can increase the electrical conductivity of the alloy due to the decrease of scattering of electron in crystal cell. Electrical conductivity increases with prolonging the aged time. The dynamics of phase transformation can be characterized through investigating the variations of electrical conductivity during aging.
Part of solute atoms precipitate from the supersaturated solid solution during aging at a certain temperature[9]. The volume fraction of precipitates, φ, is expressed as
(1)
where V is the volume of formed precipitates in a unit volume during aging for a certain time;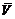 
is the balance volume of precipitates in a unit volume during aging at the same temperature for enough long time till the phase transformation finishes. After solution treatment, the alloy was aged immediately. Before aging treatment, both φ and V should be considered to be zero (V=0, φ=0), and the electrical conductivity of alloy is σ0. After enough long time, the phase transformation process ended, and it is reasonable to think V as 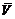and the φ as one (V=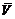, φ=1), and the electrical conductivity of alloy is σmax. σ and φ have a linear relationship according to Martition’s law, which can be expressed as
(2)
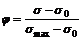(3)
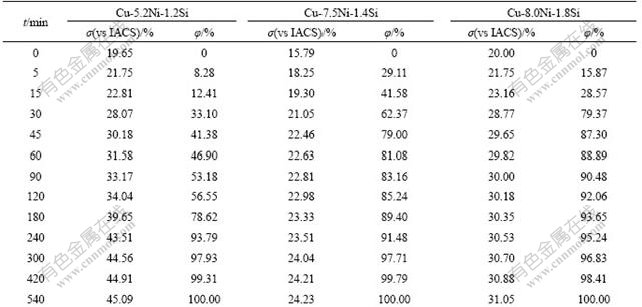
Therefore, φ can be obtained by measuring the electrical conductivity of alloy during aging for different time. The electrical conductivity and precipitates volume fraction of Cu-Ni-Si alloy aged at 723 K and 773 K for different time are shown in Table 1 and Table 2, respectively.
Table 1 Electrical conductivity (σ) and volume fraction of precipitates (φ) of Cu-Ni-Si alloys aged at 723 K for different time
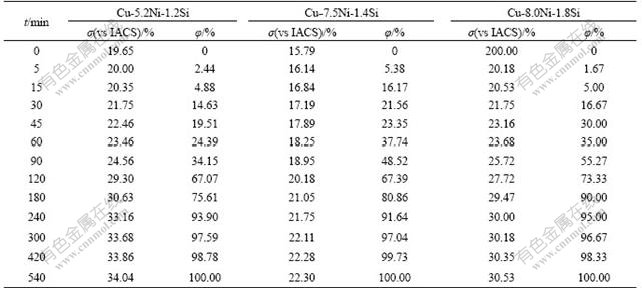
Table 2 Electrical conductivity (σ) and volume fraction of precipitates (φ) of Cu-Ni-Si alloys aged at 773 K for different time
3.2 Kinetics Avrami-equations of phase transformation and Avrami-equations of electrical conductivity
The kinetics Avrami-equations of phase transformation can be expressed as follows[15]:
(4)
where φ is volume fraction of precipitates; t is aging time, b and n are constants. b depends on the temperature of phase transformation, composition of supersaturated solid solution and size of crystal grain. n depends on the type of phase transformation and nucleation location. According Eq.(4), Eq.(2) can be expressed as
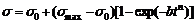(5)
where the Eq.(5) is the electrical conductivity equation.
Eq.(4), after being transposed, can be expressed as
(6)
By taking the nature logarithm of the equation in both sides, Eq.(6) can be expressed as
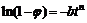(7)
After being transposed, Eq.(7) can be expressed as
(8)
Finally, by taking common logarithm of the equation in both sides, Eq.(8) can be expressed as
(9)
The relationship between lg[ln1/(1-φ)] and lgt is shown in Fig.1 and it is approximately a straight line. The n is the slope coefficient of the line and the lgb is the intercept.
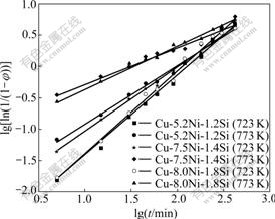
Fig.1 Relation between lg[ln1/(1-φ)] and lgt of alloys aged at 723 K and 773 K
The approximate values of n and b can be deduced from the straight line in Fig.1. Table 3 lists the values of A(=σmax-σ0), n and b.
Table 3 Values of A, n and b of alloys aged at 723 K and 773 K
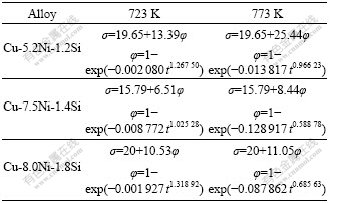
Both the kinetic Avrami-equation of phase transformation and the Avrami-equation of electrical conductivity for the alloys aged at 723 K and 773 K are expressed, respectively, in Table 4.
Table 4 Avrami-equation of electrical conductivity and kinetic Avrami-equation of phase transformation of alloys aged at 723 K and 773 K
The measured electrical conductivity and calculated ones of the alloys aged at 723 K and 773 K, respectively, for different time are shown in Fig.2. Most of the errors between the curves and symbols are less than 5%, which are in the acceptable scope. The calculation results coincide well with the experiment ones.
Fig.2 Experimental and calculated electrical conductivities of alloy aged at 723 K and 773 K
3.3 Kinetic curves of phase transformation of Cu-Ni- Si alloys during aging
The kinetic curves of phase transformation of the alloy, on the basis of equations in Table 4, are shown in Fig.3. The volume fractions of precipitates are low as the alloys are aged at 723 K and 773 K, respectively, in the early aging stage. The needed time that the volume fraction of precipitates approaches 50% of each alloy aged at 723 K and 773 K, respectively, are different. For Cu-5.2Ni-1.2Si alloy, the aging time that the volume fraction of precipitates approached 50% is about 100 min at 723 K and 70 min at 773 K; for Cu-7.5Ni-1.4Si alloy, the needed aging time is about 92 min at 723 K and 24 min at 773 K; while for Cu-8.0Ni-1.8Si alloy, the needed aged time is about 80 min at 723 K and 20 min at 773K.
Fig.3 Kinetic curves of phase transformation of alloys aged at 723 K and 773 K: (a) Cu-5.2Ni-1.2Si; (b) Cu-7.5Ni-1.4Si; (c) Cu-8.0Ni-1.8Si
Therefore, the needed aging time that the volume fraction of precipitates approach 50% decreases with increasing the saturation degree of solution as the alloys are aged at the same temperature. In addition, the higher the aging temperature is, the earlier the precipitation transformation begins. After being aged for 10 min at 723 K, the volume fractions of the three kinds of alloys are below 10%, while the volume fractions are beyond 10% when these alloys are aged for 10 min at 773 K.
Both electrical conductivity and volume fraction increase with the solute atoms precipitating from supersaturated solid solution in form of precipitates during aging. A long time later, however, the density of solute atoms decreases, so that both the rate of transformation and electrical conductivity tend to keep constant.
3.4 Microstructure of precipitates
The micrographs and the characteristics of the precipitate of the Cu-8.0Ni-1.8Si alloy aged at 723 K for different time have been investigated by TEM. The typical TEM micrographs of precipitates in the alloys during aging at 723 K for different time are shown in Fig.4. Both the volume fraction and the size of precipitates increase with the increase of aging time[16]. The diffraction patterns of this alloy aged at 723 K for 480 min, corresponding to Fig.4(c), is shown in Fig.4(d). The analysis of the diffraction pattern is shown in Fig.4(e). The precipitates have a structure corresponding to orthorhombic δ-Ni2Si and simple cubic β-Ni3Si[17-18].
Fig.4 Type TEM images of thin foil samples aged at 723 K for different time: (a) 30 min; (b) 90 min; (c) 480 min; (d) Selected field diffraction pattern corresponding to (c); (e) Analysis of diffraction pattern
4 Conclusions
1) The Avrami-equation of phase transformation kinetics and the Avrami-equation between electric conductivity and aging time are established for three typical Cu-Ni-Si alloys on the basis of the linear relationship between the electric conductivity and the volume fraction of precipitations. And the calculation results coincide well with the experimental ones.
2) The precipitates formed in Cu-Ni-Si alloys during aging at 723 K have structures corresponding to δ-Ni2Si and β-Ni3Si by the analysis of selected field diffraction pattern. The volume fraction of precipitates increases with increasing the aging time.
3) Kinetics curves of phase transformation of three kinds of Cu-Ni-Si alloys are established.
References
[1] CORSON M G. Electrical conductor alloys [J]. Electrical World, 1927, 89: 137-139.
[2] FUJIWARA H, KAMIO A. Effect of alloy composition on precipitation behavior in Cu-Ni-Si alloys [J]. Journal of the Japan Institute of Metals, 1998, 62: 301-309.
[3] SUN Z, LAITEM C, VINCENT A. Dynamic embrittlement at intermediate temperature in a Cu-Ni-Si alloy [J]. Materials Science and Engineering A, 2008, 447: 145-152.
[4] SUZUKI S, SHIBUTANI N, MIMURA K, ISSHIKI M, WASEDA Y. Improvement in strength and electrical conductivity of Cu-Ni-Si alloys by aging and cold rolling [J]. Journal of Alloys and Compounds, 2006, 417: 116-120.
[5] NAGAYOSHI H, NISHIJIMA F, WATANABE C, MONZEN R, HARA T. Bend formability and microstructure in a Cu-4 mass%Ni- 1mass%Si-0.02 mass%P alloy [J]. Journal of the Japan Institute of Metals, 2006, 70: 750-755.
[6] MONZEN R, WATANABE C. Microstructure and mechanical properties of Cu-Ni-Si alloys [J]. Materials Science and Engineering A, 2008, 483: 117-123.
[7] ZHAO Dong-mei, DONG Qi-ming, LIU Ping, KANG Bu-xi, HUANG Jin-liang, JIN Zhi-hao. Structure and strength of the age hardened Cu-Ni-Si alloy [J]. Materials Chemistry and Physics, 2003, 79: 81-89.
[8] WATANABE C, HIRAIDE H, ZHANG Z G, MONZEN R. Microstructure and mechanical properties of Cu-Ni-Si alloys [J]. Journal of the Society of Materials Science Japan, 2005, 54(7): 717-723. (in Japanese)
[9] LOCKYER S A, NOBLE F W. Precipitate structure in a Cu-Ni-Si alloy [J]. Journal of Materials Science, 1994, 29: 218-226.
[10] GRYLLS R J, TUCK C D S. Identification of orthorhombic phase in a high strength cupronickel [J]. Scripta Materialia, 1996, 43: 121-126.
[11] LI Zhou, PAN Zhi-yong, ZHAO Yu-yuan, XIAO Zhu, WANG Ming-pu. Microstructure and properties of high conductivity, super high strength Cu-8.0Ni-1.8Si-0.6Sn-0.15Mg alloy [J]. Journal of Material Research, 2009, 24: 2123-2128.
[12] LONG Yong-qiang, LIU Ping, LIU Yong, ZHANG Wei-min, PAN Jian-sheng. Simulation of recrystallization grain growth during re-aging process in the Cu-Ni-Si alloy based on phase field model [J]. Materials Letters, 2008, 62: 3039-3042.
[13] HUANG Fu-xiang, MA Ju-sheng, NING Hong-long, CAO Yu-wen, GENG Zhi-ting. Precipitation in Cu-Ni-Si-Zn alloy for lead frame [J]. Materials Letters, 2003, 57: 2135-2139.
[14] DUMANSKA M T, ZIEBA P, PAWLOSKI A, WOJEWADA J, GUST W. Practical aspects of discontinuous precipitation and dissolution [J]. Materials Chemistry and Physics, 2003, 80: 476-481.
[15] CAHN R W. Physical metallurgy [M]. 4th Ed. CAHN R W, HAASEN P. Holland: Elsevier Publishing, 1996.
[16] PAN Zhi-yong, WANG Ming-pu, LI Zhou, DENG Chu-ping, XIAO Zhu, CHEN Chang. Thermomechanical treatment of super high strength Cu-5.2Ni-1.2Si alloy [J]. The Chinese Journal of Nonferrous Metals, 2007, 17(11): 1821-1826. (in Chinese)
[17] ROBERTSON W D, GRENIER E G, NOLE V F. Institute of metals division—The structure and associated properties of an age hardening copper alloy [J]. Transactions of the Metallurgical Society of AIME, 1961, 221: 503-513.
[18] PAN Zhi-yong, WANG Ming-pu, LI Zhou, XIAO Zhu, CHEN Chang. Thermomechanical treatment of supper high strength Cu-8.0Ni-1.8Si alloy [J]. Transactions of Nonferrous Metals Society of China, 2007, 17(s1): s1076-s1080.
Foundation item: Project(2006AA03Z517) supported by the National High-tech Research and Development Program of China; Project(08MX15) supported by the Mittal Programs of Central South University, China
Corresponding author: LI Zhou; Tel: +86-731-88830264; E-mail: lizhou6931@163.com
DOI: 10.1016/S1003-6326(09)60249-1
(Edited by YANG Bing)
