J. Cent. South Univ. (2017) 24: 533-545

DOI: 10.1007/s11771-017-3456-2

Modeling and verification of comprehensive errors of real-time wear-depth detecting for spherical plain bearing tester

LI Wei(李巍), HU Zhan-qi(胡占齐), YANG Yu-lin(杨育林), FAN Bing-li(范兵利), ZHOU Hai-li(周海丽)

Aviation Key Laboratory of Science and Technology on Generic Technology of

Aviation Self-Lubricating Spherical Plain Bearing, Yanshan University, Qinhuangdao 066004, China

Central South University Press and Springer-Verlag Berlin Heidelberg 2017

Abstract: Because of various error factors, the detecting errors in the real-time experimental data of the wear depth affect the accuracy of the detecting data. The self-made spherical plain bearing tester was studied, and its testing principle of the wear depth of the spherical plain bearing was introduced. Meanwhile, the error factors affecting the wear-depth detecting precision were analyzed. Then, the comprehensive error model of the wear-depth detecting system of the spherical plain bearing was built by the multi-body system theory (MBS). In addition, the thermal deformation of the wear-depth detecting system caused by varying the environmental temperature was detected. Finally, according to the above experimental parameters, the thermal errors of the related parts of the comprehensive error model were calculated by FEM. The results show that the difference between the simulation value and the experimental value is less than 0.005 mm, and the two values are close. The correctness of the comprehensive error model is verified under the thermal error experimental conditions.

Key words: spherical plain bearing tester; self-lubricating spherical plain bearing; wear depth; multi-body system theory; comprehensive error model; thermal error

1 Introduction

The self-lubricating spherical plain bearings are the special journal bearings which inlay or bond the self-lubricating solid materials between the inner and outer races. These bearings have some special characteristics, such as maintenance-free, non- lubricating, and low coefficient of friction. Therefore, the self-lubricating spherical plain bearings are widely applied to the aviation and aerospace fields [1, 2].

In the process of operating the spherical plain bearings, the safety of aircrafts is affected by the tribological properties and the life of the bearings. So, the performance of the spherical plain bearings should be evaluated by the spherical plain bearing testers. Meanwhile, three performance parameters of the spherical plain bearings (the wear depth, the friction torque and the friction temperature) need to be detected in real time. Particularly, the wear depth is the most evident parameter for the tribological properties and the life of the self-lubricating spherical plain bearings. However, due to the various error factors, such as the thermal errors, the loading errors and the fluctuating error. They all have an effect on the detecting precision of the wear depth. How to reduce the error factors of the bearing tester has important implications for the evaluation of the self-lubricating spherical plain bearings.

At present, the error compensation technology has been deeply studied in CNC and CMM (Coordinate Measuring Machine), yet few researches in the spherical plain bearing testers. Meanwhile, the software compensating methods were mainly used to analyze and compensate the errors [3-5]. In addition, there were mainly two mathematic modeling methods for the comprehensive error, namely, the multi-body system theory and the homogeneous coordinates transformation. And more use of them was the multi-body system theory. For example, ZHONG et al [6] presented a comprehensive geometric error modeling by the rigid multi-body system for the large 5-axis machining center prototype. ZHU et al [7, 8] presented an integrated geometric error modeling and a workpiece locating error modeling by the multi-body system for the CNC machine tool. KONG et al [9, 10] presented an integrated kinematics error model for the ultra-precision machining. CUI et al [11, 12] built an comprehensive geometric error modeling by the multi-body system theories for the CNC machine tool. CHEN et al [13] established the synthesis error model for the grinder by the multi-body system theory and the D-H homogeneous transformed matrix. FAN et al [14] presented the volumetric error modeling by the multi-body system for the CNC machine tool. ZHANG [15] built a general model for the positioning errors of NC machine tools by the kinematics of the multi-body system.

Based on the self-made self-lubricating spherical plain bearing tester, in this work, the error factors affecting the wear-depth detection precision are analyzed. Simultaneously, the comprehensive error model of the wear-depth detecting system of the spherical plain bearing is built by the MBS. Finally, according to the experimental parameters of the thermal error of the wear-depth detecting system, the correctness of the comprehensive error model is verified by FEM under the thermal error experimental conditions.

2 Wear-depth detecting principle and error factor analysis of spherical plain bearing tester

The self-made self-lubricating spherical plain bearing tester is composed of six modules, namely, the transmission module, the hydraulic loading module, the bearing fixture module, the wear-depth detecting module, the environmental simulation module and the control module. The picture of the spherical plain bearing tester is shown in Fig. 1.

Fig. 1 Picture of self-lubricating spherical plain bearing tester

Firstly, the radial wear-depth direction of the spherical plain bearing (the detecting direction of the displacement sensor) is chosen as the Z-axis direction, and the moving direction of the outer ring of the worn bearing is defined as the positive direction of the Z axis. Meanwhile, the axial direction of the spherical plain bearing (the axial direction of the testing spindle) is chosen as the Y-axis direction, and the axial assembling direction of the testing spindle is defined as the positive direction of the Y axis. Then, the X axis can be identified by the right-hand rule. Finally, we define the rotation axes revolving around respectively X axis, Y axis and Z axis as A axis, B axis and C axis.

In the bearing fixture module, the spherical plain bearing is put into the T-type plate, and the testing spindle passes through the bearing inner race. In wear-depth detecting module, the displacement sensor is fixed on the detecting frame, and the contact clamp of the displacement sensor is fastened to the long pole. Meanwhile, the measuring head of the displacement sensor is pressed onto the contact clamp, and the upper end of the long pole touches with the bottom of the T-type plate by means of the spring force.

In the wear experiment, the wear of the spherical plain bearing leads to moving down of the T-type plate, the downward displacement is the wear depth of the spherical plain bearing. Meanwhile, the long pole moves down with the T-type plate, the contact clamp of the displacement sensor, fixed on the long pole, declines and presses down the measuring head of the displacement sensor, and at the moment the displacement sensor detects the wear depth of the spherical plain bearing. The schematic diagram of the wear detecting system, the combination of the bearing fixture module and the wear detecting module, is shown in Fig. 2.

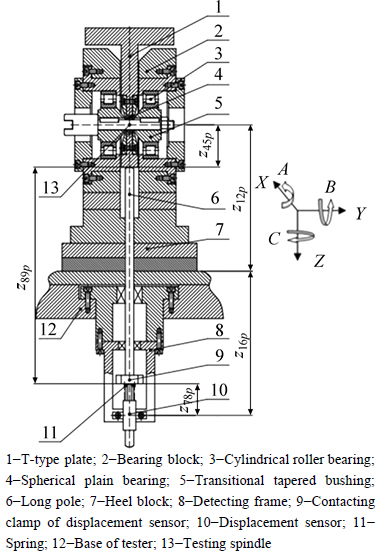
Fig. 2 Schematic diagram of wear-depth detecting system:

In the experiment process of the spherical plain bearing, there are five error factors giving rise to the detecting error, namely, the thermal errors △e^T, the loading errors △e^F, the fluctuating error △e^V, the positioning errors △e^G and the detecting interference errors △e^I. The schematic diagram of the error analysis is shown in Fig. 3.

Fig. 3 Schematic diagram of error analysis of spherical plain bearing tester

1) Thermal errors △e^T

Because of the varying environmental temperatures caused by the day and night alternate, the heat dissipation of the hydraulic pressure station and the friction heat of the bearings, the temperature field of the tester changes in the experiment, giving rise to the thermal deformation of the wear-depth detecting system.

2) Loading errors △e^F

According to the experimental outline of the spherical plain bearing, there are experimental requirements of the variable loading in addition to the constant loading, such as the loading form of the sine curve or the trapezoidal curve. So, the wear-depth detecting system generates the corresponding deformation with the changing loading.

3) Fluctuating error △e^V

Thanks to the uneven loading of the drive system and the vibration of the hydraulic system, the bearing fixture module creates the random fluctuation in the experiment, affecting the stability of the real-time wear-depth detecting data.

4) Position errors △e^G

Because of the manufacture errors or the assembly errors of the parts, there is the deviation between the actual geometrical parameters and the ideal them, so the detecting direction of the displacement sensor is not parallel with the moving direction of the worn bearing.

5) Detecting interference errors △e^I

Owing to the electromagnetic interference and the unstable dynamic characteristics of the sensor, there is the high fluctuation on the real-time wear-depth detecting curve. The fluctuation affects the correctness of the data reading of the wear depth.

3 Multi-body comprehensive error modeling of spherical plain bearing tester

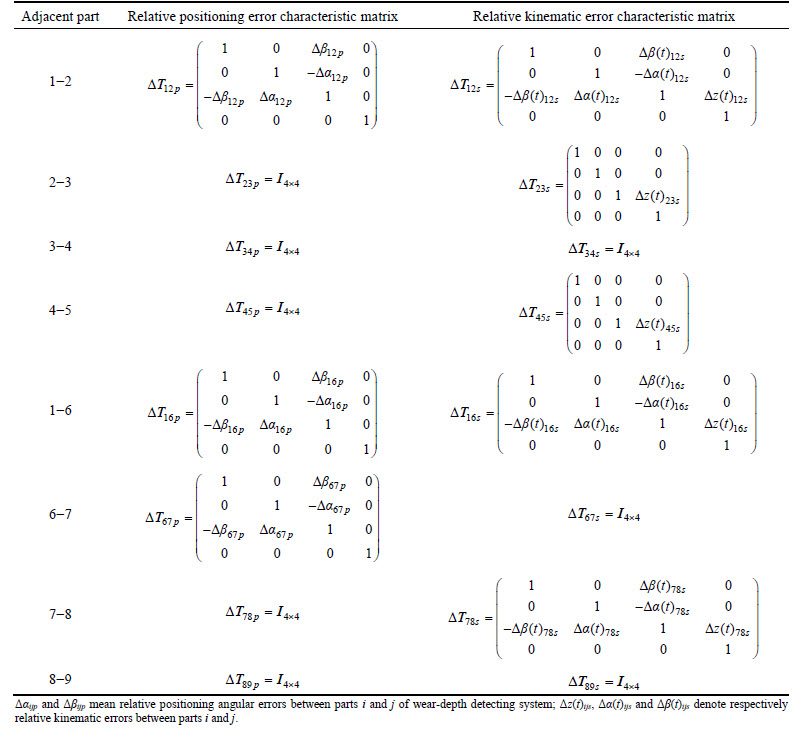
Firstly, the wear-depth detecting system of the spherical plain bearing tester is simplified as two branches. The adjacent parts of the first branch, using the base of the tester as the starting point, is numbered according to the ascending order, and the sequence of the first branch is the base of the tester (1), the bearing block (2), the testing spindle (3), the spherical plain bearing (4), the T-type plate (5). The adjacent parts of the second branch is numbered as the first branch, and the sequence is the base of the tester (1), the detecting frame (6), the displacement sensor (7), the contacting clamp of displacement sensor (8), the long pole (9). The topological structure diagram of the wear-depth detecting system of the spherical plain bearing tester is shown in Fig. 4.

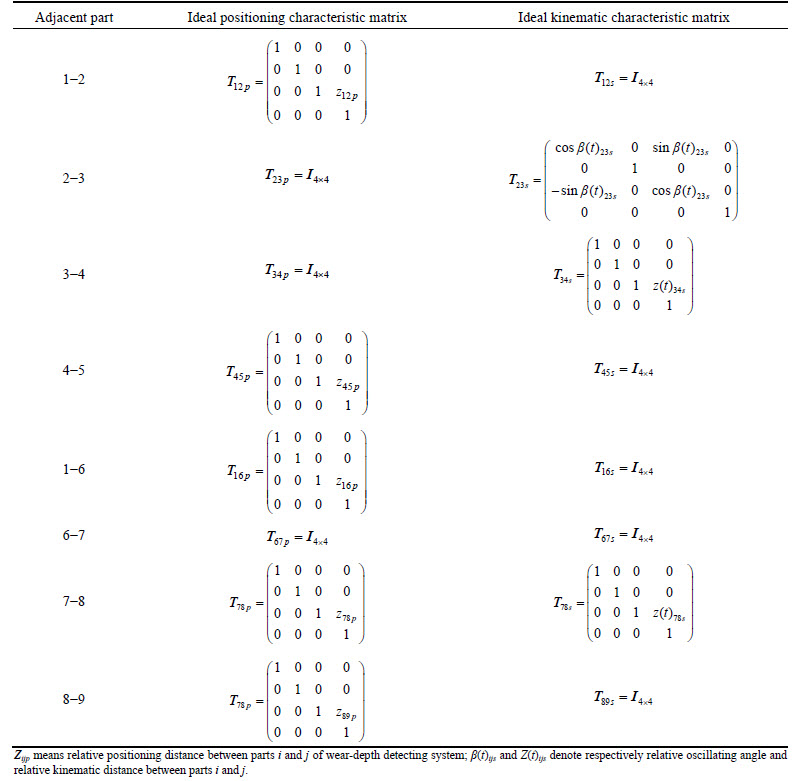
Combined the above topological structure diagram of the wear-depth detecting system with the definition of the low-order body [16], the lower order array is obtained and given in Table 1.

On the basis of the detecting principle of the real- time wear-depth detection and the schematic diagram of the relative position of the wear-depth detecting system, the ideal positioning characteristic matrices T_ijp and the ideal kinematic characteristic matrices T_ijs are given in Table 2.

Because of the various error factors under the actual condition, the positional accuracy and the kinematic accuracy of the each parts of the wear-depth detecting system are affected. With the analysis of the above errors,the relative positioning error characteristic matrices △T_ijp and the relative kinematic error characteristic matrices △T_ijs are given in Table 3.

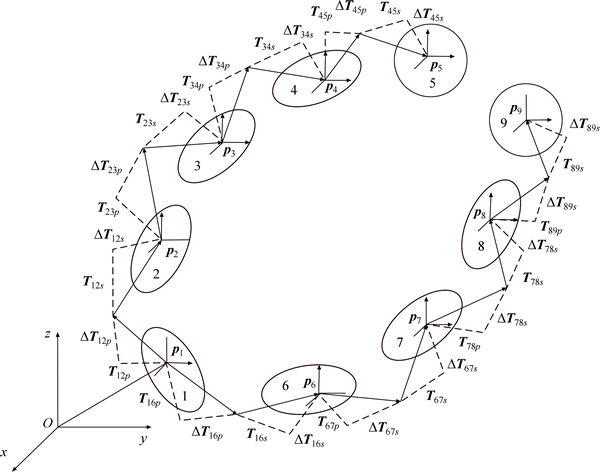
Fig. 4 Topological structure diagram of wear-depth detecting system of spherical plain bearing tester

Table 1 Lower body array of wear-depth detecting system of spherical plain bearing tester

From the above topological structure diagram of the wear-depth detecting system, in order to analyze the distances or errors of the two branch ends, the reference coordinate systems of the T-type plate (5) and the long pole (9) need to transform to the reference coordinate system of the base of the tester. In the following, the expression formulas of the reference coordinate systems of the two branch ends in the base of the tester are established under the actual condition.

Assuming that the coordinate of the origin of the T-type plate (p₅=[x₅, y₅, z₅, 1]^T) in the reference coordinate system of the base of the tester is p_1-5=[x_1-5, y_1-5, z_1-5, 1]^T, the expression of the first branch is shown below by the low-order body formula [16].

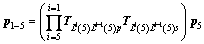
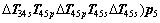            (1)

From Tables 2 and 3, the displacement expression of the first branch in the detecting direction of the displacement sensor (the Z axis) is shown as

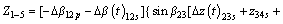
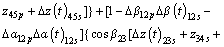
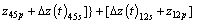       (2)

The Eq. (2), by ignoring the higher order terms, is also shown as

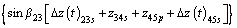  (3)

So, the error 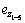of the first branch along the Z axis is

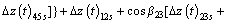
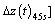                            (4)

Table 2 Positioning and kinematic characteristic matrices of each parts of wear-depth detecting system

Similarly, by assuming that the coordinate of the origin of the long pole (p₉=[x₉, y₉, z₉, 1]^T) in the reference coordinate system of the base of the tester is p_1-9=[x_1-9, y_1-9, z_1-9, 1]^T, the expression of the second branch is shown as

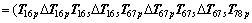
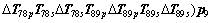     (5)

The displacement expression of the second branch in the detecting direction of the displacement sensor is shown as

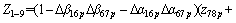
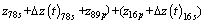       (6)

The Eq. (6), by ignoring the higher order terms, is also shown as

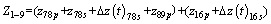  (7)

So, the error 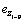of the second branch along the Z axis is

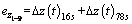                       (8)

From the above error analysis, the total errors e_z of the wear-depth detecting system in the detecting direction of the displacement sensor are

Table 3 Relative positioning and kinematic error characteristic matrices of each parts of wear-depth detecting system

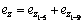
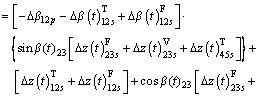
     (9)

4 Thermal error detection of spherical plain bearing tester

The statistical analysis showed that the thermal errors caused by the temperature change could account for over 50% of the total errors in the detecting equipment [17]. In the wear experiment of the spherical plain bearing, the thermal deformation caused by the varying environmental temperatures affected the detection precision of the wear-depth detecting system. In what follows, we design the experiment that detects the thermal deformation of the wear-depth detecting system.

As shown in Fig. 2, the bearing fixture module assembled by the self-lubricating spherical plain bearing was placed on the base of the tester, so that the top of the long pole tightly touched with the bottom of the T-type plate under the spring force. Then, the displacement sensor was adjusted and fixed, so that there was pre-pressing quantity between the displacement sensor and the contacting clamp of the displacement sensor. Without the start-up of the transmission module, there was no relative movement between the inner and outer races of the spherical plain bearing. And in opening embedded hydraulic pressure station, there was no applied load to the spherical plain bearing.

Seven temperature sensors, collecting the varying temperature of the wear-depth detecting system, were pasted on the different locations. The distribution diagrams and the pictures of these seven temperature sensors on the wear-depth detecting system are shown in Fig. 5.

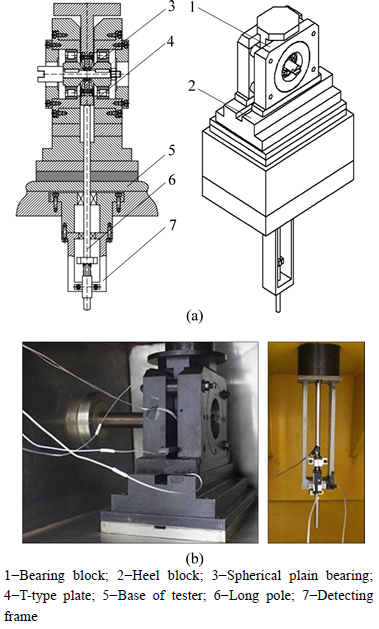
Fig. 5 Schematic diagrams (a) and pictures (b) of temperature- sensor distribution position on wear-depth detecting system:

At the same time, a temperature sensor collecting the room temperature was suspended near the life tester. During the wear experiment, the corresponding temperature changing curves of the eight temperature sensors are shown in Fig. 6, and the thermal deformation curve of the wear-depth detecting system along the Z axis is shown in Fig. 7.

As shown in Figs. 6 and 7, the total time of the experiment is 480 min, and the temperature of the eight temperature sensors gradually rises. The temperature rise levels of the eight detecting points are: the bearing block 6.5 °C, the heel block 5.8 °C, the spherical plain bearing 3.2 °C, the T-type plate 6.3°C, the base of the tester 6 °C, the long pole 9.6 °C, the detecting frame 8.9 °C and the room temperature 8.9 °C. Meanwhile, the initial indicating value of the displacement sensor is 4.805, and the final value is 4.784. So, the thermal deformation of the wear-depth detecting system along the Z axis is approximately 0.021 mm, meanwhile, the show values of the displacement sensor gradually decrease with the rising of the temperature.

Fig. 6 Temperature changing curves of eight detecting points on wear-depth detecting system

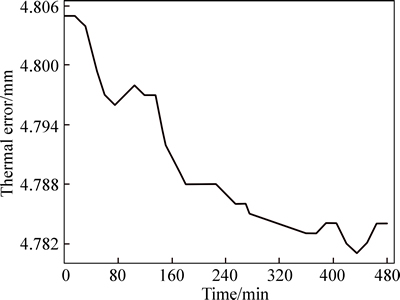
Fig. 7 Curve of thermal errors of wear-depth detecting system along Z-coordinate axis

5 Verification of comprehensive error model of spherical plain bearing tester

In the thermal error experiment, there is no relative movement between the inner and outer races of the spherical plain bearing without the start-up of the transmission module, so the oscillating angleand the fluctuating error △e^V both are zero. Meanwhile, there is no applied load to the spherical plain bearing, so the loading error △e^F is zero. The Eq. (9) of the total errors of the wear-depth detecting system becomes

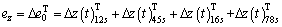 (10)

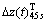As shown the Eq. (10), the total thermal errors of the wear-depth detecting system include the thermal error between the bearing block and the base of the tester, the thermal error between the T-type plate and the spherical plain bearing, the thermal error 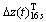between the detecting frame and the base of the tester and the thermal error between the displacement sensor and the contacting clamp. Now the above thermal errors are calculated by FEM, and then the calculation results compare with the experimental data. The correctness of the comprehensive error model is verified under the thermal error experimental conditions.

1) Thermal error between bearing block and base of tester

The thermal error was equivalent to the distance between the inner ring of the cylindrical roller bearing and the base along the Z axis, and the calculation of the error involved three parts, that is, the bearing block, the cylindrical roller bearing and the heel block. Firstly, the three dimensional models of the above parts were built by the SoildWorks software. In order to simplify calculation, these models ignored the cage of the roller bearing, the bolts, the mounting holes, the chamfers, etc. The bearing designation and the main dimensions of the models are given in Table 4. Then, the models were imported into the ANSYS software, and the calculation, considering the symmetry of the models, used the half of the models. Meanwhile, the structural element Solid 186 was adopted and freely meshed, and the grids of the contact surfaces of the rolling elements and raceway of the cylindrical roller bearing were refined. So, the number of elements was 78535, and the number of nodes was 102765. The meshed model of the thermal error is shown in Fig. 8.

The contact problem of the rolling elements and raceways of the cylindrical roller bearing selected the “face-to-face” contacting form. The surface of the rolling element was the rigid target surface, and the surface of the raceway was the flexible contact surface. Meanwhile, the parameters of the contact surfaces were normal penalty stiffness (FKN) of 1, tangent penalty stiffness (FKT) of 1, μ of 0.2, initial contact tolerance (ICONT) of 0.01 and penetration tolerance (FTOLN) of 0.1. The required parameters of FEM calculation for the thermal errors are given in Table 5.

Table 4 Cylindrical roller bearing designation and main dimensions of model (mm)

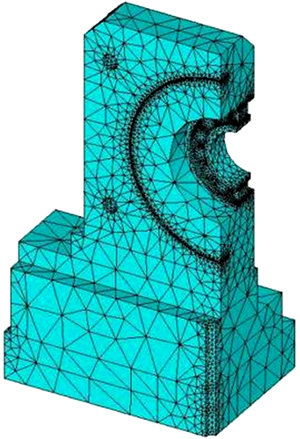
Fig. 8 Meshed model of thermal errors

Owing to ignoring the cage of the roller bearing in the model, the X axis and the Y axis directions of the rolling elements were fixed using the displacement constraint. Meanwhile, the bearing fixture module was fastened on the base of the tester, so the bottom of the heel block was fixed using the displacement constraint. According to the above thermal experiment, the temperature rise of the room temperature, the bearing block and the heel block were respectively 8.9, 6.5 and 5.8 °C, so the thermal loadings were applied on these parts. Then, the schematic diagram of the thermal errors between the bearing block and the base of the tester is shown in Fig. 9.

Table 5 Required parameters of FEM calculation for thermal errors

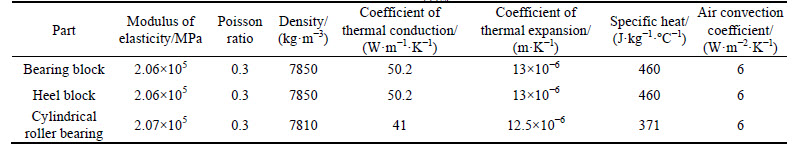
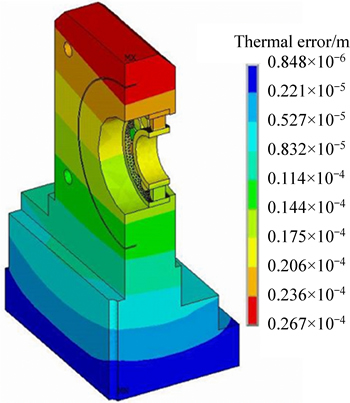
Fig. 9 Simulation results of thermal errors between bearing block and tester base

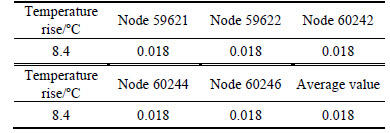
As shown in Fig. 9, the inner ring of the cylindrical roller bearing moves up (the negative direction of the Z axis) with the rising of the environmental temperature. The rising distance and the average value of the five nodes of the center line of the lower surface of the cylindrical inner ring (the node 59621, the node 59622, the node 60242, the node 60244 and the node 60246) are given in Table 6.

Table 6 Rising distance and average value of five nodes on center line of lower surface of cylindrical roller bearing (mm)

2) Thermal error 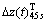between T-type plate and spherical plain bearing

The thermal error 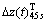was equivalent to the distance between the bottom of the T-type plate and the base along the Z axis, and the calculation of the error 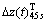involved five parts, that is, the T-type plate, the testing spindle, the spherical plain bearing, the transitional tapered bushing and the inner ring of the roller bearing. Firstly, the three dimensional models of the above parts were built by the SoildWorks software. In order to simplify calculation, these models ignored the front-end thread of the testing spindle, the keyways, the threaded holes and the chamfers. The designation of the spherical plain bearing and the main dimensions of the models are given in Table 7.

Then, the models were imported into the ANSYS software, and the calculation, considering the symmetry of the models, used the half of the models. Meanwhile, the structural element Solid 186 was adopted and freely meshed. The number of elements was 132838, and the number of nodes was 203646. The meshed model of the thermal error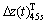is shown in Fig. 10, and the required parameters of FEM calculation for the thermal errors 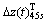are given in Table 8.

Table 7 Spherical plain bearing designation and main dimensions of model (mm)

Fig. 10 Meshed model of thermal errors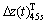

Because of the supporting role of the cylindrical roller bearing for the testing spindle and the transitional tapered bushing, the lower surface of the inner ring of the roller bearing was fixed using the displacement constraint. According to the above thermal experiment, the temperature rise of the room temperature, the T-type plate and the spherical plain bearing were respectively 8.9, 6.3 and 3.2 °C, so the thermal loadings were applied on these parts. Then, the schematic diagram of the thermal error 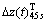between the T-type plate and the spherical plain bearing is shown in Fig. 11.

As shown in the Fig. 11, the bottom of the T-type plate moves down (the positive direction of the Z axis) with the rising of the environmental temperature. The falling distance of the central node of the bottom of the T-type plate (the contacting point of the T-type plate and the long pole) is 0.003 mm.

3) Thermal error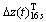between detecting frame and base of tester

The thermal error 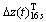was equivalent to the distance between the fixing position of the displacement sensor on the bottom of the detecting frame and the base along the Z axis. Firstly, the three dimensional model of the frame was built by the SoildWorks software. In order to simplify calculation, the model ignored the linear bearing, the threaded holes, the mounting holes, the chamfers, etc. The length and width of the detecting frame were about 362 and 50 mm, and then the model was imported into the ANSYS software. Meanwhile, the structural element Solid 186 was adopted and freely meshed. The number of elements was 7921, and the number of nodes was 14525. The meshed model of the thermal error 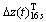is shown in Fig. 12. The material and the required parameters of FEM calculation for the thermal errors 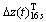are given in Table 9.

Table 8 Required parameters of FEM calculation for thermal errors

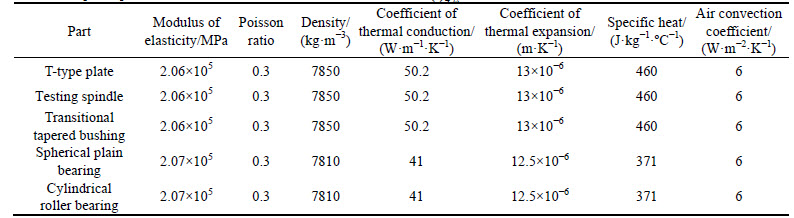
Fig. 11 Simulation results of thermal errors 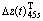between T-type plate and spherical plain bearing

As shown in Fig. 2 of the wear-depth detecting system, the top of the detecting frame was inset and fixed in the base of the tester, so the upper surface of the detecting frame was fixed using the displacement constraint. According to the above thermal experiment, the temperature rise of the room temperature, the base of the tester and the bottom of the detecting frame were respectively 8.9, 6 and 8.9 °C, so the thermal loadings were applied on these parts. Then, the schematic diagrams of the thermal error 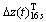between the detecting frame and the base of the tester is shown in Fig. 13.

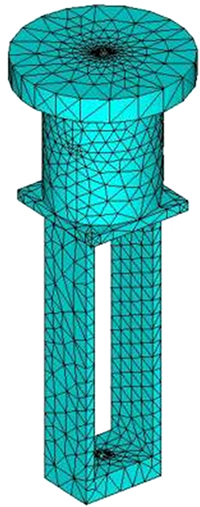
Fig. 12 Meshed model of thermal errors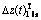

As shown in Fig. 13, the bottom of the detecting frame moves down with the rising of the environmental temperature. The falling distance of the central node of the fixing position of the displacement sensor is 0.041 mm.

4) Thermal error between displacement sensor and contacting clamp of displacement sensor

The thermal error 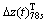was equivalent to the distance between the lower surface of the contacting clamp and the upper surface of the long pole along the Z axis, and the calculation of the error 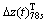involved two parts, that is, the long pole and the contacting clamp of displacement sensor. Firstly, the three dimensional models of the two parts were built by the SoildWorks software. In order to simplify calculation, the models ignored the threaded holes, the mounting holes and the chamfers. The length and diameter of the long pole were about 730 mm and 10 mm, respectively, and then the models were imported into the ANSYS software. Meanwhile, the structural element Solid 186 was adopted and freely meshed. The number of elements was 240394,and the number of nodes was 350388. The meshed model of the thermal error is shown in Fig. 14. The materials and the required parameters of FEM calculation for the thermal errors 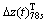are given in Table 10.

Table 9 Model material and required parameters of FEM calculation for thermal errors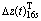

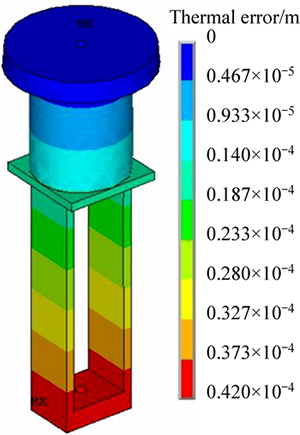
Fig. 13 Simulation results of thermal errors 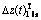between detecting frame and tester base

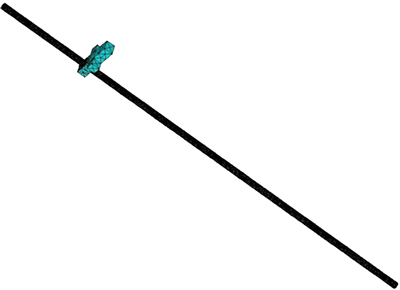
Fig. 14 Meshed model of thermal errors

As shown in Fig. 2 of the wear-depth detecting system, the long pole, passing through the detecting frame, the base of the tester and the heel block, contacted the bottom of the T-type plate, so the upper surface of the long pole was fixed using the displacement constraint. According to the above thermal experiment, the temperature rise of the room temperature, the base of the tester, the heel block and the bottom of the long pole were respectively 8.9, 6, 5.8 and 9.6 °C, so the thermal loadings were applied on these parts. Then, the schematic diagrams of the thermal error between the detecting frame and the base of the tester is shown in Fig. 15.

As shown in Fig. 15, the bottom of the long pole moves down with the rising of the environmental temperature, and the falling distance of the central node of the lower surface of the contacting clamp (the contacting point of the displacement sensor and the contacting clamp) is 0.04 mm.

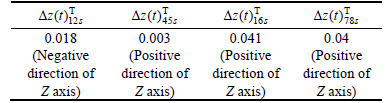
Form Eq. (10), the total thermal errors of the wear- depth detecting system are composed of the thermal error , the thermal error 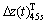, the thermal error 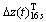and the thermal error . The above four FEA results of the thermal errors are given in Table 11.

From Table 11 and the wear-depth detecting principle of the spherical plain bearing tester, the thermal deformation direction of the bearing block is opposite to that of the T-type plate in the bearing fixture module, and the rising distance of the bearing block is larger than the falling distance of the T-type plate (>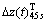). So, the long pole and the contacting clamp of displacement sensor move up along the Z axis by thespring force, and the rising displacement is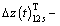 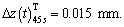Then, the contacting clamp keeps away from the displacement sensor. The measuring head of the displacement sensor relaxes gradually, and the displacement curve shows the downward trend. Meanwhile, the thermal deformation direction of the detecting frame is same as the long pole, so the thermal deformation direction of the displacement sensor fixed on the bottom of the detecting frame is same as the contacting clamp fixed the bottom of the long pole. The thermal deformation of the detecting frame and the long pole cancels each other out, and the residual thermalerror is 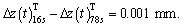The measuring head of the displacement sensor relaxes gradually, and the displacement curve shows the downward trend. So, the total thermal deformation of the wear-depth detecting system is 0.016 mm, and the experimental value differs by 0.005 mm from the FEA result (the experimental value of the thermal error is 0.021 mm). All in all, the simulation value is closer to the experimental value, which also verifies the correctness of the comprehensive error model under the thermal error experimental conditions.

Table 10 Model material and required parameters of FEM calculation for thermal errors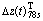

Fig. 15 Simulation results of thermal errors between displacement sensor and fixing clamp of displacement sensor

Table 11 Summary of relative thermal errors of each parts of wear-depth detecting system (mm)

6 Conclusions

Based on the self-made self-lubricating spherical plain bearing tester, the comprehensive error model of the wear-depth detecting system of the spherical plain bearing is built by the multi-body system theory. The thermal deformation of the wear-depth detecting system caused by varying the environmental temperature is detected, and the thermal deformation along the Z axis is 0.021 mm when the temperature rise of the room temperature is 8.9 °C. Meanwhile, the values of the displacement sensor gradually decrease with the rising of the temperature. Finally, the thermal errors of the wear-depth detecting system are calculated by FEA according to the above experimental parameters. By comparison, the difference between the simulation value and the experimental value is less than 0.005 mm, and the two values are close. The correctness of the comprehensive error model is verified under the thermal error experimental conditions.

References

[1] SLINEY H E. Some load limits and self-lubricating properties of plain spherical bearings with molded graphite fiber reinforced polyimide liners to 320 °C [R]. USA: NASA-Lewis Research Center, 1978: 1-13.

[2] PETER H. Helicopter application puts ceramic-coated spherical plain bearings through their paces [R]. UK: SKF-AMPEP PLC, 2005: 1-6.

[3] CHEN J S. Neural network-based modelling and error compensation of thermally-induced spindle errors [J]. International Journal of Advanced Manufacturing Technology, 1996, 12(4): 303-308.

[4] WANG Kun-chieh, TENG Pai-chung, LIN Kuo-ming. Thermal error modeling of a machining center using grey system theory and adaptive network-based fuzzy inference system [J]. JSME International Journal: Series C, Mechanical Systems, Machine Elements & Manufacturing, 2007, 49(4): 1179-1187.

[5] YAN J Y, YANG J G. Application of synthetic grey correlation theory on thermal point optimization for machine tool thermal error compensation [J]. International Journal of Advanced Manufacturing Technology, 2009, 43(11, 12): 1124-1132.

[6] ZHONG Gao-yan, WANG Chao-qun, YANG Shou-feng. Position geometric error modeling, identification and compensation for large 5-axis machining center prototype [J]. International Journal of Machine Tools & Manufacture, 2015, 89: 142-150.

[7] ZHU Shao-wei, DING Guo-fu, QIN Sheng-feng. Integrated geometric error modeling, identification and compensation of CNC machine tools [J]. International Journal of Machine Tools & Manufacture, 2012, 52(1): 24-29.

[8] ZHU S W, DING G F, MA S W. Workpiece locating error prediction and compensation in fixtures [J]. The International Journal of Advanced Manufacturing Technology, 2012, 67(5-8): 1423-1432.

[9] KONG L B, CHEUNG C F, TO S. A kinematics and experimental analysis of form error compensation in ultra-precision machining [J]. International Journal of Machine Tools & Manufacture, 2008, 48: 1408-1419.

[10] KONG L B, CHEUNG C F. Prediction of surface generation in ultra-precision raster milling of optical freeform surfaces using an integrated kinematics error model [J]. Advances in Engineering Software, 2012, 45(1): 124-136.

[11] CUI Gang-wei, LU Yong, GAO Dong. A novel error compensation implementing strategy and realizing on Siemens 840D CNC systems [J]. International Journal of Advanced Manufacturing Technology, 2012, 61(5-8): 595-608.

[12] CUI Gang-wei, LU Yong, LI Jian-guang. Geometric error compensation software system for CNC machine tools based on NC program reconstructing [J]. International Journal of Advanced Manufacturing Technology, 2012, 63(1-4): 169-180.

[13] CHEN Shu-han, YAN Hong-zhi, MING Xing-zu. Analysis and modeling of error of spiral bevel gear grinder based on multi-body system theory [J]. Journal of Central South University of Technology, 2008, 15(5): 706-711.

[14] FAN J W, GUAN J L, WANG W C. A universal modeling method for enhancement the volumetric accuracy of CNC machine tools [J]. Journal of Materials Processing Technology, 2002, 129(1-3): 624-628.

[15] ZHANG Qing. Study on the compensation technique of positioning errors for NC machine tools [J]. Journal of Tianjin University, 1998, 4(2): 184-187.

[16] JOSEPHS H R. Dynamics of mechanical systems [M]. New York: CRC Press, 2000: 605-613.

[17] GUPTA V, BASTIAS P C. Thermal-mechanical modelling of the rolling-plus-sliding with frictional heating of a locomotive wheel [J]. Journal of Manufacturing Science & Engineering, 1995, 117(3): 418-422.

(Edited by YANG Bing)

Cite this article as: LI Wei, HU Zhan-qi, YANG Yu-lin, FAN Bing-li, ZHOU Hai-li. Modeling and verification of comprehensive errors of real-time wear-depth detecting for spherical plain bearing tester [J]. Journal of Central South University, 2017, 24(3): 533-545. DOI: 10.1007/s11771-017-3456-2.

Foundation item: Project(2014E00468R) supported by Technological Innovation Fund of Aviation Industry Corporation of China

Received date: 2015-09-21; Accepted date: 2016-03-22

Corresponding author: HU Zhan-qi, Professor, PhD; Tel: +86-13933660163; E-mail: ronghu118@163.com