J. Cent. South Univ. (2017) 24: 1654-1662
DOI: 10.1007/s11771-017-3571-0
Thermophoresis and concentration effects in a fourth grade peristaltic flow with convective walls
Ali Aamir1, Asghar S2, 3, Awais M1
1. Department of Mathematics, COMSATS Institute of Information Technology, Attock 43600, Pakistan;
2. Department of Mathematics, COMSATS Institute of Information Technology, Islamabad 44000, Pakistan;
3. Department of Mathematics, King Abdulaziz University, Jeddah 21432, Saudi Arabia
 Central South University Press and Springer-Verlag Berlin Heidelberg 2017
Central South University Press and Springer-Verlag Berlin Heidelberg 2017
Abstract: In this investigation, we have studied the peristaltic fluid flow in an asymmetric channel with convective walls. Fourth grade fluid model has been utilized in view of the fact that the results of all other differential type models can be deduced as the special case. Combined effects of heat and mass transfer are considered. The thermophoresis effects occur in the energy equation. Convective heat and mass boundary conditions have been given special attention. Long wave length and low Reynolds number approximations are utilized for the simplifications. Approximate analytic solutions for the velocity, temperature and concentration profiles are calculated using perturbation technique and elaborated in the form of graphical observations for various physical quantities.
Key words: fourth grade fluid; convective boundary conditions; pumping and trapping; peristalsis
1 Introduction
In nature variety of liquids exist and all have quite different and interesting rheological properties. Most of these have complex characteristics and do not obey the famous Navier-Stokes equations. In view of this fact, several researchers and scientists proposed various mathematical models in order to analyze and predict the rheological flow properties. In general the fluids are classified into three main type namely the differential, rate and integral. There is one subclass of differential type fluids (the fourth grade fluid model) on which the recent researcher and mathematicians have showed interest to tackle and study under different flow situations. For-instance, HAYAT et al [1] presented a note on some solutions for the flow of a fourth grade fluid in a porous space, and considered the time independent flow in a porous half space and utilize the modified Darcy’s law in mathematical modelling. They developed the travelling wave and conditional symmetry solutions. NADEEM and ALI [2] computed the analytical solutions for the pipe flow of a fourth grade fluid model with Reynold and Vogel’s models of viscosities. Incompressible fourth grade fluid down a vertical cylinder is considered and heat transfer is analyzed for the variable viscosity case. The obtained results are compared with finite difference and perturbation solutions in the limiting sense. An optimal homotopy asymptotic method applied to the steady flow of a fourth-grade fluid past a porous plate is presented by MARINCA et al [3]. In this analysis, authors have presented a new analytical method for the nonlinear problems namely the optimal homotopy asymptotic method (OHAM). The series solutions are developed and recurrence relations are given explicitly. AZIZ and MAHMOOD [4] presented reductions and solutions for the unsteady flow of a fourth grade fluid on a porous plate, and performed the symmetry reductions to transform the governing nonlinear partial differential equations into ordinary differential equations and presented the analytic and numerical solutions. Effects of rotation and initial stress on peristaltic transport of fourth grade fluid with heat transfer and induced magnetic field has been discussed by ABDALLA et al [5]. Constitutive equations obeying the fourth grade fluid model are employed and the assumptions of long wavelength and low Reynolds number are utilized in computing the solutions. Steady state flow for inside porous flat channel using analytical solution to the fourth grade fluid has been investigated by EBRAHIMPOUR et al [6]. They have considered the fourth grade fluid inside porous flat channel with monotonous injection and suction rates. AZIZ et al [7] computed the invariant solutions for the unsteady hydro-magnetic (MHD) flow of a fourth grade fluid induced due to the impulsive motion of a flat porous plate. NAZ et al [8] investigated the flow of fourth grade fluid in a porous medium. They have computed the fourth grade fluid dynamics and studied the heat transfer analysis between two channel walls.
The aim of current work is to venture further in the regime of fourth grade fluid model. Therefore, we have extended the rheology of fourth grade fluid model into new direction. We have considered convective heat and mass transfer analysis in a peristaltic flow of a fourth grade fluid. We have also considered the effects of magnetic field into the system. Long wave length and low Reynold’s number approximations are utilized. Stream line analysis is presented showing the behavior of bolus under various rheological situations.
2 Problem formulation
We consider the flow of an incompressible magnetohydrodynamic non-Newtonian fourth grade fluid in an asymmetric channel (see Fig. 1). We consider 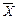 axis along and
axis along and 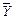 axis perpendicular to the channel walls. The fluid is electrically conducting through an applied magnetic field strength B0 in the direction perpendicular to the flow. The flow is induced due to the sinusoidal waves which are of the form:
axis perpendicular to the channel walls. The fluid is electrically conducting through an applied magnetic field strength B0 in the direction perpendicular to the flow. The flow is induced due to the sinusoidal waves which are of the form:
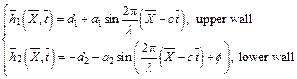 (1)
(1)
where a1 and a2 represent the amplitudes; λ is the wavelength; φ is the phase difference having range 0≤φ≤π. It is pointed out that φ=0 represents symmetric channel with wave out of phase and φ=π represents that the waves are in phase.
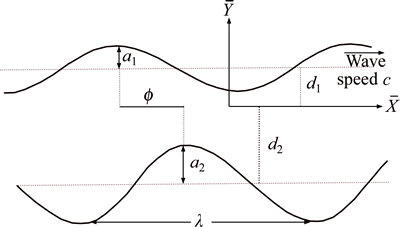
Fig. 1 Geometry of problem
The equations that govern the flow of an incompressible fluid of fourth grade with heat and mass transfer in the presence of magnetic field are:
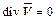 (2)
(2)
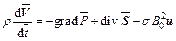 (3)
(3)
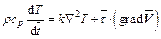 (4)
(4)
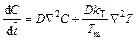 (5)
(5)
where 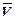 is the velocity; ρ is the density;
is the velocity; ρ is the density; 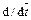 is the material time derivative; B0 is the magnetic field strength; T is the fluid temperature; cp is the specific heat capacity, k is the thermal conductivity of the material;
is the material time derivative; B0 is the magnetic field strength; T is the fluid temperature; cp is the specific heat capacity, k is the thermal conductivity of the material; 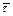 is the Cauchy stress tensor for fourth grade fluid; C is the fluid concentration; Tm is the mean temperature; kT is the thermal diffusion ratio; and D is the coefficient of mass diffusivity. The heat and mass transfer due to convective boundaries yields:
is the Cauchy stress tensor for fourth grade fluid; C is the fluid concentration; Tm is the mean temperature; kT is the thermal diffusion ratio; and D is the coefficient of mass diffusivity. The heat and mass transfer due to convective boundaries yields:
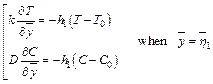 (6)
(6)
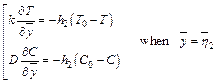 (7)
(7)
where h1 and h2 denote the heat and mass transfer coefficients at the upper and lower walls, respectively. Moreover, T0 and C0 are the ambient temperature and concentration at the channel walls. The velocity vector 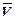 is given by
is given by
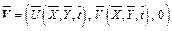 (8)
(8)
The following quantities are introduced to transform the system from laboratory frame to wave frame:
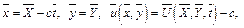
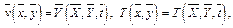
 (9)
(9)
Introducing the following dimensionless variables and making use of the stream function, we get the following equations in simplified form after using the low Reynolds number and long wave length approximations:
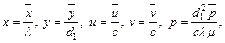
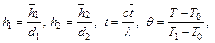
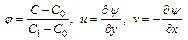 (10)
(10)
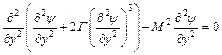 (11)
(11)
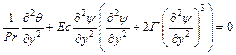 (12)
(12)
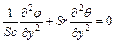 (13)
(13)
where the dimensionless parameters, the Hartman number M, the Prandtl number Pr, the Eckert number Ec, the Schmidt number Sc and the Soret number Sr are defined respectively as follows:
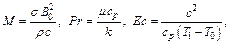
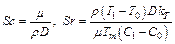 (14)
(14)
The dimensionless walls conditions are given by
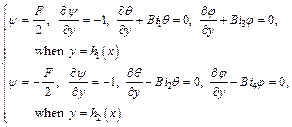 (15)
(15)
where 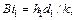
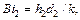
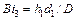 and
and 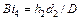 are Biot numbers which are dimensionless and the dimensionless time-mean flows in the laboratory and wave frames are:
are Biot numbers which are dimensionless and the dimensionless time-mean flows in the laboratory and wave frames are:
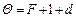 (16)
(16)
with
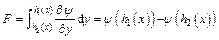 (17)
(17)
where the dimensionless forms of hi (i=1, 2) are:
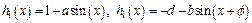 (18)
(18)
in which 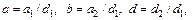 and φ satisfies the following relation:
and φ satisfies the following relation:
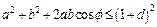 (19)
(19)
3 Computations
It is noted that Eqs. (11)-(13) along with prescribed wall conditions (15) are coupled and highly nonlinear. Therefore, we employed perturbation approach to compute the approximate solution for velocity, temperature and concentration profiles. The physical quantities are perturbed in the following way:
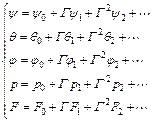 (20)
(20)
1) Zeroth order system:
By using the above expansions (20) into Eqs. (11)– (13) and the boundary conditions (15), collecting the terms of Γ0 we have:
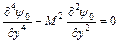 (21)
(21)
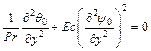 (22)
(22)
 (23)
(23)
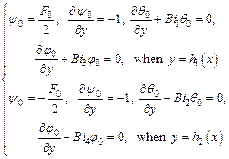 (24)
(24)
Equations (21)-(23) are linear differential equations with constant coefficients which can be solved easily. The solutions of Eqs. (21)–(23) satisfying the boundary conditions (24) are given by
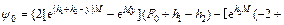
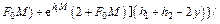
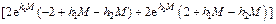 (25)
(25)
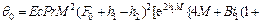
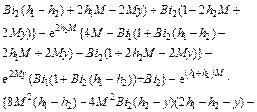
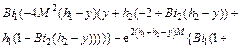
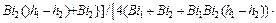
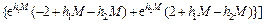 (26)
(26)
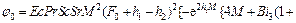
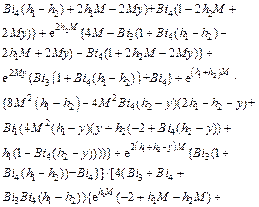
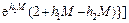 (27)
(27)
2) First order system
In order to proceed for the first order system we use expansions (20) into Eqs. (11)–(13) and the boundary conditions (15), collecting the terms of Γ1 we have:
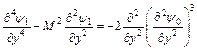 (28)
(28)
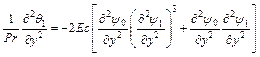 (29)
(29)
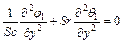 (30)
(30)
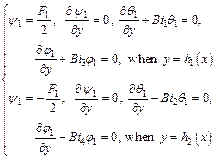 (31)
(31)
Equations (28)-(30) are non-homogeneous linear differential equations with constant coefficients which can be solved using the boundary conditions (31). The expressions for the solution are:
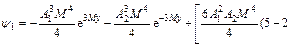
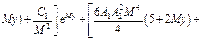
 (32)
(32)
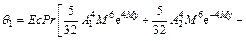
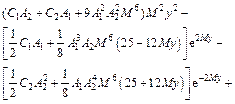
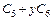 (33)
(33)
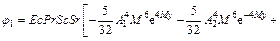
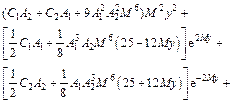
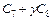 (34)
(34)
where Ai (i=1 … 2) and Ci (i=1 … 8) are integration constants whose values are given in appendix.
4 Results and discussion
In this section we have discussed the effects of different physical parameters on the pressure gradient, velocity profile, temperature profile and concentration profile. The effects of fourth grade parameter Γ, and phase difference φ on the pressure gradient dp(1)/dx with x for some fixed values of other parameters (a=0.7, b=1.2, d=2, Θ=3 and M=1) are shown in Figs. 2(a) and (b) respectively. We can observe a rise in the peak of pressure gradient by increasing the values of Γ and decrease by increasing values of φ. The pressure gradient for fourth grade fluid is higher in magnitude as compared to the Newtonian fluid i.e. Γ=0. From Fig. 2(b) we observe that the pressure gradient decreases in magnitude with an increase in phase difference φ.
The effects of fourth grade parameter Γ, and Hartmann number M on the axial velocity are shown in Figs. 3(a) and (b) for fixed values of other parameters (a=0.3, b=0.6, d=0.9, Θ=1.2, φ=π/4 and x=-π/3). As the fourth grade parameter is taken as the perturbation parameter so effects for small values of Γ are analyzed. We observe that by increasing values of Γ the velocity decreases at the boundaries of the channel while it increases at the centre of the channel, i.e. fluid offers less resistance in terms of small viscous effects. The opposite effects are analyzed in the case of Hartmann number, i.e. the magnitude of the velocity increases at the boundaries and decreases at the centre of the channel by increasing values of Hartmann number.
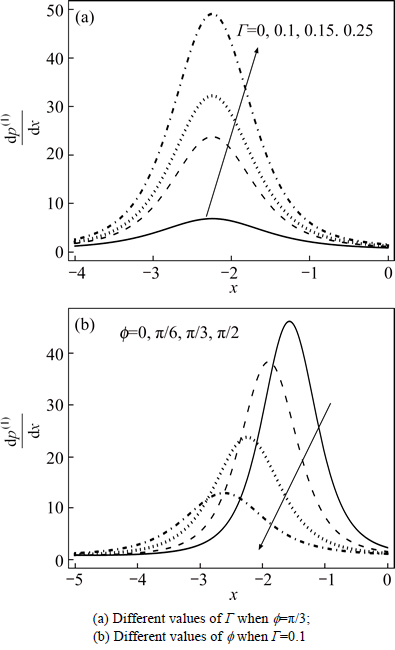
Fig. 2 Pressure gradients
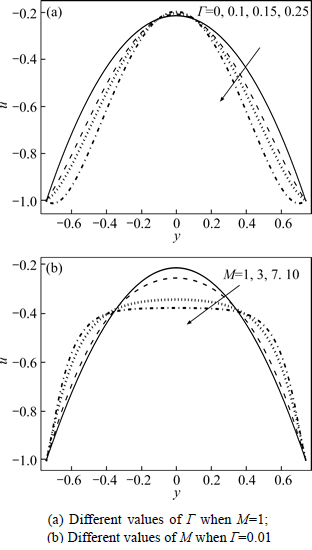
Fig. 3 Velocity profiles
The effects of fourth grade parameter Γ on the temperature are shown in Fig. 4 when a=0.3, b=0.5, d=0.9, Θ=0.5, φ=π/4, x=-π/3, M=1, Pr=1, Ec=1, Bi1=10 and Bi2=10. We have observed that the temperature profile decreases by increasing values of Γ since Γ is a non-Newtonian fluid parameter causing a reduction on molecular motion due to higher viscosity.
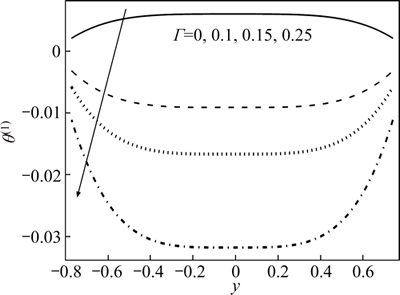
Fig. 4 Temperature profile for different values of Γ
The effects of Biot numbers Bi1 and Bi2 on temperature profile are shown in Figs. 5(a) and (b) when a=0.3, b=0.5, d=0.9, Θ=0.7, φ=π/4, x=-π/3, M=1, Pr=1, Ec=1 and Γ=0.01. We have observed that the temperature profile decreases by increasing values of Bi1 and Bi2 which are considered much larger than unity due to non-uniformity of the temperature fields within the fluid.
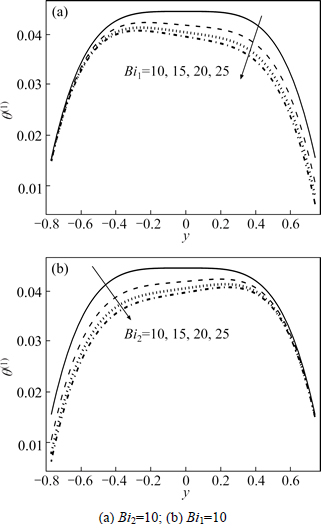
Fig. 5 Effects of Biot number on temperature profile
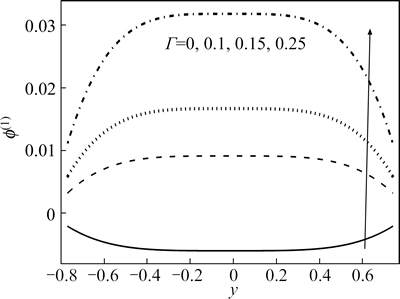
Fig. 6 Concentration profile for different values of Γ
The effects of fourth grade parameter Γ on the concentration profile are shown in Fig. 6 when the values of the parameters are a=0.3, b=0.5, d=0.9, Θ=0.5, φ=π/4, x=-π/3, M=1, Pr=1, Ec=1, Sr=1, Sc=1, Bi1=10, Bi2=10, Bi3=10 and Bi4=10. It is observed that the concentration profile increases when we increase the values of Γ.
The effects of Biot numbers Bi3 and Bi4 on concentration profile are shown in Figs. 7(a) and (b) when the values of other parameters are a=0.3, b=0.5, d=0.9, Θ=0.5, φ=π/4, x=-π/3, M=1, Γ=1, Pr=1, Ec=1, Sr=1, Sc=1, Bi1=10 and Bi2=10. We have observed that the concentration profile increases for increasing values of Bi3 and Bi4.
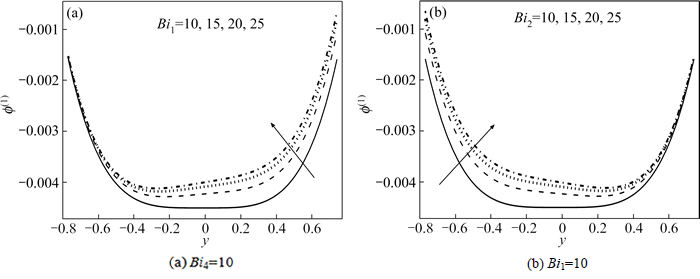
Fig. 7 Effects of Biot number on concentration profile: (a) Bi4=10; (b) Bi3=10
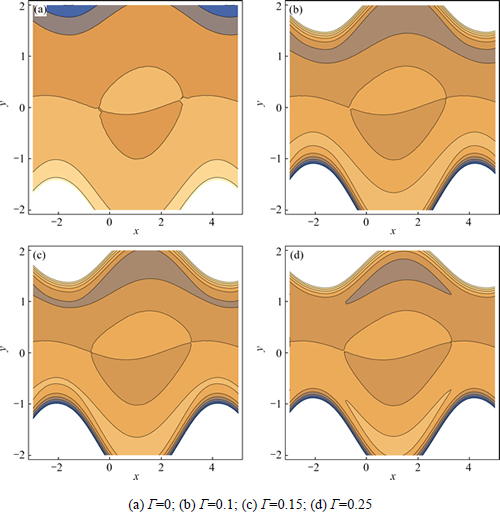
Fig. 8 Effects of Γ on streamlines
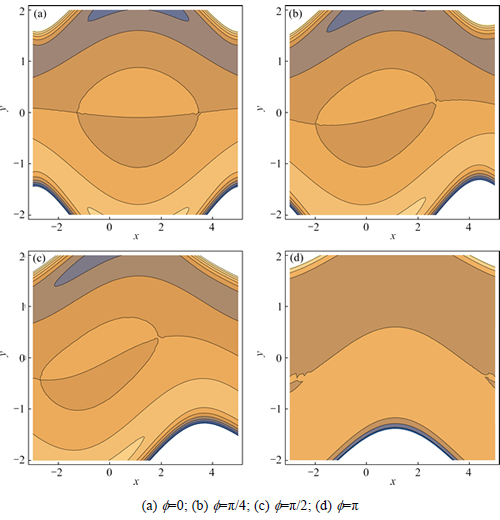
Fig. 9 Effects of φ on streamlines
The effects of fourth grade parameter Γ on the streamlines are shown in Fig. 8. Some streamlines are splitting and enclosing a bolus under some conditions and that bolus completely moves with the wave. Such type of phenomenon is known as trapping. The effects of Γ on trapping are shown when a=0.3, b=0.6, d=0.9, Θ=1.3, φ=π/6 and M=1. It is observed that the size of the trapped bolus decreases with increasing values of Γ.
The effects of phase difference φ on the streamlines are shown in Fig. 9 when a=0.5, b=0.7, d=1, Θ=1.28, Γ=0.1 and M=1. It is observed that the bolus appears at the center for φ=0 and it moves towards left and decreases in size when φ increases and it disappears when φ=π.
5 Conclusions
1) The axial pressure gradient dp/dx increases with increasing values of non-Newtonian fluid parameter Γ while it decreases with increasing values of phase difference φ.
2) The effects of fourth grade parameter Γ are opposite to that of Hartmann number M on the axial velocity.
3) The effects of Γ on temperature profile and concentration profile are quite opposite.
4) The increasing values of convective heat transfer Biot numbers decrease the temperature profile, while convective mass transfer Biot number increases the concentration profile.
5) The size of the trapped bolus decreases with increasing values of fourth grade parameter Γ and phase φ.
Appendix
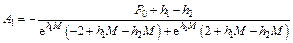
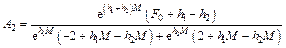
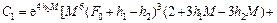
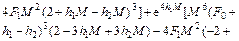
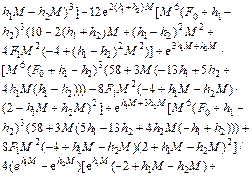
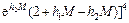
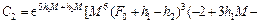
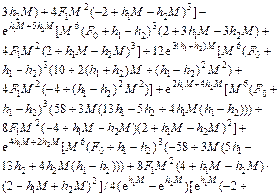
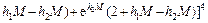
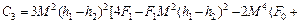
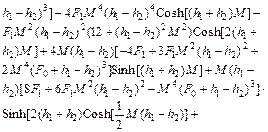
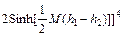
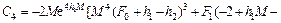
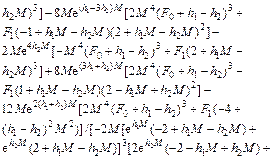
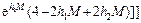
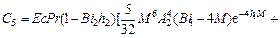
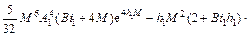
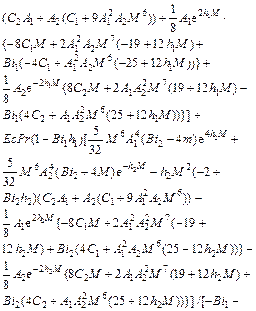
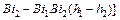
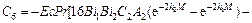
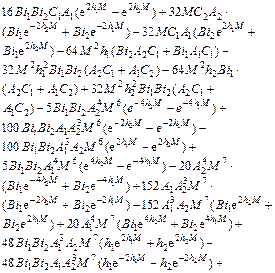
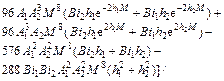
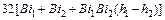
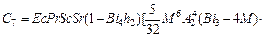
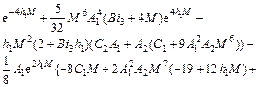
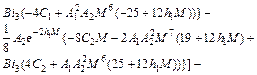
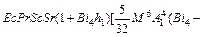
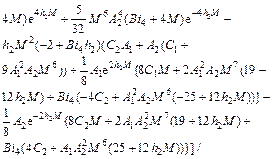
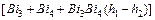
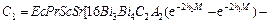
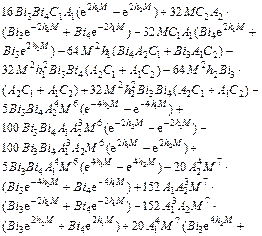
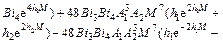
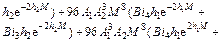
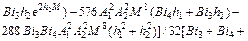
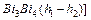
References
[1] HAYAT T, MAMBOUNGOU H M, MAHMOOD F M. A note on some solutions for the flow of a fourth grade fluid in a porous space [J]. Nonlinear Analysis: Real World Applications, 2009, 10(1): 368-374.
[2] NADEEM S, ALI M. Analytical solutions for pipe flow of a fourth grade fluid with Reynold and Vogel’s models of viscosities [J]. Communications in Nonlinear Science and Numerical Simulation, 2009, 14(5): 2073-2090.
[3] MARINCA V, HERISANU N, BOTA C, MARINCA B. An optimal homotopyanymptotic method applied to the steady flow of a fourth grade fluid past a porous plate [J]. Applied Mathematics Letters, 2009, 22(2): 245-251.
[4] AZIZ T, MAHMOOD F M. Reductions and solutions for the unsteady flow of a fourth grade fluid on a porous plate [J]. Applied Mathematics Computations, 2013, 219(17): 9187-9195.
[5] ABDALLA A M, ABODAHAB S M, ELSHAHRANY H D. Effects of rotation and initial stress on peristaltic transport of fourth grade fluid with heat transfer and induced magnetic field [J]. Journal of Magnetism and Magnetic Materials, 2014, 349: 268-280.
[6] EBRAHIMPOUR M, GANJI D D, FOROUGHNIA H. Steady state flow for inside porous flat channel with using analytical solution to fourth grade fluid [J]. Journal of the Brazilian Society of Mechanical Sciences and Engineering, 2015, 37(2): 525-531.
[7] AZIZ T, MAGAN A B, MAHMOOD F M. Invarient solutions for the unsteady magnetohydrodynamics (MHD) flow of a fourth grade fluid induced due to the impulsive motion of a flat plate [J]. Brazilian Journal of Physics, 2015, 45: 120-131.
[8] NAZ R, ALSAEDI A, HAYAT T. Flow of fourth grade fluid in a porous medium [J]. Applied and Computational Mathematics, 2015, 14(2): 125-140.
(Edited by DENG Lü-xiang)
Cite this article as: Ali Aamir, Asghar S, Awais M. Thermophoresis and concentration effects in a fourth grade peristaltic flow with convective walls [J]. Journal of Central South University, 2017, 24(7): 1654-1662. DOI: 10.1007/s11771-017-3571-0.
Received date: 2016-02-26; Accepted date: 2017-03-13
Corresponding author: Ali Aamir, PhD; Tel: +923125264086; E-mail: aamir_ali@ciit-attock.edu.pk