J. Cent. South Univ. Technol. (2011) 18: 223-229
DOI: 10.1007/s11771-011-0683-9
Coupling effects of influence factors on probability of corrosion initiation time of reinforced concrete
ZHANG Xiao-gang(张小刚)1, ZHAO Yan-gang(赵衍刚)2, XING Feng(邢锋)1, LU Zhao-hui(卢朝辉)3
1. College of Civil Engineering, Shenzhen Municipal Key Laboratory for Durability of Civil Engineering Structure,
Shenzhen University, Shenzhen 518060, China;
2. Department of Architecture and Building Engineering, Kanagawa University, Yokohama 2218686, Japan;
3. School of Civil Engineering and Architecture, Central South University, Changsha 410075, China
? Central South University Press and Springer-Verlag Berlin Heidelberg 2011
Abstract: The coupling model of major influence factors such as environmental relative humidity, load-induced crack and stress state affecting the chloride diffusion process in concrete is discussed. The probability distributions of the critical chloride concentration CC, the chloride diffusion coefficient D, and the surface chloride concentration Cs were determined based on the collected natural exposure data. And the estimation of probability of corrosion initiation considering the coupling effects of influence factors is presented. It is found that the relative humidity and curing time are the most effective factors affecting the probability of corrosion initiation before and after 10 years of exposure time. At the same exposure time, the influence of load-induced crack and stress state on the probability of corrosion initiation is obvious, in which the effect of crack is the most one.
Key words: reinforced concrete; corrosion initiation time; coupling influence model; influence factors; natural exposure data; probability distribution
1 Introduction
Steel bar corrosion of reinforced concrete (RC) structures due to chloride ingress is a growing and serious problem all over the world. In general, it is considered that a RC structure will lose its durability [1] when the concentration of chloride ions in concrete at the surface of reinforcing bars reaches a critical threshold value CC to cause the dissolution of the protective passive film around the reinforcement, thereby initiating the corrosion of reinforcement. Therefore, the corrosion initiation time predictions are of great importance.
Numerous researchers [2-4] have found that time to corrosion initiation depends on various factors including the rate of penetration of chloride ions in concrete (expressed as the chloride diffusion coefficient D), the surface chloride concentration (namely Cs), the critical chloride concentration CC and the depth of the concrete cover c. Also, the values of D, Cs, and CC are further influenced by more complex factors, such as environmental temperature, relative humidity, water-to- cement ratio, exposure time, load-induced cracks and stress states. Previous models of corrosion initiation time [2-4] have been developed within a deterministic framework and obtained from particular conditions or in a special laboratory corrosion environment. It is in this reason that the values of CC, D, and Cs, subsequently the corrosion initiation time, vary a lot in these studies.
Actually, in order to accurately gain the corrosion initiation time of a real engineering structure under local aggressive chloride environments, more attention should be paid to the exposure data gathered from natural structure [5], not those obtained from special laboratory environments. At the same time, because there is significant uncertainty associated with the factors related to the chloride ingress into concrete, it might be more appropriate to use a probabilistic approach to predict the time to corrosion initiation [6].
At present, most of previous researches [2-6] have concerned the single influence factor affecting the corrosion initiation time, which means there is no coupling influence analysis of all influence factors. And these researches have been generally limited to the sound concrete without considering load-induced cracks and stress state effect. Since the occurrence of visible macro-cracks and the stress states are inevitable for RC structures under service loads, the influence of cracks and stress states on the time to corrosion initiation should be taken into account.
In this work, the coupling influence model of major factors such as environmental temperature and relative humidity, load-induced cracks and stress states on chloride diffusion process in concrete is discussed. And the probability distributions of CC, D, and Cs are determined based on the natural exposure data in marine environment corrosion published in the literature. According to the above analysis, the estimation of the probability of time to corrosion initiation of RC structure considering the coupling effect of influence factors is presented.
2 Coupling influence model for chloride diffusion process
Many studies [2-6] indicate that the penetration of chlorides through concrete can be treated as a diffusion process and is given empirically by Fick’s second law:
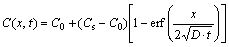 (1)
(1)
where C(x, t) is the chloride content at a distance x from the concrete surface at time t, C0 is the initial chloride content in concrete, Cs is the surface chloride content, D is the chloride diffusion coefficient of concrete, erf(z) is the Gaussian error function and it can be defined by the following equation:
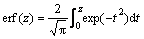 (2)
(2)
Basically, many significant variables such as load- induced cracks and stress states have no effect on CC and Cs but have a great effect on D. In this work, focus is made on their influence on D, subsequently on the probability of time to corrosion initiation. The coupling influence of these factors can be gained from their different correction coefficients.
2.1 Load-induced cracks
Transverse cracks caused by load-induced flexure will increase the penetration speed of chloride ions in concrete. In order to consider the influence of load-induced cracks on the chloride diffusion coefficient for cracked concrete, the average diffusion coefficient in Eq.(1) is transformed into two parts, D0 and DC, as illustrated in Fig.1:
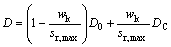 (3)
(3)
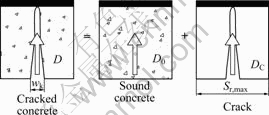
Fig.1 Average chloride diffusion coefficient for cracked concrete
where D is the average chloride diffusion coefficient for cracked concrete, wk is the crack width induced by service load and sr, max is the maximum crack spacing. D0 is the value of chloride diffusion coefficient for sound concrete, and DC is the value of chloride diffusion coefficient inside the crack [7].
According to the researches provided by DJERBI et al [8], the empirical expression of DC is given as follows:
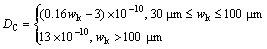 (4)
(4)
Based on the national standard GB 50010—2010, wk can be gained as
 (5)
(5)
where
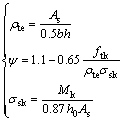 (6)
(6)
For a simple rectangular beam with size of 200 mm× 500 mm, also with cover thickness of 25 mm, reinforcing bars of 4 16 HRB335, and Mk=80 kN·m, the values of Sr, max and wk are equal to 127 mm and 0.276 mm, respectively, according to Eq.(5), which will be used in the following discussion.
16 HRB335, and Mk=80 kN·m, the values of Sr, max and wk are equal to 127 mm and 0.276 mm, respectively, according to Eq.(5), which will be used in the following discussion.
2.2 Stress states
The tensile stress states of concrete lead to gradual micro damage, which will increase the diffusion velocity of chloride in concrete. On the contrary, the compressive stress states will restrict the occurrence of micro damage, which will restrain the diffusion of chloride in concrete.
In order to consider the influence of tensile or compressive stress states on D0 in sound concrete, a correction coefficient λσ is suggested, and D0 is empirically given by
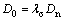 (7)
(7)
where Dn is the corresponding chloride diffusion coefficient without considering the influence of stress states, λσ is the correction coefficient of the influence of stress states.
Based on the experimental results of chloride diffusion in concrete under flexural load described in Ref.[9], the influence rules of tensile and compressive stress states on D0 are illustrated in Fig.2 and Fig.3. As can be seen from Fig.2 and Fig.3, the relative reduction of chloride diffusion coefficient under compressive stress is about 30% that without load action, and the corresponding relative increase under tensile stress is about 60%. As a result, λσ is assumed to be 0.7 (under compressive stress) and 1.6 (under tensile stress state), respectively, in this work for the safety and convenience.
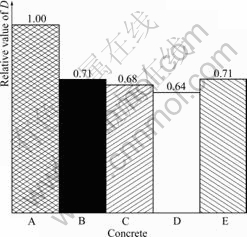
Fig.2 Relative values of chloride diffusion coefficient under compressive stress (A: No stress; B: C25, under 30% of ultimate stress; C: C25, under 60% of ultimate stress; D: C35, under 30% of ultimate stress; E: C35, under 60% of ultimate stress)
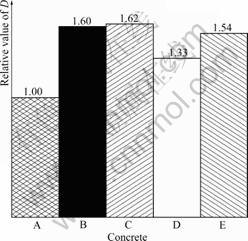
Fig.3 Relative values of chloride diffusion coefficient under tensile stress (A: No stress; B: C25, under 30% of ultimate stress; C: C25, under 60% of ultimate stress; D: C35, under 30% of ultimate stress; E: C35, under 60% of ultimate stress)
2.3 Curing conditions and environment
The curing conditions and environment affect the hydration and hardening process of concrete, and thereby the quality of concrete [10]. Two coefficients accounting for the effect of curing conditions and environment on Dn are introduced, which is expressed as
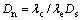 (8)
(8)
where Ds is the corresponding chloride diffusion coefficient without considering the influence of curing conditions and environment. λc is the conservation coefficient, depending mainly on the curing time. For the curing time of 1, 3, 7 and 28 d, λc takes the value of 2.1, 1.5, 1 and 0.79 respectively. λe is the environmental coefficient by taking the effects of cementitious material and marine environment into account (Table 1).
Table 1 Environmental coefficient

2.4 Temperature, relative humidity and time
Relative humidity and temperature have a significant effect on the chloride diffusion process in concrete [11]. Here, two coefficients accounting for the effect of relative humidity and temperature on Ds at time t are introduced:
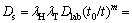
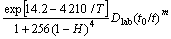 (9)
(9)
where Dlab is the standard result of chloride diffusion coefficient from laboratory, which means that the experimental conditions such as relative humidity, temperature and curing conditions are the same. λH and λT are the correction coefficients of relative humidity and temperature, respectively. T is the current temperature. H is the relative humidity in concrete. t0 is the time when the hydration of concrete initiates. t is the current time. And m is the age factor, which is related to water-to- cement (w/c) ratio (R). For w/c ratio varying from 0.25 to 0.45, m=3(0.55-R).
3 Probability distributions of CC, D and Cs based on nature exposure data
As mentioned above, there are so many complex factors affecting the chloride diffusion process in concrete. In order to accurately gain the corrosion initiation time of a real engineering structure, more attention should be paid to the exposure data gathered from natural structure. The probability distributions of CC, D and Cs based on natural exposure data are discussed.
Most of the data in previous references are summarized in this work (as shown in Table 2), and some of them are also presented in Refs.[2, 23-26]. The measured values of CC, D, and Cs in previous references, actually are the mean values from the beginning of exposure to the time inspected. For the sake of the statistical accuracy, the random parameter is selected in the following restrictive conditions: 1) the water cement ratio is 0.4-0.66 (few of them have no water-to-cement ratio), 2) the exposure time is between 1 to 17 years, and 3) the critical threshold chloride concentration is originated from the summarized data in Ref.[27], which are not presented in this work. From the corresponding data fitting results of exposure data for CC, D, and Cs (seen in Fig.4), the respective probability distributions of CC, D, and Cs are as follows.
Table 2 Summarized nature exposure data of D and Cs
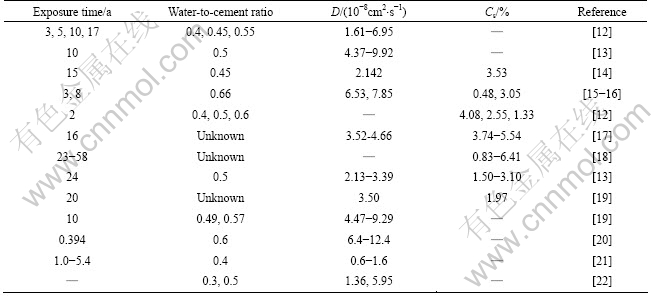
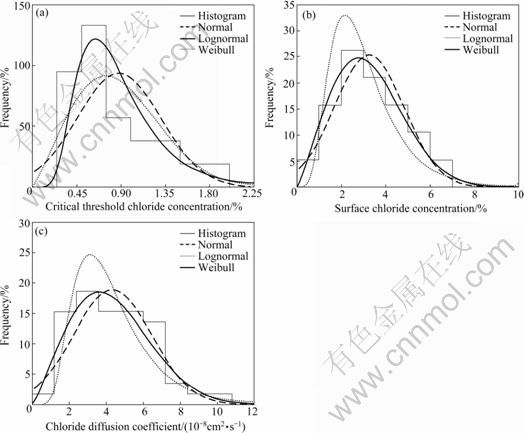
Fig.4 Histogram and data fitting results of exposure data: (a) Critical threshold chloride concentration; (b) Surface chloride concentration; (c) Chloride diffusion coefficient
1) Probability distribution of the chloride diffusion coefficient follows Weibull distribution:
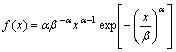 (10)
(10)
where α=0.533 3 and β=3.077 8.
2) Probability distribution of the surface chloride concentration fits Weibull distribution too. The formula is the same as Eq.(10), and here α=0.520 8 and β= 3.058 7.
3) Probability distribution of critical threshold chloride concentration fits lognormal distribution:
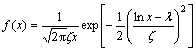 (11)
(11)
where λ=-0.235 5 and ζ=0.459 3.
4 Probabilistic analysis of corrosion initiation
The concentration of chloride ions at time t at the surface of steel bar C(c, t) and the critical threshold chloride concentration CC can be expressed as exterior deterioration load effect (S) and interior resistance (R), respectively. According to Eq.(1), C(c, t) is the value of C(x,t) when x=c. The performance function for corrosion initiation of reinforcing steel in concrete can be expressed as
 (12)
(12)
The probability of depassivation of reinforcing steel, Pd(t), can be estimated as
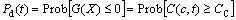 (13)
(13)
The reliability analysis is based on the use of the Monte Carlo simulation technique, which involves sampling at random to simulate artificially a large number of experiments and to observe the result. Once the probability of corrosion initiation Pd(t) is gained, the corrosion initiation time of steel bar Td can be obtained based on the following equation:
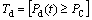 (14)
(14)
where PC is the given acceptable probability.
The statistical parameters for corrosion random variables considered are given in Table 3. Based on the values of wk and Sr, max gained above and the statistical parameters in Table 3, the probability of corrosion initiation changed with the exposure time for sound or cracked concrete subjected to different stress states is illustrated in Fig.5. Similarly, the effects of other influence factors such as curing time, environment, temperature and relative humidity on probability of corrosion initiation can be illustrated in Figs.6-9. As can
Table 3 Statistical parameters for corrosion random variables based on natural exposure data
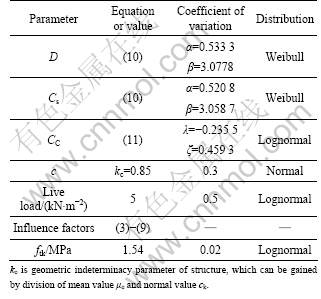
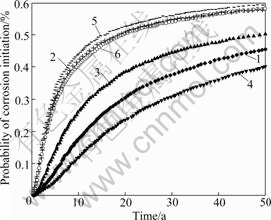
Fig.5 Influence of crack and stress state on probability of corrosion initiation (1—No stress state, sound concrete; 2—No stress state; cracked concrete; 3—Tensile stress state, sound concrete; 4—Compressive stress state, sound concrete; 5—Tensile stress state, cracked concrete; 6—Compressive stress state, cracked concrete)
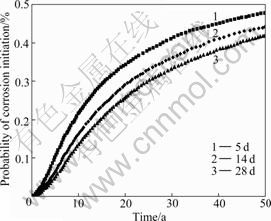
Fig.6 Influence of curing time on probability of corrosion initiation
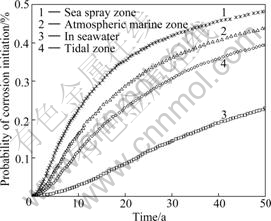
Fig.7 Influences of environment on probability of corrosion initiation
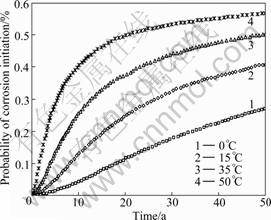
Fig.8 Influences of temperature on probability of corrosion initiation

Fig.9 Influences of relative humidity on probability of corrosion initiation
be seen from Figs.5-9, the probability of corrosion initiation of reinforcement increases as the exposure time increases. The probability increases rapidly at the first phase of exposure time and after some year’s exposure the probability tends to be moderate. From Figs.5-9, one can further find that, the relative humidity is the most effective factor affecting on the probability of corrosion initiation in the early 10 years of exposure. After that stage, the curing time has the greatest effect on this probability, subsequently the environment, crack and stress state comes, then the temperature follows. On the contrary, the relative humidity is the last one affecting the probability of corrosion initiation after 10 years of exposure. Also, one can see from Fig.5 that, at the same exposure time, the influence of crack and stress state on the probability of corrosion initiation is obvious, in which the effect of crack is the most one. Compared with a sound concrete in no stress state, the probability of corrosion initiation tends to become smaller under compressive stress and increases under tensile stress. However, the influence of stress state on the probability of corrosion initiation is lower than the corresponding influence of crack. For a cracked concrete, the probability of corrosion initiation increases highly compared with a sound concrete at the same exposure time. When the concrete cracks, the effect of stress state on the probability of corrosion initiation decreases.
5 Conclusions
1) The relative humidity and curing time are the most effective factors affecting the probability of corrosion initiation before and after 10 years of exposure time.
2) At the same exposure time, the influence of crack and stress state on the probability of corrosion initiation is obvious, in which the effect of crack is the most one. For a cracked concrete, the probability of corrosion initiation increases highly compared with a sound concrete. When the concrete cracks, the effect of stress state on the probability of corrosion initiation decreases.
References
[1] ZHANG Xiao-gang, ZHAO Yan-gang, LU Zhao-hui. Dynamic corrosion-induced cracking process of RC structures considering the influence of initial defects [J]. Journal of Asian Architecture and Building Engineering, 2010, 2: 439-446.
[2] STEWART M G, ROSOWSKY D V. Time-dependent reliability of deteriorating reinforced concrete bridge decks [J]. Structural Safety, 1998, 20: 91-109.
[3] ERDOGDU S, KONDRATOVA I L, BREMNER T W. Determination of chloride diffusion coefficient of concrete using open-circuit potential measurements [J]. Cement and Concrete Research, 2004, 34: 603-609.
[4] SONG Hawon, LEE Changhong, ANN Kiyong. Factors influencing chloride transport in concrete structures exposed to marine environments [J]. Cement & Concrete Composites, 2008, 30: 113-121.
[5] ANDERS L. Chloride ingress data from field and laboratory exposure—Influence of salinity and temperature [J]. Cement & Concrete Composites, 2007, 29: 88-93.
[6] MAHESWARAN T, SANJAYAN J G. A semi-closed-form solution for chloride diffusion in concrete with time-varying parameters [J]. Magazine of Concrete Research, 2004, 56(6): 359-366.
[7] LU Zhao-hui, ZHAO Yan-gang, ZHANG Xiao-gang. Reliability based service life prediction of corrosion affected RC flexural members. Part I: Time to corrosion initiation [C]// Proceedings of the 4th International Forum on Engineering Decision Making. Hakone, Japan, 2009: 53-60.
[8] DJERBI A, BONNET S, KHELIDJ A, BAROGHEL-BOUNY V. Influence of traversing crack on chloride diffusion into concrete [J]. Cement and Concrete Research, 2008, 38(6): 877-883.
[9] ZHAO Shang-chuan. Test of chloride diffusion rules in concrete at tidal zone under flexural load [J]. China Journal of Highway and Transport, 2007, 20(4): 76-82. (in Chinese)
[10] CHEN Zhao-yuan, FAN Li-chu, LIU Jian-hang. CCES 01-2004, Guide to durability design and construction of reinforced structures [M]. China Architecture & Building Press, 2005: 65-78. (in Chinese)
[11] VU Kat, STEWART M G. Structural reliability of concrete bridges including improved chloride-induced corrosion models [J]. Structural Safety, 2000, 22: 313-333.
[12] GJΦRV O E, VENNESLANDΦ. Diffusion of chloride ions from seawater into concrete [J]. Cement and Concrete Research, 1979, 9: 229-238.
[13] LIAM K C, ROY S K, NORTHWOOD D O. Chloride ingress measurements and corrosion potential mapping study of a 24-year-old reinforced concrete jetty structure in a tropical marine environment research [J]. Magazine of Concrete Research, 1992, 44: 205-215.
[14] MOHAMMED T U, YAMAJI T, HAMADA H. Chloride diffusion, microstructure and mineralory of concrete after 15 years of exposure in tidal environment [J]. ACI Material, 2002, 99: 256-263.
[15] BAMFORTH P B, PRICE W F. Factors influencing chloride ingress into marine structures [M]// DHIR R K, JONES M R. Concrete. London: Elsevier Science, 1993: 1105-1118.
[16] DHIR R K, EL-MOHR M, DYER T D. Chloride binding in GGBS concrete [J]. Cement and Concrete Research, 1996, 26: 1767-1773.
[17] FUNAHASHI M. Predicting corrosion free service life of a concrete structure in a chloride environment [J]. Aci Material, 1990, 87: 581-587.
[18] UJI K, MATSUOKA Y, MARUYA T. Formation of an equation for surface chloride content of concrete due to permeation of chloride [M]// Corrosion of Reinforcement in Concrete. London: Elsevier Applied Science, 1990: 258-267.
[19] KUDOH K, HIROTANI A, MORIWAKE A, YASUDA M. Study on durability of a repaired concrete structure in Tokyo bay [C]// Evaluation and Rehabilitation of Concrete Structures and Innovations in Design. ACI 128, Hong Kong, 1991: 1293-12307.
[20] ERDOGDU S, KONDRATOVA I, BREMNER T. Determination of chloride diffusion coefficient of concrete using open-circuit potential measurements [J]. Cement and Concrete Research, 2004, 34: 603-609.
[21] SANDBERG P, TANG L, ANDERSEN A. Recurrent studies of chloride ingress in uncracked marine concrete at various exposure times and elevations [J]. Cement and Concrete Research, 1998, 28(10): 1489-1503.
[22] POUPARD O, AIT-MOKHTAR A, DUMARGUE P. Corrosion by chlorides in reinforced concrete: Determination of chloride concentration threshold by impedance spectroscopy [J]. Cement and Concrete Research, 2004, 34: 991-1000.
[23] FAN Zhi-hong, YANG Fu-lin, HUANG Jun-zhe, WANG Shen-nian. Experimental study on long-term exposure test of marine concrete [J]. Port & Waterway Engineering in China, 2005(9): 45-57. (in Chinese)
[24] HWAN Byungoh, JANG Seungyup. Effects of material and environmental parameters on chloride penetration profiles in concrete structures [J]. Cement and Concrete Research, 2007, 37: 47-53.
[25] THOMAS M, MATTHEWS J. Performance of PFA concrete in a marine environment—10 year results [J]. Cement & Concrete Composites, 2004, 26: 5-20.
[26] STERRITT G, CHRYSSANTHOPOULOS M K. Probabilistic limit state modeling of deteriorating RC bridge using a spatial approach [C]// Proceedings of the International Conference on Current Trend in Bridge Design, Construction and Maintenance. London, 1999: 518-528.
[27] ALONSO C, ANDRADE C, CASTELLOTE M, CASTRO P. Chloride threshold values to depassivate reinforcing bars embedded in a standardized OPC mortar [J]. Cement and Concrete Research, 2000, 30: 1047-1055.
(Edited by YANG Bing)
Foundation item: Project(50925829) supported by the National Science Fund for Distinguished Young Scholars of China; Project(50908148) supported by the National Natural Science Foundation of China; Projects(2009-K4-23, 2010-11-33) supported by the Research of Ministry of Housing and Urban Rural Development of China
Received date: 2009-10-01; Accepted date: 2010-09-30
Corresponding author: ZHANG Xiao-gang, Associate Professor, PhD; Tel: +86-755-26535212; E-mail: bszxg@126.com