粉末冶金Al-1.1Sc和Al-2SC合金晶体结构的实验和第一性原理研究
来源期刊:中国有色金属学报(英文版)2013年第10期
论文作者:Hamza Ya?ar OCAK Ercan U?GUN Rahmi üNAL
文章页码:3020 - 3026
关键词:Al-Sc合金;晶格常数;弹性性能;从头计算;第一性原理
Key words:Al-Sc alloy; lattice constant; elastic properties; Ab initio calculations; first principles
摘 要:采用气体雾化合金颗粒制备Al-1.1%Sc 和 Al-2%Sc合金。采用X射线衍射分析合金样品以确定样品的晶格参数。利用这些晶格参数,分别通过WIEN2K和EMTO程序对该合金的力学性能进行理论计算。将实验得到的合金的弹性模量和理论计算结果进行比较。讨论了计算得到的Al3Sc相和Al-Sc合金的力学性能以确定Al-Sc合金中最佳的Sc含量。结果表明,最高的Sc含量约为1.0%,更多的Sc不能提高合金的力学性能。
Abstract: Al-1.1%Sc and Al-2%Sc (mass fraction) alloys were prepared using gas atomized alloy particles. Samples from consolidated alloys were analyzed by XRD for the determination of lattice parameters. Using these lattice parameters, the mechanical properties of the alloys were calculated theoretically with WIEN2k and EMTO programs, respectively. The elasticity moduli of the experimentally produced alloys were compared with the theoretical calculation results. The calculated mechanical properties of Al3Sc phase and Al-Sc alloys were discussed to determine the optimum Sc content of Al-Sc alloys. It may be concluded that the Sc content should be the maximum about 1.0%, much more Sc addition could not improve the mechanical properties of the alloys.
Trans. Nonferrous Met. Soc. China 23(2013) 3020-3026
Hamza  OCAK1, Ercan
OCAK1, Ercan  1, Rahmi
1, Rahmi  2
2
1. Department of Physics, Faculty of Arts and Sciences, Dumlupinar University, Kutahya, Turkey;
2. Mechanical Engineering Department, Engineering Faculty, Gazi University, Ankara, Turkey
Received 7 January 2013; accepted 25 March 2013
Abstract: Al-1.1%Sc and Al-2%Sc (mass fraction) alloys were prepared using gas atomized alloy particles. Samples from consolidated alloys were analyzed by XRD for the determination of lattice parameters. Using these lattice parameters, the mechanical properties of the alloys were calculated theoretically with WIEN2k and EMTO programs, respectively. The elasticity moduli of the experimentally produced alloys were compared with the theoretical calculation results. The calculated mechanical properties of Al3Sc phase and Al-Sc alloys were discussed to determine the optimum Sc content of Al-Sc alloys. It may be concluded that the Sc content should be the maximum about 1.0%, much more Sc addition could not improve the mechanical properties of the alloys.
Key words: Al-Sc alloy; lattice constant; elastic properties; Ab initio calculations; first principles
1 Introduction
It is well known that the transition metals (TM) have small solid solubility and low diffusivity in aluminum, but a small amount of TM exerts a pronounced influence on the structure and properties of pure aluminum and aluminum alloys [1]. Among intermetallic compounds, the transition metal trialuminides are interest because of their low density, high melting point, good oxidation resistance and potentially useful high-temperature strength for aerospace and automotive applications [2]. In the case of Al alloys, scandium (Sc) contributes significantly to improving strength by forming nanoscale coherent Al3Sc precipitates [1,3]. Al-rich Al-Sc alloys are generally used as high-strength materials. The effects of Sc addition in Al alloys are mainly attributed to the formation of Al3Sc with cubic L12 structure, as precipitation strengthener, grain refiner, recrystallization inhibitor [1,2].
Many papers were published dealing with the influence of scandium on structure and properties of cast Al alloys [4-9]. It is a common knowledge nowadays that alloying of aluminum alloys by scandium contributes to the increase of their strength and in some cases to the plasticity properties in cast alloys [10-12]. The most important fact is that the Al3Sc phase formed in scandium-bearing Al alloys has the same crystal structure (cubic face centered) and very similar lattice constant (0.4105 nm) compared with pure Al (0.4049 nm). This similarity in the crystalline lattice is the main reason for the crystallization of primary Al3Sc particles from the molten metal and for the formation of fine dispersed secondary coherent Al3Sc particles from the supersaturated solid Al solution (sub-solids formation) [13]. The primary Al3Sc particles should act as crystal nucleus, therefore cause a significant grain size decrease and an increase in strength and hardness [14]. The peculiarities of scandium interaction with aluminum and main alloying elements and the effect of scandium on structure and properties of aluminum alloys give the scientific basis for the development of new alloys. The solid solubility of scandium in aluminum is about 0.38%. Rapid cooling can increase the alloying limits in aluminum by enhancing super saturation enabling greater precipitation hardening without harmful segregation effects that occur when ingot metallurgy alloys are over alloyed. Elements that are essentially insoluble in the solid state are often soluble in the liquid state and may be uniformly distributed in the powder particles during rapid solidification. Non-equilibrium metastable phases or atom “clusters” that do not exist in more slowly cooled ingots can be created by the rapid solidification rate and these phases often increase the strength. By powder metallurgy (PM) route, precipitation of equilibrium phases that are deleterious to mechanical or corrosion properties can be suppressed. Therefore, in this study powder metallurgy route was used to increase the solid solubility of the Al-Sc alloy particles. Al-1.1%Sc and Al-2%Sc alloys were prepared using gas atomized alloy particles. Samples from the produced alloys were analyzed by XRD for the determination of lattice parameters. By these lattice parameters, the mechanical properties of the alloys were calculated theoretically with WIEN2k and EMTO programs, respectively. The modulus of elasticity values of the produced alloys were compared with the theoretical calculation results. The calculated mechanical properties of Al3Sc phase and Al-Sc alloys were discussed to determine the optimum Sc content of Al-Sc alloys.
2 Experimental
Pure aluminum (99.99%) and Al-2Sc master alloy were used for the preparation of Al-Sc alloys used in this study. Alloys were prepared by melting in silica crucible in the melting chamber of the atomization unit under protective atmosphere and atomized by gas atomization system. Argon gas and same atomization conditions were used for powder production of different samples. Powders were sieved under 200 μm and encapsulated into aluminum cans of 70 mm in diameter and 150 mm in length, cold compacted by uniaxial pressing, evacuated and preheated for 1 h before extrusion. The billets were extruded on a 400 t horizontal extrusion press at 300 °C to 14 mm round bars with an extrusion ratio of 25. A series of specimens were prepared from the extruded rods by mechanical method and were prepared into the metallographic samples. The structure of the Al-Sc alloys was analyzed by X-ray diffractometer. The chemical composition of the alloys was determined by EDX from the consolidated alloys.
3 Computational method
The mechanical properties of PM Al-Sc alloys were used to verify the results of the theoretical calculations by WIEN2k and EMTO programs. The structure of the alloys were designed to be ordered for WIEN2k and disordered for EMTO programs. The two different alloy structure designs were compared for the determination of mechanical properties of the alloys. After verification of the theoretical solutions, the mechanical properties of Al-Sc alloys containing 0.5%, 1.1%, 2.0%, 3.0%, 4.0%, 5.0% Sc were calculated using EMTO program to find out the optimum scandium content.
Elastic properties were determined by Charpin method which is integrated in the WIEN2k package [15] and MEHL [16,17] method separately using WIEN2k program. We also employed the exact muffin-tin orbitals method (EMTO) with the coherent-potential approximation (CPA) that has been proven to be a reliable tool for the calculation of elastic constants of alloys [18-21]. The generalized gradient approximation (GGA) approach [22] was used for the calculation.
The following parameters were used for WIEN2k calculations to separate core, and the valance state energy threshold was -81.636 eV, the muffin-tin radii were 1.296 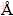 for both Al and Sc in AlSc structure, 1.286
for both Al and Sc in AlSc structure, 1.286 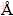 for both Al and Sc in Al3Sc structure and 1.270
for both Al and Sc in Al3Sc structure and 1.270 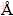 for both Al and Sc in Al31Sc1 structure. To achieve convergence, the energy criterion was chosen as 13.606×10-5 eV. For the number of plane waves, RMTKmax=10 was taken. The integrals over the Brillouin zone (IBZ) were performed with 21×21×21 grids.
for both Al and Sc in Al31Sc1 structure. To achieve convergence, the energy criterion was chosen as 13.606×10-5 eV. For the number of plane waves, RMTKmax=10 was taken. The integrals over the Brillouin zone (IBZ) were performed with 21×21×21 grids.
The following approach and parameters were used for EMTO calculations. 16 complex energy points for the Green’s function integration were used. The k-space sampling of the irreducible part of the Brillouin zone was done by 21×21×21 points for the all AlSc alloys. The total energy was calculated for the Wigner-Seitz radii Rws=(2.90, 2.92, …, 3.06, 3.08) Bohr corresponding to the atomic volumes V≈15.14, 15.45, 15.77, 16.10, 16.43, 16.76, 17.10, 17.44, 17.79, 18.14 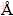 3. Wigner-Seitz radius at the minimum energy value was also used for the calculation of elastic constants. Lattice parameters were obtained from the volume-energy graphics for both calculations.
3. Wigner-Seitz radius at the minimum energy value was also used for the calculation of elastic constants. Lattice parameters were obtained from the volume-energy graphics for both calculations.
In the theoretical study by WIEN2k, the alloy structure was designed as a supercell (Fig. 1) with 32 atoms with a two times greater unit cell (2×2×2). The reason of using 32 atom supercell is to simulate the designed structure with the experimental alloy compositions. If the supercell structure is increased one step forward, the resultant structure will have 108 atoms. This design does not match with our experimental alloy compositions. In the theoretical XRD study of 108 atoms design, there are only three peaks which are similar to the peaks of the experimental XRD analysis. Hence, we decided to use 32 atoms supercell in this study.
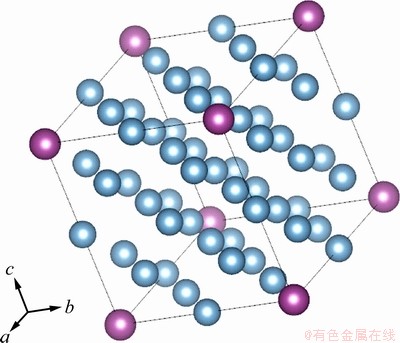
Fig. 1 Designed structure of Al31Sc1 alloy (Scandium atom is at each corner)
The results of the theoretical study were compared with the corresponding experimental values. The lattice parameters given in Table 1 were calculated from the energy versus volume graphics of AlSc alloys given in Figs. 2-4. The calculated energies data as a function of the primitive cell volume were fitted to a MURNAGHAN [23] equation of state (EOS) for WIEN2k calculation. In EMTO calculation the energy—volume curve was fitted to a Birch-Murnaghan [24] EOS. The calculation was made according to the minimum energy point in the graphics where the structure is at the most stable state. Increasing Sc content increases the energy level and changes the stability point as shown in Fig. 4.
Table 1 Calculated bulk properties from energy minimization of PM Al-Sc alloys
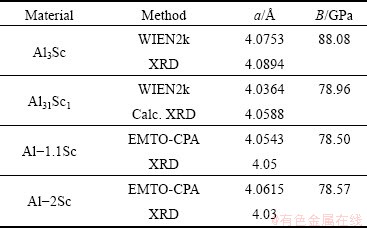

Fig. 2 Calculated energy as function of primitive cell volume for Al3Sc compound

Fig. 3 Calculated energy data as function of primitive cell volume for Al31Sc1
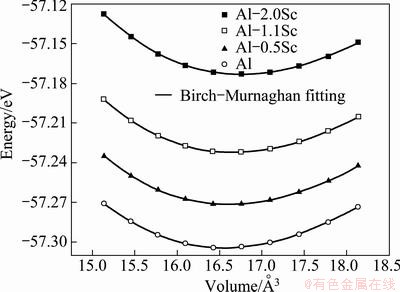
Fig. 4 Calculated energy as function of primitive cell volume for Al-1.1Sc and Al-2Sc alloys
There are three independent elastic constants in a cubic crystal structure. The elastic constants are defined by the following formula:
 (1)
(1)
Determination of the elastic constants requires knowledge of the curvature of the energy curve as a function of strain for different deformation of the unit cell. Some of these deformations change the volume of the unit cell but maintain the tetragonal symmetry, whereas others break the tetragonality. The detailed information for the calculation of the elastic constants is given in Refs. [15-17,25]. The elastic constants are related to the bulk modulus (B).
B=(C11+2C12)/3 (2)
The necessary conditions for mechanical stability are given by C11>0, C44>0, C11>|C12| and (C11+2C12)>0. Anisotropy parameter is defined as A=2C44/(C11-C12). This parameter has an important implication in engineering science since it is highly correlated with the possibility of introducing microcracks in the material [26]. There is also another anisotropy parameter that is defined as [27]
 (3)
(3)
and known as Voigt-Reuss-Hill anisotropy. This parameter is zero for isotropic crystals and its deviation from zero gives a measure of the anisotropy.
The calculated elastic constants are valid only for the single crystals. Generally, all the metallic materials have polycrystalline structures. Equations of the polycrystalline structures are given as follows [28-30]:
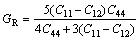 (4)
(4)
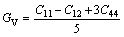 (5)
(5)
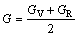 (6)
(6)
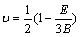 (7)
(7)
Shear modulus (G) is a measure of the resistance to reversible deformation upon shear stress and it plays a dominant role in predicting the hardness rather than the bulk modulus. Another important parameter is the elastic modulus (E) which is related with the stiffness of the materials. There are generally many parameters for mechanical properties. Ductility and brittleness of the material are related with the parameters of Cauchy pressure (C12-C44), Pugh’s index of ductility on G/B and Poisson ratio (v) [31].
4 Results and discussion
Figure 5 shows the XRD patterns of Al-1.1Sc and Al-2Sc alloys. The XRD patterns show that the specimens have FCC crystal structure having Al and Al3Sc two phases. According to our XRD results, there is Al3Sc phase (L12 crystal structure and 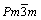 space group) in two of the Al-Sc alloys. The lattice constants used for the theoretical study were calculated from XRD results given in Table 1. Al3Sc phase peaks are difficult to see on the XRD pattern of the alloys, because the difference between the diffraction angle of Al and Al3Sc phases is very small. Al3Sc phases are marked in order to show the Al3Sc peaks which are near the Al peaks.
space group) in two of the Al-Sc alloys. The lattice constants used for the theoretical study were calculated from XRD results given in Table 1. Al3Sc phase peaks are difficult to see on the XRD pattern of the alloys, because the difference between the diffraction angle of Al and Al3Sc phases is very small. Al3Sc phases are marked in order to show the Al3Sc peaks which are near the Al peaks.
The calculated mechanical properties for single and polycrystals are given in Table 2. The calculated elastic properties of Al3Sc are well matched with the values given in the literature. Al3Sc structure is stronger than Al31Sc1 structure, according to the bulk modulus which is a measure of the mechanical strength. The bulk moduli of Al31Sc1, Al-1.1Sc and Al-2Sc are lower than that of Al3Sc due to very low Sc content in Al-Sc alloys. The bulk moduli of pure aluminum, Al31Sc1, Al-1.1Sc and Al-2Sc are 76.89, 78.96, 78.50 and 78.57 GPa, respectively. The bulk modulus of Al31Sc1 calculated by WIEN2k, and the bulk moduli of Al-1.1Sc, Al-2Sc and Al calculated by EMTO are very close to each other. According to these results, Al31Sc1 ordered structure gives similar result with the other disordered structures. Elastic constants of the studied alloy were matched with the criteria of the mechanical stability which is stated by BORN [36]. The calculated elastic constants are also in agreement with the experimental data [33] and other theoretical results given in Table 2.

Fig. 5 XRD patterns for Al-1.1Sc (a) and Al-2Sc (b) alloys
Experimentally determined elasticity moduli of the Al-1.1Sc and Al-2Sc are 68.0 and 69.0 GPa, respectively [34,35]. In order to compare the experimental results, the moduli of elasticity of Al-1.1Sc and Al-2Sc alloys were calculated by the following equation [37]:
 (8)
(8)
The modulus of elasticity for the Al31Sc1 supercell structure was 107.93 GPa by WIEN2k program. The reason of this great difference is that C11 is very high for this ordered design structure of the alloy. The calculated modulus of elasticity by EMTO of Al-1.1Sc and Al-2Sc alloys are 86.38 GPa and 86.67 GPa, respectively. Because the theoretical results are very close to the experimental values, it is concluded that EMTO program is suitable for calculation of modulus elasticity of the alloy. If the elastic modulus is higher for the material, stiffness of the material is higher. Shear modulus which is related with three elastic constants, it is a measure of the strength of the material. The shear modulus of Al31Sc1 is higher than that of the Al, Al-1.1Sc and Al-2Sc alloys. This result shows that the ordered supercell structure has higher shear modulus than the disordered structure.
Table 2 Calculated elastic constants Cij, shear modulus G, elastic modulus, Poisson ratios υ and anisotropy factors A for FCC Al-Sc alloys
According to the results of anisotropy parameter that is an indicator of an isotropic material, it is seen that Al3Sc structure is approximately isotropic because it has an anisotropy parameter of near one according to the results of two methods calculated by WIEN2k (see Table 2). It can be concluded that this structure has the possibility of microcracks, because this parameter has an important implication in engineering science since it is highly correlated with the possibility of introducing microcracks in a material [26]. Since Al31Sc1 structure has an anisotropy value of A=0.76, this indicates that the structure is slightly anisotropic. Al-1.1Sc and Al-2Sc theoretical alloys are far away from isotropy (2.41 vs 2.43). The reason could be the disordered design of the theoretical alloy structure. Avrh parameters also support this result.
There are many parameters to indicate if a material has ductile or brittle property. In our study, B/G ratio and Poisson ratio (n) were calculated. The Cauchy pressure is defined as C12-C44. In the present work, the Cauchy pressure is negative for Al3Sc which shows that this structure is brittle while the others have ductile properties. Furthermore, if B/G ratio is higher than 1.75, the material would have ductile properties [38]. According to the calculated results, Al3Sc (B/G=1.21) has brittle properties and Al31Sc1 (B/G=1.86), Al-1.1Sc (B/G=2.39), Al-2Sc (B/G=2.39) have ductile properties.
Poisson ratio is the ratio of transverse contraction strain to longitudinal extension strain in the direction of stretching force. Tensile deformation is considered positive and compressive deformation is considered negative. The definition of Poisson ratio contains a minus sign so that normal materials have a positive ratio. According to the literature, if a material has a Poisson ratio less than 0.26, the material is brittle [39]. Aluminum has a Poisson ratio of 0.32. The calculated Poisson ratios are 0.18, 0.27, 0.32 and 0.32 for Al3Sc, Al31Sc1, Al-1.1Sc and Al-2Sc, respectively. Al3Sc has brittle properties while the others have ductile properties.
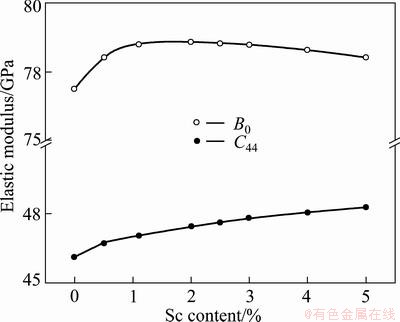
Fig. 6 Variation of B0 and C44 constants with Sc content
Variation of B0 and C44 constants according to the Sc content is given in Fig. 6. Bulk modulus is increasing until 1% Sc, then, bulk modulus is decreased with increasing Sc content. C44 is increased continuously with increasing Sc content. As a result, it may be concluded that the Sc content should be maximum about 1%, much more Sc addition could not improve the mechanical properties of the alloys.
5 Conclusions
1) The bulk moduli of Al and Al-Sc alloys calculated by EMTO methods are very close to each other, and the bulk modulus of Al31Sc1 is also very near to the EMTO value. The bulk modules of the Al3Sc is calculated by the energy minimization using CHARPIN and MEHL methods. The results are close to each other which are agreement with that reported in the literature.
2) The calculated elastic constants are in agreement with Max Born mechanical stability criteria and the data given in the related references.
3) The modulus of elasticity of the Al-Sc alloys determined by EMTO program is much better than at calculated by WIEN2k program.
4) The bulk modulus of Al-Sc alloys increases as Sc content increases until 1%. Further increase of scandium addition does not improve the mechanical properties of the alloys. The calculated results show that Al-1.1Sc and Al-2Sc alloys have anisotropy and ductile properties.
References
[1] HYUNG-HO J, FUJIKAWA S I. Kinetics of precipitation in Al3Sc alloys and low temperature solid solubility of scandium in aluminium studied by electrical resistivity measurements [J]. Materials Science and Engineering A, 1993, 171: 151-161.
[2] ROYSET J, RYUM N. Scandium in aluminium alloys [J]. International Materials Reviews, 2005, 50: 19-44.
[3] LI Yan-feng, XU Hui, ZHANG Biao, ZHANG Li-gang. Electronic structure, stability and thermodynamic properties of Al-Sc intermetallic compounds [J]. The Chinese Journal of Nonferrous Metals, 2010, 20(s1): s946-s953. (in Chinese)
[4] NOVOTNY G M, ARDELL A J. Precipitation of Al3Sc in binary Al-Sc alloys [J]. Materials Science and Engineering A, 2001, 318: 144-154.
[5] RESHAK A H, CHARIFI Z, BAAZIZ H. Ab-initio calculation of structural, electronic, and optical characterizations of the intermetallic trialuminides ScAl3 compound [J]. Journal of Solid State Chemistry, 2010, 183: 1290-1296.
[6] ASTA M, FOILES S M, QUONG A A. First-principles calculations of bulk and interfacial thermodynamic properties for fcc-based Al-Sc alloys [J]. Physical Review B, 1998, 57: 11265-11275.
[7] TAO X, OUYANG Y, LIU H, ZENG F, FENG Y, JIN Z. Calculation of the thermodynamic properties of B2 AlRE (RE=Sc, Y, La, Ce-Lu) [J]. Physica B, 2007, 399: 27-32.
[8] MANH D N, PETTIFOR D G. Electronic structure, phase stability and elastic moduli of AB transition metal aluminides [J]. Intermetallics, 1999, 7: 1095-1106.
[9]  G. Phonon and elastic properties of AlSc and MgSc from first-principles calculations [J]. Computational Materials Science, 2010, 48: 866-870.
G. Phonon and elastic properties of AlSc and MgSc from first-principles calculations [J]. Computational Materials Science, 2010, 48: 866-870.
[10] TAO X, OUYANG Y, LIU H, ZENG F, FENG Y, JIN Z. Ab initio calculations of mechanical and thermodynamic properties for the B2-based AlRE [J]. Computational Materials Science, 2007, 40: 226-233.
[11] GAO Ying-jun, BAN Dong-mei, HAN Yong-jian, ZHONG Xia-ping, LUI Hui. Atomic bonding and mechanical properties of Al-Mg-Zr-Sc alloy [J]. Transactions of Nonferrous Metals Society of China, 2004, 14(5): 922-927.
[12] CACCIAMANI G, RIANI P, BORZONE G,  N, SACCONE A, FERRO R, PISCH A, FETZER R S. Thermodynamic measurements and assessment of the Al-Sc system [J]. Intermetallics, 1999, 7: 101-108.
N, SACCONE A, FERRO R, PISCH A, FETZER R S. Thermodynamic measurements and assessment of the Al-Sc system [J]. Intermetallics, 1999, 7: 101-108.
[13] LIAO X, XIE H, ZHAI Y, ZHANG Y. Preparation of Al3Sc intermetallic compound by FFC method [J]. J Mater Sci Technol, 2009, 25: 717-720.
[14] LAMIKHOV L K, SAMSONOV G V. Modification of aluminum by transition metals [J]. Soviet Physics Doklady, 1964, 9: 376-378.
[15] BLAHA P, SCHWARZ K, MADSEN G K H, KVASNICKA D, LUITZ J. WIEN2k, an augmented plane wave+local orbitals program for calculating crystal properties [M]. Wien, Austria: Karlheinz Schwarz, Tech. Universitat, 2001.
[16] MEHL M J, OSBURN J E, PAPACONSTANTOPOULOUS D A, CLEIN B. Structural properties of ordered high-melting-temperature intermetallic alloys from first-principles total-energy calculations [J]. Physical Review B, 1990, 41: 10311-10323.
[17] MEHL M J. Pressure dependence of the elastic moduli in aluminum-rich Al-Li compounds [J]. Physical Review B, 1993, 47: 2493-2500.
[18] VITOS L, SKRIVER H L, JOHANSSON B, KOLLAR J. Application of the exact muffin-tin orbitals theory: the spherical cell approximation [J]. Computational Materials Science, 2000, 18: 24-38.
[19] VITOS L. Total-energy method based on the exact muffin-tin orbitals theory [J]. Physical Review B, 2001, 64: 014107-1-014107-11.
[20] VITOS L, ABRIKOSOV I A, JOHANSSON B. Anisotropic lattice distortions in random alloys from first-principles theory [J]. Physical Review Letters, 2001, 87: 156401-1-156401-4.
[21] VITOS L. Computational quantum mechanics for materials engineers: the EMTO method and applications [M]. London: Springer-Verlag, 2007.
[22] PERDEW J P, RUZSINSZKY A, CSONKA G I, VYDROV O A, SCUSERIA G E, CONSTANTIN L A, ZHOU X, BURKE K. Restoring the density-gradient expansion for exchange in solids and surfaces [J]. Physical Review Letters, 2008, 100: 136406-1-136406-4.
[23] MURNAGHAN F D. Finite deformations of an elastic solid [J]. American Journal of Mathematics, 1937, 59: 235-260.
[24] BIRCH F. Finite elastic strain of cubic crystals [J]. Physical Review, 1947, 71: 809-824.
[25] ASKER C, VITOS L, ABRIKOSOV I A. Elastic constants and anisotropy in FeNi alloys at high pressures from first-principles calculations [J]. Physical Review B, 2009, 79: 214112-1-214112-9.
[26] TVERGAARD V, HUTSHINSON J W. Microcracking in ceramics induced by thermal expansion or elastic anisotropy [J]. J Am Ceram Soc, 1988, 71: 157-166.
[27] GRIMVALL G. Thermophysical properties of materials [M]. New York: Elsevier, 1999.
[28] HILL R. The elastic behaviour of a crystalline aggregate [J]. Proceedings of the Physical Society A, 1952, 65: 349-354.
[29] VOIGT W. Lehrbuch der Kristallphysik [M]. Teubner Verlag Berlin, Leipzig, 1928.
[30] REUSS A. Berechnung der flie?grenze von mischkristallen auf grund der  einkristalle [J]. Z Angew Math Mech, 1929, 9: 49-58.
einkristalle [J]. Z Angew Math Mech, 1929, 9: 49-58.
[31] PETTIFOR D G. Theoretical predictions of structure and related properties of intermetallics [J]. Materials Science and Technology, 1992, 8: 345-349.
[32] FU L, KE J L, ZHANG Q, TANG B Y, PENG L M, DING W J. Mechanical properties of L12 type Al3X (X=Mg, Sc, Zr) from first-principles study [J]. Physica Status Solidi B, 2012, 249: 1510-1516.
[33] HAYLAND R W, STIFFLER R C. Determination of the elastic constants of polycrystalline Al3Sc [J]. Scripta Metall Mater, 1991, 25: 473-477.
[34]  R. Production, consolidation and investigation of PM Al-Mg-Sc alloys [D]. Germany: Technical University of Clausthal, 1999.
R. Production, consolidation and investigation of PM Al-Mg-Sc alloys [D]. Germany: Technical University of Clausthal, 1999.
[35]  R, KAINER K U. Production of high strength Al-Mg-Sc alloys by PM [J]. Powder Metallurgy, 1998, 41-2: 119-122.
R, KAINER K U. Production of high strength Al-Mg-Sc alloys by PM [J]. Powder Metallurgy, 1998, 41-2: 119-122.
[36] BORN M. On the stability of crystal lattices I [J]. Proc Cambridge Philos Soc, 1940, 36: 160-172.
[37] HIBBELER R C. Mechanics of materials [M]. 8th edition. NJ: Pearson Prentice Hall, 2011: 511.
[38] RAVINDRAN P, FAST L, KORZAUYI P A, JOHANSSON B, WILLS J, ERIKSSON O. Density functional theory for calculation of elastic properties of orthorhombic crystals: application to TiSi2 [J]. Journal of Applied Physics, 1998, 84: 4891-4904.
[39] PUGH S F. Relations between the elastic moduli and plastic properties of polycrystalline pure metals [J]. Philosophical Magazine, 1954, 45: 823-843.
Hamza  OCAK1, Ercan
OCAK1, Ercan  1, Rahmi
1, Rahmi  2
2
1. Department of Physics, Faculty of Arts and Sciences, Dumlupinar University, Kutahya, Turkey;
2. Mechanical Engineering Department, Engineering Faculty, Gazi University, Ankara, Turkey
摘 要:采用气体雾化合金颗粒制备Al-1.1%Sc 和 Al-2%Sc合金。采用X射线衍射分析合金样品以确定样品的晶格参数。利用这些晶格参数,分别通过WIEN2K和EMTO程序对该合金的力学性能进行理论计算。将实验得到的合金的弹性模量和理论计算结果进行比较。讨论了计算得到的Al3Sc相和Al-Sc合金的力学性能以确定Al-Sc合金中最佳的Sc含量。结果表明,最高的Sc含量约为1.0%,更多的Sc不能提高合金的力学性能。
关键词:Al-Sc合金;晶格常数;弹性性能;从头计算;第一性原理
(Edited by Xiang-qun LI)
Corresponding author: Ercan  ; Tel: +90-274-2652031-3107; Fax: +90-274-2652056; E-mail: eucgun@gmail.com
; Tel: +90-274-2652031-3107; Fax: +90-274-2652056; E-mail: eucgun@gmail.com
DOI: 10.1016/S1003-6326(13)62855-1

