J. Cent. South Univ. Technol. (2008) 15: 276-279
DOI: 10.1007/s11771-008-0051-6
Seismic failure mechanisms for loaded slopes with
associated and nonassociated flow rules
YANG Xiao-li(杨小礼), SUI Zhi-rong(眭志荣)
(School of Civil and Architectural Engineering, Central South University, Changsha 410075, China)
Abstract: Seismic failure mechanisms were investigated for soil slopes subjected to strip load with upper bound method of limit analysis and finite difference method of numerical simulation, considering the influence of associated and nonassociated flow rules. Quasi-static representation of soil inertia effects using a seismic coefficient concept was adopted for seismic failure analysis. Numerical study was conducted to investigate the influences of dilative angle and earthquake on the seismic failure mechanisms for the loaded slope, and the failure mechanisms for different dilation angles were compared. The results show that dilation angle has influences on the seismic failure surfaces, that seismic maximum displacement vector decreases as the dilation angle increases, and that seismic maximum shear strain rate decreases as the dilation angle increases.
Key words: earthquake; seismic failure mechanism; soil slope; nonassociated flow rule
1 Introduction
The determination of the seismic failure mechanisms of loaded slopes is a very important issue for most engineers. For example, many bridge abutments, building and retaining walls involve the construction of strip footing on soil slopes. In failure mechanism analysis of geotechnical engineering, the common calculation methods are divided into the following four types, which are the limit equilibrium method, characteristic method, limit analysis method and numerical methods based on either the finite element or finite difference code. The mechanism analysis is often valid for a situation where the soil and rock follow the nonassociated flow rule. However, the published literature is very limited in this area. The mechanical behavior of soil has dilation property. It is well known that the general calculations of loaded slope stability do not involve the dilative influences.
Considering the influences of dilation angle on static problem, many researchers investigated the stability of dilation soil. For example, YIN et al[1] examined the bearing capacity of a strip footing located at the level surface of a dilation soil using the finite difference method, employing the software FLAC. WANG et al[2] investigated the stability of the dilation slopes using the upper bound method in conjunction with the translational failure mechanism. WANG and YIN[3] evaluated the wedge stability of dilation rock. KUMAR[4] analyzed the stability of the dilation slopes using the upper bound method in conjunction with the rotational failure mechanism. Limit analysis method was used to calculated the stability of geotechnical problems with linear and nonlinear failure criterion[5-11]. However, no investigation on seismic failure mechanisms of loaded slopes seems to have been performed.
In this work, the seismic failure mechanisms of loaded slopes were investigated using upper bound and finite difference methods with nonassociated flow rule. The homogenous and isotropic soil slope was loaded by strip force, where the effects of earthquake forces were considered. The earthquake forces can be replaced with different inertia forces. These inertia forces concern the soil mass weight, the surcharge, and the base shear load. The seismic failure mechanisms were investigated for the loaded soil slope where the influence of soil dilation angle was considered.
2 Dilation description
Many experiments have shown that almost types of soils have the nature of dilation[12]. The adoption of the associated flow rule results in an over prediction of soil dilation in upper bound analysis. The introduction of a nonassociated flow rule in upper bound analysis is necessary for a reasonable representation of the soil dilation characteristics. The nonassociated flow rule can be classified into two types. The first type is coaxial nonassociated flow rule, which shows the coaxiality of
the principal directions of stresses and strain rates. The second type is nocoaxial nonassociated flow rule, which shows the noncoincidence of the principal directions of stresses and strain rates. Using the characteristics methods, DRESCHER and DETOURNAY[12] found that the modified cohesion c* and friction angle φ* can be used when the soils obey an nonassociated flow rule, which are the following relationships:
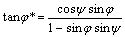 (1)
(1)
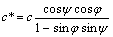 (2)
(2)
where c and φ are the cohesion and friction angle in the linear Mohr-Coulomb failure criterion, and ψ is a dilation angle varying from zero to the internal friction angle φ (0≤ψ≤φ). The φ=ψ means that the soil follows an associated flow rule. Correspondingly, dilation factor, η, which relates the dilation angle to the soil friction angle, is defined as η=ψ/φ. In the present analysis, Eqns.(1) and (2) are considered in the dilation influences using the upper bound and finite difference methods.
3 Upper bound analysis
An earthquake has two kinds of influences on the seismic failure mechanisms of loaded soil slopes. One is to increase the driving forces, and the other is to decrease the shearing resistance of the soil masses. The reduction in the shearing resistance of the soil masses during an earthquake is effective only when the magnitude of the earthquake exceeds a certain limit and the ground conditions are favorable for such a reduction. In the present analysis, only the increase of the driving forces is investigated under an earthquake, and the shearing strength is assumed unaffected. A constant horizontal seismic coefficient kh is assumed for the entire soil masses involved, which is the same as the hypothesis in seismic calculations by some researchers[13-17].
3.1 Upper bound method
The upper bound theorem shows that the rate of work done by actual forces is less than or equal to the rate of energy dissipation in any kinematically admissible velocity fields. A kinematically admissible velocity field is governed by the flow rule, and the velocity field is compatible with the velocities at the boundary. The application of the upper bound theorem leads to upper bounds to the true limit load acting on the materials that are assumed to obey Mohr-Coulomb failure criterion in conjunction with the associated flow rule. The lowest possible upper bound solution is sought with an optimization scheme by trying various possible kinematically admissible failure mechanisms. The potential sliding soil is divided into a number of triangular wedges by a series of inclined straight lines. Each of triangular wedges moves as a rigid wedge. The geometry of wedge i is characterized by the length of the base di, the angles αi and βi (i=1, ???, n), and the length of interface Li (i=1, ???, n-1). The angles αi and βi are unspecified. The wedge velocity and the wedge relative velocity of wedge i with respect to wedge i+1 along the interface are determined by the velocity hodograph. According to the velocity hodograph, if the velocity v1 of the first wedge is given, the velocities and relative velocities of all wedges can be found. In general, the velocity v1 of the first wedge is assumed to be unity for convenience.
3.2 Work and energy calculations
The work rate done by the external load and internal energy dissipation rates can be calculated by superposition. The external rate of work is done by the surcharge q on the inclined surface, the soil mass weight Wi (i=1, ???, n), the ultimate bearing capacity qu, and earthquake forces replaced with the inertia forces. These inertia forces concern the base shear load khqu, the surcharge khq0, and the soil mass weight khWi (i=1, ???, n). Since the soil mass is regarded as perfectly rigid and no general plastic deformation is permitted to occur, the internal energy is dissipated only along velocity discontinuity di (i=1, ???, n) between the soil at rest and the soil in motion, and along the relative velocity discontinuity interface Li (i=1, ???, n-1) between adjoining two wedges.
Employing the velocity field for the translational failure mechanism, the work rates and internal energy dissipation rates can be calculated. Since the rock masses are regarded as perfectly rigid materials and no general plastic deformation is permitted to occur, the internal energy is dissipated only along velocity discontinuity base di (i=1, ???, n) between the material at rest and the material in motion, and along the relative velocity discontinuity interface Li (i=1, ???, n-1) between adjoining two wedges, which is
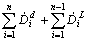 (3)
(3)
where the first term is the summation of energy dissipation along the base of each wedge, and the second term is the summation of energy dissipation along the interface between adjoining two wedges. While the external work rate are done by the surcharge load, the weight of each wedge, the pressure acting on the footing and inertia forces, which can be expressed as
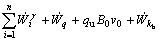 (4)
(4)
where B0 is the load width, q is the equivalent
surcharge load, and v0 is the velocity of load qu. In
Eqn.(4), 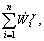
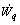 , quB0v0 and
, quB0v0 and 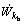 are work rates
are work rates
of external loads related to the unit weight γ of wedges (i=1, ???, n), the surcharge load q, the load qu and the inertia forces, respectively. Equating the work rates of external loads to the total internal energy dissipation rates, the general equation of the failure mechanism can be obtained by using the upper bound method, which is
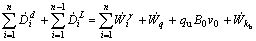 (5)
(5)
Due to the assumption of the rough between the footing and rock masses, no relative slip occurs, and the velocity of the first wedge is also v0. It is assumed that v0 is unit since the absolute value of the velocity has no influence on the final results. Based on the upper bound theorem of limit analysis, the failure mechanism is obtained when Eqn.(5) is minimized with respect to αi and βi (i=1, ???, n) that determine the failure mechanism.
4 Failure mechanisms with dilation
By the upper bound theorem of limit analysis, the failure mechanisms have been obtained by minimizing objective function with respects to αi and βi (i=1, ???, n). The failure mechanisms can be improved by increasing the number of triangular wedges n. In the present calculations, the triangular wedge number n is equal to 15, which means that the minimization procedure is made with regard to 30 variables and a constraint (∑αi= π-θ). The failure mechanisms are presented in Fig.1. For the completeness, using the finite difference method, the seismic maximum displacement vector figure and the seismic maximum shear strain rate figure for different dilation angles are presented for practice use in engineering.
4.l Influence of earthquake
To investigate how the critical slip surface is influenced by earthquake forces when the foundation rests on a soil slope, Fig.1 shows the critical slip surfaces with associated flow rule corresponding to θ=15?, φ=35?, q=γ=0 and η=1.0, while the horizontal seismic coefficient kh is equal to 0.10, 0.30 and 0.50. Using the upper bound method of limit analysis theory, it is found from Fig.1 that the failure surfaces become shallower as the seismic coefficient kh increases.
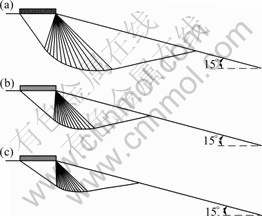
Fig.1 Seismic failure surfaces with associated flow rule for θ=15? and φ=35?: (a) kh=0.10; (b) kh=0.30; (c) kh=0.50
4.2 Influence of dilation angle on displacement vector
The elastic-plastic model and the linear Mohr-Coulomb failure criterion are used in the finite difference numerical simulation. The elastic modulus is E=30 MPa, and the Posson ratio is υ=0.3. Unit weight of soil is γ=20 kN/m3, and the cohesion and internal friction angle are c=10 kPa and φ=30?, respectively. Fig.2 illustrates the displacement vector plots for the loaded slope. It is noted that the maximum seismic magnitude of displacement vector varies with the dilation angle. For example, the seismic maximum displacement vector is 1.005 m for kh=0.2 and η=0 in Fig.2(a), is 0.756 4 m for kh=0.2 and η=0.4 in Fig.2(b), and is 0.299 9 m for kh=0.2 and η=1.0 in Fig.2(c). From Fig.2, it is found that maximum seismic displacement vector decreases as the dilation angle increases.

Fig.2 Seismic displacement vector: (a) kh=0.2, η=0; (b) kh= 0.2, η=0.4; (c) kh=0.2, η=1.0
4.3 Influence of dilation angle on shear strain rate
The influences of shear strain on the dilative soil slope subjected to strip load and earthquake force are investigated corresponding to the same problem depicted in Fig.2. It is found that the size of shear zone decreases with decreasing dilation angle. The seismic maximum shear strain rate is 4.55×10-4 s-1 for kh=0.3 and η=0, is 2.98×10-6 s-1 for kh=0.3 and η=0.4, and is 3.40×10-7 m for kh=0.3 and η=1.0. With the increase of earthquake force and dilation factor, maximum shear strain rate decreases, and shear strain zone becomes shallow, which resembles the failure mechanisms depicted in Fig.3.
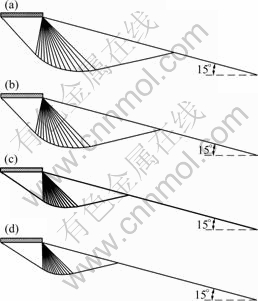
Fig.3 Seismic failure surfaces with nonassociated flow rule for θ=15? and φ=35?: (a) kh=0.2 and η=0.8; (b) kh=0.2 and η=0.4; (c) kh=0.4 and η=0.8; (d) kh=0.4 and η=0.4
4.4 Dilation angle influence on slip surfaces
Fig.3 illustrates effects of the nonassociated flow rule on the critical slip surfaces corresponding to θ=15?, φ=35? and q0=γ=0. From Fig.3, it is found that the dilation factor η has small influences on the critical slip surfaces, with the horizontal seismic coefficient kh being 0.20. The same phenomenon can also be found in Fig.3 corresponding to kh=0.4.
5 Conclusions
1) Incorporating the effects of horizontal earthquake forces, the failure mechanisms of loaded slopes are investigated using an upper bound and finite difference methods, with associated and nonassociated flow rules. The failure mechanisms are presented.
2) The effects of the seismic coefficient kh on seismic critical slip surface are discussed. The seismic failure surfaces become shallow as the earthquake force increases.
3) The dilation angle has influences on the seismic failure surfaces. The seismic maximum displacement vector decreases as the dilation angle increases, and the seismic maximum shear strain rate decreases as the dilation angle increases.
References
[1] YIN Jian-hua, WANG Yu-jie, SELVADURAI A P S. Influence of non-association on bearing capacity of a strip footing [J]. Journal of Geotechnical and Geoenvironmental Engineering, 2001, 127(11): 985-989.
[2] WANG Yu-jie, YIN Jian-hua, LEE C F. The influence of a non-associated flow rule on the calculation of the factor of safety of the soil slopes [J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2001, 25(13): 1351-1359.
[3] WANG Yu-jie, YIN Jian-hua. Wedge stability analysis considering dilatancy of discontinuities [J]. Rock Mechanics and Rock Engineering, 2002, 35(2): 127-137.
[4] KUMAR J. Stability factors for slopes with nonassociated flow rule using energy consideration [J]. International Journal of Geomechanics, 2004, 4(4): 264-272.
[5] YANG Xiao-li, YIN Jian-hua. Linear Mohr-Coulomb strength parameters from the nonlinear Hoek-Brown rock masses [J]. International Journal of Non-Linear Mechanics, 2006, 41(8): 1000-1005.
[6] YANG Xiao-li, ZOU Jin-feng. Stability factors for rock slopes subjected to pore water pressure based on the Hoek-Brown failure criterion [J]. International Journal of Rock Mechanics and Mining Sciences, 2006, 43(7): 1146-1152.
[7] YANG Xiao-li, YIN Jian-hua. Upper bound solution for ultimate bearing capacity with a modified Hoek-Brown failure criterion [J]. International Journal of Rock Mechanics and Mining Sciences, 2005, 42(4): 550-560.
[8] YANG Xiao-li, LI Liang, YIN Jian-hua. Stability analysis of rock slopes with a modified Hoek-Brown failure criterion [J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2004, 28(2): 181-190.
[9] YANG Xiao-li, YIN Jian-hua. Slope stability analysis with nonlinear failure criterion [J]. Journal of Engineering Mechanics, ASCE, 2004, 130(3): 267-273.
[10] YANG Xiao-li, WANG Zhi-bin, ZOU Jin-feng, LI Liang. Bearing capacity of foundation on slope determined by energy dissipation method and model experiments[J]. Journal of Central South University of Technology, 2007, 14(1): 125-128.
[11] YANG Xiao-li, GUO Nai-zheng, ZHAO Lian-heng, ZOU Jin-feng. Influences of nonassociated flow rules on seismic bearing capacity factors of strip footing on soil slope by energy dissipation method [J]. Journal of Central South University of Technology, 2007, 14(6): 842-847.
[12] DRESCHER A, DETOURNAY C. Limit load in translational failure mechanisms for associative and non-associative materials[J]. Geotechnique, 1993, 43(3): 443-456.
[13] BUDHU M, AL-KARMI A. Seismic bearing capacity of soils [J]. Geotechnique, 1993, 43(1): 181-187.
[14] YANG Xiao-li. Upper bound analysis of active earth pressure with different fracture surface and nonlinear yield criterion [J]. Journal of Theoretical and Applied Fracture Mechanics, 2007, 47(1): 46-56.
[15] YANG Xiao-li, YIN Jian-hua. Estimation of seismic passive earth pressure with non-linear failure criterion [J]. Engineering Structures, 2006, 28(3): 342-348.
[16] YANG Xiao-li, LI Liang, YIN Jian-hua. Seismic and static stability analysis for rock slopes by a kinematical approach [J]. Geotechnique, 2004, 54(8): 543-549.
[17] RICHARDS R, ELMS D, BUDHU M. Seismic bearing capacity and settlement of foundations [J]. Journal of Geotechnical and Geoenvironmental Engineering, ASCE, 1993, 119(4): 662-674.
(Edited by CHEN Wei-ping)
Foundation item: Project(200550) supported by the Foundation for the Author of National Excellent Doctoral Dissertation of China; Project(200631878557) supported by the West Traffic of Science and Technology, China
Received date: 2007-09-19; Accepted date: 2007-12-28
Corresponding author: YANG Xiao-li, Professor; Tel: +86-731-2656248; E-mail: yxnc@yahoo.com.cn