J. Cent. South Univ. (2019) 26: 2930-2942

DOI: https://doi.org/10.1007/s11771-019-4225-1

Modified constitutive model and workability of 7055 aluminium alloy in hot plastic compression

ZHANG Tao(张涛)¹, ZHANG Shao-hang(张少航)¹, LI Lei(李磊)¹,LU Shi-hong(鲁世红)¹, GONG Hai(龚海)²

1. College of Mechanic and Electrical Engineering, Nanjing University of Aeronautics and Astronautics,Nanjing 210016, China;

2. State Key Laboratory of High Performance Complex Manufacturing, Central South University,Changsha 410083, China

Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2019

Abstract: To obtain flow behavior and workability of 7055 aluminium alloy during hot deformation, hot compression tests at different temperatures and strain rates are conducted. True stress-strain curves of 7055 aluminium alloy under different conditions are obtained and the flow stress increases with ascending strain rate and descending temperature. For Arrhenius constitutive equation, each material parameter is set as a constant, which will bring forth large error for predicting flow behavior. In this work, material parameters are fitted as a function of temperature or strain rate based on experimental results and a modified constitutive equation is established for more accurate prediction of flow behavior of 7055 aluminium alloy. The effects of temperature and strain rate on power dissipation and instability are analyzed to establish a processing map of 7055 aluminium alloy. The dominant deformation mechanism for microstructure evolution at different deformation conditions can be determined and high efficiency of power dissipation may be achieved from power dissipation map. Meanwhile, proper processing parameters to avoid flow instability can be easily acquired in instability map. According to the processing map, optimized processing parameters of 7055 aluminium alloy are temperature of 673-723 K and strain rate of 0.01-0.4 s^-1, during which its efficiency of power dissipation is over 30%. Finite element method (FEM) is used to obtain optimized parameter in hot rolling process on the basis of processing map.

Key words: 7055 aluminium alloy; flow behavior; modified constitutive equation; processing map; optimized parameters

Cite this article as: ZHANG Tao, ZHANG Shao-hang, LI Lei, LU Shi-hong, GONG Hai. Modified constitutive model and workability of 7055 aluminium alloy in hot plastic compression [J]. Journal of Central South University, 2019, 26(11): 2930-2942. DOI: https://doi.org/10.1007/s11771-019-4225-1.

1 Introduction

Aluminium alloy is a kind of key material used in aerospace field for its excellent comprehensive performances. Key structures make aluminium alloy plates play a significant role in weight reduction of airplane [1]. With T77 heat treatment, better properties of strength and corrosion resistance can be obtained for 7055 aluminium alloy compared to other 7XXX aluminium alloys. Hot working process plays an important role in complex components forming and enhancement of material properties. Flow stress is an important parameter in hot working and it varies with changes of temperature, strain and strain rate.

Therefore, relationships between flow stress and strain, strain rate and temperature are necessarily to be studied and the constitutive equation of the material during hot working process should be established. Mechanical properties depend on the microstructure inside the material and the microstructure varies with different processing parameters. Meanwhile, hot processing parameters will change the deformation mechanism, which will affect the microstructure evolution. For example, work hardening occurs at low temperature and the microstructure turns to banded structure with ascending strain; however, with the increase of temperature and strain, dynamic recrystallization may occur and the deformed structure will change to equiaxed grains. Therefore, different deformation mechanism can be obtained by adjusting processing parameters, such as strain, temperature and strain rate. In some cases, there may be failure of hot working process due to flow instability of the material at improper deformation parameters. Therefore, optimized hot working parameters should be studied to guarantee smooth hot forming and obtain excellent mechanical properties.

Many studies [2-4] had been conducted on flow behavior and constitutive equations of different materials. CISSE et al [5] reviewed main constitutive models for shape memory alloys, including micromechanics with or without scale transition, classical plasticity methods, energy approaches coupled with thermodynamic and conservation principles.WEI et al [6] studied the relationships between flow stress, strain rate and temperature by Arrhenius equation. ZHANG et al [7] established a two-stage constitutive model, which considered work hardening-dynamic recovery and dynamic recrystallization based on dislocation density theory and dynamic recrystallization kinetics. WANG et al [8] established a revised strain-dependent hyperbolic sine constitutive model, in which the material parameters varied as functions of the strain and strain rate.CAI et al [9] proposed a new kind of modified parallel constitutive model considering the independent effects of strain, strain rate and temperature of Ti-6Al-4V alloy. LIN et al [10] established Arrhenius type phenomenological models combining multi-gene genetic programming and artificial neural network for prediction of the flow stress. XIAO et al [11] found that when predicting flow stress, the accuracy of Avrami-type model is higher than that of the Arrhenius-type constitutive equation of Al-Zn-Mg-Cu alloy. CAI et al [12] made a comparison on the capability of the three constitutive models (Johnson Cook, Modified Zerilli-Armstrong and Arrhenius-type) and found that the accuracy of Johnson Cook model is lower than that of other two models for flow behavior of Ti-6Al-4V alloy. For 7XXX aluminium alloy, YAN et al [13] analyzed flow stress characteristics considering work hardening and dynamic softening at hot deformation.

In addition to studies of constitutive equations of metals during hot forming process, many researchers studied its processing map to obtain optimized forming parameters. ZHOU et al [14] constructed the processing maps of AZ61 magnesium alloy via the dynamic material model (DMM) and studied the microstructure evolution by optical microscopy and transmission electron microscopy. BABU et al [15] developed processing maps of super austenitic stainless steel at different strains and characterized microstructures corresponding to different conditions by electron back scatter diffraction (EBSD). JIN et al [16] found that flow instability with bands of flow localization and cracking usually occurred at low temperature and high strain rate from analysis of hot workability of Mg-3.7Gd-2.9Y-0.7Zn-0.7Zr alloy. KUMAR et al [17] studied strain rate sensitivity maps and processing maps using DMM and modified DMM with different instability criteria. BASANTH et al [18] developed processing maps on the basis of true stress-strain curves and studied the microstructure evolution of different strains. RIEIRO [19] presented a new stability criterion using single sine hyperbolic equation of Garofalo for all values of the thermomechanical variables, which simplified operations and minimized errors.

Arrhenius constitutive equations were widely used to describe flow behavior of metal materials during hot compression and the material parameters in the equation were set as constants. However, for 7055 aluminium alloy, research on flow behavior and workability during hot deformation was rarely published. From the experimental true stress-strain curves, material parameters changed greatly at different temperatures and strain rates, and therefore, constant material parameters were inadequate for prediction of flow behavior for 7055 aluminium alloy. Moreover, small ranges of temperature and strain rate should be applied to industrial hot working process for good and stable mechanical performances. Therefore, how to acquire optimized processing parameters efficiency improvement of power dissipation and excellent mechanical properties need to be further studied. Processing maps are essential for the selection of hot deformation parameters and avoidance of flow instability. In this study, hot compression tests of 7055 aluminium alloy at different temperatures and strain rates were conducted. Material parameters were fitted as the function of temperature and strain rate, then a modified Arrhenius constitutive equation was established. A processing map was developed to characterize microstructure evolution mechanism at different deformation conditions and acquired optimized hot processing parameters.

2 Experiments

The material was 7055 aluminium alloy ingot casting and it was machined into cylindrical specimens with a height of 15 mm and a diameter of 10 mm. Before hot compression test, homogenization treatment was conducted to obtain equiaxed grains and eliminate casting segregation. The hot compression tests were conducted on Gleeble-3180 thermo-simulation machine to study the flow behavior of 7055 aluminium alloy. Four different strain rates0.01, 0.1, 1, 10 s^-1) and four different temperatures (T=573, 623, 673, 723 K) were adopted in this study. The total true strain was 0.693 and specimens were quenched immediately to reserve microstructure after hot compression.

Figure 1 shows true stress-strain curves of 7055 aluminium alloy for different temperatures and strain rates. The true stress increases with ascending strain rate and descending temperature. The variation of flow stress depends on two processes: work hardening and dynamic softening (including dynamic recovery and dynamic recrystallization). The flow stress first increases sharply with ascending strain for intensive work hardening as the dislocation inside the material increases significantly. Then the flow stress almost keeps steady for the dynamic balance of dynamic softening and work hardening, because the deformation energy accumulated in work hardening provides driving force for dislocation decrease in dynamic softening process.

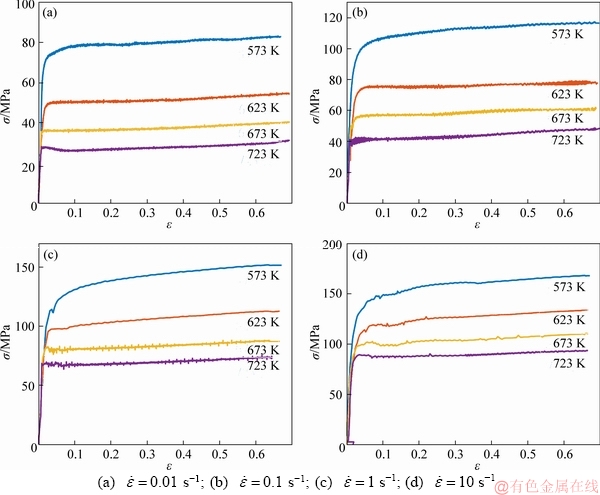
Figure 1 True stress-strain curves of 7055 aluminium alloy during hot compression:

3 Modified constitutive equation

Based on the above results, it is obvious that flow stress is quite sensitive to temperature and strain rate. The relationship between flow stress and deformation parameters is quite important in hot forming process. Therefore, a constitutive equation should be established to have a better understanding of the deformation of the material. The constitutive equations for different stress levels during hot compression are shown in Eq. (1). It can be seen that the flow stress is related to temperature and strain rate. As stress exponent n is a key parameter in determining the accuracy of the constitutive equations, successive approximation method [20] is used to calculate the value of n, as shown in Figure 2. First, the stress exponent for low stress level n₁ and stress coefficient β for high stress level are calculated. Then, the stress exponent for high stress level n₂ and activation energy Q_act are obtained. Finally, the stress exponent n for all stress level is fitted, which is the function of temperature.

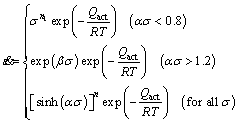       (1)

where n₁ and n are stress exponent for low strress level and all strress level; Q_act is the activation energy, J/mol; is the strain rate, s^-1; R is the ideal gas constant, 8.314J/(K·mol); T is the absolute temperature, K;is the flow stress, MPa; A, β and α are material parameters.

Figure 2 Steps of successive approximation method for solution of stress exponent n

Least square method is used to obtain different material parameters in Arrhenius equation. Usually, the average values of slopes or intercepts at different temperatures or strain rates are adopted in Arrhenius equation. However, the values of material parameters change greatly with variation of temperature or strain rate from experimental results. If only using the average values, large error will be introduced as small changes of temperature or strain rate will significantly change the material parameters. Therefore, each material parameter is fitted as the function of temperature or strain rate in this study. Then, a modified constitutive equation can be obtained to accurately describe the flow behavior of 7055 aluminium alloy during hot compression. The fitting steps are described as follows:

For low stress level (ασ<0.8) and high stress level (ασ>1.2), stress exponent n₁ for low stress level and material parameter β can be calculated by Eqs. (2)-(3).

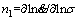                            (2)

                              (3)

Figure 3 shows the relationship between 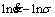andThe slopes at different temperatures are 10.10(573 K), 7.84(623 K), 6.47(673 K) and 5.77(723 K), which vary greatly and it indicates that adoption of average value will bring large error. Similarly, the value of β in Figure 3(b) also changes a lot with temperature variation. According to Eq. (4), the value of α at each temperature can be calculated and its relation to temperature is shown in Figure 4. The value of α is linear to temperature and it can be expressed as a function of temperature by linear fitting, as shown in Eq. (5).

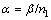                                (4)

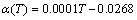                    (5)

Stress exponent n needs to be successive fitted to obtain its accurate value for all stress level. According to Figure 2, after calculation of n₁ for low stress level and α for high stress level, the stress exponent and activation energy for all stress level are calculated. As shown in Eq. (6), the n₂ can be calculated from the relationship between 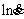and ln[sinh(as)]. Single variable method is used in the fitting process, and therefore, the fitting of n₂ should be under the the premise of constant temperature. Similarly, the Q_act can be calculated from the relationship between ln[sinh(as)] and 1/T under the premise of constant strain rate. The values of n₂ and material constant (K) can be calculated, as shown in Eqs. (6)- (7) and Figure 5.

Figure 3 Relationships between:

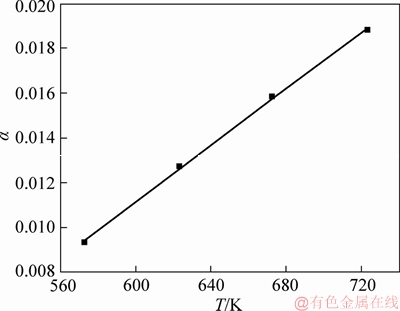
Figure 4 Relationship between α and T

                        (6)

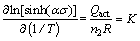                    (7)

The values of n at different temperatures are calculated from Figure 5(a) and they are linear to temperature, as shown in Figure 6(a). Linear function is used to describe the relationship, as shown in Eq. (8). The value of K changes with strain rate variation in Figure 5(b) and it can be fitted by polynomial, as shown in Figure 6(b) and Eq. (9). As a result, according to Eq. (7), the activation energy Q_act can be expressed as the function of temperature and strain rate. Figure 7 shows that the activation energy decreases with ascending temperature and descending strain rate and the predicted values according to Eq. (7) agree with experimental values:

                    (8)

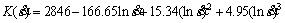 (9)

By calculating the intercepts of four lines in Figure 5(a), the value of A can be obtained and its relationship with temperature is shown in Figure 8. Quadratic function can be used to describe the relation and the result is shown in Eq. (10). By substituting Eqs. (8)-(9) into Eq. (10), the value of A can be obtained.

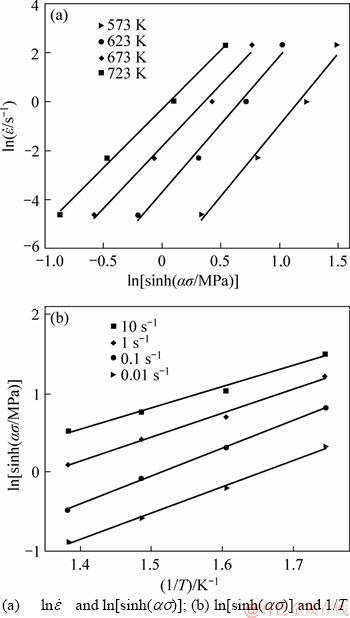
Figure 5 Relationships between:

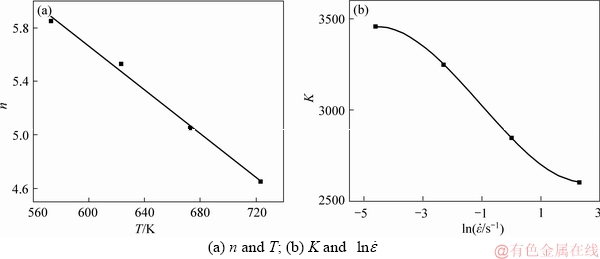
Figure 6 Relationships between:

Figure 7 Predicted activation energy Q_act (a) and its comparison to experimental activation energy (b)

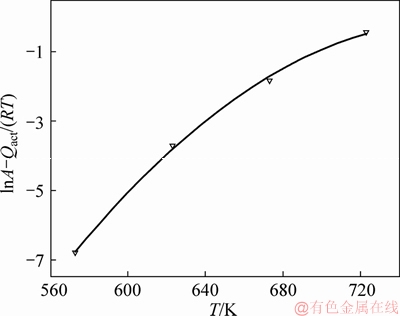
Figure 8 Relationship between lnA-Q_act/(RT) and T

 (10)

Therefore, the modified constitutive equation of 7055 aluminium alloy is obtained and the material parameters are expressed as the function of temperature and strain rate, as shown in Eq. (11):

     (11)

In order to verify the accuracy of the modified constitutive equation, comparisons between calculated flow stress from two types of constitutive equation and experimented results are shown in Figure 9. The maximum error in Figure 9(a) is 10.52% and it locates at 573 K and 0.01 s^-1, which agrees with the discussion in Figure 3(a). The value of n₁ at 573 K is much larger than that at other temperatures, and therefore, the average value brings big error. The maximum error in Figure 9(b) is 4.75% and it illustrates that modified constitutive equation can predict flow behavior more accurately.

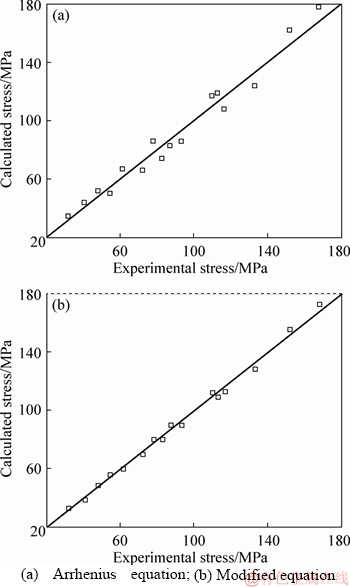
Figure 9 Comparisons between calculated and experimented flow stress:

4 Processing map

Processing map, based on dynamic material model, is an effective means to analyze the feasibility of hot working process. It provides guidance for judgement for softening mechanism at different deformation conditions as well as analysis of stability region and instability region. On the basis of processing map, optimized process parameters can be obtained and the defects of adiabatic shear band and cavity can be avoided during hot working. A processing map is constructed by superimposition of the instability map over the power dissipation map.

4.1 Power dissipation map

PRASAD et al [21] proposed DMM on the basis of continuum mechanics of large plastic deformation, physical system model and irreversible thermodynamics. The dynamic response characteristics were described in this model and the processed material was considered as a non-linear energy dissipation unit. During dynamic softening process, the flow stress at a given temperature depended on strain rate, while the strain had little effect on the variation of flow stress.

Based on DMM established by PRASAD et al [22], the instantaneous power dissipated (P) consists of two parts: G content (heat energy caused by plastic deformation) and J content (microstructure evolution energy used for dynamic recovery, recrystallization and phase transformation). The instantaneous power dissipated is the function of strain rate and flow stress, as shown in Eq. 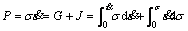(12).

            (12)

It is known that flow stress is related to temperature, strain and strain rate, as shown in Eq. (13). As discussed in DMM above, the flow stress depended on strain rate for the given temperature and strain. Therefore, the strain rate sensitivity can be calculated, which is the most important parameter in processing map.

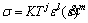                          (13)

where j and l are exponents of temperature and strain, respectively; m is the strain rate sensitivity and it can be calculated by Eq. (14), which represents the partition of the power into G content and J content. The J content will reach its maximum value J_max when m is equal to 1, as shown in Eq. (14).

                   (14)

In order to quantificational describe the energy used for microstructure evolution, a parameter η is defined in Eq. (15).

                 (15)

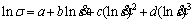In order to improve the accuracy of m, cubic spline function is used to describe the relationship between lnσ andas shown in Eq. (16).

            (16)

According to Eq. (14), values of m under different temperatures are calculated, as shown in Figure 10. Therefore, the effects of temperature and strain rate on parameter η can be obtained and the power dissipation map is shown in Figure 11.It is obvious that the parameter η increases with ascending temperature and descending strain rate and contour lines are intensive at high strain rate, which indicates that parameter η is quite sensitive to strain rate. The peak value of parameter η appears on temperature range of 673-723 K and strain rate range of 0.01-0.1 s^-1, where dynamic recrystallization is the main deformation mechanism, while dynamic recovery mainly occurs on low temperature and high strain rate.

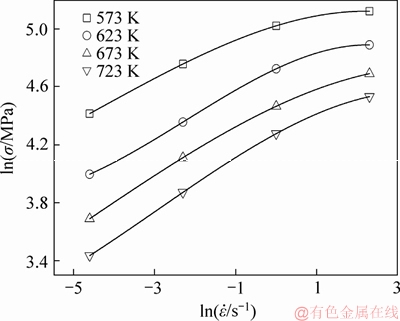
Figure 10 Relationship between lnσ and

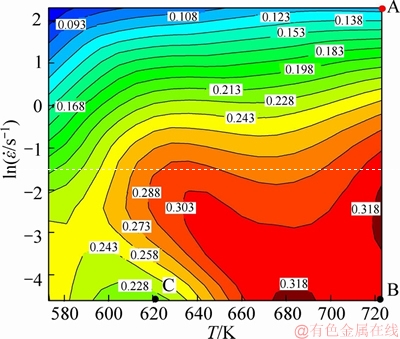
Figure 11 Effects of temperature and strain rate on power dissipation map at strain of 0.693

4.2 Instability map

Deformation mechanism under different deformation conditions can be judged by power dissipation map; however, the flow instability region during hot deformation can not be determined. Based on the principle of the maximum rate of entropy of production, a continuum criterion [23, 24] for the occurrence of flow instability is defined in Eq. 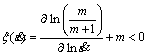(17).

                  (17)

where ξ is instability parameter and it is the function of temperature and strain rate. Figure 12 shows the effects of temperature and strain rate on instability parameter ξ, which represents instability map. The flow instability regions locate at the places where the value of ξ is negative. Large strain rate contributes to inducing flow instability and the lower limiting value of strain rate to induce flow instability increases with ascending temperature. For example, the lower limiting value of strain rate is 0.4 s^-1 at 573 K, while it changes to 1 s^-1 at 723 K.

Figure 12 Effects of temperature and strain rate on instability map at strain of 0.693

A processing map can be acquired by superimposition of the instability map over the power dissipation map [25]. It determines the dominant mechanism for microstructure evolution and shows the limiting conditions for avoidance of flow instability. The thermal deformation will affect the microstructure evolution of 7055 aluminium alloy during hot compression. There are two mechanisms: work hardening and dynamic softening (including dynamic recovery and dynamic recrystallization). The grains will be elongated through deformation direction and compressed vertical to deformation direction in work hardening, and banded structure can be obtained. The banded structure will turn to equiaxed grains in dynamic recrystallization due to the nucleation and growth of recrystallized grains.

The EBSD micrograph in Figure 13(a) corresponds to point A in Figure 11. The initial microstructure is elongated into banded structure at high temperature during hot compression. Only a small quantity of equiaxed grains with small size appear at grain boundaries. There are small equiaxed grains as the forming temperature exceeds critical temperature for dynamic recrystallization. However, the dynamic nuclei hardly grow up at high strain rate due to lack of enough time for growth. Microstructure in Figure 13(b) corresponds to point B in Figure 11, in which the grains are obviously refined due to dynamic recrystallization under high temperature and small strain rate. The refined grains are most generated in grain boundaries for its large stacking fault energy as the dislocation density provides driving force for grain growth. In Figure 13(c), the microstructure turns to banded structure, but the width of the banded structure is smaller than that in Figure 13(a). The deformation resistance is larger due to lower temperature, as a result, the dynamic recrystallization hardly occurs at low temperature of 623 K. Meanwhile, it can be seen that there are small internal subgrains in Figures 13(a) and (c) as well as jagged grain boundaries, which is caused by the cross slipping and climbing of dislocation during hot compression inside the material. According to Ref. [26], the instability domain occurs at low deformation temperature (613 to 653 K), the primary softening mechanism was dynamic recovery when the alloys were deformed at low temperature (633 K) and high strain rate (0.1 and 1 s^-1). It can be seen that point A locates at flow instability regions from Figure 12. The instability can be caused by cracks, adiabatic shear bands, flow localization, cavity nucleation, and adiabatic shear bands and flow localization are the two basic mechanisms for damages. Stress concentration caused by sliding of the neighbouring grains and brittle Fe-rich particles formed during solution process before hot compression both have vital effect on instability [25]. The recrystallised grain fraction obviously increases at high deformation temperature and small strain rate, indicating that the main softening mechanism was dynamic recrystallisation. In this study, the results are similar to them in Ref. [27]. From the processing map, optimized processing parameters are temperature of 673-723 K and strain rate 0.01-0.4 s^-1, during which the efficiency of power dissipation is over 30% and flow instability can be avoided.

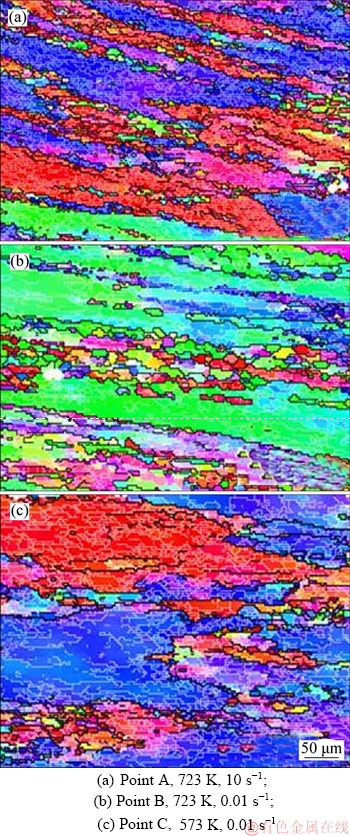
Figure 13 EBSD micrographs of aluminium alloy 7055 corresponding to different points in Figure 11:

5 Numerical simulation for hot rolling process

Hot rolling is the key process of plate preparation and plays a vital role in grain refinement and improvement of mechanical properties. However, the temperature, strain and strain rate vary at different positions of the plate and different time during hot rolling process. Finite element models are used to obtain optimized parameters of hot rolling. HU et al [27] obtained the optimized parameters of rib-web forgings for 6061 aluminum alloy through finite element method (FEM) simulation. The rolls are defined as rigid bodies and the plate is defined as plastic body and its constitutive equation is described in Eq. (11). The rolling parameters are shown in Table 1. The rolling velocity will affect the temperature distribution and the strain rate of the plate, which is studied as an example for the application of processing map.

The effects of rolling velocity on temperature distribution is shown in Figure 14. The surface temperature decreases obviously during rolling process due to the large heat exchange with rolls. During hot rolling process, emulsion is adopted as lubricant, which will decrease the surface temperature. The surface temperature will increase when the plate moves out of the roller gap as there is heat conduction between the center layer and the surface of the plate. It can be seen the depth of temperature decrease is large under small rolling velocity and this depth decreases with ascending rolling velocity. The temperature at center layer will increase due to the heat induced by large deformation. However, the temperature at center layer is almost the same under different rolling velocities. The surface temperature increases from 593 to 660 K when the rolling velocity changes from 0.25 to 1.5 m/s. Figure 15 shows the effects of rolling velocity on strain rate distribution. The strain rate at center layer and the maximum strain rate both increase with increasing rolling velocity. The strain rate at center layer increases sharply when rolling velocity is larger than 0.5 m/s. According to Figure 11, the power dissipation efficiency at center layer of the plate is 0.243, 0.228, 0.153 and 0.108 for rolling velocity changing from 0.25 to 1.5 m/s. Meanwhile, the power dissipation efficiency at surface is about 0.1 for 0.25 m/s and 0.5 m/s, and it decreases obviously with increasing rolling velocity. Therefore, rolling velocity of 0.5 m/s is the best by taking into consideration of the power dissipation efficiency at surface and center layer of the plate. In addition, the layer depth of low temperature at 0.5 m/s is much larger than that at 0.25 m/s.

Table 1 Rolling parameters

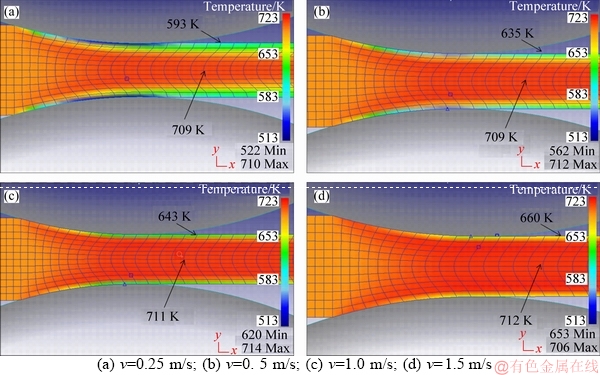
Figure 14 Effect of rolling velocity on temperature distribution:

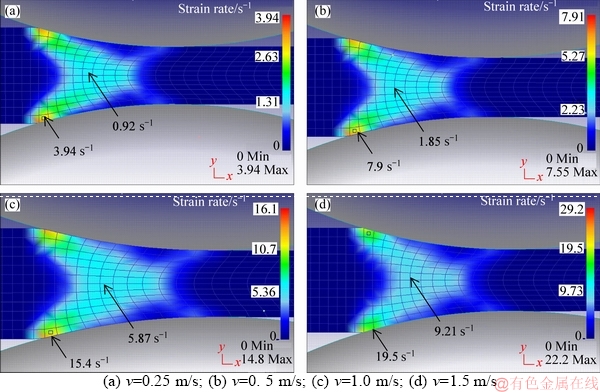
Figure 15 Effect of rolling velocity on strain rate distribution:

6 Conclusions

1) True stress-strain curves of 7055 aluminium alloy during hot compression are obtained and the flow stress increases with ascending strain rate and descending temperature.

2) A modified constitutive equation of 7055 aluminium alloy is established and it has higher accuracy than Arrhenius equation in predicting flow behavior during hot processing.

3) The effects of temperature and strain rate on efficiency of power dissipation and flow instability are studied and then a processing map is constructed.

4) Based on processing map, optimized processing parameters of 7055 aluminium alloy are temperature of 673-723 K and strain rate 0.01-0.4 s^-1.

5) Numerical simulation for hot rolling process is conducted and optimization of parameter and workability characterization can be realized on the basis of processing map.

References

[1] HEINZ A, HASZLER A, KEIDEL C, MOLDENHAUER S, BENEDICTUS R, MILLER W S. Recent development in aluminium alloys for aerospace applications [J]. Materials Science and Engineering A, 2000, 280(1): 102-107. DOI: 10.1016/s0921-5093(99)00674-7.

[2] QIAN Dong-sheng, PENG Ya-ya, DENG Jia-dong. Hot deformation behavior and constitutive modeling of Q345E alloy steel under hot compression [J]. Journal of Central South University, 2017, 24(2): 284-295. DOI: 10.1007/s11771-017-3429-5.

[3] WANG Meng-han, WANG Gen-tian, WANG Rui. Flow stress behavior and constitutive modeling of 20MnNiMo low carbon alloy [J]. Journal of Central South University, 2016, 23(8): 1863-1872. DOI: 10.1007/s11771-016-3241-7.

[4] LI Bo, PAN Qing-lin, LI Chen, ZHANG Zhi-ye, YIN Zhi-min. Hot compressive deformation behavior and constitutive relationship of Al-Zn-Mg-Zr alloy with trace amounts of Sc [J]. Journal of Central South University, 2013, 20(11): 2939-2946. DOI: 10.1007/s11771-013-1816-0.

[5] CISSE C, ZAKI W, BEN ZINEB T. A review of constitutive models and modeling techniques for shape memory alloys [J]. International Journal of Plasticity, 2016, 76: 244-284. DOI: 10.1016/j.ijplas.2015.08.006.

[6] WEI Guo-bing, PENG Xiao-dong, HU Fa-ping, HADADZADEH A, YANG Yan, XIE Wei-dong, WELLS M A. Deformation behavior and constitutive model for dual-phase Mg–Li alloy at elevated temperatures [J]. Transactions of Nonferrous Metals Society of China, 2016, 26(2): 508-518. DOI: 10.1016/S1003-6326(16)64139-0.

[7] ZHANG Peng, YI Cen, CHEN Gang, QIN He-yong, WANG Chuan-jie. Constitutive model based on dynamic recrystallization behavior during thermal deformation of a nickel-based superalloy [J]. Metals, 2016, 6(7): 161. DOI: 10.3390/met6070161.

[8] WANG L, LIU F, CHENG J J, ZUO Q, CHEN C F. Arrhenius-type constitutive model for high temperature flow stress in a nickel-based corrosion-resistant alloy [J]. Journal of Materials Engineering and Performance, 2016, 25(4): 1394-1406. DOI: 10.1007/s11665-016-1986-7.

[9] CAI Jun, SHI Jia-min, WANG Kuai-she, LI Fu-guo, WANG Wen, WANG Qing-juan, LIU Ying-ying. A modified parallel constitutive model for elevated temperature flow behavior of Ti-6Al-4V alloy based on multiple regression [J]. International Journal of Materials Research, 2017, 108(7): 527-541. DOI: 10.3139/146.111514.

[10] LIN Y C, NONG Fu-qi, CHEN Xiao-min, CHEN Dong-dong, CHEN Ming-song. Microstructural evolution and constitutive models to predict hot deformation behaviors of a nickel-based superalloy [J]. Vacuum, 2017, 137: 104-114. DOI: 10.1016/j.vacuum.2016.12.022.

[11] XIAO Dan, PENG Xiao-yan, LIANG Xiao-peng, DENG Ying, XU Guo-fu, YIN Zhi-min. Research on constitutive models and hot workability of as-homogenized Al-Zn-Mg-Cu alloy during isothermal compression [J]. Metals and Materials International, 2017, 23(3): 591-602. DOI: 10.1007/s12540-017-6526-y.

[12] CAI Jun, WANG Kuai-she, HAN Ying-ying. A comparative study on Johnson cook, modified zerilli–Armstrong and Arrhenius-type constitutive models to predict high-temperature flow behavior of Ti-6Al-4V alloy in α+β phase [J]. High Temperature Materials and Processes, 2016, 35(3): 297-307. DOI:10.1515/htmp-2014-0157.

[13] YAN Liang-ming, SHEN Jian, LI Zhou-bing, LI Jun-peng, YAN Xiao-dong, MAO Bai-ping. Modeling for flow stress and processing map of 7055 aluminium alloy based on artificial neural networks [J]. The Chinese Journal of Nonferrous Metals, 2010, 20(7): 1296-1301. http://en.cnki.com.cn/Article_en/CJFDTOTAL-ZYXZ201007009.htm. (in Chinese)

[14] ZHOU X, LIU R R, ZHOU H T, JIANG W X. A revisited study of the processing map and optimized workability of AZ61 magnesium alloy [J]. Journal of Materials Engineering and Performance, 2017, 26(5): 2423-2429. DOI: 10.1007/ s11665-017-2670-2.

[15] BABU K A, MANDAL S, ATHREYA C N, SHAKTHIPRIYA B, SARMA V S. Hot deformation characteristics and processing map of a phosphorous modified super austenitic stainless steel [J]. Materials & Design, 2017, 115: 262-275. DOI: 10.1016/j.matdes.2016. 11.054.

[16] JIN Xue-ze, XU Wen-chen, SHAN De-bin, LIU Chang, ZHANG Qi. Deformation behavior, microstructure evolution and hot workability of Mg-3.7Gd-2.9Y-0.7Zn-0.7Zr alloy [J]. Metals and Materials International, 2017, 23(3): 434-443. DOI: 10.1007/s12540-017-6352-2.

[17] KUMAR N, KUMAR S, RAJPUT S K, NATH S K. Modelling of flow stress and prediction of workability by processing map for hot compression of 43CrNi steel [J]. ISIJ International, 2017, 57(3): 497-505. DOI: 10.2355/ isijinternational.ISIJINT-2016-306.

[18] BASANTH K K, SAXENA K K, DEY S R, PANCHOLI V, BHATTACHARJEE A. Processing map-microstructure evolution correlation of hot compressed near alpha titanium alloy (TiHy 600) [J]. Journal of Alloys and Compounds, 2017, 691: 906-913. DOI: 10.1016/j.jallcom.2016.08.301.

[19] RIEIRO I, CARS M, RUANO O A. A new stability criterion for the hot deformation behavior of materials: application to the AZ31 magnesium alloy [J]. Metallurgical and Materials Transactions A, 2017, 48(7): 3445-3460. DOI: 10.1007/ s11661-017-4102-1.

[20] TAO Zhang, WU Yun-xin, GONG Hai, SHI Wen-ze, JIANG Fang-min. Flow stress behavior and constitutive model of 7055 aluminum alloy during hot plastic deformation [J]. Mechanics, 2016, 22(5): 359-365. DOI: 10.5755/j01. mech.22.5.12527.

[21] PRASAD Y V R K, GEGEL H L, DORAIVELU S M, MALAS J C, MORGAN J T, LARK K A, BARKER D R. Modeling of dynamic material behavior in hot deformation: forging of Ti-6242 [J]. Metallurgical Transactions A, 1984, 15(10): 1883-1892. DOI: 10.1007/BF02664902.

[22] PRASAD Y V R K, SESHACHARYULU T. Processing maps for hot working of titanium alloys [J]. Materials Science and Engineering A, 1998, 243(1, 2): 82-88. DOI: 10.1016/S0921-5093(97)00782-X.

[23] WANG Zhe, WANG Xin-nan, ZHU Zhi-shou. Characterization of high-temperature deformation behavior and processing map of TB17 titanium alloy [J]. Journal of Alloys and Compounds, 2017, 692: 149-154. DOI: 10.1016/ j.jallcom.2016.09.012

[24] EZATPOUR H R, SAJJADI S A, SABZEVAR M H, CHAICHI A, EBRAHIMI G R. Processing map and microstructure evaluation of AA6061/Al₂O₃ nanocomposite at different temperatures [J]. Transactions of Nonferrous Metals Society of China, 2017, 27(6): 1248-1256. DOI: 10.1016/S1003-6326(17)60145-6.

[25] WANG S, HOU L G, LUO J R, ZHANG J S, ZHUANG L Z. Characterization of hot workability in AA 7050 aluminum alloy using activation energy and 3-D processing map [J]. Journal of Materials Processing Technology, 2015, 225: 110-121. DOI: 10.1016/j.jmatprotec.2015.05.018.

[26] HU H E, WANG X Y, DENG L. Comparative study of hot-processing maps for 6061 aluminium alloy constructed from power constitutive equation and hyperbolic sine constitutive equation [J]. Materials Science and Technology, 2014, 30(11): 1321-1327. DOI: 10.1179/1743284714Y. 0000000569

[27] HU H E, WANG X Y, DENG L. An approach to optimize size parameters of forging by combining hot-processing map and FEM [J]. Journal of Materials Engineering and Performance, 2014, 23(11): 3887-3895. DOI: 10.1007/ s11665-014-1182-6.

(Edited by ZHENG Yu-tong)

中文导读

7055铝合金热压缩变形修正本构模型及热加工性

摘要：为了获得热变形中7055铝合金流变行为及热加工性，开展材料在不同温度和应变速率下热压缩实验。基于实验获得7055铝合金在不同变形条件下真应力-真应变曲线，流变应力随着应变速率的升高和温度的降低而增大。Arrhenius双曲正弦本构方程中每个材料参数均设置为常数，预测材料流变行为时误差较大。本文建立修正的Arrhenius本构方程，能更准确地预测材料高温流变行为，根据热压缩实验结果，修正方程中每个材料参数均拟合为温度和应变速率的函数。同时，建立了7055铝合金热加工图，分析了温度和应变速率对能量耗散及流变失稳因子的作用规律。通过热加工图，能获得不同变形条件下材料主导变形机制；通过能量耗散图，能获得高效率的能量耗散；通过流变失稳图能获得合理的工艺参数，避免热加工中的流变失稳现象。通过热加工图，可获得较优的7055铝合金热变形参数：变形温度673~723 K, 应变速率0.01~0.4 s^-1，在该参数范围内能量耗散率超过30%。基于热变形图，采用有限元模型获得了该材料热轧变形较优的工艺参数。

关键词：7055铝合金；流变行为；修正的本构方程；热加工图；参数优化

Foundation item: Project(51175257) supported by National Natural Science Foundation of China; Project(BK20170785) supported by the Natural Science Foundation of Jiangsu Province, China; Project(BE2016179) supported by Science and Technology Planning Project of Jiangsu Province, China; Project(Kfkt2017-08) supported by Open Research Fund of State Key Laboratory for High Performance Complex Manufacturing, Central South University, China

Received date: 2018-09-05; Accepted date: 2019-06-25

Corresponding author: ZHANG Tao, PhD, Lecturer; Tel: +86-15250997258; E-mail: 297zhangtao@nuaa.edu.cn; ORCID: 0000-0001- 8065-1927