Optimal design for buckets layout based on muck removal analysis of TBM cutterhead
来源期刊:中南大学学报(英文版)2020年第6期
论文作者:夏毅敏 杨妹 林赉贶 乔硕 暨智勇
文章页码:1729 - 1741
Key words:optimal design; buckets layout; discrete element method; muck removal performance; tunnel boring machine
Abstract: The layout of the buckets for tunnel boring machine (TBM) directly affects the muck removal efficiency of cutterhead during excavation. In order to improve the muck removal performance for TBM,the optimal design of bucket layout was investigated. The whole muck transfer process was simulated by discrete-element method (DEM), including the muck falling, colliding, pilling up, shoveling and transferring into the hopper. The muck model was established based on size distribution analysis of muck samples from the water-supply tunnel project in Jilin Province, China. Then, the influence of the bucket number and the interval angle between buckets on muck removal performance was investigated. The results indicated that, as the number of buckets increased from four to eight, the removed muck increased by 29% and the residual volume decreased by 40.5%, and the process became steadier. Different interval angles between buckets were corresponding to different removed muck irregularly, but the residual muck number increased generally with the angles. The optimal layout of buckets for the cutterhead in this tunnel project was obtained based on the simulation results, and the muck removal performance of the TBM was verified by the actual data in the engineering construction.
Cite this article as: YANG Mei, XIA Yi-min, LIN Lai-kuang, QIAO Shuo, JI Zhi-yong. Optimal design for buckets layout based on muck removal analysis of TBM cutterhead [J]. Journal of Central South University, 2020, 27(6): 1729-1741. DOI: https://doi.org/10.1007/s11771-020-4403-1.

J. Cent. South Univ. (2020) 27: 1729-1741
DOI: https://doi.org/10.1007/s11771-020-4403-1
YANG Mei(杨妹)1, 2, XIA Yi-min(夏毅敏)1, 2, LIN Lai-kuang(林赉贶)1, 2,QIAO Shuo(乔硕)1, 2, JI Zhi-yong(暨智勇)1, 2
1. School of Mechanical and Electrical Engineering, Central South University, Changsha 410083, China;
2. State Key Laboratory of High Performance Complex Manufacturing, Central South University,Changsha 410083, China
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2020
Abstract: The layout of the buckets for tunnel boring machine (TBM) directly affects the muck removal efficiency of cutterhead during excavation. In order to improve the muck removal performance for TBM,the optimal design of bucket layout was investigated. The whole muck transfer process was simulated by discrete-element method (DEM), including the muck falling, colliding, pilling up, shoveling and transferring into the hopper. The muck model was established based on size distribution analysis of muck samples from the water-supply tunnel project in Jilin Province, China. Then, the influence of the bucket number and the interval angle between buckets on muck removal performance was investigated. The results indicated that, as the number of buckets increased from four to eight, the removed muck increased by 29% and the residual volume decreased by 40.5%, and the process became steadier. Different interval angles between buckets were corresponding to different removed muck irregularly, but the residual muck number increased generally with the angles. The optimal layout of buckets for the cutterhead in this tunnel project was obtained based on the simulation results, and the muck removal performance of the TBM was verified by the actual data in the engineering construction.
Key words: optimal design; buckets layout; discrete element method; muck removal performance; tunnel boring machine
Cite this article as: YANG Mei, XIA Yi-min, LIN Lai-kuang, QIAO Shuo, JI Zhi-yong. Optimal design for buckets layout based on muck removal analysis of TBM cutterhead [J]. Journal of Central South University, 2020, 27(6): 1729-1741. DOI: https://doi.org/10.1007/s11771-020-4403-1.
1 Introduction
With the rapid development of underground engineering technology, tunnel boring machine (TBM) has been widely adopted in water-electricity, railway, highway and subway tunnel projects, providing a high advance rate and excellent work safety [1-3]. The cutterhead is a core component of the TBM, located at the front of the machine, playing a role in breaking rock, supporting tunnel, and removing muck [4]. The buckets installed in the cutterhead are used to remove the muck, and optimizing the buckets layout is one of the most effective ways to improve the muck removal performance of TBM and tunneling efficiency [5].
In terms of cutterhead design, many scholars focused on the cutters and supporting ribs layout design based on geological conditions and engineering properties of different projects [6]. The cutters spacing has been geological designed by numerical simulation method [7-9] and experimental method [10, 11]. Various layout patterns of cutters have been adopted to maintain the balance torque of cutters [12, 13]. And the supporting ribs layout was designed coupled with cutters [14]. All these abovementioned studies concerned on design method for cutters and supporting ribs layout while little attention has been paid to buckets design for cutterhead. HUO [15] and XIA et al [16] have optimized the opening area of cutterhead and the bucket structure based on muck fluidity, respectively. However, the layout of buckets was not considered in the cutterhead design.
During excavation, there is a complex interaction between the rock stratum and TBM including rock breaking and muck discharging [17, 18]. Numerous studies have been conducted in rock breaking process, where the rock breaking mechanism and load characteristic were explored through mathematic, numerical and experimental method [19-22]. With respect to muck removal process, HUO et al [23] and GENG et al [24] established the simplified muck removal simulation model, where the cutters influencing the layout of buckets and the flow characteristic of muck seriously were omitted.
Therefore, the comprehensive buckets layout for TBM cutterhead design based on muck removal simulation with the real cutterhead model is presented. In this work, a numerical study on the muck removal process of TMB is proposed using discrete element method (DEM). In order to study the effects of the layout parameters for buckets on the muck removal performance, the whole model of the TBM mucking removal system consisting of cutters, buckets, supporting ribs and collection hopper is established, and particularly, the muck particle model for the water-supply tunnel project in Jilin Province, China, is modeled. In addition, the muck generated during tunneling is considered to study the influence of the bucket layout on the muck removal performance. Finally, the optimal buckets layout of the cutterhead is designed based on the simulation results and the in-site data is analyzed to validate the feasibility of the cutterhead design.
2 Numerical simulation of muck removal process
2.1 Modelling of muck removal system
The muck removal system consists of a cutterhead, buckets, scrapers, a collection hopper and a conveyor, as shown in Figure 1. The muck removal process can be divided into four stages: the muck of guiding, shoveling, sliding and transporting. Firstly, with the rotation and advancement of cutterhead, the tunnel is excavated continuously by the disc cutters sitting on the cutterhead. Simultaneously, the rock stratum is broken into muck, generated at tunnel face. Most of the muck fall down under gravity and heap up at the bottom between the cutterhead and the tunnel face. Subsequently, the muck is shoveled into the cutterhead by the scrapers, which are installed on the buckets. In the third step, with the rotation of the cutterhead, the muck slides along the supporting ribs to the collection hopper. Finally, the collected muck is transported out of the tunnel by the conveyor.

Figure 1 Muck removal system:
The EDEM software based on DEM is used to simulate the movement characteristics of discrete muck during the muck removal process [25, 26], which is convenient and powerful to make discrete element analyses and track the movement of each particle at any time.
A three-dimensional CAD model of the muck removal system is generated using SolidWorks and imported into EDEM as shown in Figure 2. The cutterhead structure is made of steel, and input parameters of steel in simulation are assigned as shown in Table 1. To ensure that the muck at the bottom is carried away, buckets are usually arranged at the border of the cutterhead, and the scrapers are installed at the buckets. The simulated cutterhead is equipped with four buckets, whose diameter is 8 m. A tunnel face and a surrounding face are built to simulate the working environment of TBM, where the tunnel face is at the front of the TBM and 135 mm away from the cutterhead face, the same as the install height of cutters. It means that the distance between tunnel face and cutterhead face is 135 mm when cutters interacts with tunnel face. The surrounding face is at the periphery of the cutterhead, with the same diameter of cutterhead. The particle factory in EDEM is built at the tunnel face, which is used to generate the muck particles. The number of particles, the generating rate and the generation location could be set in the simulation software. The discharged muck particles in the collection hopper are counted to obtain the muck removal efficiency.
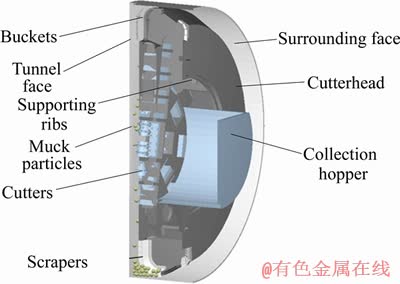
Figure 2 Simulation model of muck removal system
Table 1 Input parameters of muck removal system
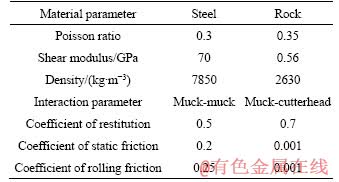
The muck represents rock fragments broken by cutters, and the muck particle model is established by the EDEM software. Based on the interaction between particles and Newton’s law of motion, the cyclic iterative calculation is operated to determine the force and displacement of particles at each time and step. In this way, the motion property of the whole particles could be obtained. Taking the water-supply tunnel project in Jilin Province, China, as the research object, the geological condition is a typical granite ground and the material parameters of the rock is shown in Table 1 [27]. Muck shape has a few influences on the efficiency of the muck removal. However, to study the optimal design of buckets layout for TBM and get the acceptable calculation speed, the muck is simplified as spherical particles [23].
In addition, the diameter of the muck particles is an essential parameter in this simulation. Consequently, in-site sampling and statistical investigation on muck was conducted. Three muck specimens were sieved through standard square holes with the pieces on each sieve [28], as shown in Figure 3. The cumulative weight mass fraction from each sieve screen was counted, as shown in Table 2.
The particle size distribution function of the muck was calculated by the Rosin–Rammler equation [29], as follows:
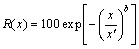 (1)
(1)
where R(x) is the cumulative percent of muck retained; x is the sieve screen size; x′ is the mean particle size parameter and b is the distribution parameter measure of particle sizes [30]. x′ and b can be estimated via linear regression of data graphically represented in Rosin–Rammler (RR) diagrams.

Figure 3 Analysis of muck specimens in-site:
Table 2 Sieve result of three sets of muck specimens in-site

By performing logarithmic operations twice on both sides of Eq. (1) simultaneously,the Rosin–Rammler function is linearized as follows:
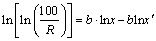 (2)
(2)
A plot of double logarithm of 100/R(x) versus logarithm of x should give a straight line. Diagram with such a combination of axis scales is called the RR diagram. The mean particle size x′ can be determined when x=x′, and this substitution in Eq. (2) will produce a constant of 36.79% muck retained R(x), which means that if the data [x, R(x)] are used to plot the lines fitting the RR distribution, distance along the x-axis is the mean particle size when R(x)=36.79% [31].
Based on sieve result of three sets of muck specimens in-site and the RR diagram calculation method, the mean muck particle size can be obtained, as shown in Figure 4. The average diameter of muck in Engineering 1 is 91.21 mm, as the red vertical line showed. The average diameter of muck in Engineering 2 is 69.91 mm, as the blue vertical line showed. The average diameter of muck in Engineering 3 is 75.69 mm, as the green vertical line showed. Considering the particle model used in the simulation software is spherical, the average equivalent muck particle diameter of three specimens in simulation is confirmed to be 80 mm.
2.2 Simulation of muck removal process
According to the real situation, the boundary conditions are set by EDEM as follows:
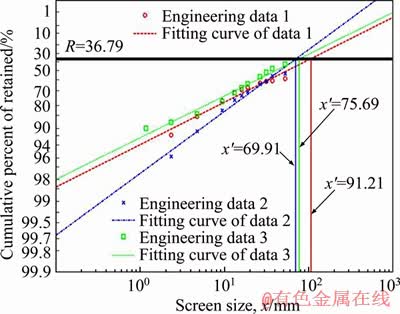
Figure 4 Rosin-Rammler distribution based on log-log coordinates
1) It is assumed that the surrounding stratum remains stable during TBM tunneling, and the tunnel face as well as the surrounding face around the cutterhead are fixed. Besides, the collection hopper does not rotate with the cutterhead.
2) The muck is generated at the contact location between cutters and tunnel face. In the simulation model, the muck particles are generated from the particle factory, at the same location with the tunnel face, and the generating area is within a circle with the same diameter of cutterhead.
3) After one revolution of the cutterhead, the total volume of all the rock fragments could be calculated as follows:
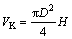 (3)
(3)
where VK is the total volume of all the rock fragments; H is the penetration of the cutterhead (6 mm); D is the cutterhead diameter (7930 mm).
The number of muck particles (N) when the cutterhead rotates a revolution can be calculated as follows:
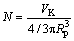 (4)
(4)
where Rp is the radius of the simulated rock particle (40 mm), and the number of muck particles produced per revolution can be determined to be 1100, as the rotate speed of cutterhead is 6 r/min; the particles generating speed is 110 s-1. As the generating time is set as 40 s, and the total number of muck particles generated in this simulation is 4400.
4) Gravity acceleration in the whole model is necessary.
5) The generated muck was given an initial velocity horizontally of 0.006 m/s to simulate the advance process. The rotation speed of the cutterhead was set as 6 rev/min, which is the average speed during excavation.
Figure 5 shows the particles movement during the muck removal process, where two muck particles are taken as samples, and other particles are hided. Muck 1 is produced at the upper-left side of the cutterhead. Theoretically, it would fall down vertically under gravity, while the muck 1 fall down in the below-left direction under the action of the cutting force from the cutters. As the muck collides with the cutters, the motion direction changes. As shown in Figure 5, muck 1 collides with the cutters twice, and it is shoveled into the cutterhead immediately when the buckets rotate to the bottom of the tunnel. Afterwards, the muck slides into the collection hopper along the supporting ribs. Muck 2,generated at the upper-right of the cutterhead, collides with the cutters four times during falling. When it collides with central cutters, the trajectory is horizontal in short segments, which means the muck is stuck with cutters, then muck 2 falls into and swings at the bottom as the cutterhead rotates. Ultimately, it is shoveled by the scraper into the cutterhead and slides into the collection hopper.
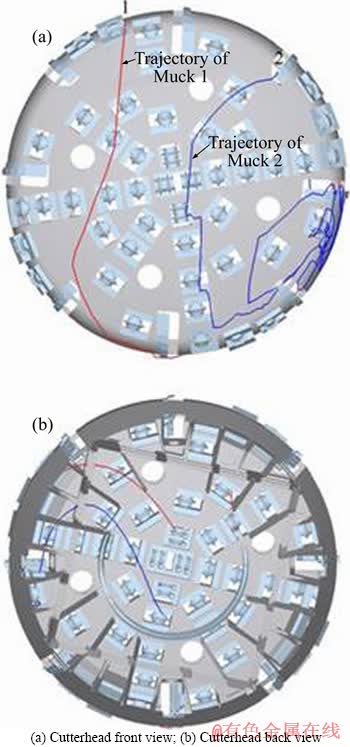
Figure 5 Movement trajectory of rock particles:
The distribution of the muck particles after the cutterhead rotates four revolutions (40 s) is shown in Figure 6. Most of the muck particles are shoveled into the collection hopper, but a small number of muck particles are left over at the bottom, and the rest remain at the internal part of the cutterhead, colliding with the supporting ribs during the muck removal process.
Figure 7 illustrates the relationship between the number of particles in the buckets and time. When t=0, the muck particles begin to generated from the tunnel face. Meanwhile, the cutterhead begin to rotate. After the cutterhead rotates a revolution, the bucket completes a muck shoveling action. When the bucket rotates at the bottom of the tunnel, the muck is shoveled into the cutterhead. While the bucket rotates at the top of the tunnel, the muck slides into the collection hopper. Therefore, the number of particles in the bucket changes with the rotating of the cutterhead. The peak of the curve refers to the muck removal capacities of the bucket.
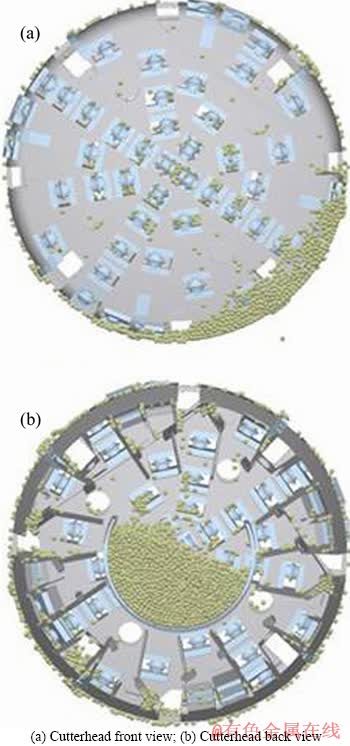
Figure 6 Distribution of muck particles after rotating four revolutions:
Figure 7 Variation of number of particles in buckets during muck removal process
The muck in the collection hopper is counted as the discharged particles. The residual muck at the bottom of tunnel is counted as the residual particles. The varying curves of discharged particles and residual particles are shown in Figure 8. During the first revolution of cutterhead (0-10 s), the particles begin to discharge. During the second to the fourth revolution (10-40 s), the discharged particles increase approximately linearly. The residual particles increase firstly during 0-10 s and then remain stable during 10-40 s with the number of 500, which indicates that the muck-discharging process reaches a stable state.

Figure 8 Variations of discharged and residual particles during muck removal process
According to the simulation results, the total discharged muck particles during the second to fourth revolution indicate the discharging efficiency. As the residual muck particles accumulate at the bottom of the tunnel, the second wear to the cutters and scrapers would be increased, and the more muck remains at the bottom of the tunnel, the more severe abrasion emerges on the cutters and scrapers. Therefore, the average residual muck particles during the second to the fourth revolution reflect the abrasion of the cutters and scrapers partly. The statistical variance of the residual muck numbers during the second to fourth revolution is the fluctuation amplitude of the residual muck numbers, which refers to the stable characteristics of the muck removal process. Therefore, the variance of the residual muck numbers is chosen for the evaluation indicator of the muck removal stability. To ensure the accuracy of the simulation result, the total discharged muck amount Nd, the average residual muck 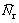 , and the variance of the residual muck numbers S2 in 2-4 revolutions are counted and calculated.
, and the variance of the residual muck numbers S2 in 2-4 revolutions are counted and calculated.
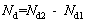 (5)
(5)
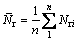 (6)
(6)
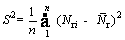 (7)
(7)
where Nd1 is the discharged particles at 10 s; Nd2 is the discharged particles at 40 s; n is the number of time point; Nri is the residual particles at time i.
3 Optimal design for buckets layout
The opening structure of the cutterhead provides space for cutters, buckets, and manhole. The buckets are the core part for TBM cutterhead, which significantly affects the excavation efficiency of the TBM. Furthermore, the number of buckets and the interval angle between buckets are the two important parameters of the layout. Therefore, the influence of these two parameters on muck removal performance is investigated and the optimal design of buckets layout is obtained based on the simulation results.
3.1 Influence of number of buckets
The number of arranged buckets largely depend on the diameter of cutterhead and increase with the diameter of cutterhead. The specific number of buckets should be designed, compressively considering the limited space of cutterhead, the capacity of the belt conveyor, and the strength and stiffness of the structure. For the cutterhead with the diameter of 8 m in this water-supply tunnel project, the number of buckets is no more than eight. And the buckets should be arranged symmetrically to reduce the unbalance torque of the cutterhead. According to the cutterhead structure, there are only 8 positions to fix up the buckets as shown in Figure 9, where a long bucket and short bucket are distributed alternately, and the buckets lengths are 975 and 645 mm, respectively. Therefore, six cutterhead schemes simulation with different number of buckets and their detailed data were obtained in Table 3.
Figure 10 shows the muck removal performance of cutterhead with different buckets number by EDEM and the statistical results are depicted in Figure 11. The muck removal amount of the cutterhead with different numbers of buckets are shown in Figure 11(a). It can be inferred that the discharged particles increase when the number of arranged buckets rises from four to eight. The cutterhead with two long buckets and two short buckets discharges the fewest particles, which is 1920. Scheme 6 with eight buckets yields the optimum performance with the maximum, which is 2470. The muck removal efficiency ranges from 58.2% to 74.8%. The residual muck amount and statistical variance of the cutterheads with different numbers of buckets are clearly shown in Figure 11(b). The residual muck amount gradually decreases with the increase of the number of arranged buckets. Scheme 1 leaves the most particles at the bottom of the tunnel, which is 818 and may increase the risk of the cutters and scrapers wearing. 501 muck particles remain at the bottom in Scheme 6, which is the minimum. The variance of the residual particles varies with the different numbers of buckets. The variance of Scheme 6 is the minimum, which is 498 and means that Scheme 6 has the most-stable muck removal process. The variance of Scheme 2 is the maximum, which is 5846.
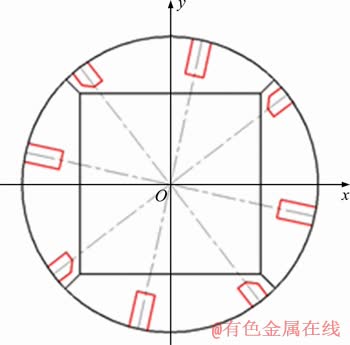
Figure 9 Buckets position of cutterhead
Table 3 Cutterhead scheme with different bucket number


Figure 10 Muck removal performance of cutterhead with different buckets number:
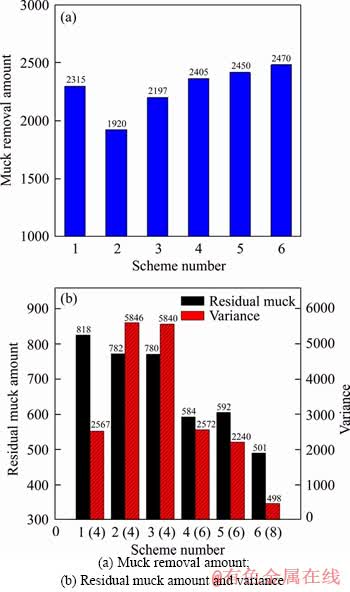
Figure 11 Muck removal performance of cutterhead with different numbers of buckets:
3.2 Influence of interval angle between buckets
The interval angle between buckets is the key factor affecting the muck removal process. Because of the complex structure of the cutterhead, the long buckets are arranged at the middle of the edge blocks, whose vertical deviation a1 varies from 11.5° to 13.5°, while the short buckets are arranged at the border, whose vertical deviation a2 varies from 35.5° to 39.5°. The interval angle between adjacent buckets a3 varies from 47° to 53°, which is the sum of the deviation angle between the long bucket and the short bucket. The sketch map of deviation angles and interval angles is shown in Figure 12. 9 cutterhead schemes with different interval angles between buckets are established, whose angles are presented in Table 4.
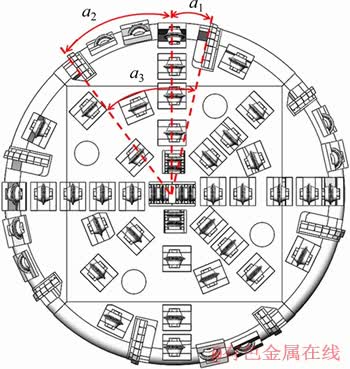
Figure 12 Deviation angles and interval angles between buckets
Table 4 Cutterhead scheme with different interval angles

Figure 13 illustrates the muck removal performance of the cutterhead with different interval angles between buckets. As can be seen from Figure 13(a), Scheme 4 and Scheme 3 have the minimum and maximum muck removal amounts, at 2374 and 2483, respectively. When the interval angle between buckets varies from 47° to 53°, the muck removal efficiency ranged from 71.9% to 75.2%. When the deviation angle of long buckets a1 is 11.5°, comparisons of Schemes 1, 3, and 7 reveal that the discharged muck increases firstly and then decreases with the increase of the deviation angle of the short buckets a2. When a2 is set as 35.5°, the muck removal amount is 2403. Similarly, when the deviation angle of short buckets a2 is 35.5°, comparisons of Schemes 1, 2, and 4 reveal that the discharged muck increases firstly and then decreases with the deviation angle of the long buckets a1. When a1 is set as 12.5°, the muck removal amount is the maximum, which is 2420. The residual muck amount and its variance of cutterhead with different interval angles between buckets are shown in Figure 13(b). As the interval angle a3 increases, the residual muck gradually increases. The residual muck of Schemes 7, 8 and 9 are much more than that of the other six schemes, and their residual particles all exceeded 530. The change law of variance is less obvious with the increase of the interval angle a3. The variance of Scheme 2 is the minimum, which is 405 and means the muck removal process of this scheme is the most stable. The variance of Scheme 8 is the maximum, which is 847, indicating the most- unstable muck removal process. As for cutterhead with the same a3, Scheme 7 has smaller a1 and larger a2 compared with scheme 6. The simulation results show that the muck removal amount of Scheme 7 is more than that of Scheme 6, and the residual muck amount is more than that of Scheme 6, and the variance is larger than that of Scheme 6. It indicates that with the same interval angle a3, the muck removal efficiency is higher with the increase of interval angle a2.
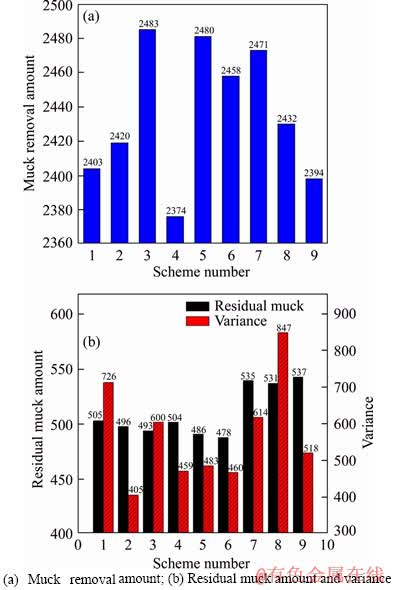
Figure 13 Muck removal performance of cutterhead with interval angles between buckets:
With the comprehensive consideration of muck removal amounts, muck removal stability and the residual muck, the bucket layout scheme with optimal muck removal performance is got according to the analysis of simulation results. There are 8 buckets on the cutterhead, including 4 longs and 4 shorts, and the interval angles between them are a1=12.5° and a2=37.5° in the optimized scheme, which was successfully applied to the water-supply tunnel project in Jilin Province, China.
4 Verification results of engineering project
Based on the simulation results, the optimal cutterhead is designed to be combined with the construction requirements and engineering experience. Ultimately, the diameter of the cutterhead is 7930 mm, where fifty-one cutters are installed at the cutterhead face, including eight center cutters, thirty-two routine cutters, and eleven gage cutters. The spacing of the center cutters is 101.5 mm, and the spacing of the routine cutters is 83 mm. Twelve supporting ribs are arranged symmetrically between the web plate and the back panel with the thickness of 50 mm. The optimal scheme is applied to the tunnel project, in which the sizes of the long bucket and the short bucket are 975 mm×500 mm×720 mm and 645 mm×500 mm× 720 mm, respectively. The final scheme of the cutterhead structure is presented as Figure 14.

Figure 14 Cutterhead structure applied in water-supply tunnel
The engineering data were analyzed to validate the effectiveness and feasibility of the design method of buckets layout. The muck removal performance in-site was evaluated by analyzing the excavation efficiency and the consumption of cutters.
As shown in Figure 15, the excavation distance was evaluated. According to the data, the TBM reflected a good performance during the period from May 21th to June 19th. The maximum excavation distance was 40 m per day on June 18th, while the minimum was 5 m on June 7th, and the total excavation distance was 544 m. If there are lots of muck particles remained at the bottom of tunnel, the TBM would not advance efficiently. The actual excavation distance indicates satisfactory muck removal performance of TBM.
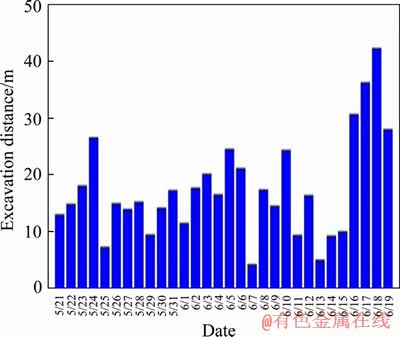
Figure 15 Tunneling distance of TBM in a month
Furthermore, if the muck was not discharged in time and accumulates in front of the cutterhead, it would lead to secondary wear of the cutter, which is an important factor for cutter failure. In the tunnel project, the replacement quantity of cutters was used to represent their consumption. The cutter in different position of the cutterhead was numbered, where the No.1 was the closest to the center of cutterhead, while the No. 51 cutter was the most peripheral to the cutterhead. The number of replaced cutters during a month was shown in Figure 16. The No. 1 to No. 14 cutters had never been replaced, which meant they worked well, while the No. 47 cutter had been renewed five times in a month, which was the maximum. The total numbers of cutter replacements were 69, which was less than the number in other tunnel projects. It can be inferred that the wear of the cutters was quite acceptable, indicating that the muck was successfully discharged. On the whole, the buckets layout of the TBM can meet the design requirements of geological adaptability.
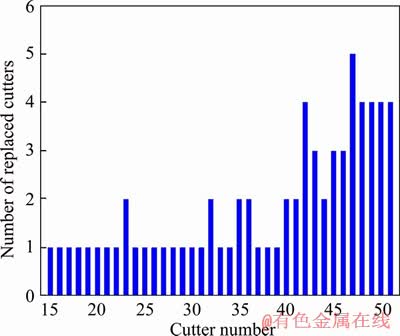
Figure 16 Number of replaced cutters in tunnel project
5 Conclusions
A numerical simulation of the muck removal process for TBM cutterhead is proposed in this paper. Based on the actual tunnel project, muck removal system and in-site muck particles model are established, and the influence of the buckets layout for TBM cutterhead on muck transfer is studied through DEM method. The simulation results are applied to optimal design for the buckets layout and verified in the engineering project. The following conclusions can be made.
The muck removal performance of the TBM is critically influenced by the buckets layout. As the number of buckets increases from four to eight, the rock removal amount increases by 29%, the residual volume decreases by 40.5%, and the muck removal process becomes steadier. With the increase of the interval angle between buckets, the rock removal amount varies irregularly, while the residual volume increases generally and its variance irregularly change. Moreover, when the interval angles between the short bucks and the long bucks a1, a2 are 12.5° and 37.5°, the comprehensive muck removal performance is the best. The optimal design of cutterhead based on the simulation results is got, specifically, the number of buckets is eight, and the interval angle a3 between buckets is 50°, which has been successfully applied to the water-supply tunnel project in Jilin Province, China. Data analysis on cutters consumption and the muck removal efficiency shows a great muck removal performance for the TBM cutterhead.
Discrete-element simulation is a powerful tool to study the complicated tunneling process and the results could be a guide to the design of TBM cutterhead to improve muck removal performance in different geological conditions.
References
[1] ROSTAMI J. Hard rock TBM cutterhead modeling for design and performance prediction [J]. Geomechanik Und Tunnelbau, 2010, 1(1): 18-28. DOI: 10.1002/geot. 200800002.
[2] HUANG Tian, WANG Xiao-hong, LIU Hai-tao, YANG Yu-hu. Force analysis of an open TBM gripping–thrusting– regripping mechanism [J]. Mechanism and Machine Theory, 2016, 98: 101-113. DOI: 10.1016/j.mechmachtheory.2015. 12.003.
[3] YAGIZ S, KARAHAN H. Prediction of hard rock TBM penetration rate using particle swarm optimization [J]. International Journal of Rock Mechanics and Mining Sciences, 2011, 48(3): 427-433. DOI: 10.1016/j.ijrmms. 2011.02.013.
[4] XIA Yi-min, TANG Lu, JI Zhi-yong, CHENG Yong-liang, BIAN Zhang-kuo. Optimal design of structural parameters for shield cutterhead based on fuzzy mathematics and multi-objective genetic algorithm [J]. Journal of Central South University, 2015, 22(3): 937-945. DOI: 10.1007/ s11771-015-2604-9.
[5] WU Li, GUAN Tian-min, LEI Lei. Discrete element model for performance analysis of cutterhead excavation system of EPB machine [J]. Tunnelling and Underground Space Technology, 2013, 37(6): 37-44. DOI: 10.1016/j.tust. 2013.03.003.
[6] CHENG Yong-liang, ZHONG Jue, JI Zhi-yong, MEI Yong-bing. Geological adaptive design method and application of TBM cutterhead [J]. Journal of Mechanical Engineering, 2018, 51(1): 1-9. DOI: 10.3901/JME.2018. 01.001. (in Chinese)
[7] ZHOU Peng, GUO Jing-jing, SUN Jian, ZOU De-fang. Theoretical research and simulation analysis on the cutter spacing of double disc cutters breaking rock [J]. KSCE Journal of Civil Engineering, 2019, 23(7): 3218-3227. DOI: 10.1007/s12205-019-1777-4.
[8] XIA Yi-min, LIN Lai-kuang, WU Dun, JIA Lian-hui, CHEN Zhuo, BIAN Zhang-kuo. Geological adaptability matching design of disc cutter using multicriteria decision making approaches [J]. Journal of Central South University, 2018, 25(4): 843-854. DOI: 10.1007/s11771-018-3788-6.
[9] HAN Dong-ya, CAO Ping, LIU Jie, ZHU Jian-bo. An experimental study of dependence of optimum TBM cutter spacing on pre-set penetration depth in sandstone fragmentation [J]. Rock Mechanics and Rock Engineering, 2017, 50(4): 3209-3221. DOI: 10.1007/s00603-017-1275-2.
[10] PAN Yu-cong, LIU Quan-sheng, LIU Jian-ping, PENG Xing-xin, KONG Xiao-xuan. Full-scale linear cutting tests in chongqing sandstone to study the influence of confining stress on rock cutting forces by TBM disc cutter [J]. Rock Mechanics and Rock Engineering, 2018, 51(6): 1697-1713. DOI: 10.1007/s00603-018-1412-6.
[11] CHO Jung-woo, JEON Seokwon, JEONG Ho-young, CHANG Soo-ho. Evaluation of cutting efficiency during TBM disc cutter excavation within a Korean granitic rock using linear-cutting-machine testing and photogrammetric measurement [J]. Tunnelling and Underground Space Technology, 2013, 35(4): 37-54. DOI: 10.1016/j.tust.2012. 08.006.
[12] SUN Wei, HUO Jun-zhou, CHEN Jing, ZHANG Xu, GUO Li, ZHAO Hai-feng, ZHAO Yue. Disc cutters’ layout design of the full-face rock tunnel boring machine (TBM) using a cooperative coevolutionary algorithm [J]. Journal of Mechanical Science & Technology, 2011, 25(2): 415-427. DOI: 10.1007/s12206-010-1225-3.
[13] HUO Jun-zhou, SUN Wei, CHEN Jing, ZHANG Xu. Disc cutters plane layout design of the full-face rock tunnel boring machine (TBM) based on different layout patterns [J]. Computers & Industrial Engineering, 2011, 61(4): 1209-1225. DOI: 10.1016/j.cie.2011.07.011.
[14] HUO Jun-zhou, SUN Wei, OUYANG Xiang-yu, YU Shi-qiang. Coupling layout design of disc cutters group and cutterhead supporting structure [J]. Journal of Mechanical Engineering, 2014, 50(21): 23-30. DOI: 10.3901/JME.2014. 21.023. (in Chinese)
[15] HUO Jun-zhou, ZHOU Lin-wei, ZHU Dong, ZHANG Wei, WANG Wei-zheng, SUN Wei. Cutterhead opening mode design of a composite earth pressure balance shield machine [J]. Journal of Harbin Engineering University, 2017, 38(3): 433-439. DOI: 10.11990/jheu.201603020. (in Chinese)
[16] XIA Yi-min, YANG Mei, WU Dun, LIN Lai-kuang, JI Zhi-yong. Influence of the TBM mucking slot structure parameters on ballast discharge characteristics [J]. Journal of Harbin Engineering University, 2018, 39(9): 1561-1567. DOI: 10.11990/jheu.201611091. (in Chinese)
[17] ZHANG Qian, HOU Zhen-de, HUANG Gan-yun, CAI Zong-xi, KANG Yi-lan. Mechanical characterization of the load distribution on the cutterhead–ground interface of shield tunneling machines [J]. Tunnelling and Underground Space Technology, 2015, 47: 106-113. DOI: 10.1016/j.tust.2014. 12.009.
[18] ZHANG Xu-hui, XIA Yi-min, ZENG Gui-ying, TAN Qing, GUO Ben. Numerical and experimental investigation of rock breaking method under free surface by TBM disc cutter [J]. Journal of Central South University, 2018, 25(9): 2107-2118. DOI: 10.1007/s11771-018-3900-y.
[19] XIA Yi-min, GUO Ben, CONG Guo-qiang, ZHANG Xu-hui, ZENG Gui-ying. Numerical simulation of rock fragmentation induced by a single TBM disc cutter close to a side free surface [J]. International Journal of Rock Mechanics and Mining Sciences, 2017, 91: 40-48. DOI: 10.1016/ j.ijrmms.2016.11.004.
[20] LAZEMI H A, SOLEIMAN DEHKORDI M. Estimation of the TBM penetration rate using the post-failure behavior of a rock mass and the equivalent thrust per cutter. A case study: The amirkabir water transferring tunnel of iran [J]. Bulletin of Engineering Geology and the Environment, 2019, 78: 1735-1746. DOI: 10.1007/s10064-017-1205-2.
[21] CHOI Sung-oong, LEE Seung-joong. Three-dimensional numerical analysis of the rock-cutting behavior of a disc cutter using particle flow code [J]. KSCE Journal of Civil Engineering, 2015, 19(4): 1129-1138. DOI: 10.1007/ s12205-013-0622-4.
[22] CHENG Yong-liang, ZHONG Jue, MEI Yong-bing, XIA Yi-min. Rock fragmentation under different installation polar angles of TBM disc cutters [J]. Journal of Central South University, 2017, 24(10): 102-109. DOI: 10.1007/s11771- 017-3642-2.
[23] HUO Jun-zhou, CHEN Wei, OUYANG Xiang-yu, ZHANG Xu. Simulation of TBM mucking process based on the ballast fluidity [J]. Applied Mechanics and Materials, 2014, 541-542: 832-835. DOI: 10.4028/www.scientific.net/AMM. 541-542.832.
[24] GENG Qi, ZHANG Hui-jian, LIU Xiao-hui, WANG Xue-bin. Numerical study on the rock muck transfer process of TBM cutterhead with clump strategy based on discrete element method [J]. Tunnelling and Underground Space Technology, 2019, 91: 103000.
[25] REGASSA Bayisa, XU Neng-xiong, MEI Gang. An equivalent discontinuous modeling method of jointed rock masses for DEM simulation of mining-induced rock movements [J]. International Journal of Rock Mechanics and Mining Sciences, 2018, 108: 1-14. DOI: 10.1016/ j.ijrmms.2018.04.053.
[26] NAGHADEHI M, RAMEZANZADEH A. Models for estimation of TBM performance in granitic and mica gneiss hard rocks in a hydropower tunnel [J]. Bulletin of Engineering Geology and the Environment, 2016, 76: 1627-1641. DOI: 10.1007/s10064-016-0950-y.
[27] MAHABADI O, COTTRELL B, GRASSELLI G. An example of realistic modelling of rock dynamics problems: FEM/DEM simulation of dynamic brazilian test on barre granite [J]. Rock Mechanics and Rock Engineering, 2010, 43(6): 707-716. DOI: 10.1007/s00603-010-0092-7.
[28] LI J, WEBB C, PANDIELLA S, CAMPBELL G. Discrete particle motion on sieves—A numerical study using the DEM simulation [J]. Powder Technology, 2003, 133(1-3): 190-202. DOI: 10.1016/S0032-5910(03)00092-5.
[29] SONG Ke-zhi, JI Li-guang, YUAN Da-jun, WANG Meng-shu. Research on distribution regularities of grain size of rock detritus from discoid cutters [J]. Chinese Journal of Rock Mechanics and Engineering, 2008, 27: 3016-3022. (in Chinese)
[30] ZHEN Gang-biao, KANG Tian-he, CHAI Zhao-yun, YING Zhi-hong. Applied the rosin-rammler distribution function to study on the law of coal dust particle-size distribution [J]. Journal of Taiyuan University of Technology, 2006, 37(3): 317-319. DOI: 10.3969/ j.issn.1007-9432.2006.03.018. (in Chinese)
[31] BREZANI I, ZELENAK F. Improving the effectivity of work with Rosin-Rammler diagram by using MATLAB R GUI tool [J]. Acta Montanistica Slovaca, 2010, 15(2): 152-157.
(Edited by ZHENG Yu-tong)
中文导读
基于全断面岩石掘进机刀盘出碴分析的出碴槽布置优化设计
摘要:在TBM掘进过程中,出碴槽布置直接影响刀盘出碴性能。为了提高TBM的出碴性能,对出碴槽最优布置进行了研究。采用离散元方法对TBM出碴过程进行数值模拟,包括岩碴掉落、与滚刀碰撞、被铲刀铲起以及运送到皮带机排出的全过程。基于吉林输水隧道工程的岩碴样本进行粒径分布分析,建立岩碴颗粒模型,得到出碴槽数量和相邻出碴槽间隔角度对出碴性能的影响规律。结果表明,当出碴槽数量从4个增加至8个时,出碴量增加了29%,残余岩碴量减少40.5%且出碴过程更稳定。出碴量随出碴槽间隔角度的变化不大,但残余出碴量随着间隔角度的增大而逐渐增多。根据仿真研究,得到了该隧道工程刀盘出碴槽的最优布置方案,并通过隧道实际施工数据验证了优化后TBM的出碴性能。
关键词:优化设计;出碴槽布置;离散元方法;出碴性能;全断面岩石掘进机(TBM)
Foundation item: Project(51475478) supported by the National Natural Science Foundation of China; Project(2012AA041801) supported by the National High Technology Research and Development Program of China; Project(2014FJ1002) supported by the Science and Technology Major Project of Hunan Province, China; Project(2013CB035401) supported by the National Basic Research Program of China
Received date: 2019-12-10; Accepted date: 2020-03-23
Corresponding author: XIA Yi-min, PhD, Professor; Tel: +86-731-88876926; E-mail: xiaymj@csu.edu.cn; ORCID: 0000-0001-6174- 0377

