J. Cent. South Univ. Technol. (2008) 15(s1): 206-209

DOI: 10.1007/s11771-008-347-6

Creep testing and viscous behavior research on carbon constructional quality steel under high temperature

YU Min(余 敏)¹, LUO Ying-she(罗迎社)¹, PENG Xiang-hua(彭相华)²

(1. Institute of Rheological Mechanics and Material Engineering, Central South University of Forestry and Technology, Changsha 410004, China;

2. Swan College of Central South University of Forestry and Technology, Changsha 410004, China)

Abstract: Creep tests under at a certain temperature and different stress levels were performed on two carbon constructional quality steels at a certain stress level and different temperatures, and their creep curves at high temperature were obtained based on analyzing the testing data. Taking 45 steel at a certain temperature and stress as the example, the integral creep constitutive equation and the differential stress-strain constitutive relationship were established based on the relevant rheological model, and the integral core function was also obtained. Simultaneously, the viscous coefficients denoting the viscous behavior in visco–plastic constitutive equation were determined by taking use of the creep testing data. Then the viscous coefficients of three carbon steels (20 steel, 35 steel and 45 steel) were compared and analyzed. The results show that the viscosity is different due to different materials at the same temperature and stress.

Key words: carbon constructional quality steel; creep under high temperature; constitutive equation; integral core function; viscosity

1 Introduction

With the rapid development of industry, the reliability the demand of components enduring high temperature and complex load has become higher. For many metals, the creep deformation under room temperature is usually small, so it can be ignored; however, it must be considered at high temperature, because it changes with time due to the interaction of fatigue and damage^[1-2]. About the investigation on creep problem, CORMEAU and ZIENKIEWICZ et al have done detailed research in 1970s; in 1985, CLIFTON R J set creep as the first item in the schedule of research items need to be launched and proposed to seek an uniform disposal method for creep through the combination of creep stress and basic mechanism^[3]. The development of modern industry and abroad use of equipments working on high temperature have endowed creep of metals with more significance. In despite of the constant emerging of new materials, the carbon constructional quality steel remains one of the most important materials in the field of technology application, and its properties under high temperature are still the keystone in research field.

The rheological properties of 20 steel under thermal tension were investigated, and its rheological stress model at high temperature was established on the basis of Perzyna model and Johnson-Cook model^[4-5]; and the creep properties at high temperature were analyzed for 35 steel^[6]. In this work, creep tests at high temperature were done for 20 steel and 45 steel; taking 45 steel as the example, the integral creep constitutive equation and the differential stress-strain constitutive relationship were established under a certain stress and temperature based on the relevant rheological model, and the integral   core function was also obtained. Simultaneously, the viscous coefficients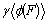, denoting the viscous property in visco–plastic constitutive equation, were determined through creep testing data, and the viscous coefficients of three kinds of carbon constructional quality steels were compared and analyzed.

2 Creep testing under high temperature

The creep tests were performed on the high temperature creep and endurance strength testing machine RC-1230 produced by Wuzhong Material Testing Limited Company in Ningxia Province. The testing materials of 20 steel and 45 steel were annealed and then processed into the samples of d10 mm×100 mm, meeting the national standard GB/T2039—1997. Then the high temperature creep tests at a certain temperature and different stress levels were preformed at a certain stress level and different temperatures, and the schedules are shown in Tables 1 and 2. There are 10 sets of testing data, and the creep curves are shown in Figs.1-4.

Table 1 Summary of temperature and stress conditions employed for creep tests of 20 steel

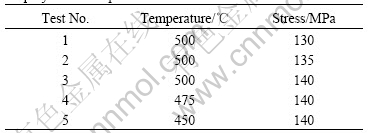
Table 2 Summary of temperature and stress conditions employed for creep tests (45 steel)

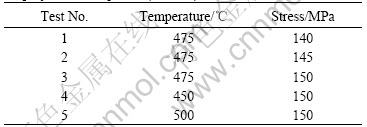
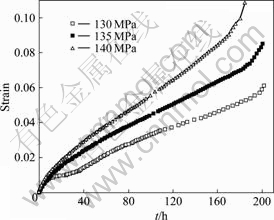
Fig.1 Creep curves of 20 steel under different stress levels at 500 ℃

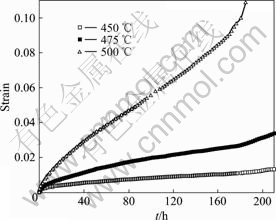
Fig.2 Creep curves of 20 steel at different temperatures and σ = 140 MPa

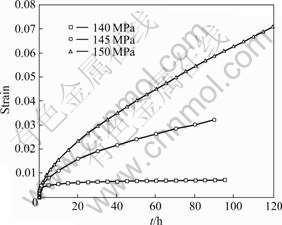
Fig.3 Creep curves of 45 steel at different stress levels and  500 ℃

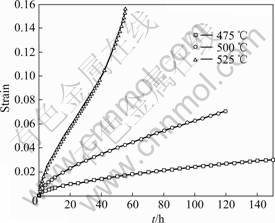
Fig.4 Creep curves of 45 steel at different temperature and σ = 150 MPa

It can be seen from Figs.1-4 that the first stage of creep is short; the creep rate in the first and second stage and the duration of the second stage changes when the stress changes at constant temperature or the temperature changes at constant stress. The second stage of creep, having constant creep rate, is shortened with the increase of stress at constant temperature or the increase of temperature at constant stress, that is, the second stage of creep becomes longer when applying small load at constant temperature or lowering the temperature at constant load. Therefore, it can be attempted to produce high strength complex parts by rheological forming at constant temperature, low load and slow rate. In addition, the creep deformation of 45 steel is samller than that of 20 steel at the same temperature of 475 ℃ and stress of 140 MPa (see Fig.5).

The creep properties of TC11 titanium alloy, Lc4

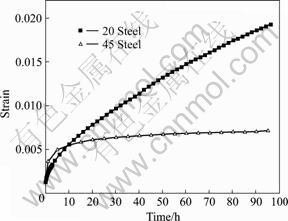
Fig.5 Comparison of deformation for 20 steel and 45 steel at  475 ℃ and σ = 140 MPa

aluminum alloy and 35 steel were researched, and some important conclusions were obtained^[6-8]. The results are shown in Figs.1-4. It can be seen from Figs.1-4 that the second stage of creep curves is similar to the modified Burgers model, so the modified Burgers Model was adopted to describe the creep behavior of carbon constructional quality steel. For creep deformation, the creep rate 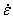of the third stage is speed up with time, and the inner structure of materials may damage abruptly and finally rupture. However, being rupture means deforming failure, so the deformation of the third stage was not considered here. The modified Burgers model is shown in Fig.6 and its constitutive equation is denoted as follows^[6-8]:

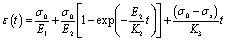, σ₀≥σ_s   (1)

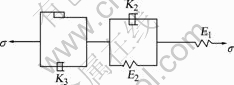
Fig.6 Modified Burgers’ model

The parameters in the model can be obtained according to the creep curves so that its creep constitutive model can be acquired^[6-7]. Taking 45 steel under the condition of θ = 475 ℃, σ =150 MPa as the example, the parameters are obtained according to the testing data:

E₁=100 GPa, E₂=8.04 GPa,

K₂=286.704 GPa?h, K₃=31.85 GPa?h,

σ_s = 136.4 MPa                               (2)

So the creep equation of 45 steel under the above conditions is

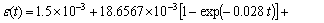
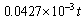                          (3)

The differential stress-strain constitutive relationship based on the modified Burgers model can be obtained according to the model theory of rheological mechanics^[6-8]:

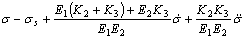
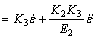                              (4)

Substituting Eqn.(2) into Eqn.(4), the differential stress-strain constitutive relationship for 45 steel (θ = 475 ℃, σ =150 MPa) can be determined:

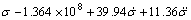
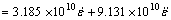               (5)

Taking the stress level as σ₀ and the initial time as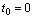, the integral creep equation is:

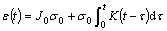                  (6)

where  is the core function of Eqn.(6), creep integral core for short. It is the eigenfunction that can reflect the rheological properties of materials and its expression is:

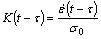                            (7)

Substituting the creep into Eqn.(5) under the stress of 140 MPa to the above equation, the creep integral core function of 45 steel under the condition of θ = 475 ℃, σ =150 MPa can be obtained:

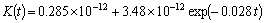   (8)

Metals may yield at low stress when suffering high temperature and will accumulate a great deformation with time, so it is necessary to consider the viscous of metals at high temperature. It is difficult to acquire the viscous coefficients of different materials under different conditions. The material constants  were used to denote the viscous properties of materials in the visco-plastic constitutive equation for TC11 titanium alloy, Lc4 aluminum alloy and 35 steel at a certain temperature and different stress levels though the creep tests^[6-7]. Here the same method was adopted to determine the values of  at a certain temperature and three different stresses based on the creep tests of 20 steel and 45 steel.  is a coefficient related to the viscosity of materials, and its relationship with stress is shown in Figs.7 and 8. σ-γ relationship of 35 steel at 500 ℃ is shown in Ref.[6].

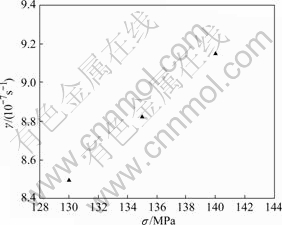
Fig.7 σ-γ relationship of 20 steel at 500 ℃

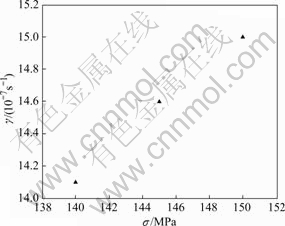
Fig.8 σ-γ relationship of 45 steel at 500 ℃steeper(62)

·steep front(9)

·steepest(70)

Comparing the viscous coefficients of the three kinds of carbon constructional quality steels, it can be found that a common characteristic that the value of  increases with stress. When stress increases, the creep curves become precipitous, that is, the equivalent strain rate 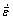 increases, so the value of  also increases. Meanwhile, the viscous coefficients of the three steels at the same temperature and stress are different. The viscous coefficient of 35 steel is larger than that of 20 steel. Under θ = 500 ℃, σ =140 MPa, the value of viscous coefficient of 35 steel is 15.43×10^-7 s^-1, and that of 20 steel is 9.15×10^-7 s^-1, which demonstrate that the viscosity of 20 steel is weaker than that of 35 steel.

4 Conclusions

1) The creep tests at high temperature are performed on 20 steel and 45 steel, and the creep curves are obtained for the two steels at a certain temperature and different stress levels and a certain stress level and different temperatures. Through analyzing the testing curves, it can be found that the high strength complex parts may be produced by rheological forming method at constant temperature, low load and slow rate.

2) The integral creep constitutive equation and the differential stress-strain constitutive relationship are established based on the relevant rheological model for 45 steel, and the integral core function is also obtained.

3) The viscous coefficients denoting the viscous properties in visco-plastic constitutive equation are determined by using the creep test data, and those of the three carbon steels (20 steel, 35 steel and 45steel) are compared and analyzed, which shows that their viscosities are different due to different materials. It is obvious that proper forming temperature and force should be selected according to different materials when machining.

References

[1] LUBARADA V A, BENSON D J, MEYERS M A. Strain-rate effects in rheological models of inelastic response[J]. Inter J Plasticity, 2003, 19(8): 1097-1118.

[2] HUESPE A E, CARFONA A, NIGRO N, FACHINOTTI V. Visco-plastic constitutive models of steel at high temperature[J]. Journal of Materials Processing Technology, 2000, 102(1/3): 143-152.

[3] CLIFTON R. Plasticity of metal[J]. Appl Mech Rev, 1985, 38(10): 1261-1263.

[4] LUO Ying-she, YANG Liu, XU Jiang-min, JI Zhong. Study on the rheological properties of steel 20 under thermal tension (PartⅠ)[J]. Natural Science Journal of Xiangtan University, 2004, 26(2): 37-39. (in Chinese)

[5] LUO Ying-she, YANG Liu, YU Min, XU Jiang-min, JI Zhong. Study on the rheological properties of 20 Steel under thermal tension (PartⅡ)[J]. Natural Science Journal of Xiangtan University, 2005, 27(1): 25-29. (in Chinese)

[6] YANGLiu, LUOYing-she, TANG Xiao-di. Rheological properties of carbon constructional quality steels[J]. Journal of Central South University of Technology, 2007, 14(s1): s285-288.

[7] LUO Ying-she, HU Yun-gui, ZHOU Jian-ping, LI Su-yang. Creep experimental research on aluminum alloy Lc4[C]// BINGDING D M, HUDSON N E. XIII^th International Congress on Rheology. Glasgow, UK: Universities Design & Print Unit, 2000: 368-370.

[8] LUO Ying-she, DONDA K, WANG Z. Experimental solution for viscosity coefficient of solid alloy material[J]. International Journal of Applied Mechanics and Engineering, 2003, 8: 271-276.

(Edited by YANG Hua)

Foundation item: Project(07031B) supported by the Scientific Research Fund of Central South University of Forestry and Technology.

Received date: 2008-06-25; Accepted date: 2008-08-05

Corresponding author: LUO Ying-she, PhD, Professor; Tel: +86-731-5623376; E-mail: lys0258@sina.com