
Equilibrium of hydroxyl complex ions in Pb2+-H2O system
WANG Yun-yan(王云燕)1, CHAI Li-yuan(柴立元)1, CHANG Hao(常 皓)1, 2,
PENG Xiao-yu(彭小玉)1, SHU Yu-de(舒余德)1
1. School of Metallurgical Science and Engineering, Central South University, Changsha 410083, China;
2. Tianjin Pipe (Group) Corporation, Tianjin 300301, China
Received 25 February 2008; accepted 24 June 2008
Abstract: The thermodynamics equilibrium principle was used to construct the diagrams for the concentration of complex ions (pc) vs pH, the distribution ratio of lead hydroxyl complex ions (αn) vs pH, and the conditional solubility product of Pb(OH)2 vs pH in the Pb2+-H2O system. The relationship between the equilibrium concentration of each kind of lead hydroxyl complex ions in equilibrium with Pb(OH)2(s) and pH value was shown in the system. The minimum solubility of lead is at the pH value of 10.096-10.997. The distribution ratio of each kind of the lead hydroxyl complex ions is determined as a function of the pH value and the total lead concentration ([Pb]T). The diagram for the conditional solubility product, pKSP vs pH, shows that each kind of lead hydroxyl complex ions existing in the system is dependent upon an optimized pH value at the established concentration of [Pb]T, and that pKSP reaches the minimum at the pH value of 10.3-11.2. The results can provide a theoretical basis for removing lead ions from wastewater by the neutralization and hydrolyzation technology.
Key words: Pb2+-H2O system; lead-containing wastewater; diagram of pc—pH; thermodynamics equilibrium principle
1 Introduction
Heavy metals are known to be detrimental to the living beings. The toxic heavy metal ions in industrial wastewaters have been considerably concerned in recent years[1]. These important contaminants are discharged from many industry fields such as electroplating, dyes and dye intermediates, textiles, tanneries, oil refineries, mining and smelters. It has been observed that increased concentration of heavy metals in the aquatics environments can cause phyto toxicity, bio-concentration and bio-magnification by organisms. Lead is hazardous and highly toxic to humans, plants and animals. Primary health effects due to Pb(Ⅱ) poisoning are nervous and renal breakdown, weakness, headache, brain damage, convulsions, behavioral disorders and constipation. Lead is a substitute for calcium in bony tissues and accumulates there. The presence of lead in drinking water is known to cause various types of serious health problems, leading to death in extreme cases[2-4].
Removal of heavy metal-bearing industrial effluents has become one of the primary challenges of wastewater treatment. Filtration, adsorption, reverse osmosis, solvent extraction, and membrane separation techniques are used for removal and recovery of heavy metals from industrial wastewater. The neutralization and metal hydroxide precipitation are the conventional techniques[5-6]. In order to offer theoretical foundation for neutralization, thermodynamic analysis of lead ion hydrolyzation has been carried out, but either all kinds of lead hydroxyl complex ions are ignored[7-8] or only single kind of complex ion is considered[9-12]. As a result, the incomplete conclusion would lead to the incorrect guidance for the technology[13-14]. It is necessary to construct the complete equilibrium diagrams of Pb2+-H2O system based on the equilibrium of various kinds of complex ions.
In this study, thermodynamic equilibria of hydroxyl complex ions in Pb2+-H2O system were analyzed and diagrams for the concentration of complex ions (pc) vs pH, the distribution ratio of the lead hydroxyl complex ions (αn) vs pH, and the conditional solubility product of Pb(OH)2 (pKSP) vs pH in Pb2+-H2O system, were respectively drawn based on the simultaneous equilibria of all complex ions[15-19]. The aim is at exploring the various forms of the lead species existing in aqueous solution and the rule of change in solubility of Pb(OH)2 with the pH value, and determining the minimum solubility of Pb(OH)2 and the optimum range of pH value. These diagrams have more advantages over pE—pH diagram in the neutralization and metal hydroxide precipitation process[20-21].
2 Effect of pH value on coordination equilibrium of lead hydroxyl complex ions
Assuming that there are only six kinds of lead hydroxyl complex ions, that is, PbOH+, Pb(OH)2, Pb(OH)3-, Pb2OH3+, Pb4(OH)44+ and Pb6(OH)84+ in lead- containing wastewater, correspondingly the cumulative formation constants for them at 298.15 K are as follows [22-23]:
Pb2++OH-=PbOH+
 (1)
(1)
Pb2++2OH-=Pb(OH)2
 (2)
(2)
Pb2++3OH-=Pb(OH)3-
 (3)
(3)
2Pb2++OH-=Pb2(OH)3+
 (4)
(4)
4Pb2++4OH-=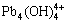
 (5)
(5)
6Pb2++8OH-=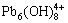
 (6)
(6)
It is known that
Pb(OH)2(s)=Pb2++2OH-
KS0=[Pb2+][OH-]2=1.2×10-15 (7)
Multiplying Eqs.(1)-(6) by Eq.(7), respectively, the following equations can be obtained:
Pb(OH)2(s)=PbOH++OH-
KS1=[PbOH+][OH-]=1.902×10-9 (8)
Pb(OH)2(s)=Pb(OH)2
KS2=[Pb(OH)2]=2.394×10-5 (9)
Pb(OH)2(s)+OH-=Pb(OH)3-
 (10)
(10)
2Pb(OH)2(s)=Pb2(OH)3++3OH-
 (11)
(11)
4Pb(OH)2(s)=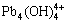 +4OH-
+4OH-
 (12)
(12)
6Pb(OH)2(s)=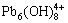 +4OH-
+4OH-
 (13)
(13)
Taking the logarithm of both sides of Eqs.(7)-(13) gives
lg KS0=lg[Pb2+]+2lg[OH-]=lg[Pb2+]+2lg Kw+2pH
p[Pb2+]=-13.073+2pH (14)
lg KS1=lg[PbOH+]+lg[OH-]=lg[PbOH+]+lg Kw+pH
p[PbOH+]=-5.276+pH (15)
lg KS2=lg[Pb(OH)2]
p[Pb(OH)2]=4.621 (16)
lg KS3=lg[Pb(OH)3-]-lg[OH-]=lg[Pb(OH)3-]-lg Kw-pH
p[Pb(OH)3-]=15.618-pH (17)
lg KS4=lg[Pb2(OH)3+]+3lg[OH-]=
lg[Pb2(OH)3+]+3lg Kw+3pH
p[Pb2(OH)3+]=-19.749+3pH (18)
lg KS5=lg[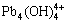 ]+4lg[OH-]=
]+4lg[OH-]=
lg[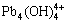 ]+4lg Kw+4pH
]+4lg Kw+4pH
p[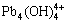 ]=-32.405+4pH (19)
]=-32.405+4pH (19)
lg KS6=lg[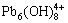 ]+4lg[OH-]=
]+4lg[OH-]=
lg[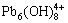 ]+4lg Kw+4pH
]+4lg Kw+4pH
p[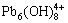 ]=-35.763+4pH (20)
]=-35.763+4pH (20)
The concentration of OH- is calculated at a given pH based on the water ionization equation and the concentration of Pb2+ is determined by Eq.(14). The latter is used in Eq.(15) to calculate [PbOH+], and in turn continue until the concentration of each complex is known. These values as functions of the pH value are plotted in Fig.1, which constructs the pc—pH diagram of Pb2+-H2O system.
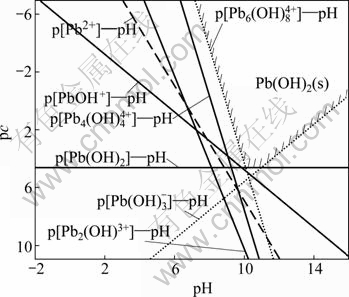
Fig.1 Diagram of pc—pH of Pb2+-H2O system at 298.15 K
Fig.1, it can be seen that the minimum solubility of lead is 4.959 mg/L at the pH value of 10.096-10.997, beyond which the solubility of lead will increase.
The lines in Fig.1 represent the relationship between the equilibrium concentration of the complex ions and the pH value. The right-upper area surrounded by these lines (e.g. the cross-hatching area) is the stable zone of Pb(OH)2(s). Boundaries constituting this stable zone can illustrate approximately the relationship between the total solubility of Pb2+ and the pH value. Fig.1 illustrates that Pb2+ is the predominant soluble species at the low pH, and Pb6(OH)84+ is the predominant species at the high pH. Obviously, if complex formation were ignored and lead solubility were calculated solely by use of Eq.(7), an erroneous conclusion could be drawn. From
3 Effect of pH value on species of lead hydroxyl complex ions
Complex formation also affects the solubility of salts. Lead is commonly removed from acidic wastewaters by adding lime-milk to form the insoluble Pb(OH)2(s). However, if excess base is added, lead will form soluble complexes with OH- and will return to or remain in solution. In aqueous solution, lead hydroxyl complex ions of PbOH+, Pb(OH)2, Pb(OH)3-, Pb2(OH)3+,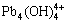 and
and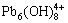 may exist. From Eqs.(1)-(6), the following equations can be deduced:
may exist. From Eqs.(1)-(6), the following equations can be deduced:
 (21)
(21)
 (22)
(22)
 (23)
(23)
 (24)
(24)
 (25)
(25)
 (26)
(26)
In addition, a mass balance for lead in solution is
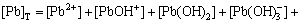
 (27)
(27)
[Pb]T represents the total soluble concentration of lead, i.e. the solubility of lead.
Definition of parameters, α0-6, is as follows:
 (28)
(28)
 (29)
(29)
 (30)
(30)
 (31)
(31)
 (32)
(32)
 (33)
(33)
 (34)
(34)
Substitution of Eqs.(21)-(26) and Eq.(28) into Eqs.(29)-(34) gives
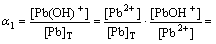
 (35)
(35)
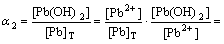
 (36)
(36)
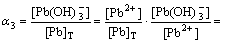
 (37)
(37)
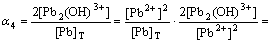
 (38)
(38)
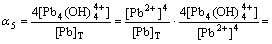
 (39)
(39)
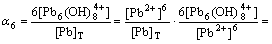
 (40)
(40)
Inserting Eq.(28) and Eqs.(35)-(40) into Eq.(27) gives
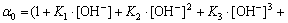
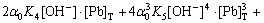
 (41)
(41)
From the above equations, it can be concluded that the concentration ratio of each ions depends greatly on the pH value and total lead concentration. Supposing that [Pb]T is 10-4 mol/L and 10-2 mol/L, respectively, substituting the different pH values into Eq.(41) gives the corresponding α0; similarly, α1, α2, α3, α4, α5 and α6 can be calculated according to Eqs.(35)-(40). Fig.2 shows the diagram of the ratio of lead hydroxyl complex ions as functions of the pH value.
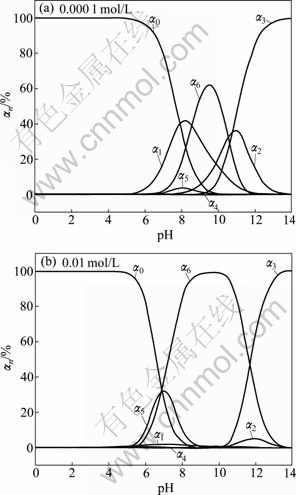
Fig.2 αn—pH diagrams illustrating ratio of lead hydroxyl complex ions as function of pH value
The various kinds of species of the different lead hydroxyl complex ions exist in the solution with the different pH values. Lead ion exists mainly in free state and a slight amount of PbOH+ ([Pb]T=0.000 1 mol/L) or 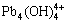 ([Pb]T =0.01 mol/L) at the pH value below 7.0. Their concentrations increase gradually with pH value declining. When the pH value is in the range of 8.0-11.0,
([Pb]T =0.01 mol/L) at the pH value below 7.0. Their concentrations increase gradually with pH value declining. When the pH value is in the range of 8.0-11.0, 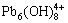 is dominant, together with a small quantity of PbOH+ ([Pb]T=0.000 1 mol/L) or Pb(OH)2 ([Pb]T=0.000 1 mol/L). The more the total concentration of lead, the more the concentration of
is dominant, together with a small quantity of PbOH+ ([Pb]T=0.000 1 mol/L) or Pb(OH)2 ([Pb]T=0.000 1 mol/L). The more the total concentration of lead, the more the concentration of  Pb(OH)3- is the primary species with a small quantity of Pb(OH)2 and
Pb(OH)3- is the primary species with a small quantity of Pb(OH)2 and 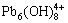 at the pH value over 12.0. There is an evident effect of total concentration of lead on the concentration of the different lead hydroxyl complex ions.
at the pH value over 12.0. There is an evident effect of total concentration of lead on the concentration of the different lead hydroxyl complex ions.
4 Effect of pH value on solubility of Pb(OH)2
Lead can form polynuclear complexes containing two or more lead atoms, which will make the solubility of Pb(OH)2 increase. Effect of the pH value on solubility of Pb(OH)2 can be expressed by the conditional solubility product[9].
The conditional solubility product of Pb(OH)2(s) is defined as
KSP=[Pb]T?[OH-]T2 (42)
where [Pb]T represents the total lead concentration, i.e. the solubility of lead (Eq.(27)). [Pb2+], [PbOH+], [Pb(OH)2], [Pb(OH)3-], [Pb2(OH)3+], [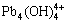 ] and [
] and [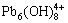 ] can be calculated from Eqs.(7)-(13). Inserting these values into Eq.(27) can determine the total concentration of soluble lead.
] can be calculated from Eqs.(7)-(13). Inserting these values into Eq.(27) can determine the total concentration of soluble lead.
The mass balance of OH- is:
[OH-]T=[OH-]+[Pb(OH)+]+2[Pb(OH)2]+3[Pb(OH)3-]+
[Pb2(OH)3+]+4[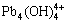 ]]+8[
]]+8[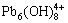 ] (43)
] (43)
where [OH-]T represents the total concentration of OH-.
The conditional solubility product of Pb(OH)2(s) at the different pH can be calculated by combination of Eq.(27), Eq.(42) and Eq.(43), and the results are shown in Fig.3. Simultaneously, the conditional solubility product of Pb(OH)2 is also drawn.
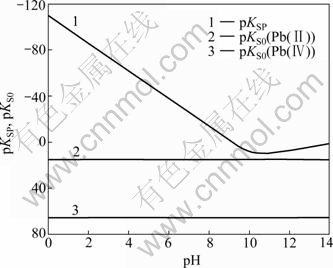
Fig.3 Relationship between conditional solubility product of Pb(OH)2 and pH value
The area above the curve is the supersaturation region of Pb(OH)2(s) dissolution, where Pb(OH)2 precipitation occurs, while other regions are unsaturated ones. Fig.3 indicates that the minimum solubility of Pb(OH)2 is in the pH range of 10.3-11.2, beyond which the solubility product of Pb(OH)2 increases obviously. In addition, pKS0 locates at the unsaturated region, which indicates that lead in wastewater cannot be removed to the minimum concentration determined by the solubility product of Pb(OH)2 using the neutralization and hydrolyzation method.
5 Conclusions
1) In Pb2+-H2O system, the diagrams for the concentration of the lead complex ions, the distribution ratio of lead hydroxyl complex ions and the conditional solubility product of Pb(OH)2 as a function of the pH value are respectively constructed based on the thermodynamics equilibrium principle.
2) The pc vs pH diagram represents the relationship between the equilibrium concentration of the complex ions in equilibrium with Pb(OH)2(s) and the pH value. The minimal solubility of lead is 4.959 mg/L at the pH value of 10.096-10.997, beyond which the solubility of lead will increase.
3) The αn—pH diagram shows the distribution ratio of the lead hydroxyl complex ions as a function of the pH value. For each kind of the lead hydroxyl complex ions, there is an optimum pH value scope.
4) The pKSP—pH diagram shows the relationship between the conditional solubility product of Pb(OH)2 and the pH value. The minimum solubility of Pb(OH)2 is in the pH range of 10.3-11.2, beyond which the conditional solubility product of Pb(OH)2 increases obviously.
References
[1] DOYURUM S, CELIK A. Pb(Ⅱ) and Cd(Ⅱ) removal from aqueous solutions by olive cake [J]. Journal of Hazardous Materials, 2006, 138(1/2): 22-28.
[2] GABALLAH I, KILBERTUS G. Recovery of heavy metal ions through decontamination of synthetic solutions and industrial effluents using modified barks [J]. Journal of Geochemical Exploration, 1998, 62(1/3): 241-286.
[3] LOW K S, LEE C K, LIEW S C. Sorption of cadmium and lead from aqueous solutions by spent grain [J]. Process Biochemistry, 2000, 36(1/2): 59-64.
[4] BULUT Y, BAYSAL Z. Removal of Pb(Ⅱ) from wastewater using wheat bran [J]. Journal of Environmental Management, 2006, 78(2): 107-113.
[5] XIE Hong-bin. Study on neutralized method to treating heavy metal wastewater [J]. Hunan Nonferrous Metals, 2000, 16(9): 91-92.
[6] DENG Li-ping, SU Ying-ying, SU Hua, WANG Xin-ting, ZHU Xiao-bin. Sorption and desorption of lead(Ⅱ) from wastewater by green algae Cladophora fascicularis [J]. Journal of Hazardous Materials, 2007,143(1/2): 220-225.
[7] ZHONG Zhu-qian, MEI Guang-gui, HE Qing-pu. Theoretical analysis of purification of wastewater by neutralization and hydrolyzation [J]. Nonferrous Smelt, 1981(4): 44-51. (in Chinese)
[8] YAO Qing-song. The effect of equilibrium theory on treating heavy metals wastewater by chemical methods [J]. Pollution and Preventing Technology, 1994(12): 10-12. (in Chinese)
[9] LI Bo. Thermodynamics analysis on the precipitation process of metal carbonates [J]. Rare Metals and Cemented Carbides, 2005, 32 (6): 4-8. (in Chinese)
[10] GAI Qing-chun, GUO Yan-he, LI Su-qi, LIN Hua-kuan, WANG Xu, ZHU Shou-rong, CHEN Rong-ti. Thermodynamic properties of complexes of 1,3,5-tri (n-2,5-diaminonhexaane)-bebzene with Co(Ⅱ), Ni(Ⅱ), Cu(Ⅱ), Zn(Ⅱ) [J]. Acta Scientiarum Naturalium Universitatis Nankaiensis, 2001, 34(2): 13-19. (in Chinese)
[11] GAO Dong-zhao, GUO Yan-he, ZHU Shou-rong, LIN Hua-kuan, XU Xin-he. Thermodynamic studies on a new type of carboxamide metal complexs [J]. Chemical Journal of Chinese University, 2004, 25(4): 740-742. (in Chinese)
[12] DRIESNER T, HA T K, SEWARD T M. Oxygen and hydrogen isotope fractionation by hydration complexes of Li+, Na+, K+, Mg2+, F-, Cl-, and Br-: A theoretical study [J]. Geochimica et Cosmochimica Acta, 2000, 64(17): 3007-3033.
[13] ARMADA M P G. A program for calculation and graphic representation of conditional constants(Ⅱ): Solubility products [J]. Computers Chem, 1996, 20(3): 385-387.
[14] HOU Miao-lin, BAUGHMAN G L. Predicting the precipitation of acid and direct dyes in natural waters [J]. Dyes and Pigments, 1992, 18(1): 35-46.
[15] ZHANG Xiang-lin, KANG Heng. Coordination chemistry [M]. Changsha: Central South University of Technology Press, 1986.
[16] STUMM W, MORGAN J J. Aquatic chemistry—An introduction to emphasizing chemical equilibria in natural waters [M]. TANG Hong-xiao. Beijing: Science Press, 1987.
[17] WANG Qing-cheng, HE Wen-meng, YIN Guang-fu. Evaluation ionic distribution for the complicated complex equilibrium by means of trial-iteration [J]. Journal of Sichuan University: Natural Science Edition, 2000, 37(6): 906-910. (in Chinese)
[18] ZHANG Ping-min. Advanced chemistry for engineering [M]. Changsha: Hunan Education Press, 2002.
[19] LI Ke-an, WEI Yong-ju, LIU Feng, SUN Bi-yun, TONG Shen-yang. Spectophotometric study on the complexation equilibria of Pb(Ⅱ) and Zn(Ⅱ) with 5-Br-PADAP [J]. Universitatis Pekinensis: Acta Scientiarum Naturalium, 1994, 30(6): 655-658. (in Chinese)
[20] TROJANOWICZ M, ALEXANDER P W, HIBBERT D B. Flow- injection potentiometric determination of free cadmium ions with a cadmium ion-selective electrode [J]. Analytica Chimica Acta, 1998, 370(2): 267-278.
[21] CHEN Ding-wang, RAY A K. Removal of toxic metal ions from wastewater by semiconductor photocatalysis [J]. Chemical Engineering Science, 2002, 56(11): 1561-1570.
[22] FANG Jing-li. Electroplating of multi-coordination [M]. Beijing: National Defence Industry Press, 1985.
[23] CHEN Shao-yan. Aquatic chemistry [M]. Beijing: Irrigation and Electrics Press, 1989.
Foundation item: Project(2007BAC25B01) supported by the National Key Project of Scientific and Technical Supporting Programs Funded by Ministry of Science and Technology of China during the 11th Five-Year Plan; Project(308019) supported by the Key Scientific and Technical Project of Ministry of Education of China; Project(08JJ3020) supported by the Natural Science Foundation of Hunan Province, China
Corresponding author: CHAI Li-yuan; Tel: +86-731-8836921; Fax: +86-731-8710171; E-mail: lychai@mail.csu.edu.cn
DOI: 10.1016/S1003-6326(08)60295-2
(Edited by YANG Bing)