J. Cent. South Univ. (2021) 28: 418-428

DOI: https://doi.org/10.1007/s11771-021-4612-2

Analysis of axial force of double circular arc helical gear hydraulic pump and design of its balancing device

WU Yi-fei(吴一飞)¹, GE Pei-qi(葛培琪)^{1, 2}, BI Wen-bo(毕文波)¹

1. School of Mechanical Engineering, Shandong University, Jinan 250061, China;

2. Key Laboratory of High Efficiency and Clean Mechanical Manufacture (Ministry of Education), Shandong University, Jinan 250061, China

Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2021

Abstract: In view of the axial force produced in the working process of double arc helical gear hydraulic pump, the theory of differential equation of curve and curved surface was utilized so that the calculation formula of axial force was obtained and the relationship between the axial force and structure parameters of gears was clarified. In order to balance the axial force, the pressure oil in the high pressure area was introduced into the end face of the plunger to press the plunger against the gear shaft, and the hydrostatic bearing whose type is plunger at the end of the shaft was designed. In order to verify the balance effect of axial force, the leakage owing to end clearance and volume efficiency of gear hydraulic pump before and after the balancing process was analyzed. This paper provides a new analysis idea and balance scheme for the axial force produced in the working process of the double arc helical gear hydraulic pump, which can reduce the leakage owing to end clearance caused by the axial force and improve the volume efficiency of the gear hydraulic pump.

Key words: double arc; helical gear; gear hydraulic pump; axial force; hydrostatic bearing

Cite this article as: WU Yi-fei, GE Pei-qi, BI Wen-bo. Analysis of axial force of double circular arc helical gear hydraulic pump and design of its balancing device [J]. Journal of Central South University, 2021, 28(2): 418-428. DOI: https://doi.org/10.1007/s11771-021-4612-2.

1 Introduction

As one of the power components of hydraulic systems, the gear hydraulic pump can provide power to the hydraulic system, which is of great significance to improve the pressure of the hydraulic system. The driving and driven gears in most of the gear hydraulic pumps are spur gears whose tooth profile is involute and this type of gear pump has the advantages of simple structure, convenient processing and low price. However, due to the structure characteristics of the gear hydraulic pump, the phenomenon of trapping oil, high flow pulsation amplitude and large noise will exist in it, which will affect the stability of the entire hydraulic system and improvement of the output pressure. Therefore, it is of great importance to design a gear hydraulic pump which can realize the effect of high output pressure, low noise and stable operation [1-5].

One kind of double arc helical gear hydraulic pump was designed by a Japanese researcher and the type of its tooth profile is shown in Figure 1. The tooth profile of arc ensures that only one pair of gears are engaged in the working process of the gear hydraulic pump, so that the problems of trapping oil in common gear hydraulic pumps can be solved. However, because of the gears in the double arc helical gear hydraulic pump are helical gears with helix angle, the driving and driven gears of the gear hydraulic pump will be affected by the axial force. The axial force will increase the clearance between the end face of the gear shaft and the shaft sleeve, which will intensify the internal leakage of the gear hydraulic pumpand and affect its volumetric efficiency [6-12]. Therefore, the axial force should be analyzed and effective balancing measures should be taken, so that the internal leakage of the gear hydraulic pump can be reduced and its volumetric efficiency can be improved, which will realize the better application of gear hydraulic pump in engineering.

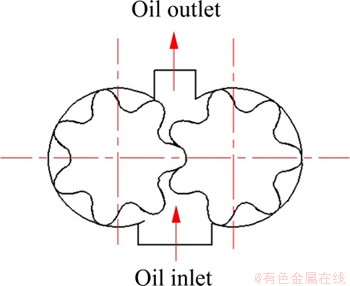
Figure 1 Two-dimensional structure diagram of double arc helical gear hydraulic pump

In this paper, the axial force in the working process of the gear hydraulic pump will be analyzed by utilizing the knowledge of differential equation of curve and curved surface and its theoretical expression formula will be obtained. In order to balance the axial force, the pressure oil in the high pressure area will be introduced into the end face of the plungers and the plungers of floating hydrostatic bearings at end of gear shafts and channels for oiling will be designed. The leakage owing to end clearance and the volumetric efficiency of the gear hydraulic pump before and after the balancing process will be analyzed and calculated, and the improvement effect of the performance of the gear hydraulic pump will be investigated.

2 Establishment of equation of tooth profile curve

The standard tooth profile curve of double arc helical gear is shown in Figure 2, which is composed of two arcs and a transition curve. The transition curve is tangent to the arc at the contact point. A straight line, an involute, a sine curve, a cosine curve and other curves can all serve as the transition curve.

If the straight line serves as the transition curve, the wear of the gear hydraulic pump in its working process will be serious. If the sine curve or the cosine curve is chosen as the transition curve, the problem of serious wear will be solved and the bearing capacity of the gear hydraulic pump will be improved. The involute can not only have the advantages of the sine curve, the cosine curve and other transition curves, but also make the transmission process more stable. So the “arc involute arc” will be chosen as the tooth profile curve of the driving and driven gears in the gear hydraulic pump.

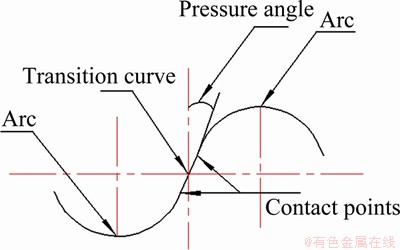
Figure 2 Standard tooth profile curve of double arc helical gears

On the basis of the tooth profile curve with the characteristics of “arc involute arc”, the plane rectangular coordinate system was established, which is shown in Figure 3. It can be seen from Figure 3 that section AB is an arc whose radius is r; section BC is an involute; and section CD is an arc whose radius is the same as that of section AB. Points B and C are the transition points of the arc and the involute.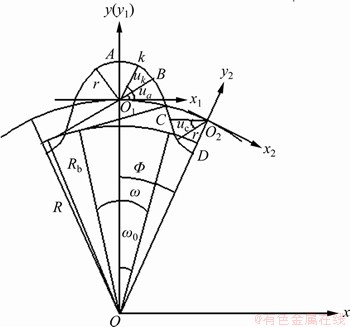

Figure 3 Plane rectangular coordinate system

Referring to the plane rectangular coordinate system established in Figure 3 and the parameter equation of the circle, the parameter expression of section AB can be written as follows:

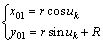                         (1)

where u_k is the angle parameter of section AB; r is the radius of AB and R is the radius of the pitch circle of the gears.

Referring to the basic equation of the involute, the parameter expression of section BC can be written as follows:

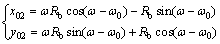         (2)

where R_b is the radius of the pitch circle of the involute; ω is the variable of section BC and ω₀ is the angle between OC and y axis and it is also the angle parameter of the involute.

Referring to the parameter equation of the circle, the parameter expression of section AB can be written as follows:

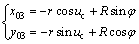                    (3)

where u_c is the angle parameter of section CD and r is its radius.

Referring to the knowledge of the plane geometry, we can obtain that φ is the value obtained by dividing π by z₀, which is the number of the teeth.

Since arc AB and arc CD are smoothly connected by involute BC, point B is the common point of arc AB and involute BC and it should be the solution of the parameter equation of arc AB and involute BC. Point C is the common point of involute BC and arc CD and it should be the solution of the parameter equation of involute BC and arc CD. Smooth connection also requires that the first derivative of the parameter equation of arc and involute is equal at both point B and point C. Therefore, the continuous condition of the arc and the involute at point B can be written as follows:

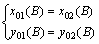                          (4)

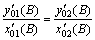                          (5)

The continuous condition of the arc and the involute at point C can be written as follows:

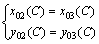                          (6)

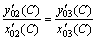                          (7)

The continuous condition can be obtained as follows by Eqs. (4)-(7):

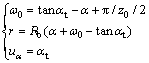                 (8)

where α_t is the transverse pressure angle and u_α is the value of parameter u_b at point B.

It can be concluded that the tooth profile curve of the gears is related to the parameters such as the transverse module, the number of teeth and the transverse pressure angle by referring to the continuous condition. Therefore, after the primary selection of these parameters, the tooth profile curve of the gears can be determined.

3 Analysis of axial force

Combined with the tooth profile curve of gears, the space rectangular coordinate system, the axomometric drawing and the transverse model of the gears can be obtained, which is shown in Figure 4. The number of teeth Z is 7; the transverse pressure angle α_t is 14.5°; the helix angle β is 32.14°. The transverse module m_t can be calculated by m_t=L_AB/π and it is 4 mm. The pitch P can be calculated by P=πd/tanβ, where d is the diameter of the pitch circle of the gear 140 mm, and it is also the production of m_t and Z.

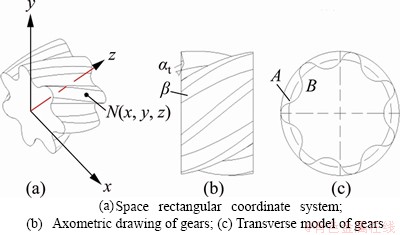
Figure 4 Space rectangular coordinate system, axomometric drawing and transverse model of gears:

The axial force produced by the gear hydraulic pump in the working process can be divided into two parts. One is produced by the pressure difference between the surfaces on both sides of the tooth, which is usually called the axial hydraulic force; the other is the produced in the meshing process of the driving and driven gears, which is usually called the axial meshing force. Because the axial meshing force can be calculated directly by referring to the calculation formula of the meshing force in the driving process of helical gears, the calculation process is relatively simple. However, the axial hydraulic force needs to be solved by integrating the hydraulic pressure on the spiral surface. Here, the derivation process of the axial hydraulic pressure is introduced first.

According to the working characteristics of the gear hydraulic pump, the pressure in the tooth surface of the gear closet to the oil outlet of the gear hydraulic pump is the highest. Generally speaking, the value is close to the outlet pressure, i.e., the rated pressure of the gear hydraulic pump, which is expressed by p_g. The tooth surface of the gear closet to the inlet is the lowest and the value can be regarded as the atmospheric pressure, which is expressed by p_d. From the oil outlet to the inlet, the pressure of the gear hydraulic pump will be reduced every time it passes through a gear tooth surface, until the pressure at the oil inlet is reduced to atmospheric pressure. The approximate pressure distribution in the whole pump can be seen in Figure 5. In order to simplify the calculation process, it can be approximately considered that the reduction value of the pressure is constant after each tooth. Therefore, the pressure distribution can be approximately centralized. It is considered that the sum of n times of the outlet pressure and the inlet pressure act on one of the tooth surfaces, where n is half of the number of the teeth, and then the integral solution of this tooth surface should be carried out.

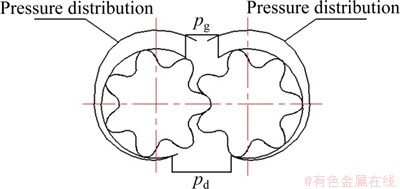
Figure 5 Approximate distribution of hydraulic pressure in gear hydraulic pump

The general idea of solving the axial hydraulic force is to solve the double integration of the effective pressure of one tooth surface in axial direction (generally z coordinate direction). For a helical surface, the normal vector of any point in x, y and z directions can be expressed by the following formula:

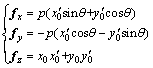                  (9)

where θ is parametric variable; p is the helix parameter; and and 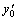are the first derivative of end coordinates.

Let M (x, y, z) be the coordinate of any point on the helical surface of the gear, and the axial hydraulic force F_zy of any point can be expressed by the product of the effective pressure and the projection of the micro element area on z axis. The specific expression is listed as follows:

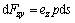                            (10)

where p is the effective pressure, which is equal to the sum of n times p_g and p_d; ds is the microelement area and e_z is the unit vector of the microelement in z direction, which can be written as follows:

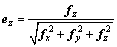                      (11)

After solving the double integration of hydraulic pressure, Eq. (10) can be transformed into:

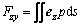                           (12)

By substituting the expression of e_z into Eq. (12), the formula of F_zy can be further transformed into:

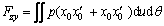                (13)

As the tooth profile curve is composed of arc and involute, the arc part and involute part shall be calculated respectively, and then the total axial hydraulic force can be obtained on a tooth surface. The final calculation result of the axial hydraulic pressure can be obtained as follows by referring to the parameter equation of arc and involute and Eqs. (9)-(13):

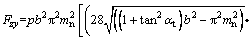
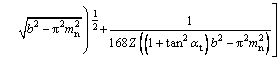 (14)

where b is the breath of the tooth; α_t is the transverse pressure angle; m_n is the normal module, which can be obtained by the product of the transverse module and the cosine of the helix angle; β is the helix angle; and p is the effective pressure.

The meshing force generated by a pair of gears during operation can be described as follows by referring to the relevant contents of mechanical design [13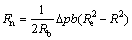-15].

                    (15)

where Δp is the pressure difference between the high pressure zone and the low pressure zone; R_b is the radius of the base circle; R_e is the radius of the addendum circle and R is the radius of the pitch circle.

The axial component of the meshing force can be described as follows by referring to transmission process of the helical gears 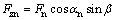[16]:

                      (16)

As for the driving gear, the direction of the axial meshing force is the same as that of the axial hydraulic force; as for the driven gear, the two directions are opposite. Therefore, the calculation formulas of the axial force F_z1 of the driving gear shaft and the axial force F_z2 of the driven gear shaft can be described as follows:

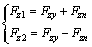                         (17)

The specific expressions of F_z1 and F_z2 can be described as follows by substituting the expressions of F_zy and F_zn:

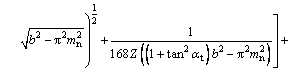
           (18)

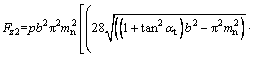
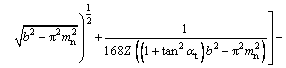
           (19)

The main structural parameters of the driving and driven gears of the gear hydraulic pump studied in this paper are as follows: m_t is 4 mm; Z is 7; α_t is 14.5°; β is 32.14°; the breath of the tooth which is represented by b is 20 mm; and the working pressure of the pump is 25 MPa. Therefore, by substituting the data above, the axial force of the driving gear shaft is 2424.2 N, and the axial force of the driven gear shaft is 1454.4 N.

4 Design of device for balancing axial force

In order to balance the axial force of the gear hydraulic pump, an axial force balance plunger is arranged in the rear pump cover of the gear hydraulic pump. Through the pressure oil holes in the rear pump cover, the pressure oil in the high-pressure area is introduced into the small end of the plunger, so that the plunger can be pressed against the gear shaft, generating a force opposite to the axial force. Therefore, the axial force can be balanced. In the working process of gear hydraulic pump, the plunger and the gear shaft are friction pairs with relatively high-speed movement. In order to prevent the solid friction caused by the direct contact between them and ensure the service life of the plunger and the gear shaft, the hydrostatic bearing structure is adopted here. An axial damping hole is machined in the center of the plunger, and an oil chamber is arranged at the bottom of the plunger. The pressure oil enters the oil chamber through the damping hole. In the oil chamber, a supporting force will be generated due to the hydraulic pressure and a layer oil film will be formed between the plunger and the end face of the gear shaft, i.e., the hydrostatic bearing, which can separate the working surface of the plunger and the gear shaft and ensure sufficient lubrication. The pressure oil flows to the periphery through the gap between the plunger and the gear shaft, and finally returns to the oil suction chamber of the gear pump. The diagram of hydrostatic bearing formed between the plunger and gear shaft is shown in Figure 6.

The maximum axial force of the driving gear shaft is 2424.2 N, and here it can be approximately regarded as 2500 N; the maximum axial force of the driven gear shaft is 1454.4 N, and here it can be approximately as 1500 N. Assuming that the diameter of the small end of the plunger at the shaft end of the driving gear is d₁, and the diameter of the small end of the plunger at the shaft end of the driven gear is d₂, the pressure acting on the end of the plunger can be approximately considered the rated pressure of the gear hydraulic pump, which is 25 MPa. Therefore, the relationship between d₁ and d₂ is as follows:

                         (20)

The result shows that d₁ should be greater than or equal to 11.2 mm and d₂ should be greater than or equal to 8.6 mm. Because the pressure loss can’t be avoided when the oil flows through the oil hole to the end face of the plunger, the value of d₁ and d₂ should be greater than the value of theoretical calculation. Therefore, d₁ is selected as 12 mm and d₂ is selected as 9 mm.

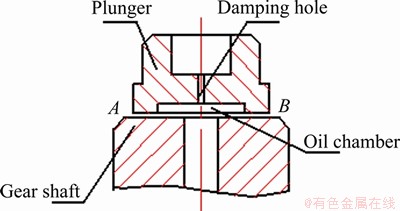
Figure 6 Hydrostatic bearing formed between plunger of gear shaft

The structure of the rear pump cover used to balance the axial force of the gear hydraulic pump is shown in Figure 7. The hole 7 for guiding oil, the inclined oil passage 8, the plunger hole 9 and the straight oil passage 10 are arranged on the rear pump cover, and the plunger is placed in the plunger hole. The pressure oil in the high pressure area can act on the small end of the plunger through the inclined oil passage and the plunger can press against the end face of the gear shaft, generating a force opposite to the axial force. Therefore, the axial force can be balanced. Because the axial force of the driving gear shaft is larger than that of the driven gear shaft, the diameter of plug hole on one side of the driving gear shaft is also greater. In order to prevent the formation of a closed volume between the shaft sleeve and part of the pump cover which is close to the oil suction chamber, that causes the pressure to rise and affects the service life of the shaft sleeve and the gear shaft (eccentric wear), an oil unloading groove 1 is arranged on the rear pump cover, through which the hydraulic oil leaked to this area is led back to the oil suction chamber. In order to prevent the leakage of hydraulic oil through the gap between the pump body and the pump cover, an annular sealing groove 2 is provided for placing the sealing ring, and a sealing groove 3 is also provided on one part of the pump cover which is close to the high-pressure region, which is needed to be equipped with a sealing ring. The area enclosed by the sealing ring is filled with high-pressure oil with the help of the high-pressure groove 4, which can generate a force to move the shaft sleeve (slide bearing) to the gear shaft side. Therefore, the end clearance can be compensated automatically. As the components of the gear hydraulic pump are connected together by bolts, four bolt holes 6 are arranged on the rear pump cover to install the bolts connecting the pump body with the front pump cover, the rear pump cover and other parts. In order to ensure assembly accuracy of the gear hydraulic pump, two holes 5 for the dowel are arranged on the same side of the rear pump cover.

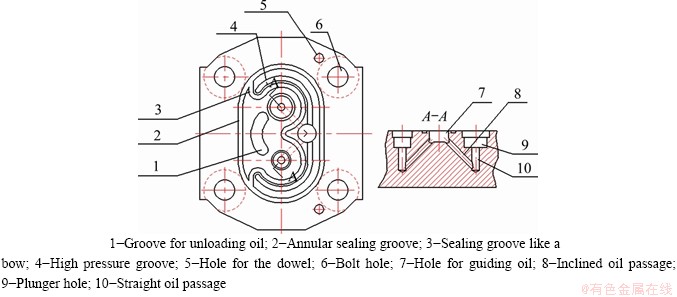
Figure 7 Structure of rear pump cover:

5 Design and calculation of hydrostatic bearing system

Because there should be a gap between the plunger and the gear shaft to form a pressure oil film, which is usually considered the initial oil film thickness and it is equal to 20 μm. The depth of the oil chamber in the plunger is generally considered to be between 30 and 60 times the thickness of the oil film [4], so it is selected to be 1 mm. In order to form the oil film between the plunger and the gear shaft so as to realize the state of liquid lubrication, the supporting force of the plunger shall balance the pressing force. The pressing force is used for balancing the axial force of the gear hydraulic pump, and the supporting force is generated by the hydraulic oil in the oil chamber. Because certain pressure loss can’t be avoided when the hydraulic oil flows through the damping hole, resulting in the pressure in the oil chamber less than that in the small end of the plunger, the plunger should be made into the same shape as the step, and the supporting force of the plunger should be analyzed.

In order to make the damping hole play the role of throttling and depressurizing, the damping hole needs to be slim. For a slim hole, its diameter needs to be greater than 0.55 mm, where d₃ is initially selected as 0.6 mm. The ratio between its length and its diameter should be equal to or greater than 4. In order to balance the supporting force and pressing force of the plunger, the pressure loss of the oil shouldn’t be too much when it flows through the slim hole, so the length of the slim hole can not be too long. Therefore, the length of the sliming hole which is represented by l₃ is initially selected as 3 mm.

Assuming that the pressure of the hydraulic oil decreases to p_s1 after it reaches the oil chamber in the plunger close to the driving gear, the flow q₁ through the damping hole of the plunger close to the driving gear can be obtained as follows by referring to the flow calculation formula of the slim hole [17].

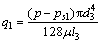                        (21)

where p is the rated pressure of the gear hydraulic pump and μ is the dynamic viscosity of the pressure oil which can be obtained by referring to some academic papers [16, 17].

When the hydraulic oil reaches the oil chamber, a part of the hydraulic oil will leak along the gap between the plunger and the end face of the driving gear shaft and the amount of this part of oil which is represented by q₂ can be calculated by the flow formula of the clearance of the rings. Assuming that the radius of the oil chamber opened in the plunger which is close to the driving gear shaft is r₅, and the radius of the driving gear shaft is r₇, which is equal to 9 mm, q₂ can be expressed by Eq. (22) [18-20]:

                 (22)

where δ is the clearance between the plunger and the gear shaft, which is equal to the thickness of the oil film; and p_A(B) is the pressure at point A or point B in Figure 6.

Generally, the initial thickness of the oil film is 20 μm, and it is approximately considered that when the hydraulic oil enters point A and point B, its pressure will drop to 0. Therefore, the expression of q₂ can be simplified as:

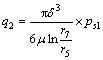                        (23)

According to the principle of energy conservation, the flow of hydraulic oil through the damping hole should be equal to the leakage generated by the gap between the plunger and the shaft, so it should be established by Eq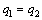. (24):

                                (24)

Supposing that the radius of the big end of the plunger close to the driving gear shaft is r₈, the distribution rule of the hydraulic oil pressure between the plunger and the driving gear shaft can be roughly expressed in Figure 8.

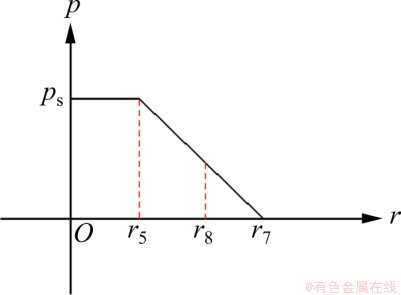
Figure 8 Distribution rule of oil pressure between plunger and driving gear shaft

Therefore, the supporting force F_N1 of the plunger can be written as follows:

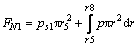                   (25)

In order to form a pressure oil film between the plunger and the shaft, the supporting force of the plunger shall be equal to the pressing force. Therefore, F_N1 should be written as follows:

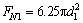                           (26)

By referring to Eqs. (21)-(26), we can obtain that the radius of the oil chamber in the plunger at the shaft end of the driving gear shaft is 4.5 mm; the radius of the large end of the plunger is 7 mm; and the pressure in this oil chamber is 22.87 MPa. Similarly, the radius of the oil chamber in the plunger close to the driven gear shaft is 3 mm; the radius of the large end of the plunger is 5.5 mm; the diameter of the damping hole is 0.6 mm; the length is 3 mm; and the pressure in the oil chamber is 23.26 MPa.

The existence of hydrostatic bearing system will lead to the increase of internal leakage of the gear hydraulic pump. The increased leakage Q_V should be equal to the sum of the flow of hydraulic oil through the damping holes of the main driving and driven gear shaft end plungers and it is equal to 0.0014 L/s by referring to Eq. (21). For the double arc helical gear hydraulic pump, its rated speed is generally 1400 r/min, and its single revolution displacement is 10 mL/r, so its flow rate is 0.233 L/s. It can be concluded that the leakage of hydraulic oil due to the hydrostatic bearing system only accounts for about 0.6% of the flow of the gear hydraulic pump, which will not have a great impact on the working performance of gear hydraulic pump. At the same time, it shows that the scheme for balancing axial force is reasonable.

6 Analysis of end clearance leakage of gear hydraulic pump and its volumetric efficiency

As for the double arc helical gear hydraulic pump, if measures are not taken for balancing its axial force, the gear shaft will be offset by a distance along the direction of the axial force, so that the end clearance on both sides of the gear is not equal. On the side with small clearance, the wear between the gear shaft and the shaft sleeve will increase, while on the side with large clearance, the leakage due to this clearance will increase, which greatly reduces the volume efficiency of the gear hydraulic pump and affects its working performance. After measures for balancing axial force are taken, the offset of the gear shaft will be greatly reduced, therefore the leakage caused by the end clearance and the wear between the gear shaft and the shaft sleeve will also be greatly reduced, so the volume efficiency and working performance of the pump will be improved to a certain extent. In this paper, the end clearance leakage and volume efficiency of gear hydraulic pump under rated working pressure with and without axial force balancing measures will be calculated and analyzed, so as to verify the performance improvement of gear hydraulic pump.

Assuming that the initial fit clearance between the gear shaft and the front and rear shaft sleeves is s, the value of which is usually between 0.02 mm and 0.04 mm according to relevant data, respectively, it is initially selected as 0.03 mm [21]. Therefore, the fit clearance between the gear shaft and the rear shaft sleeve will be less than s, and the fit clearance between the gear shaft and the front shaft sleeve will be greater than s (because the direction of the axial force is toward the rear shaft sleeve). Due to the large axial force at the end of the driving gear shaft, whose value can reach about 2500 N under the rated working pressure, it is assumed that the driving gear shaft and the front shaft sleeve are bonded together, i.e., the clearance between them is 0. The offset of the driven gear shaft can be considered to follow a linear relationship with the axial force by referring to the driving gear shaft, which is first represented by x. Therefore, by referring to hydrodynamics, the formula of the end clearance leakage of double circular arc helical gear hydraulic pump when not taking measures for balancing axial force can be written as follows:

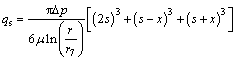      (27)

where q_s is the leakage of hydraulic oil through the end clearance; △p is the pressure difference of the gear hydraulic pump, which can be regarded as its rated pressure; μ is the dynamic viscosity of the hydraulic oil; r is the radius of the pitch circle and r₇ is the radius of the gear shaft.

It can be obtained that the leakage of hydraulic oil through the end clearance is 0.037 L/s. It is considered that the leakage through the radial clearance and the tip clearance is approximately one quarter of that through the end clearance, so the total leakage of the gear hydraulic pump is 0.0463 L/s. Under the rated working condition, the theoretical flow rate is 0.233 L/s. Therefore, the volumetric efficiency of the gear hydraulic pump is only 80.14%, which is not able to meet the working conditions of the gear hydraulic pump. If the measures for balancing axial force are not taken, the working pressure of the gear hydraulic pump will be relatively low and not reach to 25 MPa.

After measures for balancing the axial force are taken, the offsets of the driving and driven gear shafts are expressed by y₁ and y₂, respectively, and they can be approximately calculated according to the residual axial force of the driving and driven gears. Since the damping hole and oil chamber are arranged on the plungers when balancing the axial force, the pressure difference causing the leakage of the hydraulic oil through end clearance leakage will decrease. The leakage of the hydraulic oil through end clearance after taking measures for balancing the axial force can be expressed by Eq. 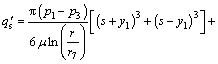(28):

        (28)

where 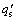is the leakage of the hydraulic oil through end clearance after measures are taken; p₁ is the pressure of the hydraulic oil in the chamber of the plunger close to the driving gear shaft; p₂ is the pressure in the chamber of the plunger close to the driven gear shaft; and p₃ is the inlet pressure of the gear hydraulic pump, which is usually regarded as 0.

It can be obtained that the leakage of the hydraulic oil through end clearance is only 0.0112 L/s at this time. It is also considered that the leakage through the radial clearance and the tip clearance is approximately one quarter of that through the end clearance, namely 0.0028 L/s. In order to balance the axial force, the hydrostatic bearing system is designed and the increased leakage due to this part is 0.0014 L/s. Therefore, it can be calculated that the total leakage of the gear hydraulic pump at this time is 0.0099 L/s and the volumetric efficiency can reach to 93.2%. In practical application, the volumetric efficiency of the gear hydraulic pump should be more than 90% under its rated working pressure [22-26], which also shows that the working pressure of the gear hydraulic pump can reach to 25 MPa after measures for balancing axial force are taken, which can avoid the situation that the output pressure of the gear hydraulic pump is extremely low due to axial force.

7 Conclusions

In this paper, the axial force produced in the working process of the double arc helical gear hydraulic pump is analyzed and calculated. The axial end plunger hydrostatic bearing is designed to realize the bearing and balance function of the axial force. And the volume efficiency of the gear hydraulic pump is analyzed and calculated. The conclusions are as follows:

1) The tooth profile equations of the driving and driven gears were derived. The axial force produced in the working process of the gear hydraulic pump was analyzed and its theoretical calculation formula was obtained by referring to the knowledge of differential equation of curve and curved surface. The relationship between axial force and parameters such as the transverse module, the number of teeth, the transverse pressure angle, the helix angle and the tooth width of the gears was clarified.

2) Measures for balancing the axial force were taken. The oil supplying hole and plunger hole were set on the rear pump cover of the gear hydraulic pump, and the plungers for balancing axial force are added. The pressure oil in the high pressure area was introduced into the end face of the plunger through the oil supplying hole, so that the plunger is pressed against the gear shaft and the axial force can be balanced. The static pressure bearing system whose type is plunger at the end of the shaft was designed.

3) The leakage of the hydraulic oil through the end clearance and the volumetric efficiency of gear hydraulic pump were analyzed. The result shows that after the measures for balancing axial force are taken, the leakage through the end clearance of the gear hydraulic pump is greatly reduced and the volume efficiency can reach over 93%. So the working pressure of the gear hydraulic pump can reach to 25 MPa or higher.

Contributors

WU Yi-fei wrote the draft of the whole manuscript. GE Pei-qi provided the concept and edited the draft. BI Wen-bo gave some advice on the design of the hydrostatic bearing system.

Conflict of interest

WU Yi-fei, GE Pei-qi and BI Wen-bo declare that they have no conflict of interest.

References

[1] GAMAUF M. Hydraulic system maintenance--tips and advice: Though safe and reliable, vigilance is essential during a repair [J]. Econometrica, 2014, 59(3): 711-730. DOI: 10.2307/2938225.

[2] HUO Xing-xing, WANG Xu-yang, GE Tong. Impulse control method for hydraulic propulsion system used in 3500 m work-class ROV [J]. Applied Ocean Research, 2016, 60: 75-83. DOI: 10.1016/j.apor.2016.08.008.

[3] MOHAMAD B, MAHYAR N, MOHAMMD Z. Adaptive super-twisting observer for fault reconstruction in electro- hydraulicsystems [J]. ISA Transactions, 2018, 76: 235-245. DOI: 10.1016/j.isatra.2018.03.014.

[4] WU De-fa, LIU Ying-shun, LI Dong-lin. Effects of materials on the noise of a water hydraulic pump used in submersible [J]. Ocean Engineering, 2017, 131(1): 107-113. DOI: 10.1016/j.ocearieng.2016.12.022.

[5] BATTARRA M, MUCCHHI E. Incipient cavitation detection in external gear pumps by means of vibro-acoustic measurements [J]. Measurement, 2018, 129: 51-61. DOI: 10.1016/j.measurement.2018.07.013.

[6] ZHOU Yang, CHE Bo-wen, YUAN Ci. The design and analysis of a high-speed circular arc gear pump journal bearing [J]. Advances in Mechanical Engineering, 2018, 10(12): 1-11. DOI: 10.1177/1687814018819288.

[7] LI Ge-qiang, ZHANG Long-fei, HAN Wei-feng. Profile design and displacement analysis of the low pulsating gear pump [J]. Advances in Mechanical Engineering, 2018, 10(3): 1-11. DOI: 10.1177/1687814018767003.

[8] BAIR B, SUNG M, WANG J, CHEN C. Tooth profile generation and analysis of oval gears with circular-arc teeth [J]. Mechanism and Machine Theory, 2009, 44(6): 1306-1317. DOI: 10.1016/j.mechmachtheory.2008.07.003.

[9] CHOI T, KIM M, LEE G, JUNG S. Design of rotor for internal gear pump using cycloid and circular-arc curves [J]. Journal of Mechanical Design, 2012, 134(1): 1-12. DOI: 10.1115/1.4004423.

[10] ZHOU Yang, HAO Ming-hui. The study of leakage of circular arc-involute-circular arc gear pump [J]. Advances in Mechanical Engineering, 2017, 9(9): 1-9. DOI: 10.1177/ 1687814017720082.

[11] LIU Yuan-wei, FAN Jia. Design of asymmetric double circular arc gear for large-scale high-pressure gear pumps [J]. Advanced Material Research, 2011, 181-182: 361-365. DOI: 10.4028/www.scientific.net/AMR.181-182.361.

[12] ZHOU Yang, HAO Shuang-hui, HAO Ming-hui. Design and performance analysis of a circular-arc gear pump operating at high pressure and high speed [J]. Proceeding of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 2016, 230(2): 189-205. DOI: 10.1177/0954406215572435.

[13] LIU Da-wei, BA Yan-bo, REN Ting-zhi. Flow fluctuation abatement of high-order elliptical gear pump by external noncircular gear drive [J]. Mechanism and Machine Theory, 2019, 134: 338-348. DOI: 10.1016/j.mechmachtheory.2019. 01.011.

[14] FNU R, ANDREA V. External gear pumps operating with non-Newtonian fluids: Modeling and experimental validation [J]. Mechanical Systems and Signal Processing, 2018, 106: 284-302. DOI:10.1016/j.ymssp.2017.12.042.

[15] INAGUMA Y. Friction torque characteristics of an internal gear pump [J]. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 2011, 9(225): 1523-1534. DOI: 10.1177/0954406211399659.

[16] MO Shuai, ZHANG Yi-du, WU Qiong, HARUO H, SHIGEKI M. Research on natural characteristics of double-helical star gearing system for GTF aero-engine [J]. Mechanism & Machine Theory, 2016, 106: 166-189. DOI:10.1016/ j.mechmachtheory.2016.09.001.

[17] GUO An-fu, JIANG Ting-ting, WANG Tong, HU Yun-ping, ZHANG Da-jiang. Numerical simulation on the flow field of external gear pump based on FLUENT [J]. Applied Mechanics & Materials, 2014, 556-562: 1421-1425. DOI: 10.4028/www.scientific.net/AMM.556-562.1421.

[18] HOI Y, MENG H, WOODWARD S H, BENDOK B R, HANEL R A, GUTERMAN L R, HOPKINS L N. Effects of arterial geometry on aneurysm growth: Three-dimensional computational fluid dynamics study [J]. Journal of Neurosurgery, 2004, 101(4): 676-681. DOI: 10.3171/ jns.2004.101.4.0676.

[19] ARIHANT S, CHOI S, KIM K, KIM J. Leakage loss estimation and parametric study on the effect of twist in rotor shape for harnessing Pico hydropower [J]. Renewable Energy, 2020, 151: 1240-1249. DOI: 10.1016/j.renene. 2019.11.124.

[20] DINA Z, MANOJ K T, ASHOKK G, DHRUBJYOTI S. A review of leakage detection strategies for pressurised pipeline in steady-state [J]. Engineering Failure Analysis, 2020, 109: 104264. DOI: 10.1016/j.engfailanal.2019.104264.

[21] HE Xiang-yu, XIAO Guang-xin, HU Bi-li, TANG Li-sha, TANG Hong-bin, HE Shang-hong, HE Zhi-yong. The applications of energy regeneration and conversion technologies based on hydraulic transmission systems: A review [J]. Energy Conversion and Management, 2020, 205: 112413. DOI: 10.1016/j.enconman.2019.112413.

[22] PAFFONI B. Pressure and film thickness in a trochoidal hydrostatic gear pump [J]. Proceedings of the Institution of Mechanical Engineers, Part G: Journal of Aerospace Engineering, 2003, 217(4): 179-187. DOI: 10.1243/ 095441003769700744.

[23] MUCCHI E, DALPIAZ G,RIVOLA A. Elastodynamic analysis of a gear pump. Part Ⅱ: Meshing phenomena and simulation results [J]. Mechanical Systems and Signal Processing, 2010, 24(7): 2180-2197. DOI: 10.1016/j.ymssp. 2010.02.004.

[24] BATTARRA M, MUCCHHI E. On the assessment of lump parameter models for gear pump performance prediction [J]. Simulation Modelling Practice and Theory, 2019, 99(1): 1-22. DOI: 10.1016/j.simpat.2019.102008.

[25] HUANG Yun, JIAHUA Suo-lang, XIAO Gui-jian, DAI Wen-tao, HE Shui, LI Wei. Study on the surface topography of the vibration-assisted belt grinding of the pump gear [J]. The International Journal of Advanced Manufacturing Technology, 2020, 106: 719-729. DOI: 10.1007/s00170- 019-04645-7.

[26] CHAI Hong-qiang, YANG Guo-lai, WU Guo-guo, BAI Gui-xiang, LI Wen-qi. Research on flow characteristics of straight line conjugate[J]. Internal Meshing Gear Pump Process, 8(3): 269-296. DOI: 10.3390/pr803026.

(Edited by ZHENG Yu-tong)

中文导读

双圆弧斜齿齿轮液压泵轴向力的分析及其平衡装置设计

摘要：针对双圆弧斜齿齿轮液压泵在工作过程中所产生的轴向力，采用曲线与曲面微分方程，对其轴向力进行求解，明确了轴向力与齿轮结构参数之间的关系。为平衡轴向力，采取了将高压区的压力油引入柱塞端面使得柱塞压向齿轮轴的方式，并设计了轴端柱塞式静压轴承。为验证轴向力的平衡效果，对平衡之前和平衡之后齿轮液压泵的端面间隙泄漏量和容积效率进行了分析。本文为双圆弧斜齿齿轮液压泵在工作过程中所产生的轴向力提供了一种新的分析思路和平衡方案，可以降低以轴向力而带来的端面间隙泄漏量，提高齿轮液压泵的容积效率。

关键词：双圆弧；斜齿轮；齿轮液压泵；轴向力；静压轴承

Received date: 2020-06-19; Accepted date: 2020-10-11

Corresponding author: GE Pei-qi, PhD, Professor; Tel: +86-13853186095; E-mail: pqge@sdu.edu.cn; ORCID: https://orcid.org/0000- 0002-4810-1738