Trans. Nonferrous Met. Soc. China 26(2016) 835-841

Effect of ore size and heap porosity on capillary process inside leaching heap

Sheng-hua YIN¹, Lei-ming WANG¹, Xun CHEN¹, Ai-xiang WU²

1. Key Laboratory for High-Efficient Mining and Safety of Metal Mines, Ministry of Education, University of Science and Technology Beijing, Beijing 100083, China;

2. School of Civil and Environment Engineering, University of Science and Technology Beijing, Beijing 100083, China

Received 27 April 2015; accepted 4 December 2015

Abstract: The capillary process coexists with gravity flow within leaching heap due to the dual-porosity structure. Capillary rise is responsible for the mineral dissolution in fine particle zones and interior coarse rock. The effect of particle size and heap porosity on the capillary process was investigated through a series of column tests. Macropore of the ore heap was identified, and its capillary rise theory analysis was put forward. Two groups of ore particles, mono-size and non-uniform, were selected for the capillary rise test. The result shows that particle size has an inverse effect on the capillary ultimate height, and smaller particles exhibit higher capillary rise. Meanwhile, the poorly graded group exhibits small rise height and velocity, while the capillary rise in the well-graded particles is much greater. The relationship between porosity and fitting parameters of capillary rise was obtained. Low porosity and high surface tension lead to higher capillary height of the fine gradation. Moisture content increases with the capillary rise level going up, the relationship between capillary height and moisture content was obtained.

Key words: heap leaching; capillary process; ore size; heap porosity

1 Introduction

Heap leaching involves stacking of metal-bearing ore into a heap on an impermeable pad, irrigating the ore for an extended period of time (weeks, months, or years) with a chemical solution to dissolve the valuable metals, and collecting the leachant as it percolates out from the base of the heap. The process has become a widely used method of mining low-grade gold, silver, copper, uranium, and nickel laterite ores [1-4]. Recovery of the target metal can range from approximately 30% for some difficult to leach sulfide copper ores to over 90% for easier oxide gold ores [5]. The solution flow characteristic is a very important aspect of the leaching process, influencing both the overall recovery and apparent kinetics of the system [6], but these flow behaviors in ore heaps are poorly understood. The present understanding of solution flow in ore heaps is derived primarily from soil mechanics, hydrogeology and chemical engineering theory coupled with experimental information.

However, the investigation of solution flow in ore heap has made significant progress in recent years. To analyze, design, control and optimize the heap leaching process, some optimal flow rates were computed by using analytical models based on the first order kinetic equation [7,8]. The optimum magnetic resonance imaging method was established to quantify the water distribution in both saturated and unsaturated ore packings [9]. Tracer tests using sodium bromide and sodium chloride were conducted to characterize the hydrodynamic behavior of heap leach systems [10]. The computational fluid dynamics simulation was used to estimate the flow and ferric iron concentration profiles around a single cell or pairs of cells of A. ferrooxidans, immobilized on the surface of a sulfide crystal [11]. A bed of non-overlapping spherical particles in cylindrical geometry were employed, and a computational model was developed to analyze the flow of fluid through the cylindrical bed of ore particles and the transport of liquid within the heap [12]. Error estimates were described for a finite element approximation to partial differential systems describing two-phase immiscible flows in porous media, with applications to heap leaching of copper ores [13]. A hydrodynamic study performed in a bench scale column, where the axial dispersion and the liquid holdup, were presented as a function of the liquid flow rate, is estimated using residence time distribution experiments [14].

The above research focuses on the solution flow driven by gravity, and the ore heap is frequently assumed to be rigidity and homogeneity. In practice, layers of coarse and fine textured ore inevitably develop within heap and dump leach piles as natural processes segregate coarse and fine material during material placement. During leaching process, pore sizes and flow paths may change over time due to chemical precipitation and dissolution of minerals, as well as weathering of rock surfaces and subsequent transport of fine material [15]. Under such conditions, gravity flows preferentially in the coarser and more conductive layer [16]. Portions of the heap, where solution does not enter laterally into the finer zones or inside the rock fractures, do not receive sufficient contact with the solution and remain unleached. Usually, leach piles are unsaturated systems, therefore solution can enter the micropores by capillary action. The capillary penetration process is of vital importance for the mineral extraction. But few attention has been focused on this process. This work attempts to reveal the capillary theory for heap leaching, and investigates factors (such as porosity and ore size) influencing the capillary process.

2 Capillary theory inside leaching heap

For the purpose of solution flow discussion, it is important to distinguish between macropore and micropore in the stacked heap [17]. The former refers to the inter-rock large-size void within the coarse zone. And the latter often refers to the small size pore inside the fine zone and intra-rock fracture. A schematic diagram of stacked ore heap is shown in Fig. 1, and there are two zones of different flow regimes.

1) Gravity flow, which refers to the solution percolation primary driven by gravity. As the solution application starts and application rate increases, macropores tend to conduct solution rapidly. Very large vertical flow velocities can be attained with only a small increase in solution application, which result in short circuiting of water in pores.

2) Capillary flow, which refers to the diffusion of solution into small-size pores of fine zones and rock fractures. There are three forces acting on the solution in an ore heap undergoing percolation leaching: gravity, surface tension and atmospheric pressure. Axial dispersion of water occurs in the lateral direction because the surface tension is greater than atmospheric pressure [18].

Besides the vertical gravity flow, lateral dispersion also occurs because of capillary action drawing water sideways. An unsaturated porous heap is often conceptualized as a bundle of capillary tubes. The solution/rock interface is of lower surface energy than the air/rock interface, causing capillary penetration of the micropores. Fine pores with higher suction dominate the capillary flow. The driving force is a vector sum of gravity and capillary forces (or capillary pressure or suction) [19].

As shown in Fig. 2, in the balance condition, the upward force caused by interfacial tension in capillaries equals the gravity:

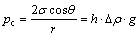                     (1)

where r is the capillary radius, △ρ is the density difference of the media, g is the gravitational acceleration, σ is the interface tension, θ is the contact angle, and the h is the height of fluid column.

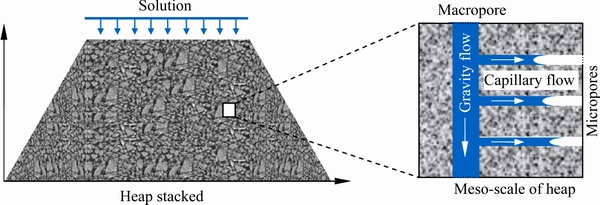
Fig. 1 Dual-porosity structure and solution flow inside heap

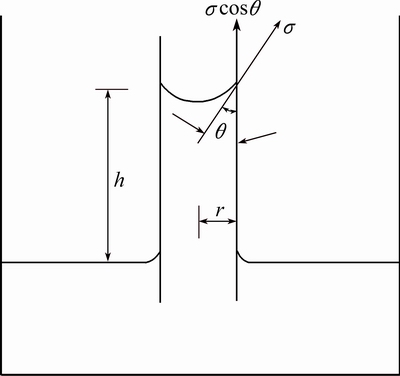
Fig. 2 Capillary rise in porous media

This expression explains for the cause and calculation of capillary pressure. In fact, most of the parameters are not easy for experimental measurement in specific condition, let alone the standardization. Actually, capillary rise is a dynamic process, during which h is variable. It has been experimentally proved that for the glass-bead-filled columns, early stage data are well fitted by the Washburn equation:

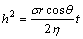                              (2)

where t is the time for solution to rise, η is the viscosity of the wetting liquid.

The ores own wetability, and their absorbability to water makes the situation more complicated. On the basis of Eq. (1), excluding permeability, connection among capillary rise height, matric suction, capillary pressure and moisture content can be derived for steady state:

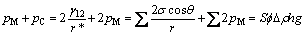    (3)

where p_M is the matric suction, p_C is the capillary pressure, γ₁₂ is interfacial tension; r is radius of curvature, 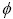 is the solution content, S is the cross section area of the packed bed.

The capillary rise flow is an important phenomenon that would occur in every unsaturated ore heap. The flow rate at which solution flows through a saturated ore/sand bed can be described by Darcy’s law and is proportional to the hydraulic head gradient [20]. However, the flow rate of solution through unsaturated soil is equal to the hydraulic conductivity, which is not constant but is related to ore size, porosity and saturation degree. The capillary rise has seldom been measured or calculated in precedent works. This manuscript tries to reveal the relationship between capillary rise and ore size, porosity and saturation degree.

3 Experimental

The capillary rise is used to investigate the capillary flow between micropores. The capillary process was measured in a series of columns loaded with ore particles with different sizes. The experimental methods and procedure are described below.

3.1 Ore samples

Low grade copper ore was obtained from Wushan Mine in Inner Mongolia, China. The chemical composition of the ore samples is mainly covellite, chalcocite, chalcopyrite, molybdenite, and gangue minerals are comprised of quartz, dickite, and sericite. The original ore was crushed, screened and grouped into seven mono-size gradations (shown in Table 1). Five non-uniform gradations were obtained by mixing several mono-size gradations (shown in Table 2). These two groups of samples have been respectively prepared to find influencing factors of capillary rise.

Table 1 Porosity and average diameter of mono-size gradations

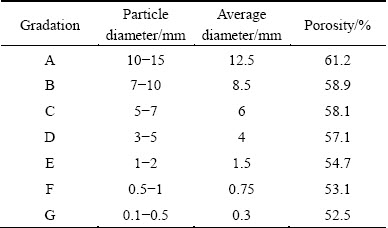
Table 2 Porosity and average diameter of non-uniform gradations

3.2 Apparatus

The capillary rise measurement apparatus is shown in Fig. 3. Ore sample was dried and loaded into a column with height of 500 mm and diameter of 100 mm, which is 6 times larger than the largest diameter ore particle to minimize wall effects. A perforated plastic sheet and support base were used at the bottom of the column to hold the ore particles. A barrel with an over flow port was used to keep the solution at a constant level.

3.3 Method

Firstly, the column was loaded with the ore sample supported by perforated sheet. The column, screen, and support base were placed in the barrel. After the column as assembled, solution was added into the barrels. An outlet was set to maintain the solution level approximately 1 cm above the perforated sheet. For columns with different ore gradations, the time to achieve the final wet interfaces was different. The capillary rise height of each ore sample was measured at an interval time of one hour until the final wet interface was obtained. Due to relatively large porosity of ores, the direct observation method was used in this study instead of some measurement instrument, and there are scales on the outlet surface of pipe.

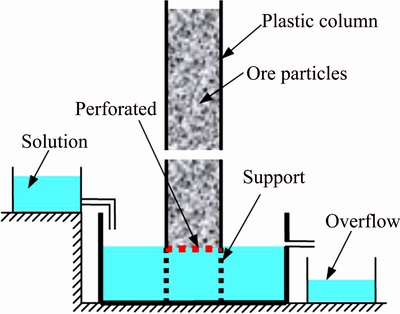
Fig. 3 Schematic diagram of capillary rise measurement apparatus

4 Results

4.1 Effect of mono-size particle diameter on capillary rise

Particle size and surface area are very important parameters that are closely related to the capillary rise. The importance of this relationship led to an evaluation of the effect of diameter on capillary rise. Seven groups of particle with different diameters were used to evaluate the capillary rise capacity. The suction of solution into column containing mono-size particles was measured by a rule for a period of 600 h. The results from these tests are shown in Fig. 4.

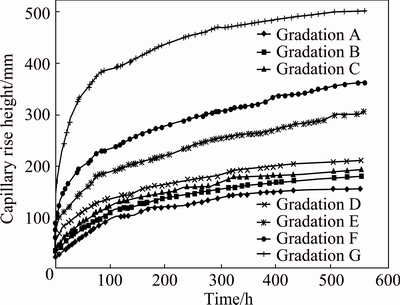
Fig. 4 Capillary rise height of mono-size particles

The data in Fig. 4 show the expected result that the capillary rise velocity and height are closely related with the particle size. At the beginning stage, the solution rises rapidly. The wetting front level goes up slowly after about 100 h. Meanwhile, the smaller particles exhibit higher capillary rise. The capillary height of Gradation G with particle diameter smaller than 0.5 mm reaches almost as high as the column height. But the capillary rise of coarse particles like Gradation A is less than 150 mm. Thus, from an ore crushing and dumping perspective, it is expected that fine particles are required to facilitate the capillary process.

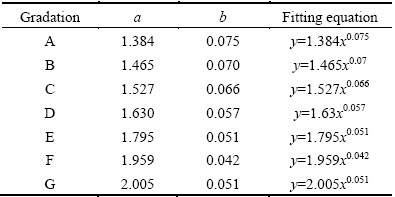
This test result is consistent with the previous theoretical analysis. The pore radius between the fine particles is small. According to Eq. (1), the capillary pressure has an inverse relationship with pore radius. Therefore, the capillary rise within the fine particle is higher than that within the coarse particles. Taking logarithm of the height, the relationship between the capillary height and time can be expressed by

y=ax^b                                     (4)

where y is lgh, h is the capillary rise height, x is the time. The capillary rise process could be expressed by the fitting equations as shown in Table 3.

Table 3 Fitting equations for mono-size gradations

4.2 Effect of non-uniform particle size on capillary rise

Non-uniform ores are usually used in the practice of heap leaching, which means that the heap contains different particle sizes. Particle gradation is an important aspect for the heap’s hydraulic conductivity and capillary process. A well graded heap contains particles of a wide range of sizes. A poorly graded heap does not have a good representation of all sizes of particles, such as the mono-size particles. Five particle gradations were obtained by mixing the mono-size particles (shown in Table 2). The capillary rise test lasted for 900 h, and the monitoring result is shown in Fig. 5.

Similar to the mono-size particles, the capillary solution between the non-uniform particle raises swiftly at the first 100 h. Within the following time, the capillary height keeps on increasing slowly or remains constant. Because the particles of Gradation J show small variability in size (poorly graded), the capillary rise velocity and eventual height are much smaller than those of other gradations. For the other four well-graded groups, such as Gradation H, fine particles (0.1-0.5 mm) coexist with coarse particles (7-10 mm), the big-size pores are filled with fine particles, reducing the capillary radius. Therefore, the capillary rise in the well graded particles is much greater than that in the poor graded ones. A wide range particle size benefits the capillary flow and solution holdup in macropores.

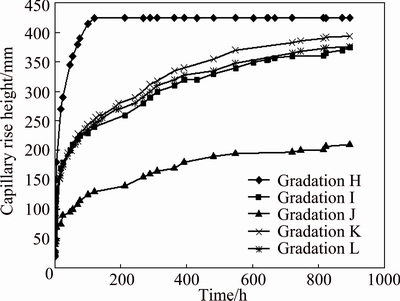
Fig. 5 Capillary rise height of non-uniform particles

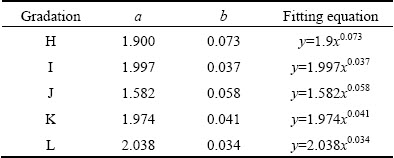
According to Eq. (4), the capillary rise process of the non-uniform particles could be expressed by the fitting equations shown in Table 4.

Table 4 Fitting equations for non-uniform gradations

4.3 Effect of porosity on capillary rise

The height of capillary rise, which appears in Eq. (1), is inversely proportional to the diameter of void space. In a random packing of ores, the representation of pore system as a bundle of capillary tubes does not hold in this situation. It is difficult to estimate such a parameter due to the arbitrary pore geometry. Porosity is the other factor that could affect the capillary rise. Porosity is defined as the volume fraction of pores to ore column, and the porosity of mono-size gradation is shown in Table 1. The parameters, a and b, of the fitting Eq. (4) are selected as the indicator of capillary rise process. The relationship between parameters and porosity of mono-size gradations is shown in Fig. 6.

Parameter a decreases linearly with the porosity, while b increases with the increase of porosity. The relationship between porosity and capillary height can not be obtained from the inverse trend of the parameters. The ultimate capillary height can be obtained from Fig. 4. And the decreasing trend of ultimate height with increasing porosity is shown in Fig. 7. The fine gradation has lower porosity, more pore number, and larger surface area. All these properties result in higher surface tension, which leads to higher capillary height. On the contrary, coarse gradation has higher porosity, less pore number, and smaller surface area, which leads to lower capillary height. The test result provides the best fit with the theoretical analysis.

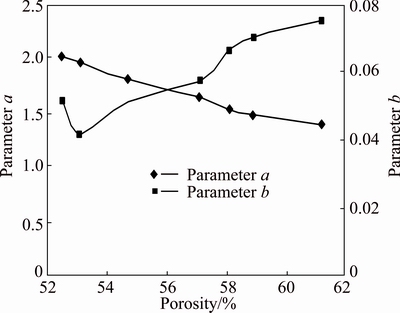
Fig. 6 Relationship between parameters and porosity

Fig. 7 Relationship between ultimate height and porosity

4.4 Evolution of moisture content during capillary process

The capillary rise results in the increase of moisture content, and water film thicknesses on particle surface increase. More and more macropores become water filled. Consequently, attractive capillary forces become less dominant. Therefore, the moisture content inside the column varies during capillary process. Before being packed into the column, the ore samples were dried by baking oven. The moisture content was directly measured using time domain reflectometry (TDR) 100 moisture gauge during the capillary process. Five groups (Gradations C, D, E, F, G) were selected with moisture content to be measured at the bottom of column, and test data are shown in Fig. 8.

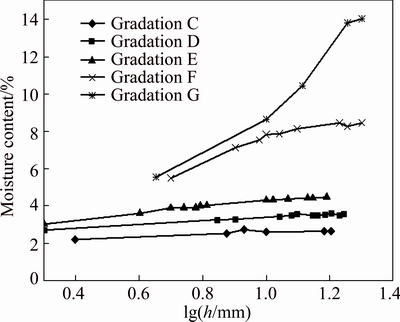
Fig. 8 Moisture content at bottom of column during capillary rise

Increased capillary time corresponds to greater water contents at the bottom of column [21]. The moisture content increases with the capillary rise level going up. By comparing the data of different gradations, we find a negative relationship between moisture content and porosity. In general, the smaller the particle size is, the higher the water content is. Taking logarithm of the capillary height, the relationship between capillary height and moisture content can be expressed by

θ=m(lg h)ⁿ                                   (5)

where θ is the moisture content, m, n are parameters related to the porosity or particle size. The relationship between moisture content and capillary rise was expressed by the fitting equations shown in Table 5.

Table 5 Relationship between moisture content and capillary rise

5 Conclusions

1) By measuring the capillary rise in mono-size particles, the relationship between rise height and time is described by the fitting equation. Both of the capillary ultimate height and rise velocity have an inverse relationship with particle size. In practice, fine particles obtained by crushing or dumping facilitate the capillary process inside the heap.

2) Capillary rise height within the non-uniform particle is tested. The poorly graded group exhibits small rise height and velocity. The capillary rise in the well graded particles is much better than that in the poor graded ones, because fine particles filling large pores reduce the capillary radius. A wide range particle size could benefit the capillary flow and solution holdup inside the heap.

3) The relationship between fitting equation parameters and mono-size gradation porosity is obtained. The low porosity and high surface tension lead to higher capillary height of the fine gradation. Moisture content increases as the capillary rise level goes up, the relationship between capillary height and moisture content is obtained.

References

[1] MCBRIDE D, GEBHARDT J E, CROSS M, A comprehensive gold oxide heap leach model: Development and validation [J]. Hydrometallurgy, 2012, 113-114: 98-108.

[2] TRUJILLO J Y, CISTERNAS L A, GALVEZ E D, MELLADO M E. Optimal design and planning of heap leaching process: Application to copper oxide leaching [J]. Chemical Engineering Research and Design, 2014, 92(2): 308-317.

[3] DING De-xin, FU Hai-ying, YE Yong-jun, HU Nan, LI Guang-yue, SONG Jian-bin, WANG Yong-dong. A fractal kinetic model for heap leaching of uranium ore with fractal dimension of varied particle size distribution [J]. Hydrometallurgy, 2013, 136: 85-92.

[4] NOSRATI A, SKINNER W, ROBINSON D J, MENSAH J A. Microstructure analysis of Ni laterite agglomerates for enhanced heap leaching [J]. Powder Technology, 2012, 232: 106-112.

[5] MCBRIDE D, CROSS M, GEBHARDT J E. Heap leach modeling employing CFD technology: A ‘process’ heap model [J]. Minerals Engineering, 2012, 33: 72-79.

[6] ILANKOON I M S K, NEETHLING S J. The effect of particle porosity on liquid holdup in heap leaching [J]. Minerals Engineering, 2013, 45: 73-80.

[7] MELLADO M E, CISTERNAS L A, GALVEZ E D. An analytical model approach to heap leaching [J]. Hydrometallurgy, 2009, 95(1): 33-38.

[8] MELLADO M E, GALVEZ E D, CISTERNAS L A. On the optimization of flow rates on copper heap leaching operations [J]. International Journal of Mineral Processing, 2011, 101(1): 75-80.

[9] FAGAN M A, SEDERMAN A J, JOHNS M L. MR imaging of ore for heap bioleaching studies using pure phase encode acquisition methods [J]. Journal of Magnetic Resonance, 2012, 216, 121-127.

[10] BOUFFARD S C, WEST-SELLS P G. Hydrodynamic behavior of heap leach piles: Influence of testing scale and material properties [J]. Hydrometallurgy, 2009, 98(1): 136-142.

[11] METODIEV B, LILOVA K, KARAMANEV D, LILOV L. Computational fluid dynamics (CFD) simulation of velocity and concentration profiles in the vicinity of immobilized Acidithiobacillus ferrooxidans cells [J]. Biochemical Engineering Journal, 2006, 29(3): 182-190.

[12] MOUSAVI S M, JAFARI A, YAGHMAEI S, VOSSOUGHI M, SARKOMAA P. Computer simulation of fluid motion in a porous bed using a volume of fluid method: Application in heap leaching [J]. Minerals Engineering, 2006, 19(10): 1077-1083.

[13] CARIAGA E, CONCHA F, SEPULVEDA M. Convergence of a MFE–FV method for two phase flow with applications to heap leaching of copper ores [J]. Computer Methods in Applied Mechanics and Engineering, 2007, 196(25): 2541-2554.

[14] ANDRADE LIMA L R P. Liquid axial dispersion and holdup in column leaching [J]. Minerals Engineering, 2006, 19(1): 37-47.

[15] YANG Bao-hua, WU Ai-xiang, MIAO Xiu-xiu, LIU Jin-zhi. 3D characterization and analysis of pore structure of packed ore particle beds based on computed tomography images [J]. Transactions of Nonferrous Metals Society of China, 2014, 24(3): 833-838.

[16] WU Ai-xiang, YIN Sheng-hua, QIN Wen-qing, LIU Ji-shan, QIU Guan-zhou. The effect of preferential flow on extraction and surface morphology of copper sulphides during heap leaching [J]. Hydrometallurgy, 2009, 95(1): 76-81.

[17] YIN Sheng-hua, WU Ai-xiang, HU Kai-jian, WANG Yi-ming, XUE Zhen-lin. Visualization of flow behavior during bioleaching of waste rock dumps under saturated and unsaturated conditions [J]. Hydrometallurgy, 2013, 133: 1-6.

[18] LIN C L, VIDELA A R, MILLER J D. Advanced three-dimensional multiphase flow simulation in porous media reconstructed from X-ray Microtomography using the He–Chen–Zhang Lattice Boltzmann Model [J]. Flow Measurement and Instrumentation, 2010, 21(3): 255-261.

[19] LOCKINGTON D A, PARLANGE J Y. A new equation for macroscopic description of capillary rise in porous media [J]. Journal of Colloid and Interface Science, 2004, 278(2): 404-409.

[20] LAGO M, ARAUJO M. Capillary rise in porous media [J]. Physica A: Statistical Mechanics and its Applications, 2001, 289(1): 1-17.

[21] VETHOSODSAKDA T, FREE M L, JANWONG A, MOATS M S. Evaluation of liquid retention capacity measurements as a tool for estimating optimal ore agglomeration moisture content [J]. International Journal of Mineral Processing, 2013, 119: 58-64.

矿石粒度与堆体孔隙率对浸出堆体内毛细过程的影响

尹升华¹，王雷鸣¹，陈 勋¹，吴爱祥²

1. 北京科技大学 金属矿山高效开采与安全教育部重点实验室，北京 100083；

2. 北京科技大学 土木与环境工程学院，北京 100083

摘  要：由于双重孔隙结构的存在，溶液毛细作用与重力流共同存在于浸堆内。毛细作用是细颗粒矿石和内部粗糙岩石溶解的主要原因。通过一系列柱浸实验探究了粒径和堆体孔隙率对毛细管过程的影响。证实堆体内存在大孔隙，并且依此提出毛细上升理论。柱浸实验使用两组矿石颗粒，即单一粒径颗粒与非均匀颗粒。研究结果表明：颗粒大小与毛细管最终高度成反比，并且颗粒越小，毛细上升越高。颗粒分级程度较低时，毛细上升高度和速度较小，然而颗粒分级程度较高时毛细上升高度和速度较大。获得了孔隙度和毛细上升的拟合参数之间的关系。柱浸颗粒较细时低孔隙度和高表面张力导致更高的毛细高度。毛细管水分含量随毛细上升高度增加而增加，并获得了毛细管高度和水分含量之间的关系。

关键词：堆浸；毛细过程；矿石粒度；堆体孔隙率

(Edited by Yun-bin HE)

Foundation item: Project (51374035) supported by the National Natural Science Foundation of China; Project (201351) supported by the Foundation for the Author of National Excellent Doctoral Dissertation of China; Project (NCET-13-0669) supported by Program for New Century Excellent Talents in University, China

Corresponding author: Sheng-hua YIN; Tel: +86-10-62334680; E-mail: csuysh@126.com

DOI: 10.1016/S1003-6326(16)64174-2