­­Quantum-mechanical study of effect of lattice defects on surface properties and copper activation of sphalerite surface

CHEN Jian-hua(陈建华)¹, CHEN Ye(陈 晔)², LI Yu-qiong(李玉琼)²

1. College of Resources and Metallurgy, Guangxi University, Nanning 530004, China;

2. College of Chemistry and Chemical Engineering, Guangxi University, Nanning 530004, China

Received 15 July 2009; accepted 2 November 2009

Abstract: The electronic properties of sphalerite (110) surface bearing Fe, Mn and Cd impurities were calculated using density-functional theory, and the effects of impurities on the copper activation of sphalerite were investigated. Calculated results indicate that both Fe and Mn impurities narrow the band gap of sphalerite surface and lead to the Fermi level shifting to conduction band. Impurity levels composed of Fe 3d and Mn 3d orbital appearing in band gap are beneficial to electrons transfer from the valence band to the conduction band and promote the surface conductivity and the electrochemical activity. The results show that Fe and Mn impurities cannot be replaced by Cu atom, which reduces the exchange sites (Zn) for Cu atom, hence Fe- and Mn-bearing sphalerites are hard to be activated by copper. Cd impurity has little effect on electronic structure of sphalerite surface; however, Cd atom is easily replaced by Cu atom, and this is the reason why the Cd-bearing sphalerite can be easily floated.

Key words: sphalerite; lattice impurity; DFT calculation; copper activation

1 Introduction

Sphalerite is an important Zn-bearing mineral and the primary source of zinc metal. Sphalerite can be recovered by froth flotation. However, it does not respond well to short chain thiol collectors due to the relative instability of zinc-xanthate; and hence, the requirement of copper activation to enhance the adsorption between collector molecules and the sphalerite surface is necessary[1]. Natural sphalerite commonly contains impurities such as iron, cadmium, manganese and copper substituted for zinc atoms, which displays a wide range of colors in nature. The iron content of sphalerite varies from 0.4% to 22% (mass fraction) with different temperatures and chemical environments of the crystallization. Sphalerite is called marmatite when the content of iron is over 6%[2]. Sphalerite enriched by cadmium is called pribramite. It is well known that Mn impurity is also found commonly in the natural sphalerite. The impurities in sphalerite play an important role in the process of sphalerite flotation. Generally, the presence of iron makes the floatability of sphalerite become poor, and inversely, the presence of cadmium makes sphalerite floated easily[3].

It has been known that the lattice defects can exert  considerable influence on the surface properties of minerals[4]. The presence of iron decreases the band gap of sphalerite, which is naturally an insulator, and affects its reactivity[5]. The iron impurity influences the copper activation and subsequent flotation behavior of sphalerite; however, contradictory results have been reported. Recently, BOULTON et al[6] reported that the presence of iron in the sphalerite lattice reduces the exchange sites (zinc) for Cu²⁺. However, the XPS analysis showed that the amount of Cu²⁺ adsorbed onto the sphalerite surface increased as the iron content of sphalerite increased[7]. HARMER et al[4] considered that the high iron content of the sphalerite increased the number of surface defect sites and allowed more amount of Cu²⁺ adsorbed onto the sphalerite surface compared with samples with low iron content (less defect sites). In addition, samples with content of Fe impurity undergo a rapid oxidation. As we know, the Cd impurity in lattice improves the floatability of sphalerite; however, the nature of cadmium affecting the flotation behaviors of sphalerite has been few reported.

In order to investigate the role of impurity in the  sphalerite flotation in nature, we used computer simulation techniques to model the structure of sphalerite (110) surface bearing three typical impurities including Fe, Mn and Cd. And the influence of these three impurities on the electronic properties and copper activation of sphalerite surface was studied using density-functional theory (DFT). This can provide an important insight into the effect of lattice defects on the surface properties and copper activation processes of sphalerite.

2 Computational methods and model

Calculations have been done using Cambridge Serial Total Energy Package (CASTEP) developed by PAYNE et al[8], which is a first-principle pseudopotential method based on the DFT. DFT calculations employing plane wave (PW) basis sets and ultrasoft pseudopotentials have been performed[9-10]. The exchange correlation functional used was the generalized gradient approximation (GGA) developed by PERDEW, BURKE and ERNZERHOF (PBE)[11]. The interactions between valence electrons and ionic core were represented by ultra-soft pseudo-potentials. Valence electrons configuration considered in this study included Zn 3d¹⁰4s², S 3s ²3p⁴, Fe 3d⁶4s ², Mn 3d⁵4s², Cd 4d¹⁰5s² states. The kinetic energy cutoff (310 eV) of the plane wave basis was used throughout, and the Brillouin zone was sampled with Monkhorst and Pack special k-points of a 2×3×1 grid for all structure calculations[12], which showed that the cutoff energy and the k-points mesh were sufficient for the system. For self-consistent electronic minimization, the Pulay Density Mixing method was employed and the convergence tolerance was 2.0×10^-6 eV/atom. The energy tolerance was 2.0× 10^-5 eV/atom; the force tolerance was 0.05 eV/?; and the displacement tolerance was 0.002 ?.

Zinc sulfide is naturally observed in two polymorphs: zinc blende and wurtzite, with cubic and hexagonal lattice structures, respectively. The calculation is based on zinc blende structure with space group of 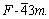 The cell parameter is a=b=c=5.41 ? and α=β=γ=90?. Studies have shown that the sphalerite (110) surface undergoes considerable relaxation, resulting in a low surface free energy[13]. Hence, in this study, the sphalerite (110) surface was chosen for the calculation. The construction of sphalerite (110) plane was as follows. The first step was to optimize primitive cell. The equilibrium lattice parameter of sphalerite is 5.50 ?, close to the experimental value of 5.41 ?. The second step was to cleave a 5 atomic-layer slab of sphalerite (110) plane based on the optimized primitive cell, then a 2×2×1 supercell was created, as shown in Fig.1. The thickness of vacuum slab was 15 ?. Finally, the geometric optimization of sphalerite (110) plane was performed.

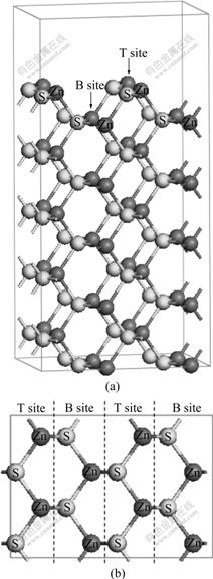
Fig.1 Model of sphalerite (110) surface: (a) Side view; (b) Top view

The substitution energy of an atom X (Fe, Mn, Cd or Cu) for Zn atom of sphalerite (110) surface is defined as

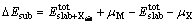              (1)

where 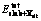and 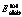 are the total energies of the slab with and without a hetero-atom X on the site, respectively. The atomic chemical potential, μ, is evaluated in its reference state. Here, we define the atomic chemical potential as a calculated total energy per atom in its optimized reference state[14]. More negativity of ΔE_sub indicates that the substitution  reaction is easier to occur.

3 Results and discussion

3.1 Geometry optimization and relaxation of sphalerite (110) surface

The calculated structural parameters of perfect sphalerite (110) surface and the results of low-energy electron diffraction (LEED) analysis are shown in Table 1. Schematically, the relaxation is shown in Fig.2, which also defines the structural parameters in Table 1. Our calculation results are in good agreement with the experimental values. We have also calculated the relaxed surface energy of the sphalerite (110), with the value of 0.34 J/m², which is very close to the value of 0.35 J/m² obtained from STEELE’s research[15]. Thereby, our surface model is credible.

Table 1 Parameters for sphalerite (110) surface relaxation

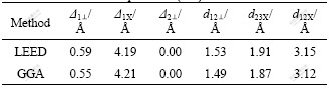
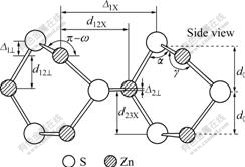
Fig.2 Side view of sphalerite surface unit cell

3.2 Effect of Fe, Mn and Cd impurities on sphalerite surface

According to the model shown in Fig.1, there are two different Zn-sites for substitution in every layer, which defined as top (T) site and bottom (B) site, respectively. The geometry optimizations of impurity atoms (Fe, Mn and Cd) substituting for different Zn-site atoms have been done by DFT. The calculated substitution energies are listed in Table 2.

The values of 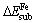 for Fe atom substituting Zn are negative, which indicates that Zn atom is replaced by Fe impurity easily. This can interpret the wide distribution of Fe-bearing sphalerite in nature. In addition, the values of  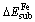of B-site in 1st layer and T-site and B-site in 2nd layer are similar, which indicates that the substitution of Zn atom with Fe atom located at these three sites are possible to occur. The values of  for Mn atom substituting Zn are also negative, which means that the substitution reaction is relatively easy to occur. Furthermore, the value of 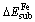 (-458.36 kJ/mol) is more negative than (-442.70 kJ/mol), which implies that Fe impurity is easier to substitute Zn atom than Mn impurity.

Table 2 ΔE_sub of Fe, Mn, Cd substituting for Zn atom on sphalerite surface

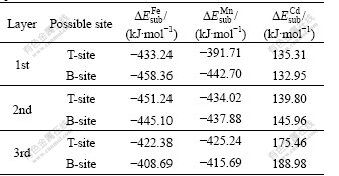
However, the values of 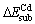 for all the possible Zn-sites are positive, which indicates that Cd atom cannot substitute for Zn spontaneously, but this substitution reaction is possible to occur in the crystallization process of sphalerite at high temperature (500-600 ℃). As the flotation reaction occurs at the first layer of the mineral surface, this study mainly discusses the effect of Fe, Mn and Cd impurities at the B site at the first layer on structure and properties of the sphalerite (110) surface.

The presence of impurities will result in the change of the electronic properties of sphalerite and will affect the subsequent reactions occurring on the sphalerite surface. The band structure and density of states of perfect, Fe-doped, Mn-doped and Cd-doped sphalerite (110) surface are shown in Figs.3-6. The calculated band gap of perfect surface is 2.76 eV, which is less than the experimental band gap value of 3.60 eV, due to the underestimation by GGA approximation[16]. However, this does not affect the accuracy of the description of the total energy and related properties of crystals.

Both Fe and Mn are the first-transition elements, and their outer electronic configurations are similar. Therefore, the effects of Fe and Mn impurities on band structure of sphalerite surface are similar. Fe and Mn impurities narrow the band gap of sphalerite surface, as shown in Fig.4(a) and Fig.5(a), which indicates that the conductivities of sphalerite bearing Fe and Mn impurities are enhanced. In addition, the Fermi level shifts to the conduction band and semiconductor type of Fe-doped and Mn-doped sphalerite changes from p-type to n-type. It should be noted that there are two new surface energy levels located at -0.65 eV and 0.04 eV in forbidden band, which is composed of two parts: one is the impurity level of splitting of Fe 3d and Mn 3d orbits in tetrahedron field, and the other is the surface level caused by splitting of  S 3p orbital due to the existence of Fe and Mn impurities. The increase of conductivity and the presence of impurity levels in band gap could enhance the electrochemical activity of sphalerite surface, and thus, could affect the subsequent surface oxidation and xanthate adsorption of sphalerite.

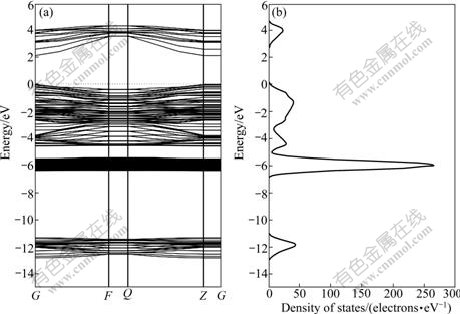
Fig.3 Band structure (a) and DOS (b) of perfect sphalerite surface

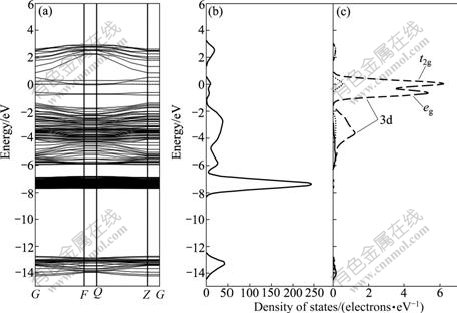
Fig.4 Band structure (a), DOS (b) and Fe PDOS (c) of Fe-doped sphalerite surface

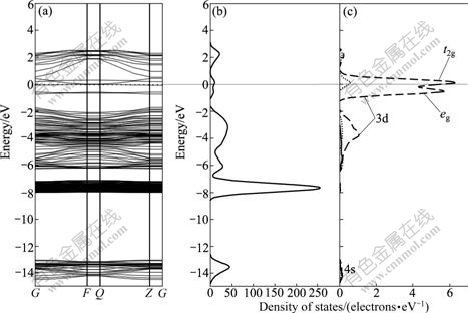
Fig.5 Band structure (a), DOS (b) and Mn PDOS (c) of Mn-doped sphalerite surface

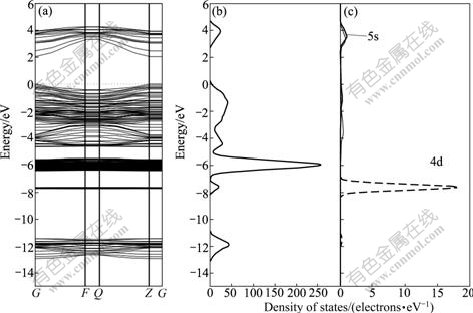
Fig.6 Band structure (a), DOS (b) and Cd PDOS (c) of Cd-doped sphalerite surface

Compared with the perfect sphalerite, band structure of Cd-bearing sphalerite changes slightly because Cd and Zn are in the same group and they have the similar electronic configuration. Figs.5(a) and (c) show that a new energy level around -7.75 eV is generated by Cd 4d state, which has little effect on the electronic properties of Cd-bearing sphalerite surface due to its deeper location in the valence band. The Fermi level and surface state density are almost the same as those of perfect sphalerite.

The total DOS of the top three layers of perfect sphalerite surface are shown in Fig.7. The valence band can be divided into two main parts. The deepest valence band located at -11.76 eV is composed of S 3s orbital.

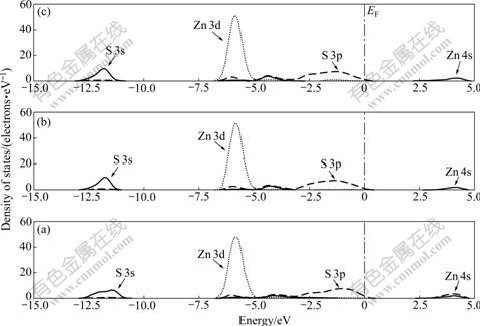
Fig.7 DOS of top three layers of perfect sphalerite surface: (a) 1st layer; (b) 2nd layer; (c) 3rd layer

The upper valence band located at -6.0 eV is mainly composed of Zn 3d and S 3p orbitals. The conduction band is mainly composed of Zn 4s and S 3p orbitals.

The DOS of the top three layers of Fe-bearing sphalerite surface and PDOS of Fe atom are shown in Fig.8. It is clear that the effects of Fe impurity on density of states of sphalerite surface are mainly on the 1st layer and the density of states of the 2nd layer and 3rd layer change slightly. Compared with perfect sphalerite surface, the deepest valence band shifts from -11.76 eV to -13.32 eV, because the electro-negativity of Fe atom (1.85) is greater than that of Zn atom (1.60); and hence, the Fe atom attracts a bonding pair of electron with S atom rather than Zn atom, resulting in the decrease of S 3p charge. The Zn 3d orbital moves from -6.0 eV to -7.44 eV due to the lack of Zn 3d contribution. Fe impurity on sphalerite surface mainly affects the distribution of DOS near Fermi level. Fe 3d orbital splits into t_2g and e_g orbitals that are near Fermi level, which are located at -0.72 eV and 0.24 eV, respectively.

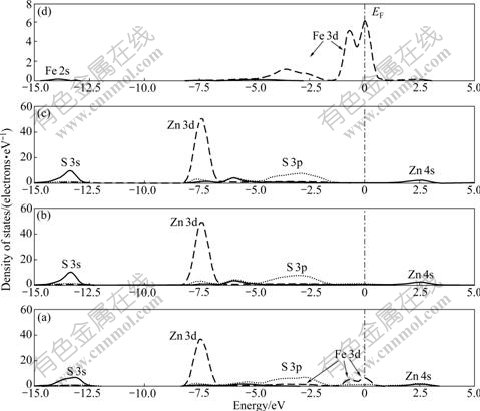
Fig.8 DOS of top three layers of Fe-bearing sphalerite surface: (a) 1st layer; (b) 2nd layer; (c) 3rd layer; (d) Fe impurity

The DOS of the top three layers of Mn-bearing sphalerite surface and PDOS of Mn atom are shown in Fig.9. It should be noted that two new energy levels appear in the 1st layer which are located at -0.50 eV and 0.25 eV, respectively. These two energy levels are composed of two parts: one is the impurity level of splitting of Mn 3d orbital in tetrahedron field and the other is the surface level of splitting of S 3p orbital due to the existence of Mn impurity. The Zn 3d orbital shifts from -5.60 eV to -7.75 eV due to the lack of one Zn atom.

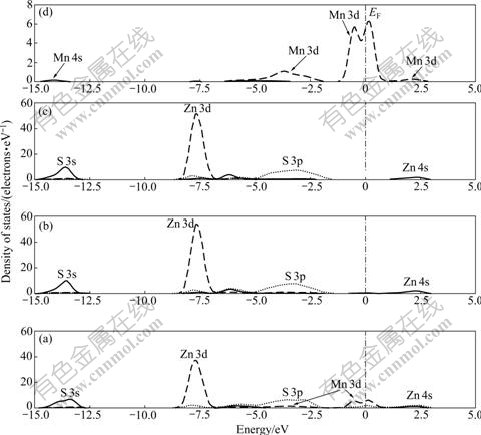
Fig.9 DOS of top three layers of Mn-bearing sphalerite surface: (a) 1st layer; (b) 2nd layer; (c) 3rd layer; (d) Mn impurity

The DOS of the top three layers of Cd-bearing sphalerite surface and PDOS of Cd atom are shown in Fig.10. Compared with Fig.7, a new peak located at about -7.75 eV appears, which is from the contribution of Cd 4d orbital because this new level is located in the deeper part of the DOS which has little effect on surface state of sphalerite.

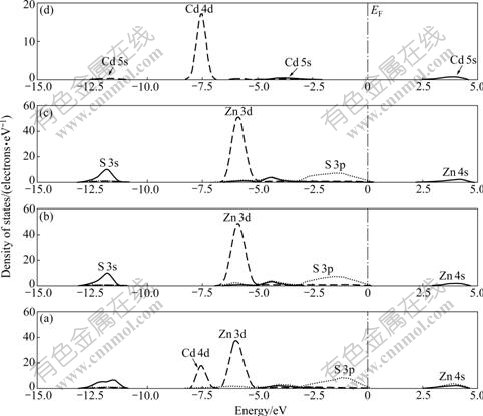
Fig.10 DOS of top three layers of Cd-bearing sphalerite surface: (a) 1st layer; (b) 2nd layer; (c) 3rd layer; (d) Cd impurity

The Mulliken populations of the first layer of perfect and Fe-, Mn-, Cd-bearing sphalerite surface are presented in Figs.11(a), (b), (c) and (d), respectively. There are two different atomic sites on the surface of sphalerite: T-site and B-site, as shown in Fig.1. It is shown in Fig.9(a) that the Mulliken charges of Zn and S atoms located at T- or B-site are different. The charges of Zn and S atom located at T-site are 0.35 and -0.50, respectively, while the charges of Zn and S atom located at B-site are 0.31 and -0.49, respectively.

For Fe-doped sphalerite surface, the charge of Fe atom is 0.12 and the charge of neighboring S atoms decreases from -0.49 to -0.41. This can be ascribed to the larger electronegativity of Fe atom (1.80), and more electrons of neighboring S atoms are attracted to Fe atom. For Mn-doped sphalerite surface, the charge of Mn atom is 0.16 and the charge of neighboring S atoms decreases from -0.49 to -0.40, while the charge of Zn atom increases from 0.31 to 0.28. It is obvious that Zn atom gets more electrons from neighboring S atoms. This is because the electronegativity of Mn (1.55) is smaller than Zn (1.65), and the electrons of neighboring S atoms are attracted to Zn atom.

However, for Cd-doped sphalerite surface, the charge of neighboring S atoms becomes more negative. The electronegativity of Cd (1.69) is almost the same as that of Zn (1.65); whereas, the covalent radius of Cd (1.48 ?) is greater than that of Zn (1.25 ?), which results in less attraction ability of Cd atom.

3.3 Effect of Fe, Mn and Cd impurities on copper activation of sphalerite

The flotation of sphalerite depends on the copper activation on its surface. The sphalerite activation has been studied extensively over several decades[17]. It has been well established that copper activation of sphalerite follows an ion exchange mechanism where the uptake of Cu(Ⅱ) results in approximately 1:1 release of Zn ²⁺ into the solution[18] and is generally represented by Eq.(2) [19]:

ZnS(s)+Cu²⁺(aq)→Cu_xZn_1-xS(s)+Zn²⁺(aq)        (2)

GERSON et al[18] considered that at low concentration, Cu ions arriving at the sphalerite surface are incorporated into Zn site on the surface with release of the Zn to solution. At higher copper concentrations, further reactions to the second layers and subsequent layers of the sphalerite take place by site exchange between the Cu in the 1st layer and Zn atom in the 2nd layer or by interstitial movement of Cu atom through the lattice.

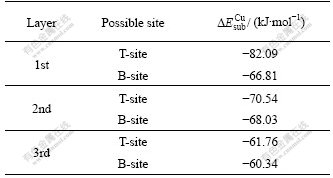
According to the model shown in Fig.1, there are two possible Zn sites for Cu substitution in every layer. The calculated results of  for different Zn sites replaced by Cu of top three layers are listed in Table 3. It can be seen from Table 3 that the exchange energy of Cu atom with T-site Zn atom in the 1st layer is the minimum, hence the T-site is the most possible site for Cu-Zn exchange and the most favorable  of Cu exchanged with Zn atom on sphalerite surface is determined to be -82.09 kJ/mol.

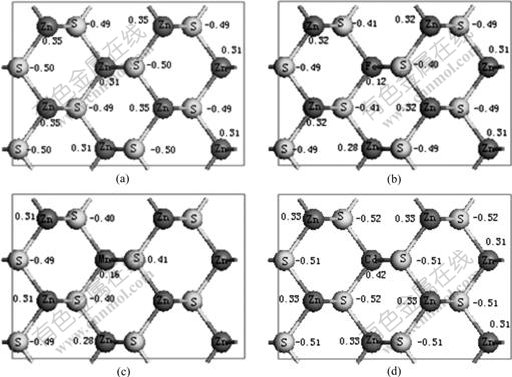
Fig.11 Mulliken charges of sphalerite surface: (a) Perfect sphalerite; (b) Fe-doped sphalerite; (c) Mn-doped sphalerite; (d) Cd-doped sphalerite

Table 3 Substitution energy  for possible Zn sites replaced by Cu

The influence of impurities on copper activation still remains controversial. For further understanding the effect of impurities on copper activation, the simulations of copper activation of Fe-, Mn- and Cd-bearing sphalerite (110) surface were carried out by DFT.

Due to the presence of impurity atom on sphalerite surface, a subsequent question about whether the impurity atom can be exchanged by Cu atom or not need to be ascertained. We simulate the replacement of Fe, Mn and Cd atom by Cu atom, respectively. The calculated substitution energies are listed in Table 4.

Table 4 ?E_sub values for Fe, Mn, Cd impurities replaced by Cu

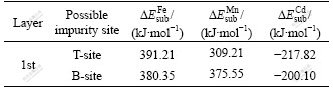
The calculated ?E_sub values of Cu substitution for Fe and Mn atom in the 1st layer are all positive, which implies that Fe atom and Mn atom cannot be replaced by Cu atom due to the unfavorable energies. It is indicated that the total exchange sites (Zn) for Cu are reduced due to the presence of Fe and Mn impurities on the surface, which leads to the decrease of amount of Cu adsorption on sphalerite surface.

On the contrary, the ?E_sub values of Cu substitution for Cd atom in the 1st layer are negative. Compared with the results listed in Table 3, it is obvious that the ?E_sub  of Cu substitution for Cd is more negative than that of Cu substitution for Zn, which means that the impurity Cd is easier to be replaced by Cu atom. In other words, sphalerite bearing Cd impurity can be activated more easily by copper than perfect sphalerite.

1) The presence of Fe and Mn impurities narrows the band gap of sphalerite surface and results in Fermi level shifting to the conduction band, which implies that the conductivities of Fe-doped and Mn-doped sphalerite are improved. The semiconductor type of Fe-doped and Mn-doped sphalerite changes from p-type to n-type. The 3d orbitals of Fe and Mn atoms split into two peaks around the Fermi level, leading to new surface energy levels appearing in forbidden band. The increase of conductivity and the presence of impurity levels in band gap are beneficial to the electron transition and could improve the electrochemical activity of sphalerite surface; and thus, affect the subsequent surface oxidation and xanthate adsorption of sphalerite. Fe impurity leads to the reduction of Mulliken charge of S atom bonded with Fe, while Mn impurity results in the increase of Mulliken charge of Zn atom.

2) The activation of copper on sphalerite surface is a substitution reaction, and the results show that Fe and Mn atoms on sphalerite surface cannot be replaced by Cu atoms. Hence, the total sites (Zn) on sphalerite surface for Cu exchange are reduced, which results in the phenomenon that Cu adsorption amount on the surface of sphalerite is decreased. The results are very helpful for explaining the worse copper activation of real sphalerite surface bearing Fe.

3) The effect of Cd impurity on sphalerite surface is very different from Fe and Mn impurities. The band structure and density of states of sphalerite (110) surface change slightly. It is easy for Cu atom to substitute Cd atom, which indicates that sphalerite bearing Cd impurity can be more easily activated by copper than perfect sphalerite. In addition, Cd impurity is beneficial to the oxygen adsorption and oxidation of sphalerite surface. Hence, it is presumed that the Cd impurity on sphalerite flotation is mainly to enhance the adsorption performance of sphalerite surface but not to change the electronic properties.

References

[1] LEPPINEN J O. FTIR and flotation investigation of the adsorption of ethyl xanthate on activated and non-activated sulfide minerals [J]. International Journal of Mineral Processing, 1990, 30: 245-263.

[2] TONG Xiong, SONG Shao-xian, HE Jian. Activation of high-iron marmatite in froth flotation by ammoniacal copper(Ⅱ) solution [J]. Mineral Engineering, 2007, 20(9): 259-263.

[3] WANG Dian-zuo, JIAN Bai-xi. Mineral processing handbook [M]. Beijing: Metallurgy Industry Press, 1999: 26. (in Chinese)

[4] HARMER S L, MIERCZYNSKA V A, BEATTIE D A, SHAPTER J G. The effect of bulk iron concentration and heterogeneities on the copper activation of sphalerite [J]. Mineral Engineering, 2008, 21(11): 1005-1012.

[5] XIONG Xiao-yong. Effect of the iron content of zinc sulphide concentrates on their semi-conductivity and chemical reactivity [J]. Nonferrous Metal, 1989, 41(4): 55-66. (in Chinese)

[6] BOULTON A, FORNASIERO D, RALSTON J. Effect of iron content in sphalerite on flotation [J]. Mineral Engineering, 2005, 18(9): 1120-1122.

[7] HARMER S L, GONCHAROVA L V, KOLAROVA R, LENNARD W N. Surface structure of sphalerite studied by medium energy ion scattering and XPS [J]. Surface Science, 2007, 601: 352-361.

[8] PAYNE M C, TETER M P, ALLAN D C, ARIAS T A, JOANNOPOULOS J D. Iterative minimization techniques for ab initio total energy calculation: Molecular dynamics and conjugate gradients [J]. Reviews of Modern Physics, 1992, 64: 1045-1097.

[9] PERDEW J P, WANG Y. Accurate and simple analytic representation of the electron-gas correlation energy [J]. Physical Review B, 1992, 45: 13244-13249.

[10] VANDERBILT D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism [J]. Physical Review B, 1990, 41: 7892-7895.

[11] PERDEW J, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letter, 1996, 77: 3865-3868.

[12] MONKHORST H J, PACK J D. Special points for Brillouin-zone integrations [J]. Physical Review B, 1976, 13: 5188-5192.

[13] DUKE C B. Atomic and electronic-structure of tetrahedrally coordinated compound semiconductor interfaces [J]. Journal of Vacuum Science and Technology, 1988, 6: 1957-1962.

[14] KAZUME N, MASAHITO Y, MASAYUKI H. Energetics of Mg and B adsorption on polar zinc oxide surfaces from first principles [J]. Physical Review B, 2008, 77: 35330-35336.

[15] STEELE H M, WRIGHT K, HILLIER I H. A quantum-mechanical study of the (110) surface of sphalerite (ZnS) and its interaction with Pb²⁺ species [J]. Physics and Chemistry of Minerals, 2003, 30: 69- 75.

[16] ANISIMOV V I, ARYASETIAWAN F, LICHTENSTEIN A I. First-principles calculations of the electronic structure and spectra of strongly correlated systems: The LDA+ U method [J]. Journal of Physics: Condensed Matter, 1997, 9(4): 767-808.

[17] FINKELSTEIN N P. The activation of sulphide minerals for flotation: A review [J]. International Journal of Mineral Processing, 1997, 52: 81-120.

[18] GERSON A R, LANGE A G, PRINCE K E. SMART R S C. The mechanism of copper activation of sphalerite [J]. Applied Surface Science, 1999, 137: 207-223.

[19] CHANDRA A P, GERSON A R. A review of the fundamental studies of the copper activation mechanism for selective flotation of the sulfide minerals, sphalerite and pyrite [J]. Advances in Colloid and Interface Science, 2009, 145: 97-110.

Foundation item: Project(50864001) supported by the National Natural Science Foundation of China

Corresponding author: CHEN Ye; Tel: +86-771-3233565; Fax: +86-771-3233566; E-mail: fby18@126.com

DOI: 10.1016/S1003-6326(09)60266-1

(Edited by YANG Bing)