Mechanical properties and energy mechanism of saturated sandstones
来源期刊:中南大学学报(英文版)2018年第6期
论文作者:杨大方 牛双建 葛双双 党元恒 俞缙 张盛
文章页码:1447 - 1463
Key words:post-peak; saturation; strength property; damage mechanism; energy
Abstract: The effects of saturation on post-peak mechanical properties and energy features are main focal points for sandstones. To obtain these important attributes, post-peak cyclic loading and unloading tests were conducted on sandstone rock samples under natural and saturated states using the RMT-150B rock mechanics testing system. After successful processing of these tests, comparisons of stress–strain, strength, deformation, damage, and degradation of mechanical properties, wave velocity, and energy features of sandstone were conducted between natural and saturated states. The results show that saturation has evident weakening effects on uniaxial cyclic loading and unloading strength and elastic modulus of post-peak fracture sandstone. With the increase of post-peak loading and unloading period, the increases in amplitude of peak axial, lateral, and volumetric strains are all enhanced at approximately constant speed under the natural state. The increase in amplitude of axial peak strain is also enhanced at approximately constant speed, while the amplitudes of lateral and volumetric peak strains increase significantly under the saturated state. Compared with the natural state, the increase in amplitude of saturated samples’ peak lateral and volumetric strains, and the post-peak cyclic loading and unloading period all conform to the linearly increasing relationship. Under natural and saturated states, the damage factor (the plastic shear strain) of each rock sample gradually increases with the increase of post-peak cyclic loading and unloading period, and the crack damage stress of each rock sample declines rapidly at first and tends to reach a constant value later with the increase in plastic shear strain. Under natural and saturated states, the wave velocities of rock samples all decrease in the process of post-peak cyclic loading and unloading with the increase in plastic shear strain. The wave velocities of rock samples and plastic shear strain conform to the exponential relationship with a constant. Saturation reduces the total absorption energy, dissipated energy, and elastic strain energy of rock samples.
Cite this article as: NIU Shuang-jian, GE Shuang-shuang, YANG Da-fang, DANG Yuan-heng, YU Jin, ZHANG Sheng. Mechanical properties and energy mechanism of saturated sandstones [J]. Journal of Central South University, 2018, 25(6): 1447–1463. DOI: https://doi.org/10.1007/s11771-018-3839-z.

J. Cent. South Univ. (2018) 25: 1447-1463
DOI: https://doi.org/10.1007/s11771-018-3839-z
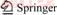
NIU Shuang-jian(牛双建)1, 2, 3, GE Shuang-shuang(葛双双)1, YANG Da-fang(杨大方)4,DANG Yuan-heng(党元恒)1, YU Jin(俞缙)2, ZHANG Sheng(张盛)1
1. School of Energy Science and Engineering, Henan Polytechnic University, Jiaozuo 454003, China;
2. Fujian Research Center for Tunneling and Urban Underground Space Engineering, Huaqiao University, Xiamen 361021, China;
3. Shenzhen Road and Bridge Construction Group Co., Ltd., Shenzhen 518028, China;
4. School of Civil Engineering, Henan Polytechnic University, Jiaozuo 454003, China
 Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2018
Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2018
Abstract: The effects of saturation on post-peak mechanical properties and energy features are main focal points for sandstones. To obtain these important attributes, post-peak cyclic loading and unloading tests were conducted on sandstone rock samples under natural and saturated states using the RMT-150B rock mechanics testing system. After successful processing of these tests, comparisons of stress–strain, strength, deformation, damage, and degradation of mechanical properties, wave velocity, and energy features of sandstone were conducted between natural and saturated states. The results show that saturation has evident weakening effects on uniaxial cyclic loading and unloading strength and elastic modulus of post-peak fracture sandstone. With the increase of post-peak loading and unloading period, the increases in amplitude of peak axial, lateral, and volumetric strains are all enhanced at approximately constant speed under the natural state. The increase in amplitude of axial peak strain is also enhanced at approximately constant speed, while the amplitudes of lateral and volumetric peak strains increase significantly under the saturated state. Compared with the natural state, the increase in amplitude of saturated samples’ peak lateral and volumetric strains, and the post-peak cyclic loading and unloading period all conform to the linearly increasing relationship. Under natural and saturated states, the damage factor (the plastic shear strain) of each rock sample gradually increases with the increase of post-peak cyclic loading and unloading period, and the crack damage stress of each rock sample declines rapidly at first and tends to reach a constant value later with the increase in plastic shear strain. Under natural and saturated states, the wave velocities of rock samples all decrease in the process of post-peak cyclic loading and unloading with the increase in plastic shear strain. The wave velocities of rock samples and plastic shear strain conform to the exponential relationship with a constant. Saturation reduces the total absorption energy, dissipated energy, and elastic strain energy of rock samples.
Key words: post-peak; saturation; strength property; damage mechanism; energy
Cite this article as: NIU Shuang-jian, GE Shuang-shuang, YANG Da-fang, DANG Yuan-heng, YU Jin, ZHANG Sheng. Mechanical properties and energy mechanism of saturated sandstones [J]. Journal of Central South University, 2018, 25(6): 1447–1463. DOI: https://doi.org/10.1007/s11771-018-3839-z.
1 Introduction
As the world industrial develops, mine resources demand is bigger and bigger. Moreover, with shallow resources drying up, more and more mining operations have entered the deep mining stage. According to incomplete statistics [1–6],more than 80 seats of super-kilometer-deep metallic resource mines were exploited at the beginning of the 21st century in foreign, including South Africa and India’s gold mines; Russia’s iron ore; and non-ferrous metal in Canada, Australia, the United States, and other countries. In coal mining, some of the main coal-producing countries have been deep mining since the 1960s, such as West Germany and the former Soviet Union. In terms of China’s proved coal reserves [7], the quantity of reserves under 1000 m accounts for approximately 53% of the total. Part of the statistical data indicate that as of 2012, more than 50 mines in China have reached kilometer-depth mining levels (average mining depth of 1086 m), which mainly distribute in Shandong, Henan, Jiangsu, and other districts in Eastern China [8]. At present, Chinese coal mine mining depth is still increasing, and it is predicted that more Chinese mines will reach depths of 1000 m to 1500 m in the next 20 years [7]. The rocks surrounding deep roadways are under high geo-stress conditions and in a deep complex hydrogeological environment, which greatly increases the difficulty of deep roadways surrounding rocks’ stability of maintenance. Statistics show that the renovation rate of deep mine roadways is as high as 200%, and most deep mine roadways need to be repaired many times to maintain the stability of surrounding rocks in the complex hydrogeological and mechanical environment [9, 10]. In the process of multiple damage repair, the surrounding rocks of roadways can experience frequent post-peak cyclic loading and unloading. A further study on the mechanical properties of saturated rock under post-peak cyclic loading and unloading conditions reveals that deepwater environment adds to the difficulty of maintaining the mechanical response characteristics of surrounding rocks of roadways in multiple maintenance operations. Therefore, it is of great theoretical and practical value to solve the bottleneck problem that affects the stability of surrounding rocks of roadways in deepwater mining environments.
In recent years, many researchers have investigated the effects of water on different rock properties. For example, CELIK et al [11], CHENG et al [12], DUDA et al [13], HASHIBA et al [14], and SHI et al [15] conducted studies on different types of rocks, examining the effects of water on compressive strength, tensile strength, flexural strength, and brittle failure strength. Their results show that compressive strength, tensile strength, flexural strength, and brittle failure strength of different types of rocks decrease in different degrees under the saturated state; hence, water has certain weakening effects on the mechanical properties of rocks. LIU et al [16] performed the creep experiments on deep saturated rock and obtained the creep curves of saturated rock under different loading stresses. Their results indicate that the rheological properties of saturated and natural rocks are quite different under high stress. Especially when unloading, the degradation and damage in rock quality are more severe, and the effects of water cannot be neglected. ZHAO et al [17] performed indirect dynamic Brazilian disc tension tests to study the failure characteristics of dry and saturated coals under impact loading conditions using a split Hopkinson pressure bar system. Their results show that saturated coal specimens have higher indirect tensile strength compared with dry ones. CHEN et al [18] prepared 54 coal-rock combinations split equally into groups containing different moisture content (dry, natural moisture, and saturated) to conduct acoustic emission testing under uniaxial compression. Their results show that the peak stress and strength-softening modulus, elastic modulus, strain-softening modulus, and post-peak modulus partly decreased with the increase in moisture content.
Other researchers have investigated the effects of cyclic loading on different rock properties. For instance, LIU et al [19] performed cyclic loading tests on rock salt to investigate the characteristics of damage evolution of surrounding rocks in gas caverns. Their results indicate that damage evolution is rather limited or negligible under cyclic loading. However, with the increase in stress levels, the damage evolution becomes more evident. AKESSON et al [20] conducted a mechanical test on drill cores from anisotropic granite, and the tested material was vacuum impregnated with epoxy resin containing fluorescent dye. Their results demonstrate that cyclic loading caused the appearance of new cracks and the extension of former microcracks. LIU et al [21] identified the relationship between the strength and deformation of rocks under cyclic loading. Later, they conducted a series of experiments to investigate the relationship. Their results show that the values of damage variables (the ratio of the dissipated energy to the constitutive energy) increased exponentially with strain. The damage constitutive models under cyclic loading have reasonable errors but the amended damage constitutive models can describe the degree of rock compactness accurately. ROBERTS et al [22] performed cyclic triaxial creep tests under various loading paths. Their results indicate that cyclic loading does not make the salt more prone to dilation compared with static loading conditions. LIANG et al [23], MA et al [24], and FUENKAJORN et al [25] performed experiments on the mechanical properties of rock salt under cyclic loading and triaxial cyclic loading conditions. LIU et al [26] performed a series of laboratory tests to assess the effects of frequency on the dynamic properties of sandstone samples subjected to cyclic loading under the confining stress state. The results from the cyclic loading tests indicate that frequency has a strong influence on dynamic deformation, dynamic stiffness, and failure mode under the same confining pressure. FATHI et al [27] conducted shear tests by two consecutive steps: load control and displacement control.
However, few researchers have investigated the effects of water on different rock properties under cyclic loading. BAGDE et al [28] conducted a test on intact sandstone samples subjected to dynamic uniaxial cyclic loading under natural and saturated states. They found that dynamic fatigue decreased under the saturated condition.
The mechanical properties of pre-peak rocks were emphasized by the mentioned scholar, but a large number of engineering practices indicate [29, 30] that most surrounding rocks in underground engineering are at the post-peak damage stage after excavation. Thus, it is more important to investigate rock properties in the post-peak stage under the saturated state. Thus, post-peak cyclic loading and unloading tests were carried out under natural and saturated states.
2 Experimental rock samples and equipment
2.1 Rock samples properties
Relatively uniform and intact sandstones were collected on the scene and made into standard cylinder samples. Machining precision met the standard requirements. There were micropores on the surface of the rock samples but no visible joints and cracks, which indicates that the homogeneity of rock samples is relatively good. The basic information of rock samples for this test is listed in Table 1.
Table 1 Basic information of rock samples
Rock samples were dried indoor for 15 d, and six rock samples were selected for this experiment. Three of them were natural rock samples, while the other three were chosen for the saturated experiment. The saturated rock samples were placed into the container. At first, water was added up to a quarter of rock samples’ height. Then, water was added every 2 h later. The water added every time that reaching to a quarter of rock samples’ height was advisable. Timing started when all rock samples were submerged in the water and the immersed rock samples were made into saturated samples for testing after 48 h.
2.2 Experimental equipment
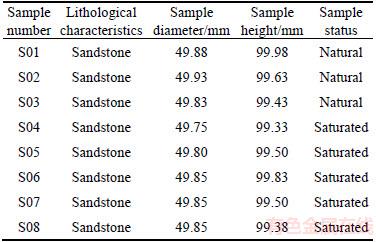
The RMT-150B rock mechanical experimental system shown in Figure 1 was used in the experiment. The axial load was measured by a 1000 kN pressure sensor (accuracy of 3‰ full scale (FS)), and the axial deformation of rock samples was measured by a 5 mm displacement sensor. The sensors were fixed by axial holding devices. Two 2.5 mm displacement sensors (accuracy of 1.5‰ FS) were fixed on the mounting plate by the lateral mounting base so that the circumferential expansion deformation of rock samples could be measured.
The experiment was automatically controlled by a digital computer, and the control modes of the computer include force, displacement, and stroke.
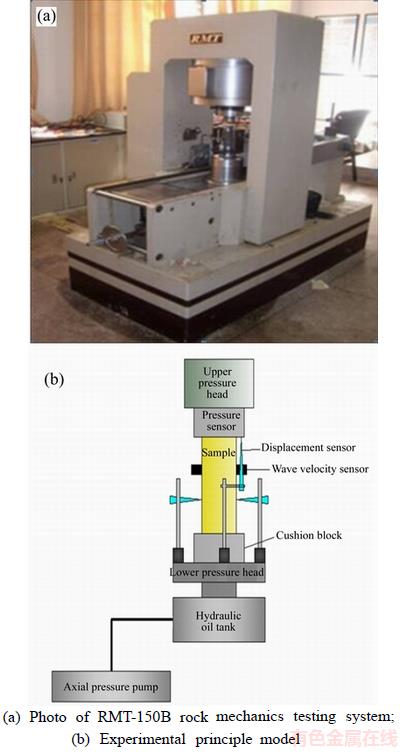
Figure 1 Test system:
In this experiment, when loading, the displacement control mode was used; when unloading, force control mode was selected. The microstructures of rock samples were observed through a scanning electron microscope.
2.3 Experimental methods
In this experiment, all rock samples were under the uniaxial stress state. In order to compare and analyze the experimental results of rock samples, loading and unloading stress paths designed for each rock sample were consistent with each other. Specific experiment process: firstly, rock samples were loaded up to the peak at 0.002 mm/s loading rate using the displacement control mode. Then, stress was reduced to 90%–95% of peak stress, and axial load was gradually unloaded to approximately 1 kN at 0.1 kN/s unloading rate using the force control mode. Finally, the rock samples experienced post- peak cyclic loading and unloading seven times in turn until the rock samples entered the residual strength stage. In addition, the change conditions of longitudinal wave velocities of rock samples in post-peak cyclic loading and unloading processes were monitored by a wave velocity monitoring device during the experiment.
3 Experimental results and analysis
Three natural and five saturated rock samples were tested in the uniaxial post-peak cyclic loading and unloading experiments. Owing to accidental human intervention in lateral sensor setting, two out of the five saturated rock samples could not display accurate circumferential deformation data in the experiment. When the experimental results were analyzed (axial and lateral deformation), only the data of the other three saturated rock samples were collected and adopted. In addition, owing to space limitations, the complete stress–strain curves of the natural state S02 and saturated state S06 rock samples are shown in Figure 2 under uniaxial post-peak cyclic loading and unloading conditions.
3.1 Stress–strain curve characteristics
Figure 2 shows that under natural and saturated states, the stress–strain curves of the rock samples can generally be divided into five stages: the initial densification stage AB, the linear elastic deformation stage BC, the pre-peak yield stage CD, the post-peak strain softening stage DE, and the residual strength stage E (not obvious), under uniaxial post-peak cyclic loading and unloading conditions.
Saturation has obvious effects on the characteristics of complete stress–strain curves’ five stages of sandstone rock samples under uniaxial post-peak cyclic loading and unloading conditions.
1) At the initial densification stage, the axial and volumetric strains of rock samples all increased under natural and saturated states; however, the circumferential deformation of each sample rises not obviously. Compared with the natural state, the axial, circumferential, and volumetric strains of saturated rock samples were more evident, especially at the densification stage, and the densification process of saturated rock samples is longer.
2) At the forepart of the linear elastic stage,under natural and saturated states, the axial and volumetric strains of all rock samples increase linearly with stress. Entering into the end of the linear elastic deformation stage, the axial strain continues to maintain linear growth; however, the circumferential strain transforms into nonlinear growth. Compared with the natural state, the transformation point of the circumferential strain of saturated rock samples occurs earlier with the increase in stress. The volumetric strain increases nonlinearly within the entire section with the increase in stress, and the volumetric strain curve approximately deflects at the middle linear elastic stage with a previous deflection point (volumetric expansion point) under saturated state. Moreover, compared with the natural state, the linear elastic stage is relatively shorter under the saturated state where the straight line slope decreases significantly. This indicates that water has obvious softening effects on the stiffness of rock.
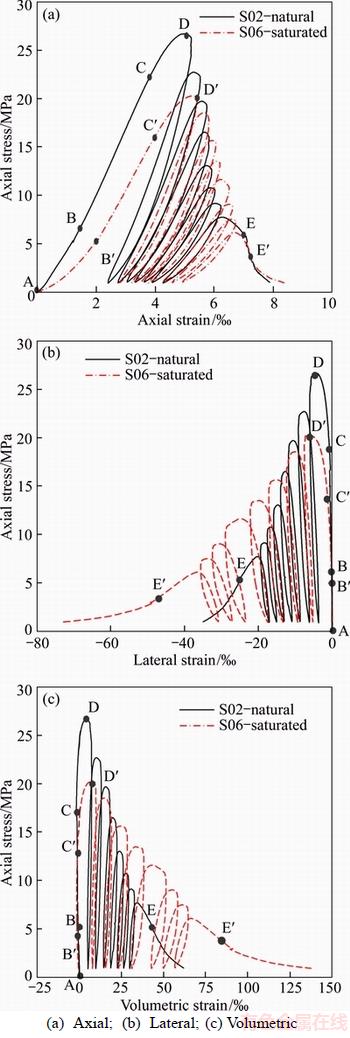
Figure 2 Stress–strain curves:
3) At the pre-peak yield stage, under natural and saturated states, the internal material with lower strength of rock samples gradually yielded in the plastic, and internal cracks formed and extended continuously. In the case of circumferential direction without effective constraint, the internal congregate energy of rock samples was constantly released in circumferential directions, which shows that the circumferential and volumetric strains of rock samples increased. In addition, volumetric strain of rock samples changed from negative to positive, and the dilation phenomenon occurred at this stage. Compared with the natural state, not only the pre-peak yield stage of saturated samples but also the curvature of the yield curve were relatively larger; hence, the yield process is more stable. The axial, circumferential, and volumetric strains of rock samples at the peak point all increased in different degrees.
4) At the post-peak strain softening stage, under natural and saturated states, the axial, circumferential, and volumetric strains all continued to increase at the initial stages of unloading. With gradual unloading, the compressed cracks gradually open, and because the material that did not yield recovers from elastic deformation, the axial, circumferential, and volumetric strains all gradually recover. Until the load decreases to approximately 1 kN, the axial, circumferential, and volumetric strains all recover in different degrees. The quantity of axial strain recovery is relatively obvious, while that of circumferential and volumetric strains are not. Compared with the natural state, saturation does not have obvious effects on the quantity of axial strain recovery, but it does affect the circumferential and volumetric strains. Saturation greatly weakens the recovery capability of rock samples from circumferential and volumetric strains after unloading. Moreover, the performance of weakening effects is increasingly obvious with the increase of post-peak cyclic loading and unloading periods and the accumulation of rocks bearing structural damage. The main reason is that the presence of water has obvious lubricating effects on the sliding friction between master fracture surfaces of the rock and rock particles, making it easier to slip and open. In addition, in the saturation state, post-peak strain softening characteristics of rock samples is more apparent and the failure process is relatively stable.
5) After experiencing post-peak cyclic loading and unloading for the same periods (seven times), the rock samples enter the residual strength stage, and the axial strain of rock samples have small differences under natural and saturated states. Owing to the heterogeneity of rock material, the axial strain of some saturated rock samples is even slightly lower than that of the natural state, but the average axial strain of saturated state is slightly higher than that of the natural state. For the circumferential and volumetric strains of rock samples, the strain of saturated rock is significantly greater than that under the natural state. At the end of cyclic loading and unloading experiments, under the saturated state, the axial stress–circumferential strain and axial stress–volumetric strain curves stretch approximately 3–4 width of the loading and unloading cycle compared with that of the natural state.
3.2 Strength and deformation characteristics
Figures 3–7 show the relationships between peak strength, elastic modulus (the slope of linear elastic section), peak strain, and loading and unloading cyclic periods in the uniaxial post-peak cyclic loading and unloading process, respectively.
From Figures 3–7, it is clear that saturation affects the strength and deformation characteristics of sandstone in the uniaxial post-peak cyclic loading and unloading process. Thus, the following conclusions can be made.
1) Saturation has obvious weakening effects on the strength of intact sandstone (zero loop). The peak uniaxial compressive strength of sandstone ranges from 25.769 to 30.538 MPa, with an average of 27.673 MPa under the natural state. The peak uniaxial compressive strength of saturated sandstone ranges from 18.442 to 22.055 MPa, and an average of 20.256 MPa. Therefore, the apparent softening coefficient of rock samples is 0.732 (the specific value of average peak strength between saturated sample and natural sample).
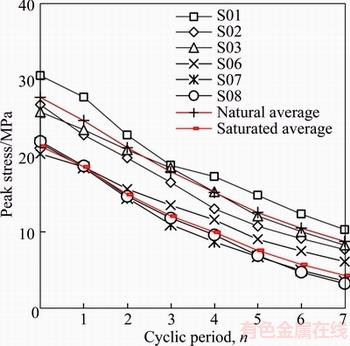
Figure 3 Relationship between peak stress and cyclic period
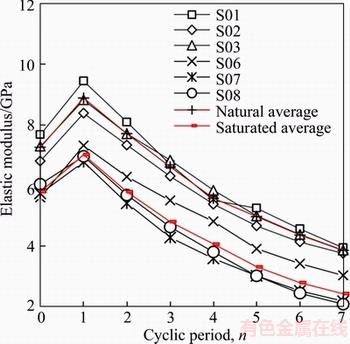
Figure 4 Relationship between elastic modulus and cyclic period
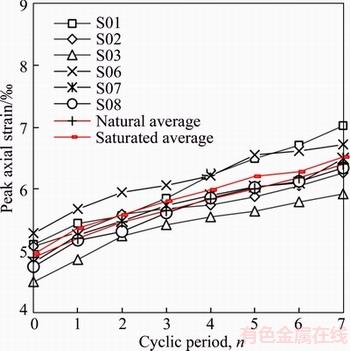
Figure 5 Relationship between peak axial strain and cyclic period
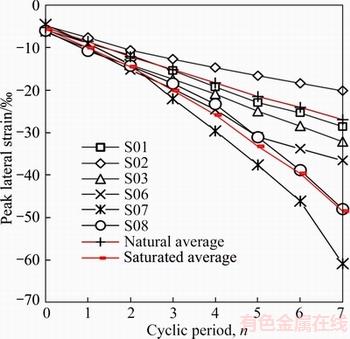
Figure 6 Relationship between peak lateral strain and cyclic period
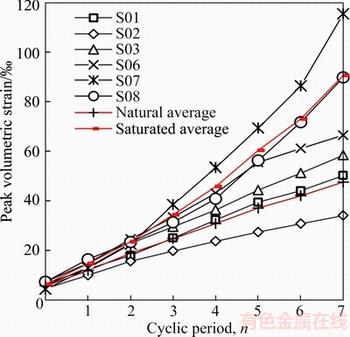
Figure 7 Relationship between peak volumetric strain and cyclic period
2) Saturation also has obvious weakening effects on the uniaxial cyclic loading and unloading strength of post-peak fracture sandstone. Under the same post-peak cyclic condition, the peak stress of cracked sandstone under the saturated state is less than that under the natural state, and the decreased magnitude of peak stress gradually increases with the increase of cyclic loading and unloading period. This means that the apparent softening coefficient of samples decreases. For example, the apparent softening coefficient falls to 0.492 after the seventh cycle, which is only 0.64 times as much as that of intact rock samples. This indicates that the cumulative damage of samples gradually rises with the increase in post-peak cyclic loading and unloading cycle. In addition, water has obvious weakening effects on strength. The main reason is that its fracture structural planes of interior under bearing state gradually increase with the cumulative damage of samples. The planes of fracture are occupied by free water, which cause water to have increasingly prominent lubricating effects on fracture surface sliding. On the macro performance, the apparent softening coefficient of samples decreases with the increase in post-peak cyclic loading and unloading period. In addition, it is not difficult to obtain the linear decreasing relationship between the apparent softening coefficient of samples and post-peak cyclic loading and unloading period by fitting the regression results shown in Figure 8.
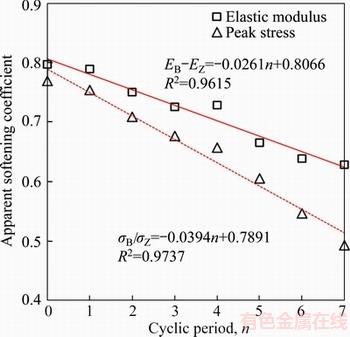
Figure 8 Relationship between peak strength or elastic modulus of weakening rate and cyclic period (Note: EB/EZ–specific value of elastic modulus between saturated samples and natural samples, σB/σZ–specific value of peak strength between saturated samples and natural samples)
3) Saturation has obvious softening effects on the elastic modulus (the approximate linear slope on the stress–strain curve), which is the deformation index of intact sandstone. Under the natural state, the elastic modulus of sandstone ranges from 6.805 to 7.680 GPa, with an average of 7.259 GPa. Under the saturated state, the elastic modulus of sandstone ranges from 5.597 to 6.036 GPa, with an average of 5.786 GPa. The apparent softening coefficient of the elastic modulus of samples (the specific value of average elastic modulus between saturated sample and natural sample) is 0.797, which shows that the weakening effects of saturation on the strength of samples are slightly greater than the softening effects of saturation on the elastic modulus of the deformation index.
4) Saturation has obvious softening effects on the elastic modulus (the approximate linear slope on each cyclic loading curve), which is the deformation index of post-peak cracked sandstone in the uniaxial cyclic loading and unloading process. Under the corresponding condition, the elastic modulus of samples under the saturated state is less than that under the natural state. Similar to the peak stress, the decreased magnitude of elastic modulus also gradually increases with the increase of cyclic loading and unloading period, which means that the apparent softening coefficient of the elastic modulus of samples decreases. This indicates that non-deformability gradually declines with the increase in cumulative damage of samples, the reason for which is the same as the cause of the decrease in apparent softening coefficient of strength; thus, it will not be restated here. In the same way, it is not difficult to obtain the linear regression relationship between the considered softening coefficient of elastic modulus of samples and post-peak cyclic loading and unloading period by fitting the regression results shown in Figure 8. Moreover, the rate of decrease (the straight regression line slope) and magnitude of elastic modulus are lower than that of peak strength, which further demonstrates that the weakening effects of saturation on the strength of samples are greater than the softening effects of saturation on the elastic modulus.
5) Saturation has incremental effects on each peak strain corresponding to intact sandstone in different degrees. Under the saturated state, the mean value of the peak (maximum) axial strain of samples increases from 4.892‰ to 4.949‰ with minimal increase in amplitude of 1.71%. The mean value of lateral strain increases from –5.260‰ to –5.633‰, with a medial increase in amplitude of 7.09%. The mean value of volumetric strain increases from 5.628% to 6.316%, with maximum increase in amplitude of 12.23%. This shows that under the condition of uniaxial compression, water has greater effects on the lateral and volumetric deformation properties of samples. The main reason is that water has lubricating effects on fracture sliding. Moreover, the lateral direction is not restrained effectively, which results in the increase in lateral deformation of samples being more obvious than that under the natural state, and finally results in obvious volume expansion in samples.
6) Each peak strain of rock samples gradually rises with the increase in post-peak cyclic loading and unloading period. However, the changes in each strain with increase in amplitude have different regularities. Under the natural state, amplification of peak axial, lateral, and volumetric strains all increase at approximately constant speed. Under the saturated state, amplification of peak axial strain increases at approximately constant speed, while the amplification of lateral and volumetric peak strains all increases significantly. In addition, under the saturated state, the amplification of the mean value of the peak axial strain is not obvious compared with that under the natural state, and does not follow monotonic function with the cyclic loading and unloading period. Amplification of mean value of the peak axial strain fluctuates between 1.17% and 3.36%, with an average of 2.31%. While amplification of the peak lateral and volumetric strains is obvious under the saturated state, the amplification of the peak lateral and volumetric strains increases continually with cyclic loading and unloading period. Up to the last (the seventh) cycle, amplification of lateral and volumetric strains reaches 79.9% and 90.41%, which is 11.282 and 7.392 times more than that of the first cycle, respectively. This indicates that under the condition without lateral effective constraint, water affects the peak axial strain, but it is not obvious with the increase in post-peak cumulative damage of rock samples; the effects of water on the peak lateral and volumetric strains are increasingly remarkable. This means that for the surrounding rocks of underground engineering affected by water, when the supporting structure is gradually destroyed and loses efficacy, the closer its bearing state is to the residual period, and the more difficult it is to control the stability of surrounding rocks. Further analysis proves that compared with the natural state, amplification of peak lateral and volumetric strains of saturated sandstone samples and the post-peak cyclic loading and unloading period all conform to the linearly increasing relationship by fitting the regression results shown in Figure 9. The incremental rate (the straight regression line slope) and the range of volumetric strain are slightly larger than that of the lateral strain.
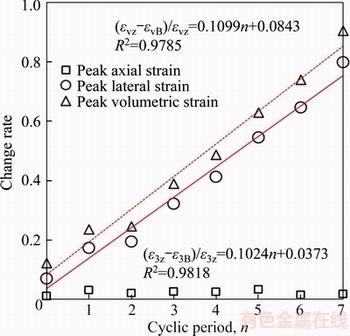
Figure 9 Relationship between change rate and cyclic period
3.3 Post-peak damage law
The peak point is the inversion point where the rock transforms from stable bearing to unstable bearing. The cumulative structural damage of rock under the unstable bearing stage gradually rises with post-peak cyclic loading and unloading period. In this part, the effects of saturation on the structural damage deterioration law of rock are discussed at the unstable bearing stage. MARTIN et al [31] adopted the cumulative value of plastic volumetric strain(εvp)to define the damage variable, which means that the irreversible volumetric strain of rock gradually increases with the increase of cyclic loading and unloading period, which indicates the degree of rock damage. In this study, the method of rock damage proposed by ZHAO et al [32] was used, which adopted the plastic shear strain of rock to define the damage factor, and its value can be obtained by
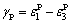 (1)
(1)
where γp is the plastic shear strain of rock, ε1p is the plastic axial strain, and ε3p is the plastic lateral strain, both of which can be obtained through the stress–strain curve of unloading of rock.
In this work, while the plastic shear strain of each rock sample was calculated under each cyclic period, the corresponding strain values when the axial stress of rock samples decreases to approximately 1 MPa were chosen. Under saturated and natural states, the relationship between post-peak damage factor (the plastic shear strain) of each rock sample and loading and unloading cycles is shown in Figure 10. The relationship between the mean values of damage factor and cyclic loading and unloading period is shown in Figure 11.
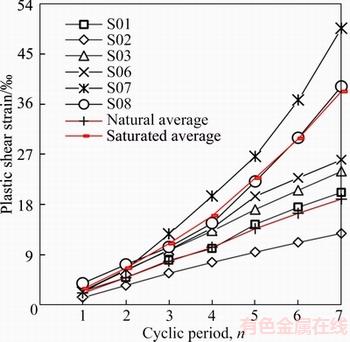
Figure 10 Variation relationship between plastic shear strain and cyclic period
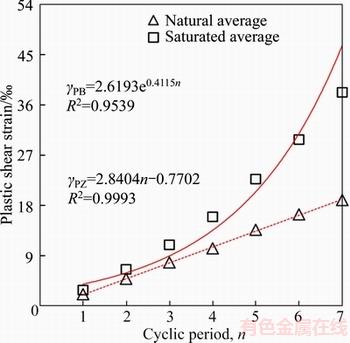
Figure 11 Variation relationship between mean value of plastic shear strain and cyclic period
From Figures 10 and 11, it can be seen that for both saturated or natural states, the damage factor (the plastic shear strain) of each rock sample gradually increases with the increase of post-peak cyclic loading and unloading period, but the increasing rates have obvious differences. Under the natural state, the damage factor of each rock sample increases at an approximately constant speed, while each rock sample increases at variable speed in the saturation. This indicates that the presence of water accelerates structural damage deterioration velocity when the rock is at the post-peak unstable bearing stage. In addition, generally speaking, the damage factor value of each saturated rock sample is greater than that of natural rock samples, and compared with the natural state, the amplitude of mean damage factor values of saturated rock samples gradually increases with the increase of cyclic loading and unloading period. The amplitude of both are the same before the third cycle, with an average increase of 37.35%. The amplitude rapidly increases from the fourth until the seventh cycle, where the increase in amplitude reaches 101.68%, which is 2.722 times greater than the average increase in amplitude of the previous three times. This indicates that the presence of water also aggravates the structural deterioration of rock at the post-peak unstable bearing stage. The main reason is because water has lubricating effects on the sliding friction within the post-peak generated plane of fracture.
Further analysis shows that under the natural state, the mean damage factor value of rock samples and the post-peak cyclic loading and unloading period conform to the linearly increasing relationship, while the mean damage factor value of saturated rock samples and the loading and unloading cycles conform to the exponential increasing relationship. The fitting regression results are shown in Figure 11.
3.4 Deterioration laws of mechanical parameters
The cumulative structural damage of rock samples rises continuously under the bearing state with the increase of post-peak cyclic loading and unloading period. Accordingly, the mechanical parameters deteriorate and attenuate continuously. The stated analysis indicates that saturation has obvious effects on the structural damage of rock samples under the bearing state. Deterioration and attenuation of rock samples are closely related to their damage, and saturation has certain effects on the deterioration and attenuation laws of rock samples.
3.4.1 Crack damage stress
The crack damage stress (σcd) is one of the key stress levels of rock in the process of compression deformation. It is also called the long-term strength [31], whose value is the corresponding stress value [32] of the volumetric strain inflection point on the stress–strain curve of rock, as shown in Figure 12.
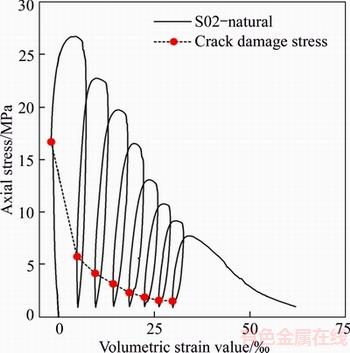
Fig. 12 Determination of crack damage stress
According to this method, the relationship between the crack damage stress (σcd) of each experimental rock sample and plastic shear strain is shown in Figure 13. The relationship between average crack damage stress and plastic shear strain under natural and saturated states is also shown in Figure 13. As shown, when the plastic shear strain is zero, the corresponding crack damage stress is equal to the axial stress that each rock sample happens fracture initially.
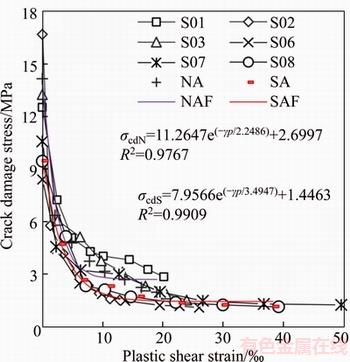
Figure 13 Relationship between crack damage stress and plastic shear strain (NA–natural average, SA–saturated average, NAF–natural average fitting line, SAF– saturated average fitting line)
From Figure 13, it is clear that under natural and saturated states, the crack damage stress of each rock sample declines rapidly at first, and then tends approximately to a constant value later with the increase in plastic shear strain. Generally, the crack damage stress value under the saturated state is less than that under the natural state. The crack damage stress of rock under the saturated state attenuates faster and reaches a constant value earlier compared with that under the natural state. The main reason is that intact rock unloads rapidly after experiencing the peak rupture, and master fracture surfaces have not been completely cut-through at this moment. Upon re-loading, fracture surfaces will continue to expand, and the stress value is bound to decline substantially. After master fracture surfaces of the rock are formed, the sliding stress of master fracture surfaces gradually tends to reach a constant value with the increase of post-peak cyclic loading and unloading period. Under the same loading and unloading conditions, because water promotes extending and slipping of fracture surfaces, crack damage stress of rock under the saturated state suffers different attenuation compared with that under the natural state. In addition, owing to the dual function of cumulative damage and water, the master fracture surfaces of rock after the peak rupture appear earlier compared with natural state in the cyclic loading and unloading process, which leads to an earlier constant value of crack damage stress.
3.4.2 Peak stress
From Figures 14 and 15, the relationship between the peak stress of each loading in post-peak cyclic loading and unloading process of each rock sample and the plastic shear strain can be summarized under natural and saturated states.
From Figures 14 and 15, it can be seen that under natural and saturated states, the peak stress of each rock sample gradually decreases with the increase of post-peak cyclic loading and unloading period and cumulative damage (the increase of plastic shear strain) of sandstone rock samples in this test. However, there are obvious differences in reduction pattern between peak stress under natural state and under saturated state.
Under the natural state, due to the damage effects, the peak stress of rock samples attenuates at constant speed with the increase in plastic shear strain. The peak stress and plastic shear strain conform to linearly decreasing relationships, which are expressed as follows:
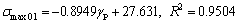 (2)
(2)
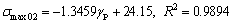 (3)
(3)
 (4)
(4)
Under the saturated state, owing to the dual function of damage and water, the peak stress rapidly attenuates with the increase in plastic shear strain. Later, the rate of attenuation gradually decreases. The peak stress and plastic shear strain conform to exponential attenuation relationship, which is shown as follows:
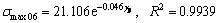 (5)
(5)
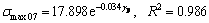 (6)
(6)
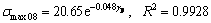 (7)
(7)
3.4.3 Elastic modulus
Under natural and saturated states, the relationship between the elastic modulus (the average slope of approximate straight line on curve) of each loading stage in the post-peak cyclic loading and unloading process of each rock sample and the plastic shear strain are shown in Figures 16 and 17.
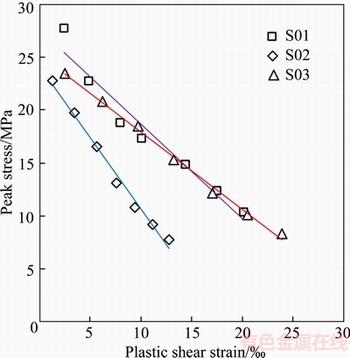
Figure 14 Relationship between peak stress of each rock sample and plastic shear strain under natural state
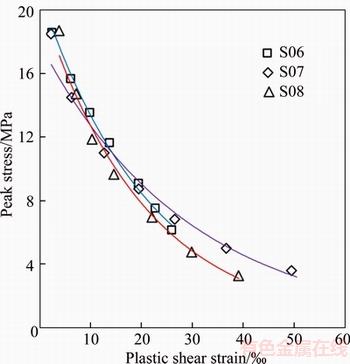
Figure 15 Relationship between peak stress of each rock sample and plastic shear strain under saturated state
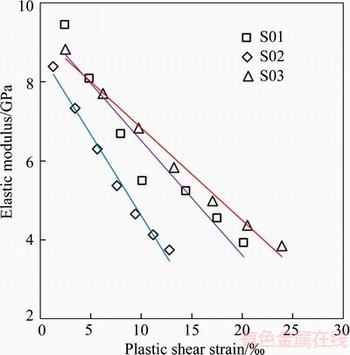
Figure 16 Relationship between elastic modulus of each rock sample at loading stage and plastic shear strain under natural state
According to Figures 16 and 17, we can see that under natural and saturated states, elastic modulus of the loading stage and peak stress of each rock sample gradually decreases in different attenuation patterns with the increase in post-peak accumulative damage of samples, and both attenuation patterns are similar.
Under the natural state, the elastic modulus of the loading stage of samples attenuates at a constant speed with the increase in plastic shear strain. The elastic modulus of the loading stage and plastic shear strain conform to linearly decreasing relationship, which is shown as follows:
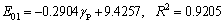 (8)
(8)
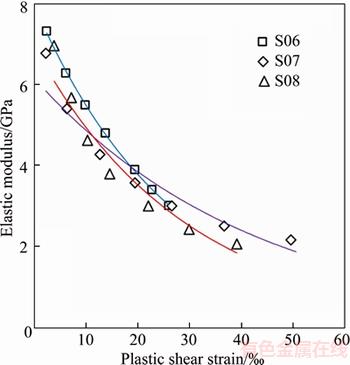
Figure 17 Relationship between elastic modulus of each rock sample at loading stage and plastic shear strain under saturated state
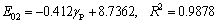 (9)
(9)
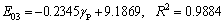 (10)
(10)
Under the saturated state, the elastic modulus of the loading stage rapidly attenuates with the increase in plastic shear strain. Later, the rate of attenuation gradually decreases. The elastic modulus of the loading stage and plastic shear strain conform to exponential attenuation relationship, which is shown as follows:
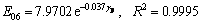 (11)
(11)
 (12)
(12)
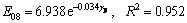 (13)
(13)
3.5 Wave velocity analysis
From Figure 18, the relationship between the longitudinal wave velocity of each loading stage in post-peak cyclic loading and unloading process of each rock sample and the plastic shear strain can be summarized under natural and saturated states. The average wave velocity and plastic shear strain conform to the exponential relationship with a constant.
From Figure 18, it can be seen that under natural and saturated states, the trends in the average longitudinal wave velocity of rock samples are roughly similar at each loading stage. The average wave velocity and plastic shear strain conform to the exponential relationship with a constant term. This indicates that water has slight effects on longitudinal wave velocity at each loading stage. When the final macro fracture surface is consistent with the testing fracture surface, the average longitudinal wave velocities at each loading stage gradually decrease with the increase in plastic shear strain. Loading and unloading periods lead to the increase in internal crack of rock samples, which causes the longitudinal wave velocity to decrease gradually. However, for S01 and S06, the wave velocity is different from the other samples at the end of cyclic loading. The reason may be that, on the one hand, because of the sample’s deformation, internal cracks on the testing fracture surface are compressed and close again under cyclic loading and unloading state, causing wave velocity increases. On the other hand, with the increase in plastic shear strain, the new fracture surface is inconsistent with the testing fracture surface or new cracks are beyond the scope of the testing fracture surface, making the number of cracks stable or even decreases in the scope of the testing fracture surface and causing wave velocity increases. Under the saturated state, the average longitudinal wave velocity of rock samples is significantly greater than that under the natural state at the same loading stage. The reason is that cracks of rock samples are filled with water under the saturated state; thus, the water replaces the air in cracks of rock samples under the natural state. Elastic wave can spread through the combination of rock particles and water medium. On the macro performance, the wave velocity increases.
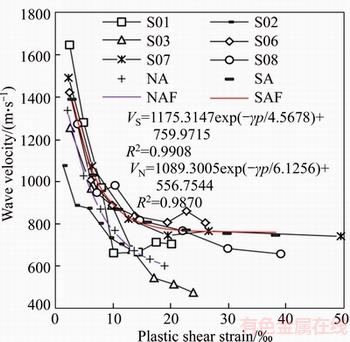
Figure 18 Relationship between wave velocity of each rock sample at loading stage and plastic shear strain under natural and saturated states
The average wave velocity changes from 1336.8 m/s under the natural state to 1389.0 m/s under the saturated state at the first loading stage, and the average relative increment is 3.97%. The average wave velocity changes from 1027.3 m/s under the natural state to 1031.3 m/s under the saturated state at the second loading stage, and the average relative increment is 0.39%. The average wave velocity changes from 869.6 m/s to 869.0 m/s at the third loading stage without average relative increment; from 769.1 m/s to 808.4 m/s with an average relative increment of 5.11% at the fourth loading stage; from 673.1 m/s to 770.9 m/s with an average relative increment of 14.53% at the fifth loading stage; from 633.0 m/s to 755.5 m/s with an average relative increment of 19.35% at the sixth loading stage; and from 601.0 m/s to 746.9 m/s at the seventh loading stage with an average relative increment of 24.28%. The effects of test error were ignored and the average relative increment of wave velocity gradually increased with the increase of loading cycles. The reason is that rock samples’ cracks develop and extend continuously.
3.6 Energy analysis
Owing to the effects of external force, rock samples deform in the uniaxial compression process. Suppose that the whole test system does not exchange heat with the outside during the process of the whole physical test. The total absorbed energy of test system is completely provided by the work of external forces. According to the first law of thermodynamics [33],
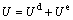 (14)
(14)
where U is the total absorption energy of rock samples, Ud is the dissipated energy of rock samples, and Ue is the releasable strain energy.
The relationship between dissipated energy Ud and releasable strain energy Ue is shown in Figure 19. Dissipated energy Ud is mainly used for damage and plastic deformation of rock samples. Releasable strain energy Ue is stored in rock samples, which is mainly formed at the elastic deformation stage. After the axial load is removed, this part of strain energy can make the deformation of rock samples achieve certain recovery. Ei is the unloading elastic modulus.
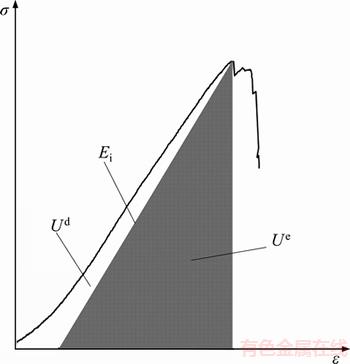
Figure 19 Relationship between dissipated energy and releasable strain energy
The axial stress called σ1 participates in the work in the process of uniaxial compression test without other stress; thus, the strain energy is expressed as follows [34]:
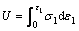 (15)
(15)
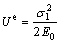 (16)
(16)
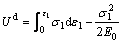 (17)
(17)
Owing to continuous loading and unloading, the phenomenon of hysteretic loop occurs in rock samples in the post-peak cyclic loading and unloading process, and each post-peak cyclic loading and unloading will generate the plastic strain. The area of the hysteretic loop represents the dissipated energy of rock samples, and the energy is mainly used for plastic deformation of rock samples.
From Figures 20–22, the relationship between total absorption, dissipated, and elastic strain energy of each rock sample and the plastic shear strain can be summarized, respectively, under natural and saturated states. The total absorption, dissipated, and elastic strain energy of each rock sample and plastic shear strain all conform to the exponential relationship with a constant.
From Figures 20–22, it is clear that under natural and saturated states, the influence of the relationship between post-peak cyclic loading and unloading of rock samples and energy features basically involves the following aspects:
1) Under natural and saturated states, the total absorption energy, dissipated energy, and elastic strain energy of rock samples all gradually decrease with the increase in plastic shear strain, which indicates that in the process of constant damage, the work of external forces gradually decreases, the damage in rock samples accumulates, and the strength continually weakens.
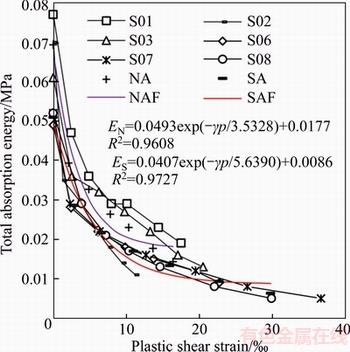
Figure 20 Relationship between total absorption energy of rock samples and plastic shear strain
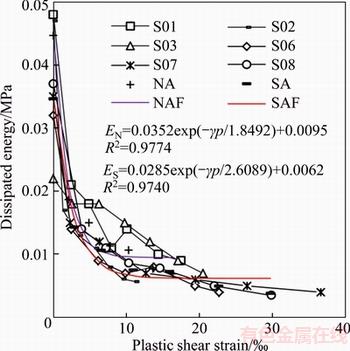
Figure 21 Relationship between dissipated energy of rock samples and plastic shear strain
2) Under the natural state, the average total absorption energy, dissipated energy, and elastic strain energy of rock samples are all greater than those under the saturated state. This indicates that the presence of water has obvious lubricating effects on the sliding friction between rock particles and master fracture surfaces of the rock, which causes rock particles and master fracture surfaces of the rock to slide and open earlier.
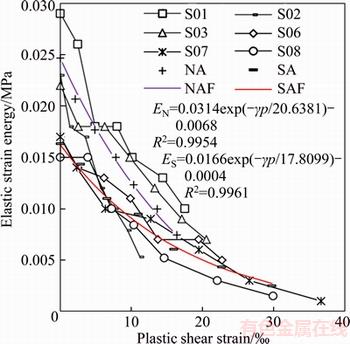
Figure 22 Relationship between elastic strain energy of rock samples and plastic shear strain (EN–average energy under natural state, ES–average energy under saturated state)
3) Under natural and saturated states, internal microcracks in rock samples continuously increase, which leads to the increase in plastic shear strain and extension of damage. Plastic shear strain of rock samples continuously accumulates with the increase of cyclic periods. When it reaches a certain value, the rock samples will lose stability.
4 Conclusions
In this work, post-peak cyclic loading and unloading tests were conducted on sandstone rock samples under natural and saturated states using the RMT-150B rock mechanics testing system to study stress–strain, strength, deformation, damage, and deterioration of mechanical properties, wave velocity, and energy property of sandstone under natural and saturated states. The following results and main conclusions are obtained.
1) Saturation has evident weakening effects on the strength of fractured sandstone under post-peak uniaxial cyclic loading and unloading. The decreased magnitude of peak stress gradually increases with the increase of cyclic loading and unloading cycles. The apparent softening coefficient of the strength and post-peak cyclic loading and unloading period conform to the linearly diminishing relationship.
2) Saturation has evident softening effects on the elastic modulus of fractured sandstone in the process of post-peak uniaxial cyclic loading and unloading. The decreased magnitude of elastic modulus gradually increases with the increase of cyclic loading and unloading cycles. The apparent softening coefficient of elastic modulus and post-peak cyclic loading and unloading period also conform to the linearly diminishing relationship, but the decreased rate (the straight regression line slope) and range of elastic modulus are less than that of peak strength.
3) With the increase of post-peak loading and unloading cycles, the amplitudes of peak axial, lateral, and volumetric strains all increased at approximately constant speed under the natural state. The amplitude of axial peak strain increased at approximately constant speed, while the amplitudes of lateral and volumetric peak strain increased significantly under the saturated state.
4) Compared with the natural state, the increase in amplitude of peak lateral and volumetric strains of saturated sandstone samples and the post-peak cyclic loading and unloading period all conform to the linearly increasing relationship. The incremental rate and magnitude of volumetric strain are slightly larger than that of the lateral strain.
5) Under saturated and natural states, the damage factor (the plastic shear strain) of each rock sample gradually increased with the increase of post-peak cyclic loading and unloading period. Under the natural state, the damage factor of each rock sample increased at approximately constant speed, while damage factor of each saturated rock sample increased at variable speed. Under the natural state, the mean damage factor value of rock samples and the post-peak cyclic loading and unloading period conformed to the linearly increasing relationship, while the mean damage factor value of rock samples in the saturation and the loading and unloading cycles conform to the exponential increasing relationship.
6) Under natural and saturated states, the crack damage stress of each rock sample attenuates rapidly at first and tends to reach a constant value later with the increase in plastic shear strain. Generally, the crack damage stress value under the saturated state is less than that under the natural state. The attenuation of rock crack damage stress under the saturated state is faster and reaches a constant value earlier compared with that under the natural state.
7) Under natural and saturated states, the wave velocities of rock samples all decrease in the process of post-peak cyclic loading and unloading with the increase in plastic shear strain. The wave velocities of rock samples and plastic shear strain conform to the exponential relationship with a constant. Moreover, the wave velocities of saturated rock samples are always greater than that of natural rock samples, which indicates that saturation promotes the spread of longitudinal wave.
8) Saturation reduces the friction coefficient between rock particles, and blocks and accelerates the damage fracture velocity of rock samples. Therefore, it reduces the total absorption energy, dissipated energy, and elastic strain energy of rock samples.
References
[1] DIERING D H. Ultra-deep level mining: Future requirements [J]. Journal of the South African Institute of Mining and Metallurgy, 1997, 97(6): 249–255.
[2] GURTUNCA R G, KEYNOTE L. Mining below 3000 m and challenges for the South African gold mining industry [C]// Proceedings Mechanics of Jointed and Fractured Rock. Rotterdam: A. A. Balkema, 1998: 3–10.
[3] DIERING D H. Tunnels under pressure in an ultra-deep Wifwatersrand gold mine [J]. Journal of the South African Institute of Mining and Metallurgy, 2000, 100(6): 319–324.
[4] VOGEL M, ANDRAST H P. Alp transit-safety in construction as a challenge, health and safety aspects in very deep tunnel construction [J]. Tunneling and Underground Space Technology, 2000, 15(4): 481–484.
[5] JOHNSON R A S. Mining at ultra-depth, evaluation of alternatives [C]// Proceedings of the 2nd North America Rock Mechanics Symposium. Montreal: NARMS’ 96, 1996: 359–366.
[6] HE Man-chao, XIE He-ping, PENG Su-ping, JIANG Yao-dong. Study on rock mechanics in deep mining engineering [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(16): 2803–2813. (in Chinese)
[7] HE Man-chao. The theory and practices of soft rock roadway support in China [M]. Beijing: China University of Mining and Technology Press, 1996. (in Chinese)
[8] ZHANG Nong, LI Xi-yong, ZHENG Xi-gui, XUE Fei. Deep mining of coal resources present situation and the technical challenges [C]// Mining technology of kilometer deep well in the national coal. Xuzhou: China University of Mining and Technology Press, 2013: 2–23. (in Chinese)
[9] HE Man-chao. Present state and perspective of rock mechanics in deep mining engineering [C]// Proceedings of the 8th Rock Mechanics and Engineering Conference. Beijing: Science Press, 2004: 88–94. (in Chinese)
[10] QIAN Qi-hu. The current development of nonlinear rock mechanics: The mechanics problems of deep rock mass [C]// Proceedings of the 8th Rock Mechanics and Engineering Conference. Beijing: Science Press, 2004: 10–17. (in Chinese)
[11] CELIK M Y, ERGIIL A. The influence of the water saturation on the strength of volcanic tuffs used as building stones [J]. Environ Earth Sci, 2015, 74(4): 3223–3239. DOI: 10.1007/s12665-015-4359-x.
[12] CHENG Jing-yi, WAN Zhi-jun, ZHANG Yi-dong, LI Wen-feng, PENG S S, ZHANG Peng. Experimental study on anisotropic strength and deformation behavior of a coal measure shale under room dried and water saturated conditions [J]. Shock and Vibration, 2015, 2015(5): 1–13. DOI: http://dx.doi.org/10.1155/2015/290293.
[13] DUDA M, RENNER J. The weakening effect of water on the brittle failure strength of sandstone [J]. Geophys J Int, 2013, 192(3): 1091–1108. DOI: 10.1093/gji/ggs090.
[14] HASHIBA K, FUKUI K. Effect of water on the deformation and failure of rock in uniaxial tension [J]. Rock Mech Rock Eng, 2015, 48(5): 1751–1761. DOI: 10.1007/s00603-014- 0674-x.
[15] SHI Xiang-chao, CAI Wu-qiang, MENG Ying-feng, LI Gao, WEN Ke, ZHANG Yun-rong. Weakening laws of rock uniaxial compressive strength with consideration of water content and rock porosity [J]. Arab J Geosci, 2016, 9(5): 369–375. DOI: 10.1007/s12517-016-2426-6.
[16] LIU Lang, WANG Ge-ming, CHEN Jian-hong, YANG Shan. Creep experiment and rheological model of deep saturated rock [J]. Transaction of Nonferrous Metals Society of China, 2013, 23(2): 478–483. DOI: 10.1016/S1003-6326(13)62488- 7.
[17] ZHAO Yi-xin, LIU Shi-min, JIANG Yao-dong, WANG Kai, HUANG Ya-qiong. Dynamic tensile strength of coal under dry and saturated conditions [J]. Rock Mech Rock Eng, 2016, 49(5): 1709–1720. DOI: 10.1007/s00603-015-0849-0.
[18] CHEN Tian, YAO Qiang-ling, WEI Fei, CHONG Zhao-hui, ZHOU Jian, WANG Chang-bin, LI Jing. Effects of water intrusion and loading rate on mechanical properties of and crack propagation in coal-rock combinations [J]. Journal of central South University, 2017, 24(2): 423–431. DOI: 10.1007/s11771-017- 3444-6.
[19] LIU Jian-feng, XIE He-ping, HOU Zheng-meng, YANG Chun-he, CHEN Liang. Damage evolution of rock salt under cyclic loading in uniaxial tests [J]. Acta Geotechnica, 2014, 9(1): 153–160. DOI: 10.1007/s11440-013-0236-5.
[20] AKESSON U, HANSSON J, STIGH J. Characterisation of microcracks in the Bohus granite, western Sweden, caused by uniaxial cyclic loading [J]. Engineering Geology, 2004, 72(1): 131–142. DOI: 10.1016/j.enggeo.2003.07.001.
[21] LIU Xue-sheng, NING Jian-guo, TAN Yun-liang, GU Qing-heng. Damage constitutive model based on energy dissipation for intact rock subjected to cyclic loading [J]. International Journal of Rock Mechanics & Mining Science, 2016, 85: 27–32. DOI: http://dx.doi.org/10.1016/j.ijrmms. 2016.03.003.
[22] ROBERTS L A, BUCHHOLZ S A, MELLEGARD K D, DUSTERLOH U. Cyclic loading effects on the creep and dilation of salt rock [J]. Rock Mech Rock Eng, 2015, 48(6): 2581–2590. DOI: 10.1007/s00603-015-0845-4.
[23] LIANG Wei-guo, ZHANG Chuan-da, GAO Hong-bo, YANG Xiao-qin, XU Su-guo, ZHAO Yang-sheng. Experiments on mechanical properties of salt rocks under cyclic loading [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2012, 4(1): 54–61. DOI: 10.3724/SP.J.1235.2012.00054.
[24] MA Lin-jian, LIU Xin-yu, WANG Ming-yang, XU Hong-fa, HUA Rui-ping, FAN Peng-xian, JIANG Shen-rong, WANG Guo-an, YI Qi-kang. Experimental investigation of the mechanical properties of rock salt under triaxial cyclic loading [J]. International Journal of Rock Mechanics & Mining Sciences, 2013, 62(9): 34–41. DOI: http://dx.doi.org/ 10.1016/j.ijrmms.2013.04.003.
[25] FUENKAJORN K, PHUEAKPHUM D. Effects of cyclic loading on mechanical properties of Maha Sarakham salt [J]. Engineering Geology, 2010, 112(1): 43–52. DOI: 10.1016/ j.enggeo.2010.01.002.
[26] LIU En-long, HUANG Run-qiu, HE Si-ming. Effects of frequency on the dynamic properties of intact rock samples subjected to cyclic loading under confining pressure conditions [J]. Rock Mech Rock Eng, 2012, 45(1): 89–102. DOI: 10.1007/s00603-011-0185-y.
[27] FATHI A, MORADIAN Z, RIVARD P, BALLIVY G. Shear mechanism of rock joints under pre-peak cyclic loading condition [J]. International Journal of Rock Mechanics & Mining Sciences, 2016, 83: 197–210. DOI: http://dx.doi.org/ 10.1016/j.ijrmms.2016.01.009.
[28] BAGDE M N, PETROS V. Fatigue properties of intact sandstone samples subjected to dynamic uniaxial cyclical loading [J]. International Journal of Rock Mechanics & Mining Sciences, 2005, 42(2): 237–250. DOI: 10.1016/ j.ijrmms. 2004.08.008.
[29] WANG Han-peng, GAO Yan-fa, LI Shu-cai. Uniaxial experiment study on mechanical properties of reinforced broken rock pre-and-post grouting [J]. Chinese Journal of Underground Space and Engineering, 2007, 3(1): 27–31, 39. (in Chinese)
[30] YANG Mi-jia, HE Yong-nian. On the strength of broken rock [J]. Chinese Journal of Rock Mechanics and Engineering, 1998, 17(4): 379–385. (in Chinese)
[31] MARTIN C D, CHANDLER N A. The progressive fracture of Lac du Bonnet granite [J]. International Journal of Rock Mechanics and Mining Sciences and Geomechanics, 1994, 31(6): 643–659.
[32] ZHAO Xing-guang, LI Peng-fei, MA Li-ke, SU Rui, WANG Ju. Damage and dilation characteristics of deep granite at Beishan under cyclic loading-unloading conditions [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(9): 1740–1748. DOI: 10.13722/j.cnki.jrme.2014.09.002. (in Chinese)
[33] XIE He-ping, JU Yang, LI Li-yun. Criteria for strength and structural failure of rocks based on energy dissipation and energy release principles [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(17): 3003–3010. (in Chinese)
[34] GUO Jia-qi, LIU Xi-liang, QIAO Chun-sheng. Experimental study of mechanical properties and energy mechanism of karst limestone under natural and saturated states [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(2): 296–308. DOI: 10.13722/j.cnki.jrme.2014.02.005. (in Chinese)
(Edited by FANG Jing-hua)
中文导读
饱水粗砂岩力学特性及能量机制
摘要:本文研究了饱水对粗砂岩峰后力学特性及能量机制的影响。为了获得这些重要的属性,采用RMT-150B型岩石力学试验系统对自然与饱水状态的粗砂岩岩样进行峰后循环加、卸载试验。在对这些试验进行成功处理后,对比研究自然与饱水状态下粗砂岩的应力–应变、强度、变形、损伤及劣化力学特性和波速、能量特性。试验结果表明:饱水对峰后破裂粗砂岩单轴循环加、卸载强度和弹性模量具有明显的弱化作用;随着峰后循环加、卸载周期的增加,自然状态下,峰值轴向、侧向和体积应变增幅均近似为等速率增加,饱水状态下,轴向峰值应变增幅近似等速率增加,而侧向、体积应变增幅均显著增加;饱水后粗砂岩试样峰值侧向应变、体积应变相对自然状态下的增幅与峰后循环加、卸载周期之间均符合线性函数递增关系;在自然与饱水状态下,各岩样的损伤因子(塑性剪切应变)随着峰后循环加、卸载周期的增加而逐渐增加,各岩样裂隙损伤应力随其塑性剪切应变的增加先期快速衰减,之后裂隙损伤应力近似趋于某一恒定值;随着峰后循环加、卸载周期的增加,岩样承载结构累计损伤程度不断提高,相应地其力学参数不断劣化衰减;自然与饱水状态岩样在峰后循环加、卸载过程中的波速均随着塑性剪切应变的增加而减小,两者之间均符合带有常数项的指数函数关系。饱水降低了岩样的总吸收能量、耗散能及弹性应变能。
关键词:峰后;饱水;强度特性;损伤机理;能量
Foundation item: Projects(51304068, 51674101, 51374112) supported by the National Natural Science Foundation of China; Project(17FTUE03) supported by the Fujian Research Center for Tunneling and Urban Underground Space Engineering (Huaqiao University), China; Project(2018M632574) supported by the Postdoctoral Science Foundation of China
Received date: 2016-12-09; Accepted date: 2017-04-12
Corresponding author: YANG Da-fang, Master, Lecturer; Tel: +86–391–3687690; E-mail: yangdafang@hpu.edu.cn

