ARTICLE

J. Cent. South Univ. (2019) 26: 2214-2223

DOI: https://doi.org/10.1007/s11771-019-4167-7

Effects analysis on catalytic combustion characteristic of hydrogen/air in micro turbine engine by fuzzy grey relation method

E Jia-qiang(鄂加强)^{1, 2}, WU Jiang-hua(吴江华)¹, LIU Teng(刘腾)^{1, 2}, CHEN Jing-wei(陈敬炜)^{1, 2},DENG Yuan-wang(邓元望)^{1, 2}, PENG Qing-guo(彭庆国)^{1, 2}

1. College of Mechanical and Vehicle Engineering, Hunan University, Changsha 410082, China;

2. Institute of New Energy and Energy-Saving & Emission-Reduction Technology, Hunan University, Changsha 410082, China

Central South University Press and Springer-Verlag GmbH Germany, part of Springer Nature 2019

Abstract: In order to enhance catalytic combustion efficiency, a premixed hydrogen /air combustion model of the micro turbine engine is established under different excess air ratio, inlet velocity and heat transfer coefficient. And effects of inlet velocity, excess air coefficient and heat transfer coefficient on the catalytic combustion efficiency of the hydrogen have been analyzed by the FLUENT with CHEMKIN reaction mechanisms and the fuzzy grey relation theory. It is showed that inlet velocity has a more intuitive influence on the catalytic combustion efficiency of the hydrogen. A higher efficiency can be obtained with a lower inlet velocity. The optimum excess air coefficient is in the range of 0.94 to 1.0, the catalytic combustion efficiency of the hydrogen will be declined if the excess air coefficient exceeded 1.0. The effect of heat transfer coefficient on the catalytic combustion efficiency of the hydrogen mainly embodies in the case of the excess air coefficient exceeded 1.0, however, the effect will be declined if the heat transfer coefficient exceeded 4.0. The fuzzy grey relation degrees of the inlet velocity, heat transfer coefficient and excess air coefficient on the catalytic combustion efficiency of the hydrogen are 0.640945, 0.633214 and 0.547892 respectively.

Key words: micro turbine engine; catalytic combustion; hydrogen; fuzzy grey relation theory

Cite this article as: E Jia-qiang, WU Jiang-hua, LIU Teng, CHEN Jing-wei, DENG Yuan-wang, PENG Qing-guo. Effects analysis on catalytic combustion characteristic of hydrogen/air in the micro turbine engine by fuzzy grey relation method [J]. Journal of Central South University, 2019, 26(8): 2214-2223. DOI: https://doi.org/ 10.1007/s11771-019-4167-7.

1 Introduction

As for the more and more serious energy crisis [1-4] and emission issues [5-9], the Power Micro Electro Mechanical Systems(MEMS)[10-12] is one of the clean combustion and low pollution emission technologies. Micro combustion technology has got wide attention of scholars over the world because of its advantages. The micro turbine engine is one of the Power MEMS, whose combustor is the most important part of the overall system.

As for the micro turbine engine, the area to volume ratio(A/V) will increase, the combustion time will be shortened, and the feature size of the micro turbine engine will be less than the flame quenching distance, etc. Therefore, the high heat loss, instability combustion and incomplete combustion [13-15] will be caused. These problems are directly impact on the overall performance and the practical application of the micro turbine engine. Therefore, the technology of catalytic combustion will be useful for solving above problems due to its advantages of fixed combustion area, low light-off temperature and the applicable surface reactions, and so on [16-18].

There are many researches about the microscale catalytic combustion. ZUO et al [19] firstly developed a micro gas turbine based on Power MEMS in older to analyze the catalytic combustion characteristics, energy density and combustion efficiency of micro combustor of a turbine, which was made by three-layer silicon wafer, and used H₂/air as the fuel. Later, JIANG et al [20-22] investigated thermal performance of and combustion characteristics of a meso-scale combustor fueled by biodiesel-ethanol blends, the convincing results are good for the development of the meso-scale combustor. BOIES et al [23] investigated the emission characteristics of a gas turbine with a double annular combustor, and it was useful for setting aggregate mobility diameter and engine thrust. ZHANG et al [24] and CHEN et al [25] analyzed the catalytic combustion of the methane/air in a micro combustor with counterflow heat exchanger; he results revealed that counterflow heat exchanger and catalyst can lead to stable and efficient micro combustion of methane. WAN et al [26] investigated the catalytic combustion characteristic of hydrogen in a micro tube by numerical simulation, and found that the surface catalytic reaction has a certain inhibitory to the space gas phase reaction. PERSSON et al [27] held that the catalytic combustion of the methane was a main surface reaction rather than a space gas phase reaction in the micro combustor. WANG et al [28] researched the catalytic combustion of H₂/air by platinum catalyst in a ceramic microscale straight tube whose diameter was 2 mm, and got the stable limits of micro combustion in different conditions. JIANG et al [29] analyzed the entropy generation, the thermodynamic irreversibility, and the effects of flow velocity and fuel equivalence ratio of H₂/air premixed flame in a micro combustor with heat recuperation.

The above researches indicate that the fuel cannot maintain normally stable combustion in the micro turbine engine due to the increasing of A/V ratio and heat loss caused the failure of the flame propagation when the combustion space shrinking to a certain size. As for the micro turbine engine, some special structures and catalytic materials can be used to make the fuel combustion on the rails. Meanwhile, the above researches show that using methane or hydrogen as fuel cannot reach the ideal combustion effect, and the main factors effects on combustion are hard to be uncovered. There were few reports on the influencing degree of various factors on the catalytic combustion performance by combining fuzzy theory and grey relevance analysis[30, 31]. In this work, the catalytic combustion characteristic of the premixed hydrogen/air in the micro turbine engine will be investigated and fuzzy grey relational analysis is used to deal with the relationship between the combustion efficient and three factors (Namely inlet velocity, excess air coefficient and heat transfer coefficient) on catalytic combustion to enhance catalytic combustion efficiency of the hydrogen/air in the micro turbine engine. The results will provide some certain theory basis to the development of the micro turbine engine.

2 Methods

2.1 Grid generation

The micro turbine engine model of the present paper is shown in Figure 1; its diameter is 20 mm and height is 4 mm. The micro turbine engine consists of three-layer silicon wafer, of which physical properties are shown in Table 1. The premixed hydrogen/air with a certain velocity will be flowed into combustor through the inlet, and burned on the surface of Pt-catalyst coating. The yellow area in Figure 1 is Pt-catalyst coating. The premixed hydrogen/air will be started flameless catalytic combustion on the surface of Pt-catalyst coating, and then discharged from the outlet.

Figure 1 x-y sectional view of micro turbine engine

In order to make the established grid model of combustion chamber in micro turbine engine have a better computing performance and convergence properties, take the following measurements while meshing: 1) To mesh easily, tetrahedral mesh is used; 2) To ensure micro-sized structures of the model no distortion, the feature angle is set to 30° and the minimum dimensions of the grid are set to 1×10^-5, 2×10^-5 and 5×10^-5 m; 3) To control the number of cells, the maximum dimensions of the grid are set to 1×10^-5, 2×10^-5 and 5×10^-5 m.

Table 1 Physical properties of silicon wafer

Table 2 shows that these three mesh intervals only have slight differences in temperature and hydrogen at the outlet. To ensure accuracy and save computation time, the grid spacing of 2×10^-5 m is used in the numerical simulation. The eventually established grid model of combustion chamber in micro turbine engine is shown in Figure 2, whose cell number is 1546, 232 and node number is 309, 008.

Table 2 Calculation results of different grid spacing

Figure 2 Grid model of combustion chamber in micro turbine engine

2.2 Governing equation

In order to investigate the catalytic combustion characteristic, the equations are employed as follows.

Continuity equation:

                          (1)

where ρ is the gas density, kg/m³; t is the time, s; u_i (i=x, y, z) are velocity components of v_x, v_y and v_z directions of the fuel gas, m/s.

The momentum equation:

 (2)

where u_i (i=x, y, z and i≠j) are velocity of the fuel gas at x_i (i=x, y, z, and i≠j) directions, m/s; u_j (j=x, y, z and i≠j) is velocity components of the fuel gas at x_j (j=x, y, z and i≠j) directions, m/s; p is absolute pressure of fuel gas, Pa; μ is dynamic viscosity of fuel gas, Pa·s.

Component equation:

     (3)

where k=1, 2, 3 is H₂, O₂, H₂O respectively; ρ_k is the density of the kth component, kg/m³; Y_k is the quality ingredient of the kth component; D_k is the diffusion coefficient of the kth component, m²/s; R_k is the generation and consumption rate of the kth component, s^-1.

Energy equation:

    (4)

where h is gas enthalpy, J/kg; T is the thermodynamic temperature of the gas, K; h_k is the enthalpy of the kth component, J/kg; q is reaction heat effect, J/(K·m³).

The ideal gas state equation:

                          (5)

where R₀ is general gas constant, R₀=8.314 J/(mol·K); M_k is he molar mass of the kth component.

For the formation and consumption rate R_k of the kth component, R_k is equal to 0 at each point in space and the R_k on the catalytic surface is determined by Eq.(6).

               (6)

where Y_k is the kth component mass fraction on catalytic surface; ρ_w is the density of the catalytic surface area, kg/m³; u_wn is normal component of gas flow velocity on the catalytic surface, m/s.

The generation and consumption rate R_k of the kth components can be determined by Eq. (7):

                   (7)

where γ_k is the concentration of the adsorbed kth component by surface, mol/m²; N_k is the number of the elementary reaction on the surface; N_g is fraction; χ_rk, χ_jr are the chemical equivalent coefficients; k_r is the reaction rate constants of the rth reaction, and it is determined by Eq. (8).

      (8)

where A_r is a pre-exponential factor; β_r is temperature index; E_r is the reaction activation energy; Θ is a surface coverage of the kth component; α and β are the coverage parameters.

2.3 Mechanism of gas phase catalytic reaction

When the mixed hydrogen/air is burned in micro burner, in order to obtain a reliable calculation results, the detailed chemical kinetic process must be considered. For this reason, this paper adopts the hydrogen catalytic reaction put forward by DEUTSCHMANN et al [32] that the mechanism of the gas has 19 reversible reactions and all the reactions involve components including H₂, O₂, H, HO₂, OH, O, H₂O, H₂O₂ and catalyzer M. According to the actual situation, the compiled CHEMKIN Mechanism file is imported into computational fluid dynamics software for calculation.

2.4 Simulation conditions

By using commercial CFD software FLUENT Release 6.3.26, the combustion of the combustion chamber in micro turbine engine is simulated under different conditions of excess air ratios, inlet temperature and cooling. For simplifying the calculation model, hydrogen/air one step reaction is used. Three-dimensional steady implicit solver based on pressure is used. Coupled algorithm which is convenient to solve the coupling between pressure and velocity is employed to discretize the governing equations. Density relaxation factor, volume force relaxation factor, specie relaxation factor and energy relaxation factor are set as 1. Momentum equation, energy equation and specie equation are set as the second order accuracy upwind. The residuals for continuity, momentum and species are set as 1×10^-3 for the criteria of convergence. 1×10^-6 is set as the energy convergence criterion.

The density of the mixture is calculated according to the incompressible ideal gas law. The specific heat is calculated according to the law of mixing. The dynamic viscosity coefficient and thermal conductivity are calculated by mass weighted average of all species. The mass diffusion rate is calculated by kinetic theory. The specific heat of specie is calculated by piecewise polynomial based on temperature. The thermal conductivity and dynamic viscosity coefficient is calculated by kinetic theory.

1) Initial conditions

Global initialization is used as follows: the initial pressure is 1.0×10⁴ Pa, the initial velocity components in x, y, z directions are 0 m/s, the initial mass fractions of hydrogen and oxygen change with the excess air ratio, the initial mass fraction of water is 0, and the initial temperature is consistent with inlet temperature.

2) Boundary conditions

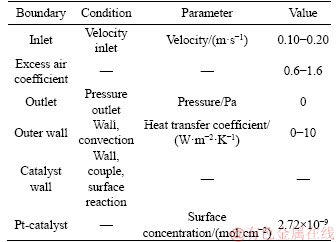
The mass flow rate boundary condition is used in the inlet, where the gauge pressure is 0.1 MPa. The outflow boundary condition is used in the outlet. No-slip and no normal specie diffusion boundary conditions are used in the wall. The key numerical simulation parameters are shown in Table 3.

Table 3 Key numerical simulation parameters of micro turbine engine

2.5 Model verification

To validate the accuracy of our numerical solution, the predicted and measured exhaust blends temperature in the micro turbine engine as reported in Ref. [33] were compared, as shown in Figure 3. The maximum temperature difference was 98 K, thereby indicating the reasonable accuracy of the proposed numerical solution.

Figure 3 Comparison between experimental values and numerical values for exhaust gas temperature in micro turbine engine

It can be observed that the experimental values and numerical values for exhaust gas temperature in the micro turbine engine have the same changing trend. The large difference between the experimental values and numerical values for exhaust gas temperature can not be ignored. The difference can be due to the errors of experimental equipments and operation and the assumptions for establishing the mathematical model. The difference is acceptable and bearable due to the fact that the maximum relative error is 7.20% and the relative error exceeding 5% only exists in a narrow range that excess air coefficient is about 1.6. As a result, the numerical model is reliable to investigate the catalytic combustion characteristic of hydrogen/air in the micro turbine engine.

3 Results and discussion

3.1 Analysis of specific combustion condition

The catalytic combustion of hydrogen in the micro turbine engine is investigated when the excess air coefficient α is 1.0, the heat transfer coefficient h is 0 W/(m²·K), the inlet velocity v are 0.10, 0.12, 0.14, 0.16, 0.18 and 0.20 m/s, respectively.

Distribution of hydrogen mass fraction in the micro turbine engine is shown in Figure 4. From Figure 4, it can be seen that there is the most intense catalytic reaction for the hydrogen near the entrance of micro combustor, where the consumption of Pt-catalyst is the highest, no matter how much the inlet velocity is. Then, the mass fraction of hydrogen decreases with the deepening of fuel gas, so the reaction rate became more and more slowly. When the inlet velocity is 0.10 m/s, majority of hydrogen would be burned completely in the place of approximately radius of the micro turbine engine. And when the inlet velocity is 0.20 m/s, the area of hydrogen catalytic combustion would reach the exit of combustor. It is said that a lower inlet velocity could be conducive to the conversion of hydrogen, but lower velocity will also decrease the power of the overall system; therefore, the reasonable choice of inlet velocity and system power will be particularly important in the design of the micro turbine engine.

Figure 4 Distribution of hydrogen mass fraction in micro turbine engine:

3.2 Combustion efficiency analysis

The effects of the inlet velocity v, excess air coefficient α and heat transfer coefficient h on the combustion efficiency η are showed in this section. The combustion efficiency η could be expressed by the following equation.

                      (9)

where w_in(gas) is the hydrogen mass fraction of inlet; w_out(gas) is the hydrogen mass fraction of outlet.

Figure 5 shows the change of hydrogen combustion efficiency for different inlet velocities v and excess air coefficient s when the heat transfer coefficient h is 0 W/(m²·K). Firstly, if the heat transfer coefficient is a constant, the hydrogen combustion efficiency will decrease as inlet velocity increases, because hydrogen could get sufficient contact with the catalyst coating by a slow flow velocity. Secondly, the combustion efficiency η can reach its maximum if the excess air coefficient α is in the range of 0.94 to 1.0.Normally, because of the different combustion conditions, the excess air coefficient α for the best heat transfer efficiency generally does not equal 1.0. Lastly, the oxygen content of fuel gas will inadequate, if the excess air coefficient α is less than 1.0, the hydrogen can’t combust completely.

Figure 5 Hydrogen combustion efficiency for different excess air coefficients and inlet velocities (1: v=0.10 m/s; 2: v=0.12 m/s; 3: v=0.14 m/s; 4: v=0.16 m/s; 5: v=0.18 m/s; 6: v=0.20 m/s)

Therefore, the hydrogen combustion efficiency will increase as the excess air coefficient increases until α reaches 1.0. After that, if the excess air coefficient α has exceeded 1.0, the excess air, which wouldn’t be participated in the reaction, could flow into the micro combustor and prevent mixed gas of hydrogen and oxygen from contacting with the Pt-catalyst coating. Meanwhile, the excess air can take away heat from reaction, and then be exhausted from the outlet. It has very bad influence on the hydrogen combustion efficiency. So the curve of h will decline if α is more than 1.0.

Figure 6 shows the change of hydrogen combustion efficiency for different heat transfer coefficients and the excess air coefficients when inlet velocity v is 0.20 m/s. As can be seen in the figure, firstly, if α is less than 1.0, the change of η will not be obvious whether h equals any value. It is said that the heat transfer coefficient has a little effect on the hydrogen combustion efficiency in this condition; if α is more than 1.0, the η will decrease with the increasing of h, but the gradient of decreasing will reduce rapidly. Meanwhile, the change of η will be not obvious if h is more than 6 W/(m²·K). Secondly, if the excess air coefficient α is in the range of 0.94 to 1.0, the hydrogen combustion efficiency η can reach its peak. Lastly, hydrogen combustion efficiency η will increase with the increasing of α if it is less than 1.0, and η will decrease with the increasing of α if it is more than 1.0.

Figure 6 Hydrogen combustion efficiency for different excess air coefficients and heat transfer coefficients (1: h=0.10 W/(m²·K); 2: h=0.12 W/(m²·K); 3: h=0.14 W/(m²·K); 4: h=0.16 W/(m²·K); 5: h=0.18 W/(m²·K); 6: h=0.20 W/(m²·K))

3.3 Fuzzy grey relation analysis

To understand the effects of inlet velocity v, excess air coefficient α and heat transfer coefficient h on the combustion efficiency η, the data in Table 4 were analyzed by fuzzy grey relation (FGR) theory. FGR is a multivariate analysis method for analyzing the intimacy degree of research objects by mathematics. The method doesn’t require lots of samples, besides the typical distribution of samples. And the calculation results have a good alignment with the results of qualitative analysis. Therefore, FGR can be widely used in various fields of social science and natural science, and have achieved good results [34-37].

Table 4 Combustion efficiency with different inlet velocity, excess air coefficient and heat transfer coefficient

Firstly, a matrix A can be established from Table 4:

(10)

where Y is the eigenvector matrix of combustion efficiency η; X is the eigenvector matrix of inlet velocity v, excess air coefficient α and heat transfer coefficient h.

The following equation has been used to transfer matrix A to dimensionless matrix A′.

                (11)

where a_m(n) is the element of row m and column n of the matrix A.

 (12)

The fuzzy grey relation degree r between hydrogen combustion efficiency and other three factors can be solved by the following equation [30].

 (13)

where y(n) is the element of first column n of matrix A′; x_m(n) is the element of row m+1 and column n of matrix A′; Δ_min is the minimum absolute difference between the dimensionless eigenvector Y′ and the corresponding value of the dimensionless eigenvector X′ and it can be defined as Δ_min=min|y_m(n)- x_m(n)|; Δ_max is the maximum absolute difference, which can be defined as Δ_max=max|y_m(n)-x_m(n)|, indicating the integrity of the researched system; Δ_m(n) is the absolute difference between the element of column n of the dimensionless eigenvector Y′ and the element of row m and column n of the dimensionless eigenvector X′ and it can be defined as Δ_m(n)= |y_m(n)-x_m(n)|; ρ is the resolution coefficient, representing the weight value of the maximum absolute difference, and indicating the correlation between research objects, and it can be confirmed by following ways:

1) Calculate the mean absolute difference by the following equation:

               (14)

2) Based on the ratio of the mean absolute difference and the maximum absolute difference, the resolution coefficient ρ can be confirmed. Assume that E_Δ equalsthe resolution coefficient ρ will in the range of E_Δ to 2E_Δ. In addition, the resolution coefficient ρ must accord with the following terms: if Δ_max is bigger than ρ will in the range of E_Δ to 1.5 E_Δ, which is defined that the sample is abnormal; if Δ_max is less than ρ will be in the range of 1.5E_Δ to 2.0 E_Δ, which is defined that the sample is normal.

The value of mean absolute difference =0.612708 can be solved by substituting Eq. (13) into matrix A′. So, E_Δ=/Δ_max=0.612708. In this condition, Δ_max is less than 3, so the sample is normal. It can be solved that ρ is in the range of 0.919062 to 1.225416. Then assuming that ρ is 1.0, the Eq. (13) can be transfer to Eq. (15).

   (15)

The fuzzy grey relation degree between the hydrogen catalytic combustion efficiency and other influence factors can be solved by substituting Eq. (15) into matrix A′. The results are listed in Table 5.

Table 5 Fuzzy grey relation degree, r between hydrogen catalytic combustion efficiency and other influence factors

From Table 5, the FGR degree of the inlet velocity v is the maximum, 0.640945, followed by excess air coefficient α and heat transfer coefficient h. It is indicated as follows: 1) The effect of the inlet velocity on the catalytic combustion efficiency of the hydrogen is the most, and the lower inlet velocity of the hydrogen/air can promote more complete hydrogen combustion on the surface of Pt catalytic layer, and the better combustion efficiency can be obtained by controlling the inlet velocity of the hydrogen/air. 2) The effect of heat transfer coefficient from the wall is the second, and the heat transfer coefficient will have more influence on the catalytic combustion efficiency only if the excess air coefficient is more than 1.0 and the heat transfer coefficient is more than 0 W/(m²·K). 3) The effect of air coefficient on the catalytic combustion is the weakest among three factors, and the excess air coefficient will have more influence on the catalytic combustion efficiency when the inlet velocity of the hydrogen/air is more than 0.1 m/s, the excess air coefficient is more than 1.0 and the heat transfer coefficient is more than 0 W/(m²·K).

4 Conclusions

1) A lower inlet velocity of gas is conducive to the catalytic combustion efficiency of the hydrogen, but it may lead to the decreasing of overall system power. Therefore, a reasonable inlet velocity of the hydrogen/air is useful for the enhancement of the catalytic combustion efficiency of the hydrogen.

2) The catalytic combustion efficiency of the hydrogen can reach its peak if the excess air coefficient is in the range of 0.94 to 1.0, and the catalytic combustion efficiency of the hydrogen will decrease if the excess air coefficient is more than 1.0, the phenomenon will be more significant if the inlet velocity of the hydrogen/air is increased.

3) The heat transfer coefficient has a little influence on the catalytic combustion efficiency of the hydrogen when the excess air coefficient is less than 1.0. If the excess air coefficient has exceeded 1.0. The efficiency will decrease as the increasing of heat transfer coefficient, but the gradient value will reduce rapidly. If the heat transfer coefficient is more than 4 W/(m²·K), it will have little influence on the catalytic combustion efficiency of the hydrogen.

4) Based on the fuzzy grey relation theory, the influence of three factors on the catalytic combustion efficiency of the hydrogen is ranked as inlet velocity, heat transfer coefficient and excess air coefficient, and the effect of inlet velocity on the catalytic combustion efficiency of the hydrogen is more visible and the other two factors will have significant influence on the catalytic combustion efficiency of the hydrogen only in a special range.

References

[1] ZUO H, LIU G, E J Q, ZUO W, WEI K, HU W, TAN J, ZHONG D. Catastrophic analysis on the stability of a large dish solar thermal power generation system with wind- induced vibration [J]. Solar Energy, 2019, 183: 40-49. DOI: https://doi.org/10.1016/j.solener.2019.03.003.

[2] CHEN J, FAN Y, E J Q, CAO W, ZHANG F, GONG J, LIU G, XU W. Effects analysis on the reaction kinetic characteristics of the food waste gasification by supercritical water [J]. Fuel, 2019, 241: 94-104. DOI: https://doi.org/ 10.1016/j.fuel.2018.12.012.

[3] CHEN J, XU W, ZUO H, WU X, E J Q, WANG T, ZHANG F, LU N. System development and environmental performance analysis of a solar-driven supercritical water gasification pilot plant for hydrogen production using life cycle assessment approach [J]. Energy Conversion and Management, 2019, 184: 60-73. DOI: https://doi.org/ 10.1016/j.enconman.2019.01.041.

[4] LI Y, TANG W, CHEN Y, LIU J, LEE C F. Potential of acetone-butanol-ethanol (ABE) as a biofuel [J]. Fuel 2019; 242: 673-686. DOI: https://doi.org/10.1016/j.fuel.2019. 01.063.

[5] E J Q, LIU G., ZHANG Z, HAN D, CHEN J, WEI K, GONG J, YIN Z. Effect analysis on cold starting performance enhancement of a diesel engine fueled with biodiesel fuel based on an improved thermodynamic model [J]. Applied Energy, 2019, 243: 321-335. DOI: https://doi.org/10.1016/ j.apenergy.2019.03.204.

[6] E J Q, ZHAO X, QIU L, ZHANG Z, WEI K, HAN D, DENG Y. Experimental investigation on performance and economy characteristics of a diesel engine with variable nozzle turbocharger and its application in urban bus [J]. Energy Conversion and Management, 2019, 193: 149-161. DOI: https://doi.org/10.1016/j.enconman.2019.04.062.

[7] WU G, LU Z, XU X, PAN W, WU W, LI J, CI J. Numerical investigation of aeroacoustics damping performance of a Helmholtz resonator: Effects of geometry, grazing and bias flow [J]. Aerospace Science and Technology 2019; 86: 191-203. DOI: https://doi.org/10.1016/j.ast.2019.01.007.

[8] WU G, LU Z, PAN W, GUAN Y, LI S, JI C. Experimental demonstration of mitigating self-excited combustion oscillations using an electrical heater [J]. Applied Energy, 2019, 239: 331-342. DOI: https://doi.org/10.1016/j.apenergy. 2019.01.133.

[9] ZUO Q, ZHU X, LIU Z, ZHANG J, WU G, LI Y. Prediction of the performance and emissions of a spark ignition engine fueled with butanol-gasoline blends based on support vector regression [J]. Environmental Progress & Sustainable Energy 2019, 38(3): e13042. DOI: https://doi.org/10.1002/ep. 13042.

[10] ZHANG Y, PAN J, TANG A, LU Q, ZHA Z, BANI S. Effects of inlet parameters on combustion characteristics of premixed CH4/Air in micro channels [J]. Journal of the Energy Institute, 2019, 92: 824-834. DOI: https://doi.org/ 10.1016/j.joei.2018.02.002.

[11] LUO Y L, GAN Y H, JIANG Z W. An improved reaction mechanism for predicting the charged species in ethanol-air flame [J]. Fuel, 2018, 228: 74-80. DOI: https://doi.org/ 10.1016/j.fuel.2018.04.157.

[12] GAN Y H, CHEN X W, TONG Y, ZHANG X, ZHANG Y. Thermal performance of a meso-scale combustor with electrospray technique using liquid ethanol as fuel [J]. Applied Thermal Engineering, 2018, 128: 274-281. DOI: https://doi.org/10.1016/j.applthermaleng.2017.09.016.

[13] YAN Y, WU G., HUANG W, ZHANG L, LI L, YANG Z. Numerical comparison study of methane catalytic combustion characteristic between newly proposed opposed counter-flow micro-combustor and the conventional ones [J]. Energy, 2019, 170: 403-410. DOI: https://doi.org/10.1016/ j.energy.2018.12.114.

[14] PENG Q, WU Y, E J Q, YANG W, XU H, LI Z. Combustion characteristics and thermal performance of premixed hydrogen/air in a two-rearward-step micro tube [J]. Applied Energy, 2019, 242: 424-438. DOI: https://doi.org/10.1016/ j.apenergy.2019.03.137.

[15] SHI B, YU H, ZHANG J. The effects of the various factors and the engine size on micro internal combustion swing engine (MICSE) [J]. Applied Thermal Engineering, 2018, 144: 262-268. DOI: https://doi.org/10.1016/j.applthermaleng. 2018.08.067.

[16] E J Q, HUANG H J, ZHAO X H. Numerical investigation on effects of bluff body in flat platemicro thermo photovoltaic combustor withsudden expansion [J]. Journal of Central South University, 2016, 23(4): 975-982. DOI: https://doi. org/10.1007/s11771-016-3145-6.

[17] ZHA Z, PAN J, YANG W, LU Q, STEPHEN B, SHAO X. Experimental investigation on the combustion characteristics of premixed CH4/O2 flame in a micro plate channel [J]. Energy Procedia, 2017, 105: 4814-4821. DOI: https://doi. org/10.1016/j.egypro.2017.03.954.

[18] PAN J, ZHU J, LIU Q, ZHU Y, TANG A, LU Q. Effect of micro-pin-fin arrays on the heat transfer and combustion characteristics in the micro-combustor [J]. International Journal of Hydrogen Energy, 2017, 42: 23207-23217. DOI: https://doi.org/10.1016/j.ijhydene.2017.06.164.

[19] ZUO W, E J Q, HU W, JIN Y, HAN D. Numerical investigations on combustion characteristics of H2/air premixed combustion in a micro elliptical tube combustor [J]. Energy, 2017, 126: 1-12. DOI: https://doi.org/10.1016/ j.energy.2017.03.011.

[20] JIANG Z W, GAN Y H, JU Y G, LIANG J L, ZHOU Y. Experimental study on the electrospray and combustion characteristics of biodiesel-ethanol blends in a meso-scale combustor [J]. Energy, 2019, 179: 843-849. DOI: https:// doi.org/10.1016/j.energy.2019.05.024.

[21] GAN Y H, LI H G, JIANG Z W, CHEN X W, LUO Y L, TONG Y, SHI Y L, JIANG X. An experimental investigation on the electrospray characteristics in a meso-scale system at different modes [J]. Experimental Thermal and Fluid Science, 2019, 106: 130-137. DOI: https://doi.org/10.1016/ j.expthermflusci.2019.04.029.

[22] GAN Y H, TONG Y, JIANG Z W, CHEN X W, LI H G, JIANG X. Electro-spraying and catalytic combustion characteristics of ethanol in meso-scale combustors with steel and platinum meshes [J]. Energy Conversion and Management, 2018, 164: 410-416.

[23] BOIES M, STETTLER E J, SWANSON J. Particle Particle Emission Characteristics of a Gas Turbine with a Double Annular Combustor [J]. Aerosol Science and Technology, 2015, 49: 842–855. DOI: https://doi.org/10.1080/02786826. 2015.1078452.

[24] ZHANG L, ZHU J, YAN Y, GUO H, YANG Z. Numerical investigation on the combustion characteristics of methane/air in a micro-combustor with a hollow hemispherical bluff body [J]. Energy Conversion and Management, 2015, 94: 293–299. DOI: https://doi.org/ 10.1016/j.enconman.2015.01.014.

[25] CHEN J, SONG W, GAO X, XU D. Hetero-/homogeneous combustion and flame stability of fuel-lean propane–air mixtures over platinum in catalytic micro-combustors [J]. Applied Thermal Engineering, 2016, 100: 932–943. DOI: https://doi.org/10.1016/j.applthermaleng.2016.02.122.

[26] WAN J, FAN A, MARUTA K, YAO H, LIU W. Experimental and numerical investigation on combustion characteristics of premixed hydrogen/air flame in a micro-combustor with a bluff body [J]. International Journal of Hydrogen energy, 2012, 37: 19190-19197. DOI: https://doi.org/10.1016/ j.ijhydene.2012.09.154.

[27] PERSSON K, PFEFFERLE L, SCHWARTZ W, ERSSON A. Stability of palladium-based catalysts during catalytic combustion of methane: The influence of water [J]. Applied Catalysis B: Environmental, 2007, 74(3, 4): 242–250. DOI: https://doi.org/10.1016/j.apcatb.2007.02.015.

[28] WANG Y, ZHOU Z J, YANG W J, ZHOU J H, LIU J Z, WANG Z H, CEN K F. Combustion of hydrogen-air in micro combustors with catalytic Pt layer [J]. Energy Conversion and Management, 2010, 51(6): 1127-1133. DOI: https:// doi.org/10.1016/j.enconman.2009.12.021.

[29] JIANG D, YANG W M, CHUA K J. Entropy generation analysis of H2/air premixed flame in microcombustion with heat recuperation [J]. Chemical Engineering Science, 2013, 98: 265-272.

[30] CAI H, LIU Y F, GONG J K, E J Q, GENG Y H, YU L P. Sulfur poisoning mechanism of three way catalytic converter and its grey relational analysis [J]. Journal of Central South University, 2014, 21(11): 4091-4096. DOI: https://doi.org/10. 1007/s11771-014-2402-9.

[31] E J Q, LI Y Q, GONG J K. Function chain neural network prediction on heat transfer performance of oscillating heat pipe based on grey relational analysis [J]. Journal of Central South University of Technology, 2011, 18(5): 1733-1737. DOI: https://doi.org/10.1007/s11771-011-0895-z.

[32] DEUTSCHMANN O, SCHMIDT R, BEHRENDT F. Numerical modeling of catalytic ignition [J]. Symposium (International) on Combustion, 1996: 26(1): 1747-1754. DOI: https://doi.org/10.1016/S0082-0784(96)80400-0.

[33] XU J, HU J, CAO H. Development and performance test of a micro gas turbine power generation system [J]. China Mechanical Engineering, 2008, 19(12): 1399-1405. DOI: https://doi.org/10.3321/j.issn:1004-132X.2008.12.003. (in Chinese)

[34] ZUO W, E J Q, LIU X, PENG Q, DENG Y, ZHU H. Orthogonal experimental design and fuzzy grey relational analysis for emitter efficiency of the micro-cylindrical combustor with a step [J]. Applied Thermal Engineering, 2016, 103: 945-951. DOI: https://doi.org/10.1016/ j.applthermaleng.2016.04.148.

[35] ZHANG B, E J Q, GONG J, YUAN W, ZHAO X, HU W. Influence of structural and operating factors on performance degradation of the diesel particulate filter based on composite regeneration [J]. Applied Thermal Engineering, 2017, 121: 838-852. DOI: https://doi.org/10.1016/ j.applthermaleng.2017.04.155.

[36] E J Q, HAN D, QIU A, ZHU H, DENG Y, CHEN J, ZHAO X, ZUO W, WANG H, CHEN J, PENG Q. Orthogonal experimental design of liquid-cooling structure on the cooling effect of a liquid-cooled battery thermal management system [J]. Applied Thermal Engineering, 2018, 132: 508-520. DOI: https://doi.org/10.1016/j.applthermaleng. 2017.12.115.

[37] ZUO Q, ZHANG D M, E J Q, GONG J. Comprehensive analysis on influencing factors of composite regeneration performance of a diesel particulate filter [J]. Environmental Progress & Sustainable Energy, 2016, 35(3): 882-890. DOI: https://doi.org/10.1002/ep.12264.

[38] E J Q, JIN Y, DENG Y, ZUO W, ZHAO X, HAN D, PENG Q, ZHANG Z. Wetting models and working mechanisms of typical surfaces existing in nature and their application on superhydrophobic surfaces: A review. Advanced Materials Interfaces 2018, 5: 1701052. DOI: https://doi.org/10.1002/ admi.201701052.

[39] ZHAO X, E J Q, WU G, DENG Y, HAN D, ZHANG B, ZHANG Z. A review of studies using graphenes in energy conversion, energy storage and heat transfer development [J]. Energy Conversion and Management, 2019, 184: 581-599. DOI: https://doi.org/10.1016/j.enconman.2019.01.092.

[40] ZHANG B, E J Q, GONG J, YUAN W, ZUO W, LI Y, FU J. Multidisciplinary design optimization of the diesel particulate filter in the composite regeneration process [J]. Applied Energy, 2016, 181: 14-28. DOI: https://doi.org/ 10.1016/j.apenergy.2016.08.051.

[41] ZUO H, LUO Z, GUAN J, WANG Y. Multidisciplinary design optimization on production scale of underground metal mine [J]. Journal of Central South University, 2013, 20(5): 1332-1340. DOI: https://doi.org/10.1007/s11771- 013-1620-x.

(Edited by YANG Hua)

中文导读

基于模糊灰色关联法的微型涡轮发动机氢气/空气催化燃烧特性的影响分析

摘要：为了提高催化燃烧效率，建立了不同过量空气比、进气速度和传热系数下的微型涡轮发动机预混氢/空气燃烧模型。应用模糊灰色关联理论和Chemkin反应机理，分析了入口速度、过量空气系数和传热系数对氢气催化燃烧效率的影响。结果表明，入口速度对氢气的催化燃烧效率有更直观的影响。入口速度越慢，效率越高。最佳过量空气系数在0.94～1.0范围内，过量空气系数超过1.0会降低氢气的催化燃烧效率。传热系数对氢气催化燃烧效率的影响主要体现在过量空气系数超过1.0的情况下，但当传热系数超过4.0时，其影响会下降。进气速度、传热系数和过量空气系数对氢气催化燃烧效率的模糊灰色关联度分别为0.640945、0.633214和0.547892。

关键词：微型涡轮发动机；催化燃烧；氢气；模糊灰色关联理论

Foundation item: Project(51776062) supported by the National Natural Science Foundation of China; Project(201208430262) supported by the National Studying Abroad Foundation Project of the China Scholarship Council

Received date: 2019-05-06; Accepted date: 2019-07-04

Corresponding author: CHEN Jing-wei, PhD, Assistant Professor; Tel: +86-731-88821750; E-mail: chenjingwei@hnu.edu.cn; ORCID: 0000-0001-6497-3635